-
Paper Information
- Next Paper
- Previous Paper
- Paper Submission
-
Journal Information
- About This Journal
- Editorial Board
- Current Issue
- Archive
- Author Guidelines
- Contact Us
American Journal of Economics
p-ISSN: 2166-4951 e-ISSN: 2166-496X
2014; 4(2A): 27-41
doi:10.5923/s.economics.201401.03
Constrained Shrinkage Estimation for Portfolio Robust Prediction
Luis P. Yapu Quispe
Universidade Federal Fluminense
Correspondence to: Luis P. Yapu Quispe, Universidade Federal Fluminense.
| Email: |  |
Copyright © 2014 Scientific & Academic Publishing. All Rights Reserved.
It is possible to reformulate the portfolio optimization problem as a constrained regression. In this paper we use a shrinkage estimator combined with a constrained robust regression and apply it to portfolio robust prediction. Starting with robust estimates  , we solve the constrained optimization problem in order to obtain a robust estimation of the portfolio weights. By varying a shrinkage parameter it is possible to 'interpolate' between the robust and least-squares cases and to find an optimal value of this parameter with the best predictive power. Indeed recurrence of outliers in financial data may require some flexibility aside robustness. In particular we derive a closed formula for linear constrained regression M-estimator and present a procedure intertwining this solution with the shrinkage estimator. Monte Carlo Simulations are used to study the behavior of the optimum values of the shrinkage parameter in some distributions arising in financial data.
, we solve the constrained optimization problem in order to obtain a robust estimation of the portfolio weights. By varying a shrinkage parameter it is possible to 'interpolate' between the robust and least-squares cases and to find an optimal value of this parameter with the best predictive power. Indeed recurrence of outliers in financial data may require some flexibility aside robustness. In particular we derive a closed formula for linear constrained regression M-estimator and present a procedure intertwining this solution with the shrinkage estimator. Monte Carlo Simulations are used to study the behavior of the optimum values of the shrinkage parameter in some distributions arising in financial data.
Keywords: Robust Optimization, Portfolio Prediction, Shrinkage
Cite this paper: Luis P. Yapu Quispe, Constrained Shrinkage Estimation for Portfolio Robust Prediction, American Journal of Economics, Vol. 4 No. 2A, 2014, pp. 27-41. doi: 10.5923/s.economics.201401.03.
Article Outline
1. Introduction
- In the analysis of financial data we often have to implement regression analysis from historical data, the aim being to predict future values of the variables. In this paper we will work mainly with regression techniques applied to portfolio prediction. Classical applications are Markowitz portfolio optimization, Marcowitz (1952), and the Capital Asset Pricing Model (CAPM), developed by many leading economists in the sixties.CAPM is a very used method for estimating the expected return of a portfolio and evaluation of risks. It is a one-factor model:
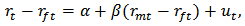 with
with 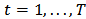 , where
, where 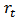 is the rate of return,
is the rate of return, 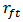 is the risk-free rate,
is the risk-free rate, 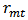 is the rate of return of the market and
is the rate of return of the market and 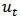 is a random error. Typically the model is fitted by ordinary least squares (OLS).We can put CAPM in the context of the standard linear model:
is a random error. Typically the model is fitted by ordinary least squares (OLS).We can put CAPM in the context of the standard linear model: 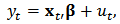 | (1) |
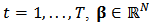 and
and 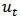 following a density
following a density 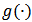 . It is useful to write the model in matrix notation:
. It is useful to write the model in matrix notation: 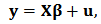 | (2) |
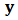 and
and 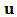 are
are 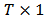 vectors and
vectors and 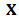 is a
is a 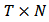 matrix.In spite of well-known shortcomings, CAPM continues tobe an important and widely used model. From a statistical point of view, it is known that standard OLS estimation of
matrix.In spite of well-known shortcomings, CAPM continues tobe an important and widely used model. From a statistical point of view, it is known that standard OLS estimation of 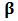 presents several drawbacks. In particular many authors have pointed out its high sensitivity in the presence of outliers and its loss of efficiency in the presence of small deviations from the normality assumption, see, for instance, the books by Huber (1981), Hampel et al.(1986) and Huber and Ronchetti (2009).Robust statistics was developed to cope with the problem arising from the approximate nature of standard parametric models. Indeed robust statistics deals with deviations from the stochastic assumptions on the model and develops statistical procedures which are still reliable and reasonably efficient in a small neighborhood of the model. In particular, several well known robust regression estimators were proposed in the finance literature as alternatives to OLS to estimate
presents several drawbacks. In particular many authors have pointed out its high sensitivity in the presence of outliers and its loss of efficiency in the presence of small deviations from the normality assumption, see, for instance, the books by Huber (1981), Hampel et al.(1986) and Huber and Ronchetti (2009).Robust statistics was developed to cope with the problem arising from the approximate nature of standard parametric models. Indeed robust statistics deals with deviations from the stochastic assumptions on the model and develops statistical procedures which are still reliable and reasonably efficient in a small neighborhood of the model. In particular, several well known robust regression estimators were proposed in the finance literature as alternatives to OLS to estimate 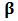 . This issue was already studied by one of the creators of the CAPM, Sharpe (1971). He suggested to use least absolute deviations (
. This issue was already studied by one of the creators of the CAPM, Sharpe (1971). He suggested to use least absolute deviations ( -estimator) instead of OLS (
-estimator) instead of OLS ( -estimator). Chan and Lakonishok (1992) used regression quantiles, linear combinations of regression quantiles, and trimmed regression quantiles. Martin and Simin (2003) proposed to estimate
-estimator). Chan and Lakonishok (1992) used regression quantiles, linear combinations of regression quantiles, and trimmed regression quantiles. Martin and Simin (2003) proposed to estimate 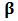 using redescending M-estimators.These robust estimators produce values of
using redescending M-estimators.These robust estimators produce values of 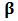 which are more reliable than those obtained by OLS in that they reflect the majority of the historical data and they are not influenced by outlying returns. In fact, robust estimators downweight abnormal observations by means of weights which are computed from the data. Following the discussion in Genton and Ronchetti (2008), robustness is important if the main goal of the analysis is to reflect the structure of the underlying process as revealed by the bulk of the data, but a familiar criticism of this approach in finance is that 'abnormal returns are the important observations', and it has some foundation from the point of view of prediction. Indeed if abnormal returns are not errors but legitimate outlying observations, they will likely appear again in the future and downweighting them by using robust estimators will potentially result in a bias in the prediction of
which are more reliable than those obtained by OLS in that they reflect the majority of the historical data and they are not influenced by outlying returns. In fact, robust estimators downweight abnormal observations by means of weights which are computed from the data. Following the discussion in Genton and Ronchetti (2008), robustness is important if the main goal of the analysis is to reflect the structure of the underlying process as revealed by the bulk of the data, but a familiar criticism of this approach in finance is that 'abnormal returns are the important observations', and it has some foundation from the point of view of prediction. Indeed if abnormal returns are not errors but legitimate outlying observations, they will likely appear again in the future and downweighting them by using robust estimators will potentially result in a bias in the prediction of 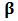 . On the other hand, it is true that OLS will produce in this case unbiased estimators of
. On the other hand, it is true that OLS will produce in this case unbiased estimators of 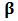 but this is achieved by paying a potentially important price of a large variability in the prediction. Therefore, we are in a typical situation of a trade-off between bias and variance and we can improve upon a simple use of either OLS or a robust estimator. This motivates the use of some form of shrinkage from the robust estimator toward OLS to achieve the minimization of the mean squared error.That discussion on CAPM and in least-squares regression model can also be extrapolated to other models in finance based on analog statistical principles. The topic which interests us here is portfolio optimization, mainly from the point of view of prediction. The goal of portfolio optimization is to find weights
but this is achieved by paying a potentially important price of a large variability in the prediction. Therefore, we are in a typical situation of a trade-off between bias and variance and we can improve upon a simple use of either OLS or a robust estimator. This motivates the use of some form of shrinkage from the robust estimator toward OLS to achieve the minimization of the mean squared error.That discussion on CAPM and in least-squares regression model can also be extrapolated to other models in finance based on analog statistical principles. The topic which interests us here is portfolio optimization, mainly from the point of view of prediction. The goal of portfolio optimization is to find weights 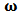 , which represent the percentage of capital to be invested in each asset, and to obtain an expected return with a minimum risk. Brodie et al. (2007) presented a way to express the optimization problem as a multiple regression with constraints. It is therefore possible to perform this regression using robust methods, e.g. M-estimators, least trimmed squares (LTS) or others.Consider a portfolio with
, which represent the percentage of capital to be invested in each asset, and to obtain an expected return with a minimum risk. Brodie et al. (2007) presented a way to express the optimization problem as a multiple regression with constraints. It is therefore possible to perform this regression using robust methods, e.g. M-estimators, least trimmed squares (LTS) or others.Consider a portfolio with 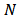 assets and
assets and 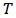 historical returns
historical returns 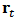 forming the rows of a matrix
forming the rows of a matrix 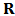 . For an expected return
. For an expected return 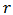 we can solve the following optimization problem:
we can solve the following optimization problem: 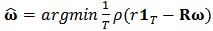 with constraints
with constraints 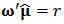 and
and 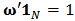 where
where 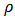 is a penalizing function such as squaring for the OLS estimator or the Huber's function for the robust M-estimator. We use robust estimations
is a penalizing function such as squaring for the OLS estimator or the Huber's function for the robust M-estimator. We use robust estimations 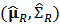 and we solve the optimization problem to obtain a robust estimation for the portfolio weights
and we solve the optimization problem to obtain a robust estimation for the portfolio weights 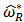 . We then use a shrinkage estimator, see Eq. (24), to 'shrink' towards the OLS estimator and find an optimal value of the shrinkage parameter
. We then use a shrinkage estimator, see Eq. (24), to 'shrink' towards the OLS estimator and find an optimal value of the shrinkage parameter 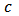 for the measures of predictive power considered in Section 4 of Genton and Ronchetti (2008).We use Monte-Carlo simulations to study the behavior of the optimum values of
for the measures of predictive power considered in Section 4 of Genton and Ronchetti (2008).We use Monte-Carlo simulations to study the behavior of the optimum values of 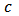 for outlying returns
for outlying returns 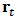 generated by contamination or long-tailed skew-symmetric laws. The simulations give us empirical heuristics for actual applications in robust asset allocation. We consider specially the flexibility of skew-symmetric distributions and study these type of distributions which allow to model return distributions with significant skewness and high kurtosis as is usually the case of hedge funds (see for instance Popova et al. (2003)).From a practical point of view, we implement the methods in the statistical software R. Some tools are already implemented (e.g. MCD estimator) but we have to program some other routines (constrained robust regression, multivariate shrinkage). Depending on the amount of data to be analyzed, execution can be expensive in time, consequently we have to take care about efficiency of the routines mainly if we want to apply Monte Carlo simulations using resampling methods.This paper could be considered as an application in portfolio optimization of the skrinkage estimators studied in Genton and Ronchetti (2008). They only treat the case of estimating beta in CAPM. That estimator have been generalized to multidimensional variables need in portfolio statistical analysis. Gramacy et al. (2008) use specific shrinkage estimators (LASSO and rigde regression) in finance to estimate covariances between many assets with histories of highly variable length (missing data) but they do not the deal with robustness. That work have been developed and extended in Gramacy and Pantaleo (2010), where they consider a Bayesian hierarchical formulation, considering heavy-tailed errors and accounting for estimation risk.The introduction to robust techniques to portfolio optimization is relatively recent compared with the Markowitz foundational paper. Nevertheless the subject have become very active in the last decade. We can mention the works of Vaz-de Melo and Camara (2003), Perret-Gentil and Victoria-Feser (2004), and Welsch and Zhou (2007). All three papers compute the robust portfolio policies in two steps. First, they compute a robust estimate of the covariance matrix of asset returns. Second, they solve the minimum-variance problem where the covariance matrix is replaced by its robust estimate. Recently, Demiguel and Nogales (2009) proposed solving a single nonlinear program, where portfolio optimization and robust estimation are performed in one step. They performed a theoretical study for M-estimators and S-estimators, in addition to a simulation using a mixture of a normal and a deviation distribution. A very recent work of Demiguel et al. (2013) have also implemented a shrinkage strategy both using shrinkage estimators of the moments of asset returns (shrinkage moments), and using shrinkage portfolios obtained by shrinking the portfolio weights directly. We have to remark that in that paper, they use shrinkage by means of a convex combination from the sample estimator (low bias), towards the target estimator (low variance). They use two calibration criteria: the expected quadratic loss minimization criterion, and the Sharpe ratio maximization criterion. We distinguish our work by the fact that use explicitly use a M-estimator as the target of the shrinkage, which enables us to use a more specific shrinkage estimator (from Genton and Ronchetti (2008)) with the calibration parameter is related to Huber's function. In fact varying that parameter allow the shrinkage model to interpolate within the family of robust estimators, the OLS estimator being a limit case for a big value of the parameter
generated by contamination or long-tailed skew-symmetric laws. The simulations give us empirical heuristics for actual applications in robust asset allocation. We consider specially the flexibility of skew-symmetric distributions and study these type of distributions which allow to model return distributions with significant skewness and high kurtosis as is usually the case of hedge funds (see for instance Popova et al. (2003)).From a practical point of view, we implement the methods in the statistical software R. Some tools are already implemented (e.g. MCD estimator) but we have to program some other routines (constrained robust regression, multivariate shrinkage). Depending on the amount of data to be analyzed, execution can be expensive in time, consequently we have to take care about efficiency of the routines mainly if we want to apply Monte Carlo simulations using resampling methods.This paper could be considered as an application in portfolio optimization of the skrinkage estimators studied in Genton and Ronchetti (2008). They only treat the case of estimating beta in CAPM. That estimator have been generalized to multidimensional variables need in portfolio statistical analysis. Gramacy et al. (2008) use specific shrinkage estimators (LASSO and rigde regression) in finance to estimate covariances between many assets with histories of highly variable length (missing data) but they do not the deal with robustness. That work have been developed and extended in Gramacy and Pantaleo (2010), where they consider a Bayesian hierarchical formulation, considering heavy-tailed errors and accounting for estimation risk.The introduction to robust techniques to portfolio optimization is relatively recent compared with the Markowitz foundational paper. Nevertheless the subject have become very active in the last decade. We can mention the works of Vaz-de Melo and Camara (2003), Perret-Gentil and Victoria-Feser (2004), and Welsch and Zhou (2007). All three papers compute the robust portfolio policies in two steps. First, they compute a robust estimate of the covariance matrix of asset returns. Second, they solve the minimum-variance problem where the covariance matrix is replaced by its robust estimate. Recently, Demiguel and Nogales (2009) proposed solving a single nonlinear program, where portfolio optimization and robust estimation are performed in one step. They performed a theoretical study for M-estimators and S-estimators, in addition to a simulation using a mixture of a normal and a deviation distribution. A very recent work of Demiguel et al. (2013) have also implemented a shrinkage strategy both using shrinkage estimators of the moments of asset returns (shrinkage moments), and using shrinkage portfolios obtained by shrinking the portfolio weights directly. We have to remark that in that paper, they use shrinkage by means of a convex combination from the sample estimator (low bias), towards the target estimator (low variance). They use two calibration criteria: the expected quadratic loss minimization criterion, and the Sharpe ratio maximization criterion. We distinguish our work by the fact that use explicitly use a M-estimator as the target of the shrinkage, which enables us to use a more specific shrinkage estimator (from Genton and Ronchetti (2008)) with the calibration parameter is related to Huber's function. In fact varying that parameter allow the shrinkage model to interpolate within the family of robust estimators, the OLS estimator being a limit case for a big value of the parameter 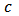 (in fact the OLS is the limit for
(in fact the OLS is the limit for 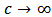 , see Section 4). This is an advantage of our shrinkage strategy compared to convex combination of estimators. Other characteristic of our work is that use use many measures of predictive errors aside the expected quadratic loss. This is specially because in our simulated study we are interested in long-tailed and asymmetric distributions. Other reference which uses skew-symmetric laws as
, see Section 4). This is an advantage of our shrinkage strategy compared to convex combination of estimators. Other characteristic of our work is that use use many measures of predictive errors aside the expected quadratic loss. This is specially because in our simulated study we are interested in long-tailed and asymmetric distributions. Other reference which uses skew-symmetric laws as 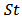 in portfolio optimization is Hu and Kercheval (2010) but they do not involve with shrinkage strategies.The paper is organized as follows, in Section 2 we explain some basic issues concerning the appearance of asymmetric and long-tailed errors and robust regression, then in Section 3 we derive the robust constrained regression model associated to portfolio allocation, in particular we have a closed formula for the shift of the estimator of the parameter vector of the linear model due to linear constraints, see. Eq. 14. In section 4, we present a shrinkage robust estimator and combine it with the constrained robust regression in a procedure for the application in portfolio optimization. Section 5 illustrates the results of the combined procedure using Monte Carlo simulation, first in an ideal standard linear model and then to simulated distributions from contaminated normal and asymmetric-long-tailed laws. Finally Section 7 presents some conclusions of our study.
in portfolio optimization is Hu and Kercheval (2010) but they do not involve with shrinkage strategies.The paper is organized as follows, in Section 2 we explain some basic issues concerning the appearance of asymmetric and long-tailed errors and robust regression, then in Section 3 we derive the robust constrained regression model associated to portfolio allocation, in particular we have a closed formula for the shift of the estimator of the parameter vector of the linear model due to linear constraints, see. Eq. 14. In section 4, we present a shrinkage robust estimator and combine it with the constrained robust regression in a procedure for the application in portfolio optimization. Section 5 illustrates the results of the combined procedure using Monte Carlo simulation, first in an ideal standard linear model and then to simulated distributions from contaminated normal and asymmetric-long-tailed laws. Finally Section 7 presents some conclusions of our study.2. Non-normal Errors and Robust Regression
- Robust statistics is an extension of classical statistics in that it takes into account the possibility of contaminated data or more generally of model misspecification. This theory was firstly developed by Huber (1964) and Hampel (1968).There are many ways to model errors with outlier. For instance we can consider a mixture of a normal distribution
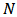 with a large-variance distribution
with a large-variance distribution 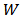 . Let
. Let 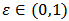 be a number representing the proportion of contamination and define the neighborhood of the parametric distribution
be a number representing the proportion of contamination and define the neighborhood of the parametric distribution 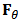 to be the set:
to be the set: 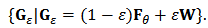 | (3) |
 can be considered as a mixed distribution between
can be considered as a mixed distribution between 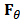 and the contamination distribution
and the contamination distribution 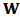 . An estimator is said robust if it remains stable in a neighborhood of
. An estimator is said robust if it remains stable in a neighborhood of 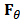 . Often in theoretical studies
. Often in theoretical studies 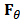 is a multivariate normal distribution in dimension
is a multivariate normal distribution in dimension  :
: 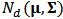 .In standard linear regression theory, least-squares estimator for the parameter
.In standard linear regression theory, least-squares estimator for the parameter 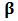 is known to be non-robust. In section 3 we will use M-estimators to find robust estimates of parameters in portfolio allocation. In the context of the linear model (1), the general M-estimators minimize the objective function:
is known to be non-robust. In section 3 we will use M-estimators to find robust estimates of parameters in portfolio allocation. In the context of the linear model (1), the general M-estimators minimize the objective function: 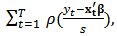 | (4) |
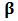 and the loss function
and the loss function 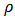 gives the contribution of each residual to the objective function.
gives the contribution of each residual to the objective function. 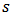 is a scale parameter. Generalizing least-squares minimization, a reasonable
is a scale parameter. Generalizing least-squares minimization, a reasonable 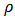 should have the following properties:
should have the following properties: 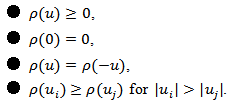 For example, for least-squares estimation we have
For example, for least-squares estimation we have 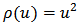 .Let
.Let 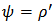 denote the derivative of
denote the derivative of 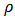 . In this paper we will work with the Huber objective function and its derivative
. In this paper we will work with the Huber objective function and its derivative 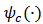 which is called Huber function and is defined by
which is called Huber function and is defined by 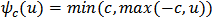 . The tuning constant
. The tuning constant 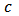 controls the level of robustness. If
controls the level of robustness. If 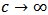 then
then 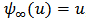 , which corresponds to least-squares estimation.Differentiating the objective function (4) with respect to
, which corresponds to least-squares estimation.Differentiating the objective function (4) with respect to 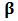 gives the following estimating equations:
gives the following estimating equations: 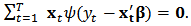 | (5) |
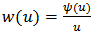 , and denote
, and denote 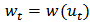 . Then the estimating equation (5) can be written as:
. Then the estimating equation (5) can be written as: 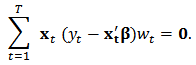 Note that solving these estimating equations can be seen as a weighted least-squares minimization problem with objective function:
Note that solving these estimating equations can be seen as a weighted least-squares minimization problem with objective function: 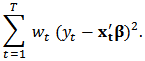 The weights
The weights 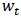 , however, depend upon the residuals, the residuals depend upon the estimated coefficients, and the estimated coefficients depend upon the weights. An iterative solution is therefore required. More details about M-estimators can be found in references, for instance Hampel et al. (1986).At the end of the procedure we obtain the weights
, however, depend upon the residuals, the residuals depend upon the estimated coefficients, and the estimated coefficients depend upon the weights. An iterative solution is therefore required. More details about M-estimators can be found in references, for instance Hampel et al. (1986).At the end of the procedure we obtain the weights 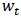 which can be collected in a
which can be collected in a 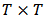 diagonal matrix
diagonal matrix 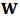 and then we can calculate the M-estimator
and then we can calculate the M-estimator 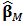 in matrix notation:
in matrix notation: 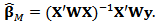
2.1. Resistant Regression (LTS)
- There are other robust techniques of estimation in order to reduce the influence of outliers on the fit of a model. Following the schema of Genton and Ronchetti (2008), we will use the least trimmed squares (LTS) regression.LTS was proposed by Rousseeuw (1985) as another robust alternative to OLS. Let us consider a linear regression model (1). The LTS estimator
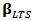 is defined as:
is defined as: 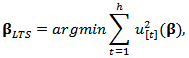 where
where 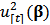 represents the
represents the 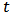 -th order statistics of squared residuals
-th order statistics of squared residuals 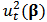 with
with 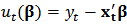 .The trimming constant
.The trimming constant 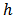 has to satisfy
has to satisfy 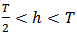 . This constant determines the robustness level of the LTS estimator, since the definition implies that
. This constant determines the robustness level of the LTS estimator, since the definition implies that 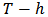 observations with the largest residuals do not have a direct influence on the estimator. The LTS robustness is the lowest for
observations with the largest residuals do not have a direct influence on the estimator. The LTS robustness is the lowest for 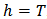 , which corresponds to the least-squares estimator.
, which corresponds to the least-squares estimator.2.2. Asymmetric and Long-tailed Errors
- Often returns in portfolio optimization do not follow a normal distribution and the empirical distribution presents asymmetry and thick tails. In those cases we can propose errors following more flexible laws such as skew-symmetric distributions.Skew-symmetric distributions were explicitly introduced in the literature by Azzalini (1985) with the aim to model departure from normality. Afterwards many generalizations have been introduced and it is nowadays a well studied topic because of its flexibility and theoretical tractability. We can mention the multivariate skew normal distribution studied by Azzalini and Dalla Valle (1996) and the multivariate skew
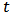 distribution studied in Azzalini and Capitanio (2003). Here we will only define notations.
distribution studied in Azzalini and Capitanio (2003). Here we will only define notations.2.2.1. The Multivariate Skew-normal Distribution
- Given a full-rank
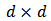 covariance matrix
covariance matrix 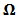 define
define 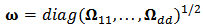 , let
, let 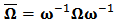 be the corresponding correlation matrix and define vectors
be the corresponding correlation matrix and define vectors 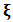 ,
, 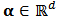 . A
. A 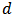 -dimensional random variable
-dimensional random variable 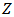 is said to follow a skew-normal distribution if its density function at
is said to follow a skew-normal distribution if its density function at 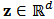 is given by:
is given by: 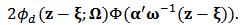 where
where 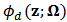 is the
is the 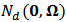
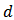 -dimensional normal density at
-dimensional normal density at 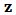 with covariance matrix
with covariance matrix 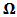 and
and 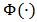 is the
is the 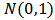 distribution function.We will then write
distribution function.We will then write 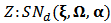 and call
and call 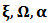 the location, dispersion and the shape or skewness parameters, respectively. If we define a new shape parameter:
the location, dispersion and the shape or skewness parameters, respectively. If we define a new shape parameter: 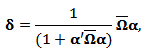 then we can write the expressions of mean vector and covariance matrix:
then we can write the expressions of mean vector and covariance matrix: 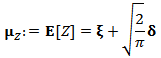
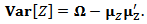
2.2.2. The Multivariate Skew-t Distribution
- In dimension 1, standard t distribution have thick tails and then it allows to model large outliers. In the multivariate case, consider random variables
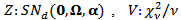 , independent of
, independent of 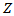 , and the constant vector
, and the constant vector 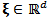 . We define the skew-t distribution as the one corresponding to the transformation:
. We define the skew-t distribution as the one corresponding to the transformation: 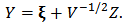 | (6) |
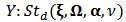 . The parameter
. The parameter 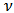 corresponds to the degrees of freedom. A small value of
corresponds to the degrees of freedom. A small value of 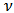 will allow the presence of large outliers and when
will allow the presence of large outliers and when 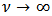 then
then 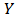 converges to a skew-normal variable.The density function and other formulas and properties can be found in Azzalini and Capitanio (2003). Figures 1 and 2 shows two scatterplots of a 4-dimensional skew-normal variable and skew-t variable. In section 6 we will perform simulations using these distributions in the context of portfolio optimization.
converges to a skew-normal variable.The density function and other formulas and properties can be found in Azzalini and Capitanio (2003). Figures 1 and 2 shows two scatterplots of a 4-dimensional skew-normal variable and skew-t variable. In section 6 we will perform simulations using these distributions in the context of portfolio optimization. 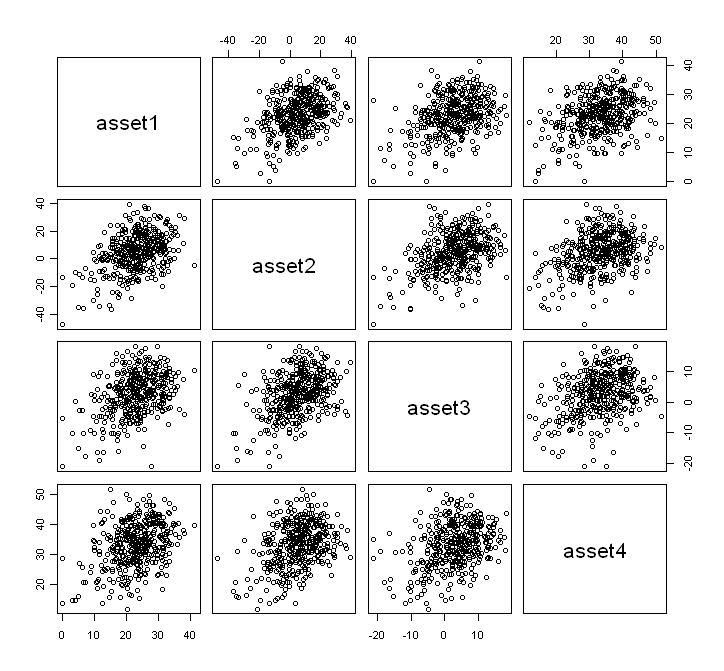 | Figure 1. Scatterplot of a 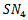 distribution distribution |
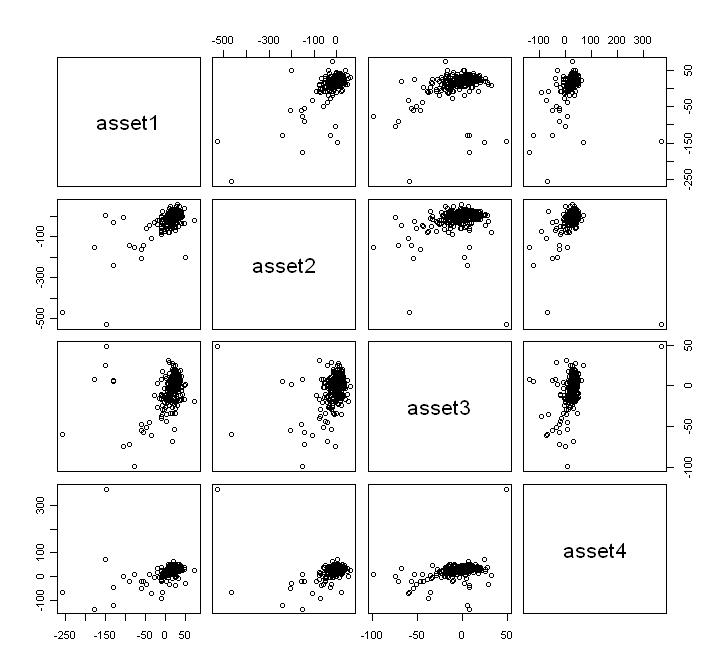 | Figure 2. Scatterplot of a 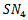 distribution distribution |
3. Portfolio Asset Allocation
- We consider
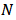 assets and denote their returns at time
assets and denote their returns at time  by
by 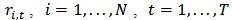 and denote by
and denote by 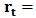
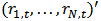 the
the 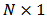 vector of returns at time
vector of returns at time 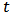 . We assume that
. We assume that 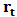 follows a multivariate distribution with
follows a multivariate distribution with 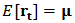 and
and 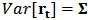 .A portfolio is defined to be a list of weights
.A portfolio is defined to be a list of weights 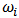 for the assets
for the assets 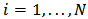 that represent the amount of capital to be invested in each asset. We assume that
that represent the amount of capital to be invested in each asset. We assume that 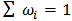 which means that capital is fully invested and denote
which means that capital is fully invested and denote 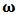 the
the 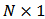 vector of weights.For a given portfolio
vector of weights.For a given portfolio 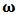 , the expected return and variance are respectively given by:
, the expected return and variance are respectively given by: 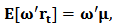 | (7) |
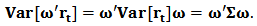 | (8) |
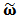 which has minimal variance for a given expected return
which has minimal variance for a given expected return 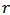 . We can express the problem as:
. We can express the problem as: 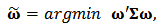 with constraints
with constraints 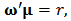 | (9) |
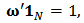 | (10) |
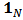 is the
is the 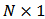 vector in which every entry is equal to 1.We can find in Brodie et al.(2007) a way to model the optimization problem using a multivariate constrained regression. Here we develop details of the derivation.We have
vector in which every entry is equal to 1.We can find in Brodie et al.(2007) a way to model the optimization problem using a multivariate constrained regression. Here we develop details of the derivation.We have 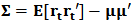 and we can write:
and we can write: 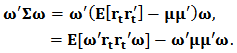 In fact
In fact 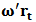 and
and 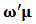 are scalars and using (7) we can write the last expression as:
are scalars and using (7) we can write the last expression as: 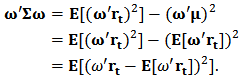 Finally using (7) and the constraint (9) we have:
Finally using (7) and the constraint (9) we have: 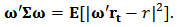 | (11) |
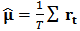 and define
and define 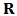 to be the
to be the 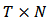 matrix of which the
matrix of which the 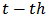 row is
row is 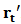 .The empirical version of expression (11) is:
.The empirical version of expression (11) is: 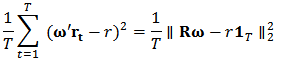 where, for a vector
where, for a vector 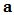 in
in 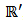 , we use the 2-norm notation:
, we use the 2-norm notation: 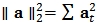 .In summary, we seek to solve the new following optimization problem:
.In summary, we seek to solve the new following optimization problem: 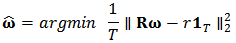 with constraints
with constraints 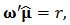 | (12) |
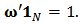 | (13) |
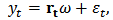
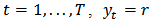 for each
for each 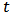 , and with the same constraints (12) and (13).In the optic of robustness we replace the 2-norm by a loss function
, and with the same constraints (12) and (13).In the optic of robustness we replace the 2-norm by a loss function 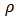 which grows slower, obtaining then the problem:
which grows slower, obtaining then the problem: 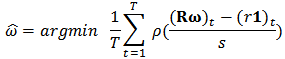 with constraints (12) and (13). As before
with constraints (12) and (13). As before 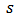 is a scale parameter which should be estimated robustly.We have seen in the last section that the non-constrained M-estimator
is a scale parameter which should be estimated robustly.We have seen in the last section that the non-constrained M-estimator 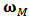 is:
is: 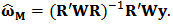 The constrained minimization is solved using Lagrange multipliers. We present the derivation in the next subsection 3.1. In the presence of
The constrained minimization is solved using Lagrange multipliers. We present the derivation in the next subsection 3.1. In the presence of 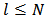 independent linear constrains
independent linear constrains 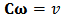 we obtain the constrained M-estimator
we obtain the constrained M-estimator 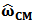 :
: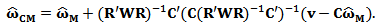 | (14) |
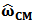 differs from the unconstrained
differs from the unconstrained 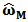 by a function of the quantity
by a function of the quantity 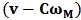 .For our problem, the constraint matrices are:
.For our problem, the constraint matrices are: 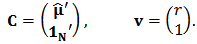 | (15) |
3.1. Constrained Robust Regression
- Using the notation of weighted least-squares regression and in the presence of`the linear constrains
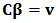 , we can write the Lagrangian:
, we can write the Lagrangian: 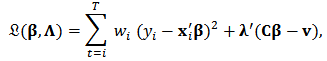 where
where 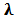 is a
is a 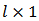 vector of lagrange multipliers,
vector of lagrange multipliers, 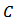 is a
is a 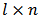 matrix and
matrix and 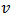 is a
is a 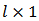 vector.In matrix notation:
vector.In matrix notation: 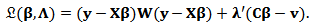 Differentiation with respect to
Differentiation with respect to 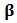 and
and 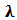 gives the equations:
gives the equations: 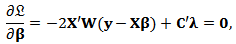
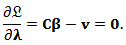 From the first equation we find:
From the first equation we find: 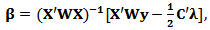 | (16) |
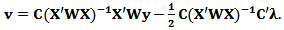 | (17) |
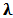 :
: 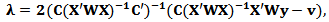 | (18) |
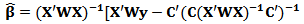
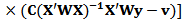 Recall the formula of the non-constrained weighted estimator:
Recall the formula of the non-constrained weighted estimator: 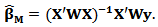 Using this the final expression of the constrained M-estimator can be written:
Using this the final expression of the constrained M-estimator can be written: 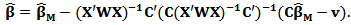 | (19) |
4. Shrinkage Robust Estimator
- Genton and Ronchetti (2008) have defined a robust estimator with shrinkage for the linear model:
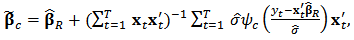 | (20) |
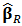 is a robust estimator of
is a robust estimator of 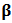 ,
, 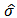 is a robust estimator of scale such as the median absolute deviation (MAD), and
is a robust estimator of scale such as the median absolute deviation (MAD), and 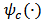 is the Huber's function. As we have seen in Section 2, there are many proposals for the robust estimator
is the Huber's function. As we have seen in Section 2, there are many proposals for the robust estimator 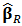 , see for instance Hampel et al. (1986).The tuning constant
, see for instance Hampel et al. (1986).The tuning constant 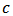 allows us to control the level of shrinkage. If
allows us to control the level of shrinkage. If 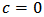 we find the robust estimator
we find the robust estimator 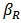 and if
and if 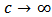 we find the least-squares estimator (OLS).Indeed, in
we find the least-squares estimator (OLS).Indeed, in 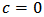 then
then 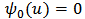 for all values of
for all values of 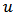 then the rightmost expression in (20) is zero and we have
then the rightmost expression in (20) is zero and we have 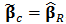 , the robust estimator. On the other side, if
, the robust estimator. On the other side, if 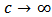 then
then 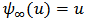 and equation (20) simplifies to:
and equation (20) simplifies to: 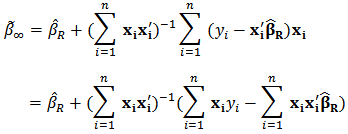 Distributing the expression
Distributing the expression 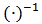 and recognizing the expression of ordinary least-squares estimator
and recognizing the expression of ordinary least-squares estimator 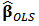 we obtain:
we obtain: 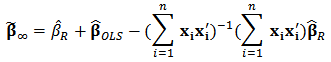 The rightmost expression simplifies and we obtain the limit expression:
The rightmost expression simplifies and we obtain the limit expression: 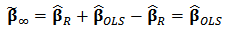 | (21) |
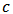 , we can improve the predictive power with respect to least-squares or robust estimators.There are many choices for measuring the quality of the prediction. For normal-distributed errors, the most used criterium is the mean squared error (MSE). However for asymmetric or long-tailed distributions there is not a standard choice.Following Genton and Ronchetti (2008) we consider a family of measures:
, we can improve the predictive power with respect to least-squares or robust estimators.There are many choices for measuring the quality of the prediction. For normal-distributed errors, the most used criterium is the mean squared error (MSE). However for asymmetric or long-tailed distributions there is not a standard choice.Following Genton and Ronchetti (2008) we consider a family of measures: 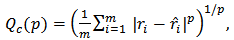 | (22) |
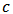 is the shrinkage constant used to estimate the expected returns
is the shrinkage constant used to estimate the expected returns 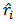 . The case
. The case 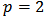 give us the MSE measure. We will be interested in
give us the MSE measure. We will be interested in 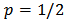 ,
, 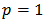 and
and 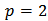 .An important tool to compare the shrinkage estimators is the relative gain:
.An important tool to compare the shrinkage estimators is the relative gain: 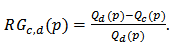 | (23) |
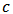 offers more predictive power than the robust estimator
offers more predictive power than the robust estimator 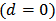 or the OLS estimator
or the OLS estimator 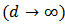 .
.4.1. Application to Portfolio Optimization
- In classical portfolio optimization the first stage in general is to estimate the mean
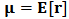 and the covariances
and the covariances 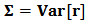 . We can use the historical data and use robust methods to obtain (robust) estimators
. We can use the historical data and use robust methods to obtain (robust) estimators 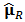 and
and 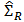 , as explained by Welsch and Zhou (2007). Some methods such as minimum covariance determinant MCD or FAST-MCD are already implemented in statistical software such as R.We have seen in section 3 how to write the classical portfolio optimization problem as a multivariate constrained regression problem and then we considered the robust setting of the problem. In this formulation, we need only a robust estimator of
, as explained by Welsch and Zhou (2007). Some methods such as minimum covariance determinant MCD or FAST-MCD are already implemented in statistical software such as R.We have seen in section 3 how to write the classical portfolio optimization problem as a multivariate constrained regression problem and then we considered the robust setting of the problem. In this formulation, we need only a robust estimator of 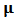 which enters into de constrains matrix
which enters into de constrains matrix 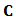 (see formula (15)). We will use the MCD estimator which is already implemented in R.In subsection 3.1 we have found the formula of the robust constrained estimator
(see formula (15)). We will use the MCD estimator which is already implemented in R.In subsection 3.1 we have found the formula of the robust constrained estimator 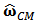 of the portfolio weights. We can now try to apply the shrinkage to
of the portfolio weights. We can now try to apply the shrinkage to 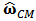 but then the constraints are no more satisfied. We need to use equation (19) one more time but for this we have to recalculate the matrix
but then the constraints are no more satisfied. We need to use equation (19) one more time but for this we have to recalculate the matrix 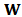 .To be precise we present next a detailed description of the procedure step by step.1. Calculate robust estimator
.To be precise we present next a detailed description of the procedure step by step.1. Calculate robust estimator 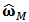 and the regression matrix of weights
and the regression matrix of weights 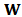 . This can be do with standard routines of statistical software such as R. 2. Use
. This can be do with standard routines of statistical software such as R. 2. Use 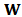 to obtain the constrained estimator
to obtain the constrained estimator 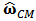 given by formula
given by formula 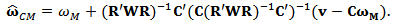 3. Let
3. Let 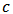 be de tuning constant of shrinkage then calculate the shrinkage estimator:
be de tuning constant of shrinkage then calculate the shrinkage estimator: 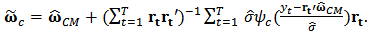 | (24) |
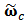 , calculate the new weight matrix
, calculate the new weight matrix 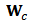 associated with this estimator. We need to calculate standardized residuals
associated with this estimator. We need to calculate standardized residuals 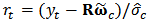 , where
, where 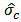 is a robust estimate of scale of the residuals. The matrix
is a robust estimate of scale of the residuals. The matrix 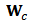 is diagonal with
is diagonal with 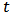 -th component:
-th component: 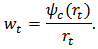 5. Use
5. Use 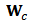 to obtain the shrinkage constrained estimator
to obtain the shrinkage constrained estimator 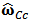 given by formula
given by formula 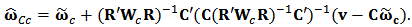 We will use Monte-Carlo simulations to study the behavior of the optimum values of
We will use Monte-Carlo simulations to study the behavior of the optimum values of 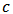 with respect to the different prediction error measures (values of
with respect to the different prediction error measures (values of 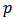 in (22)) for normal and outlying returns
in (22)) for normal and outlying returns 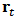 generated by contamination (mixture) and by skew-symmetric laws.A suitable shrinkage could vary in time depending on new available historical data. Anyway at any moment the Monte Carlo simulation can be performed to assess an optimum value of this shrinkage parameter for future estimations of the portfolio weights.We remark that the computation complexity of this procedure depends of the actual implementation of the robust estimation and the matrix operations involved. Supposing that Iteratively Reweighed Least Squares is used in step 1. and only a few iterations are sufficient for convergence, the number of operations involved in all the steps is of the order
generated by contamination (mixture) and by skew-symmetric laws.A suitable shrinkage could vary in time depending on new available historical data. Anyway at any moment the Monte Carlo simulation can be performed to assess an optimum value of this shrinkage parameter for future estimations of the portfolio weights.We remark that the computation complexity of this procedure depends of the actual implementation of the robust estimation and the matrix operations involved. Supposing that Iteratively Reweighed Least Squares is used in step 1. and only a few iterations are sufficient for convergence, the number of operations involved in all the steps is of the order 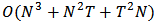 . Taking N (the number of the assets) fixed, the computational complexity becomes
. Taking N (the number of the assets) fixed, the computational complexity becomes 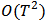 . In consequence, even if it is possible to assess efficiency of Monte Carlo simulation by changing
. In consequence, even if it is possible to assess efficiency of Monte Carlo simulation by changing 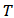 , the computational work increases too, as well as the collateral computational errors. A better study of this remains to be done.
, the computational work increases too, as well as the collateral computational errors. A better study of this remains to be done.5. Monte Carlo Simulations
- In this section we perform simulations based on the paper of Genton and Ronchetti (2008) but in the multivariate case. We will apply the general strategy to portfolio optimization in section 6. We consider the linear model:
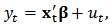 with
with  a
a 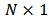 vector,
vector, 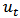 i.i.d.
i.i.d. 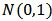 errors and
errors and 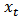 a multivariate normal. As the first example we consider two covariates:
a multivariate normal. As the first example we consider two covariates: 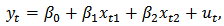 and we take the values
and we take the values 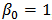 ,
, 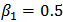 ,
, 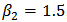 , with
, with 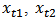 independent
independent 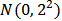 variables.We take
variables.We take 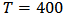 and include
and include 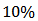 of outliers (contamination) for
of outliers (contamination) for 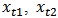 and
and 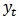 from
from 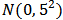 .We use M and LTS estimators,
.We use M and LTS estimators, 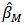 and
and 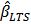 , to obtain robust estimates of
, to obtain robust estimates of 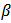 . The estimated values are:
. The estimated values are: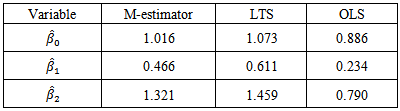 We observe that the OLS estimates are biased and will not be useful for future predictions. We can now use the shrinkage robust estimator (called
We observe that the OLS estimates are biased and will not be useful for future predictions. We can now use the shrinkage robust estimator (called 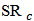 in the sequel),
in the sequel), 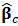 , of
, of 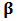 with shrinkage constant
with shrinkage constant 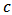 . In order to analyze the effect of outliers, we simulate 1000 training data sets of size
. In order to analyze the effect of outliers, we simulate 1000 training data sets of size 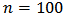 each and containing outliers as indicated. For each sample we estimate
each and containing outliers as indicated. For each sample we estimate 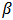 by LTS, OLS and
by LTS, OLS and 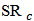 with
with 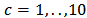 . Figures 4 show boxplots of these estimates over the 1000 simulated training data.The LTS estimators of
. Figures 4 show boxplots of these estimates over the 1000 simulated training data.The LTS estimators of 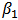 and
and 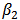 have smaller bias than OLS estimators. We observe that for some values of
have smaller bias than OLS estimators. We observe that for some values of  , the variance of
, the variance of 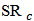 is reduced at the cost of a small increase in bias.Next we investigate the effect of outliers and shrinkage on the prediction of future observations. More precisely, for each of the 1000 estimates
is reduced at the cost of a small increase in bias.Next we investigate the effect of outliers and shrinkage on the prediction of future observations. More precisely, for each of the 1000 estimates 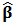 , we compute the predicted values
, we compute the predicted values 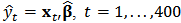 .We consider the measures of quality of prediction with the shrinkage robust estimator
.We consider the measures of quality of prediction with the shrinkage robust estimator 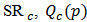 , defined in (22) with three choices for
, defined in (22) with three choices for 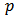 . For
. For 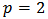 we have the root mean square error (RMSE), for
we have the root mean square error (RMSE), for 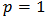 the mean absolute error (MAE) and for
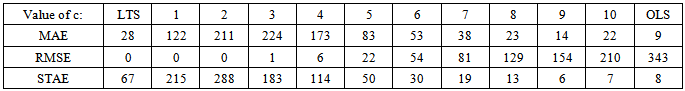
the mean absolute error (MAE) and for 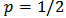 the square root absolute error (STAE).In Table 1 we report the frequencies of selection of a minimum measure of prediction for a range of values of
the square root absolute error (STAE).In Table 1 we report the frequencies of selection of a minimum measure of prediction for a range of values of  over the
over the 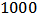 replicates. As can be seen, the optimal
replicates. As can be seen, the optimal 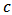 which minimizes a certain measure of quality of prediction is not exclusively concentrated at the 'limit' estimators LTS and OLS. The RMSE measure is related with least squares estimation, consequently OLS is selected most of times. We observe that a shrinkage constant of
which minimizes a certain measure of quality of prediction is not exclusively concentrated at the 'limit' estimators LTS and OLS. The RMSE measure is related with least squares estimation, consequently OLS is selected most of times. We observe that a shrinkage constant of 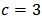 is optimal for MAE and
is optimal for MAE and 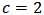 is optimal for STAE. If we are interested in a more precise value of the constant
is optimal for STAE. If we are interested in a more precise value of the constant 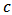 , it is possible to refine the search around the values 2 or 3 of the parameter
, it is possible to refine the search around the values 2 or 3 of the parameter 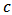 and use a smaller step size.
and use a smaller step size.
|
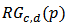 in (23). Denote by
in (23). Denote by 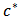 the value of
the value of 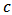 minimizing
minimizing 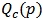 for a fixed
for a fixed 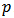 . Figure 3 depicts boxplots over the 1000 replicates of
. Figure 3 depicts boxplots over the 1000 replicates of 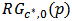 and
and 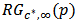 for
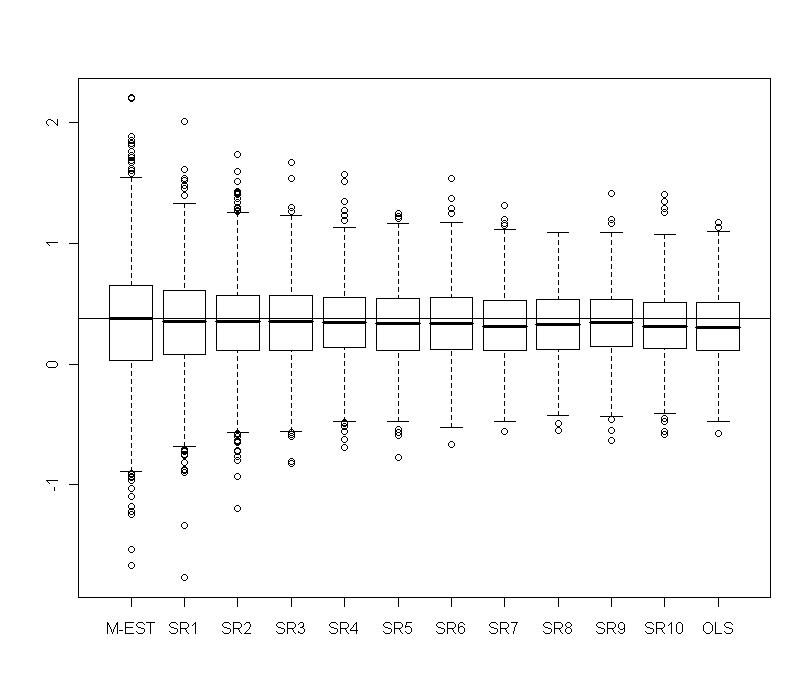
for 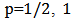 and 2, that is, the relative gain compared to LTS and OLS estimators using STAE, MAE and RMSE respectively.We remark that in terms RMSE, the gains of
and 2, that is, the relative gain compared to LTS and OLS estimators using STAE, MAE and RMSE respectively.We remark that in terms RMSE, the gains of 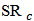 compared to OLS are small. In terms of MAE and STAE, the gains can reach
compared to OLS are small. In terms of MAE and STAE, the gains can reach 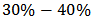 . The gains compared to LTS go rather in the other direction.
. The gains compared to LTS go rather in the other direction.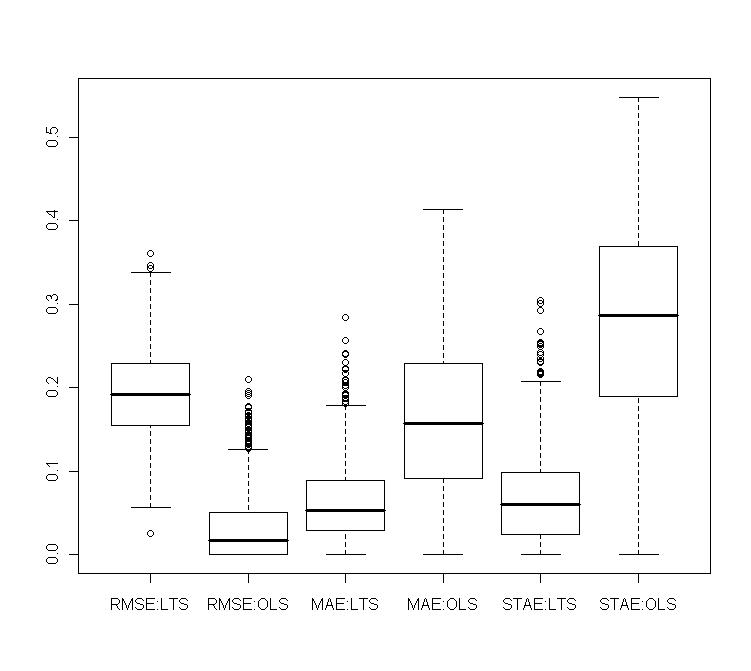 | Figure 3. Normal contamination: relative gains obtained with shrinkage robust estimators compared to LTS and OLS on various measures of prediction (RMSE, MAE, STAE) |
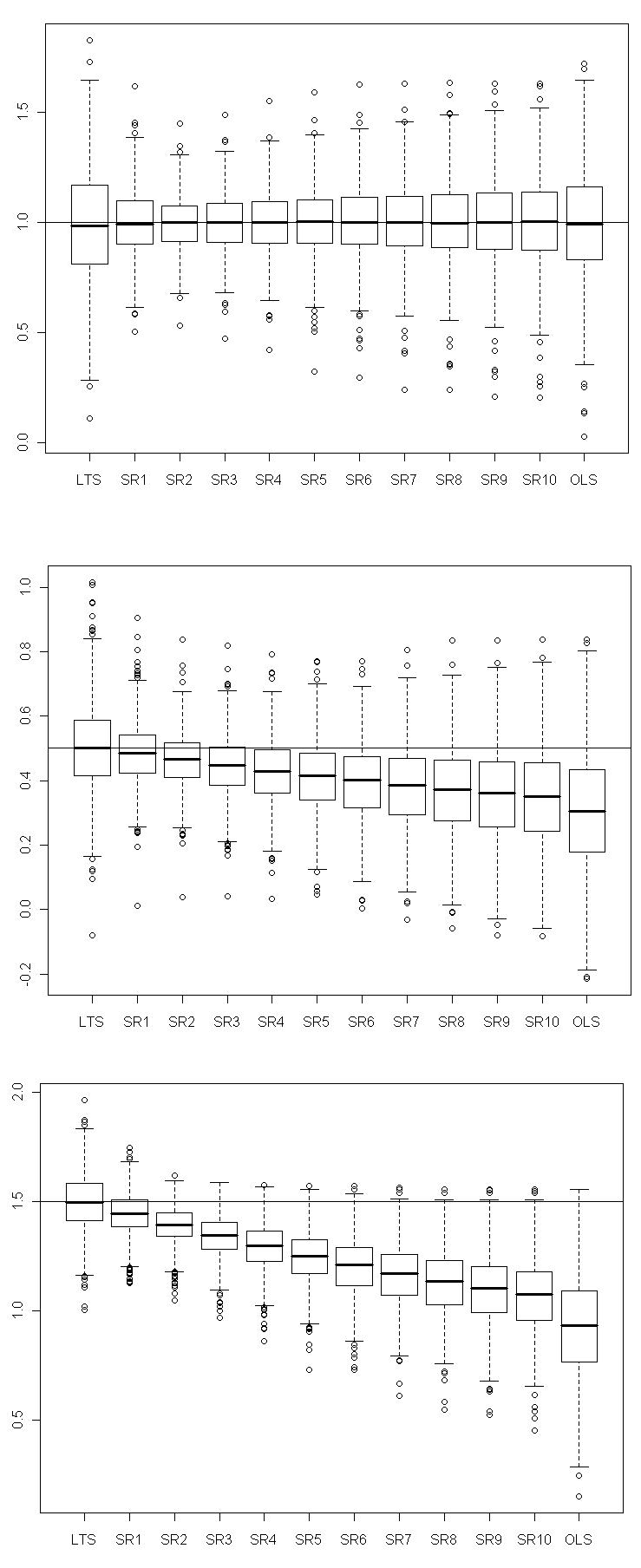 | Figure 4. Normal contamination: boxplots of  and and  for several values of the shrinkage constant for several values of the shrinkage constant 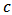 |
6. Application to Portfolio Optimization
- Monte Carlo simulations can give us empirical heuristics for actual applications of the shrinkage robust asset allocation. We have already mentioned the flexibility of skew-symmetric distributions and we will especially study these types of distributions which allow to model return distributions which have significant skewness and high kurtosis such as hedge funds (see for instance Popova et al. (2003)). In this paper we will perform only a empirical study.
6.1. Normal Data with Normal Contamination
- We consider a portfolio of
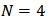 assets. In this example, we suppose that returns are generated from a 4-dimensional normal distribution
assets. In this example, we suppose that returns are generated from a 4-dimensional normal distribution 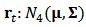 | (25) |
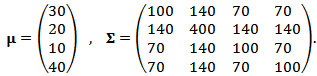 | (26) |
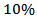 of contamination from
of contamination from 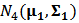 where:
where: 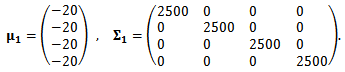 | (27) |
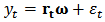 | (28) |
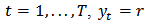 the expected return of the portfolio and with constraints (12) and (13).We simulate a contaminated test sample of size
the expected return of the portfolio and with constraints (12) and (13).We simulate a contaminated test sample of size 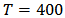 and take
and take 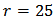 . In practice we don't know the theoretical mean vector
. In practice we don't know the theoretical mean vector 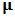 and we only have the matrix
and we only have the matrix 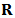 of all returns. As long as we have outliers we need a robust estimate of
of all returns. As long as we have outliers we need a robust estimate of 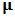 denoted
denoted 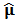 . This can be performed using the method called "fast MCD" developed by Rousseeuw and Van Driessen (1999) which is more general and compute a robust covariance matrix estimator too. The robust estimate of
. This can be performed using the method called "fast MCD" developed by Rousseeuw and Van Driessen (1999) which is more general and compute a robust covariance matrix estimator too. The robust estimate of 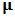 is:
is: 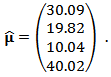 | (29) |
 | Figure 5. OLS-estimates, M-estimates and non-contaminated OLS estimates of 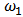 |
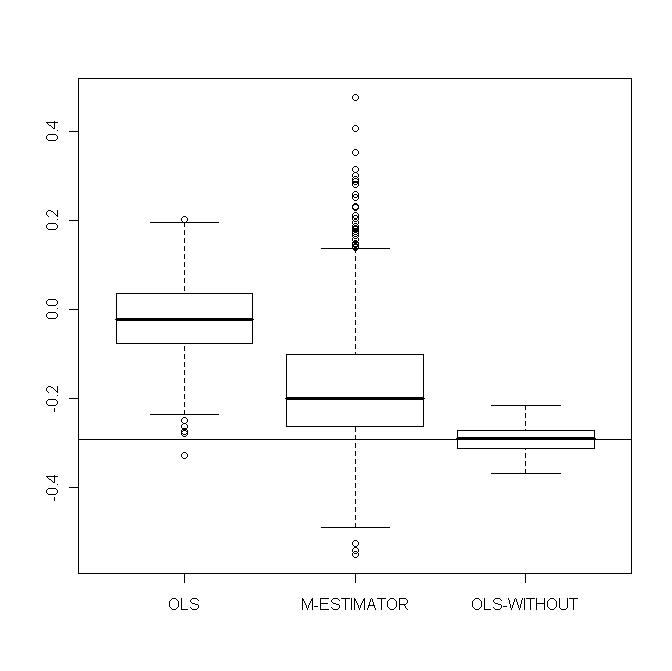 | Figure 6. OLS-estimates, M-estimates and non-contaminated OLS estimates of 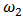 |
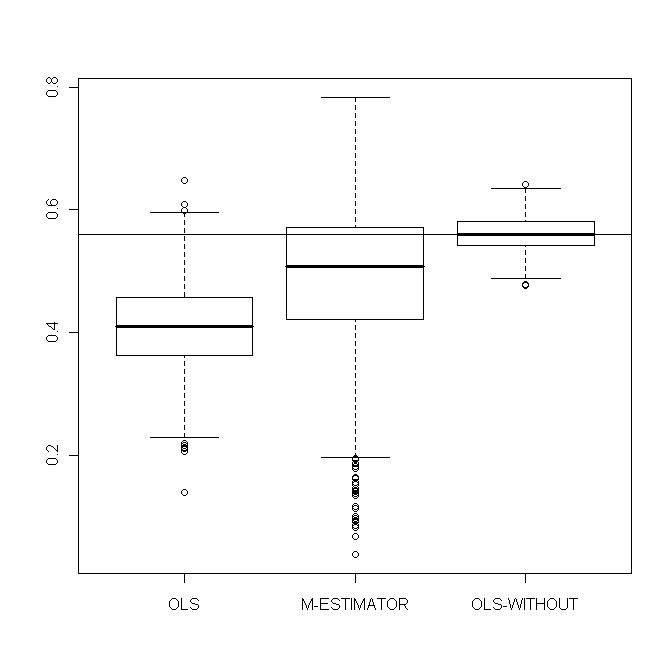 | Figure 7. OLS-estimates, M-estimates and non-contaminated OLS estimates of 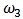 |
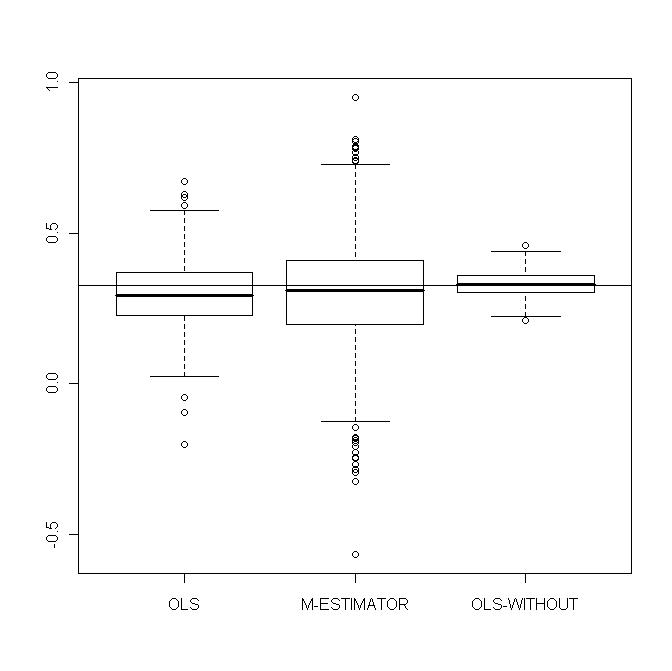 | Figure 8. OLS-estimates, M-estimates and non-contaminated OLS estimates of 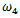 |
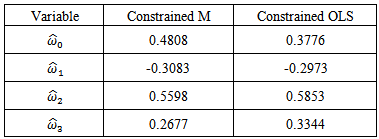 As these values are computed using constraints, it is difficult to assess the standard errors and intervals of confidence analytically. The Monte Carlo simulations will show that the interquartile ranges (IQR) are large and this reflects the instability of classical portfolio optimization.We simulate 1000 training data sets of size
As these values are computed using constraints, it is difficult to assess the standard errors and intervals of confidence analytically. The Monte Carlo simulations will show that the interquartile ranges (IQR) are large and this reflects the instability of classical portfolio optimization.We simulate 1000 training data sets of size 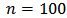 each and containing the same kind of contamination. For each sample we estimate
each and containing the same kind of contamination. For each sample we estimate 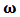 by M-estimator, OLS and
by M-estimator, OLS and 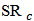 with
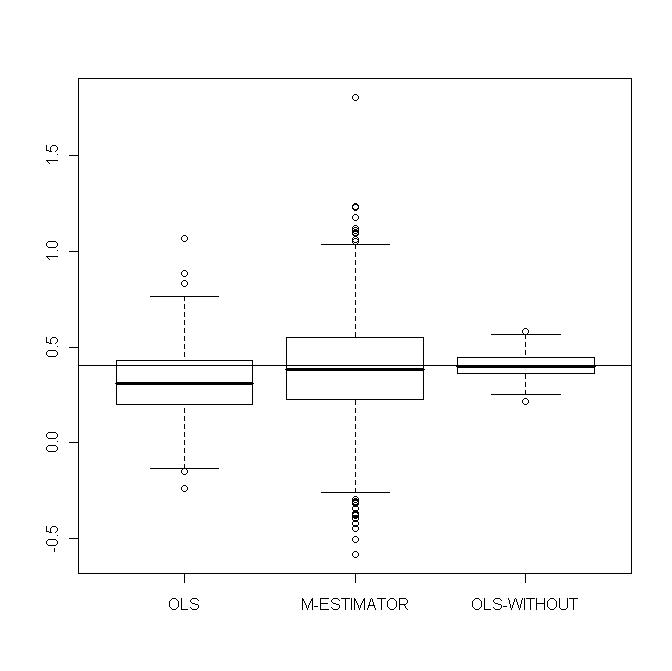
with 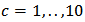 . Figures 10-13 show boxplots of these estimates over the 1000 simulated training data. We observe that the shrinkage estimators of
. Figures 10-13 show boxplots of these estimates over the 1000 simulated training data. We observe that the shrinkage estimators of 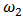 and
and 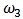 show the biasing effects but those are much less important than in non-constrained regression as presented in section 5. The other effect we can see is that variabilities are large but we observe reduction of variability with some values of the shrinking constant
show the biasing effects but those are much less important than in non-constrained regression as presented in section 5. The other effect we can see is that variabilities are large but we observe reduction of variability with some values of the shrinking constant 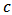 .
.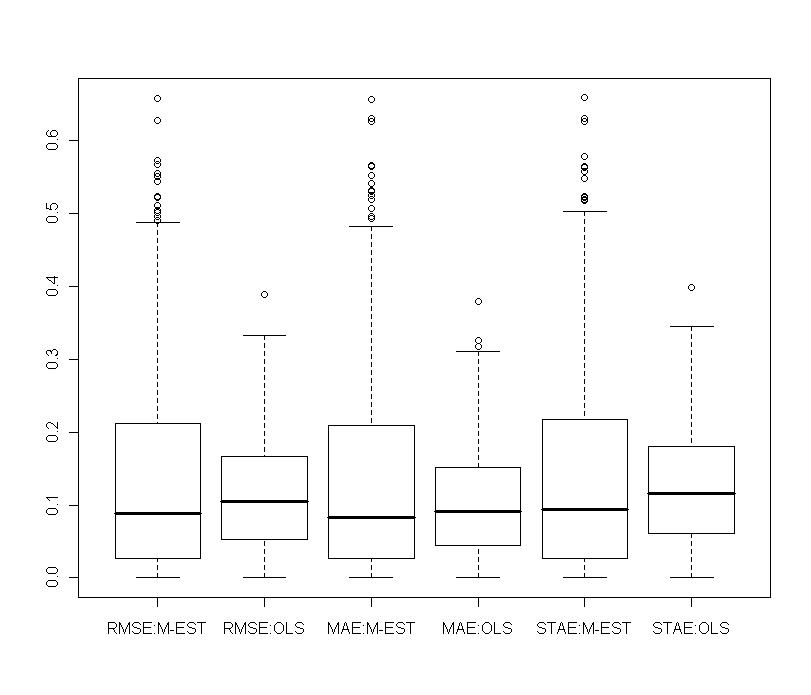 | Figure 9. Portfolio with normal contamination: relative gains obtained with shrinkage robust estimators compared to M-estimator and OLS on various measures of prediction (RMSE, MAE, STAE) |
 | Figure 10. Portfolio with normal contamination: Boxplots of 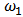 for several values of shrinkage for several values of shrinkage |
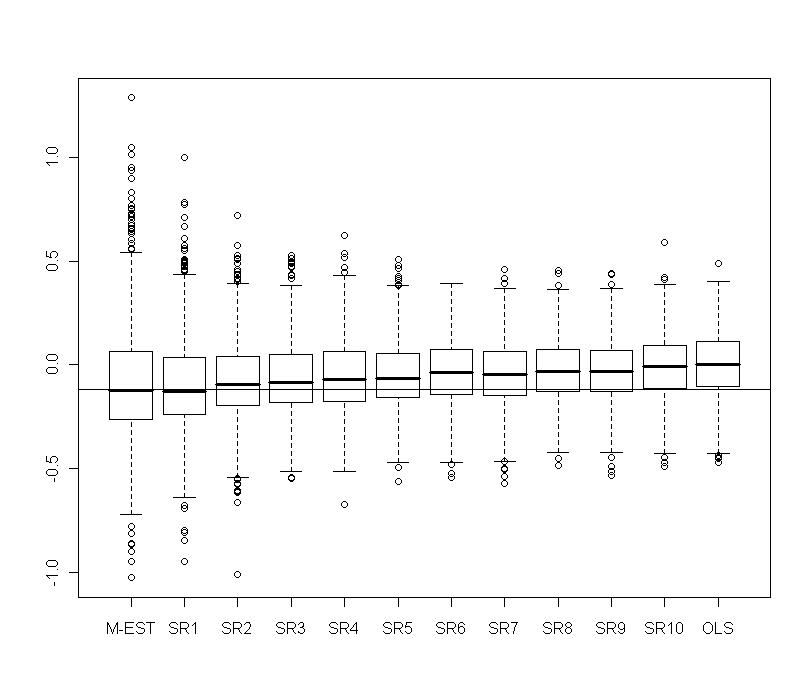 | Figure 11. Portfolio with normal contamination: Boxplots of  for several values of shrinkage for several values of shrinkage |
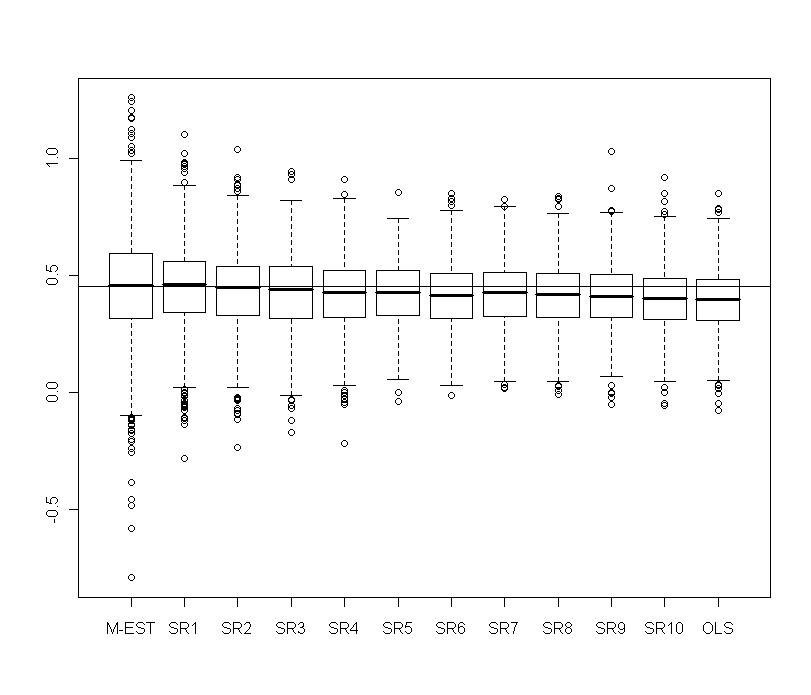 | Figure 12. Portfolio with normal contamination: Boxplots of  for several values of shrinkage for several values of shrinkage |
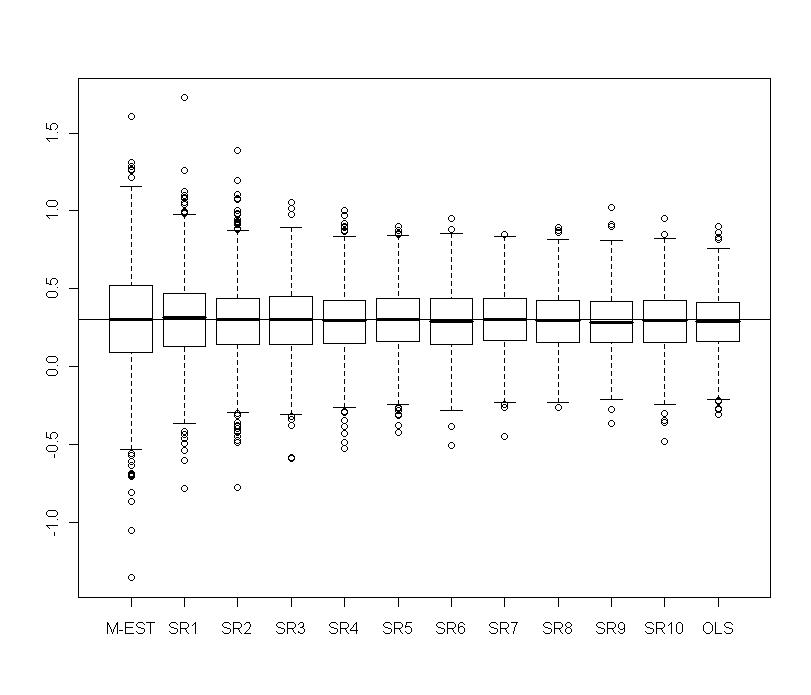 | Figure 13. Portfolio with normal contamination: Boxplots of 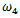 for several values of shrinkage for several values of shrinkage |
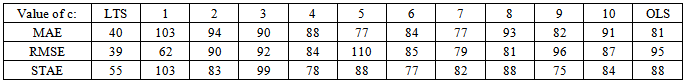
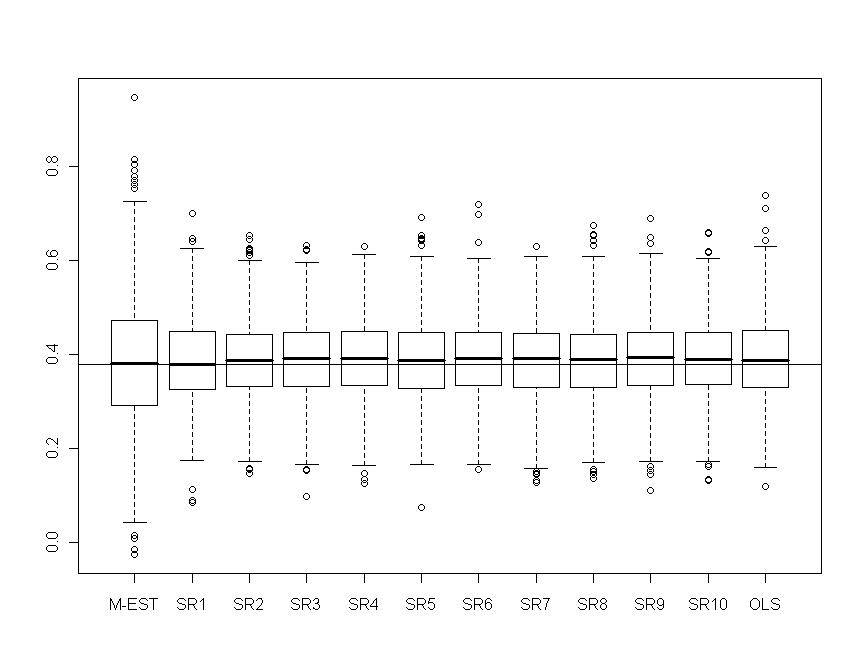
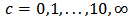 over the 1000 replicates. The optimal
over the 1000 replicates. The optimal 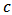 which minimizes the quality of prediction is around 1 for the three measures MAE, RMSE and STAE. At this stage, our simulations showed that with weaker contamination M-estimation is optimal and with less percentage of contamination the optima are very instable.
which minimizes the quality of prediction is around 1 for the three measures MAE, RMSE and STAE. At this stage, our simulations showed that with weaker contamination M-estimation is optimal and with less percentage of contamination the optima are very instable.
|
6.2. Skew-Normal and Skew-t Data
- In this example, we suppose that returns are generated from a 4-dimensional skew-normal distribution:
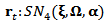 | (30) |
 | (31) |
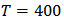 and take
and take 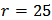 to be the expended return of the portfolio as in the last subsection. The robust estimate of
to be the expended return of the portfolio as in the last subsection. The robust estimate of 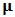 is:
is: 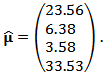 | (32) |
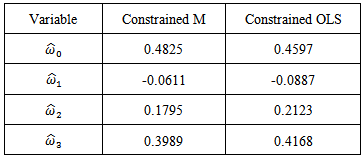 As before we simulate 1000 training data sets of size
As before we simulate 1000 training data sets of size 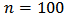 each and containing the same kind of contamination. For each sample we estimate
each and containing the same kind of contamination. For each sample we estimate  by M-estimator, OLS and
by M-estimator, OLS and 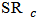 with
with 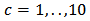 . Figures 15-18 show boxplots of these estimates over the 1000 simulated training data. We observe that the shrinkage estimators of
. Figures 15-18 show boxplots of these estimates over the 1000 simulated training data. We observe that the shrinkage estimators of 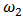 and
and 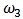 show the biasing effect when
show the biasing effect when 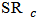 tends to OLS. Now the IRQ are smaller than in the normal contamination case. The IRQ are in general less than 0.1 excepting the IRQ for
tends to OLS. Now the IRQ are smaller than in the normal contamination case. The IRQ are in general less than 0.1 excepting the IRQ for 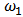 which is around 0.2 for the M-estimator. As before we observe the reduction of variability with some values of the shrinking constant
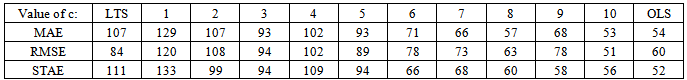
which is around 0.2 for the M-estimator. As before we observe the reduction of variability with some values of the shrinking constant 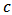 .Now in Table 3 we report the frequencies of selection of a minimum measure of prediction for a range of values of
.Now in Table 3 we report the frequencies of selection of a minimum measure of prediction for a range of values of 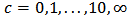 over the 1000 replicates. The optimal
over the 1000 replicates. The optimal 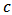 which minimizes the quality of prediction for MAE and STAE is 1, for RMSE it is around 5. Others simulations showed that these optimum values are more or less instable around 2.
which minimizes the quality of prediction for MAE and STAE is 1, for RMSE it is around 5. Others simulations showed that these optimum values are more or less instable around 2.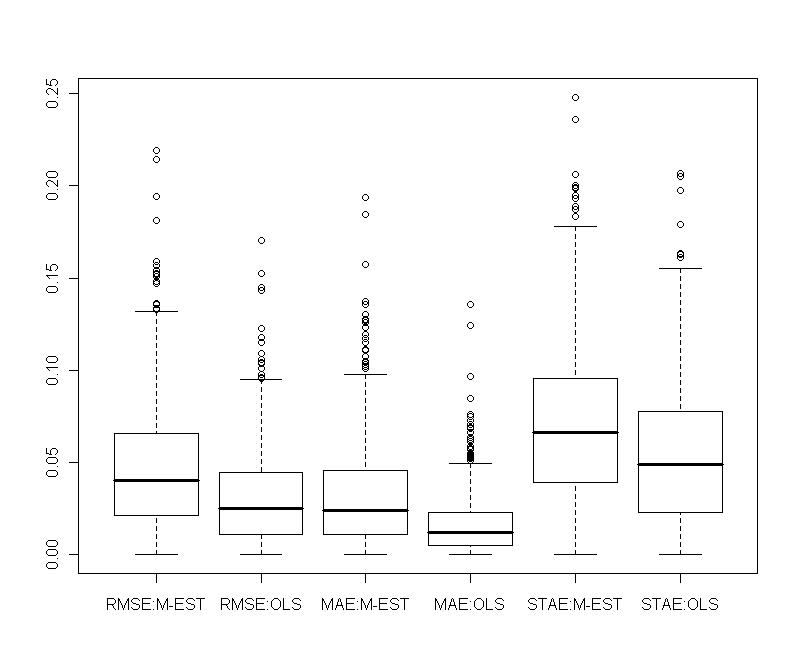 | Figure 14. Portfolio with skew-normal returns: relative gains obtained with shrinkage robust estimators compared to M-estimator and OLS on various measures of prediction (RMSE, MAE, STAE) |
 | Figure 15. Portfolio with skew-normal returns: Boxplots of 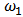 for several values of shrinkage for several values of shrinkage |
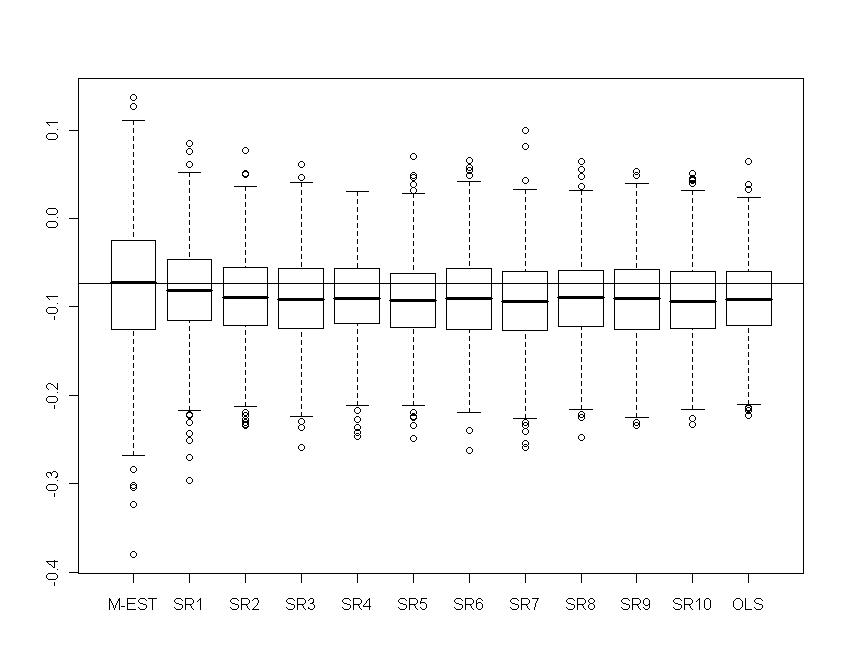 | Figure 16. Portfolio with skew-normal returns: Boxplots of  for several values of shrinkage for several values of shrinkage |
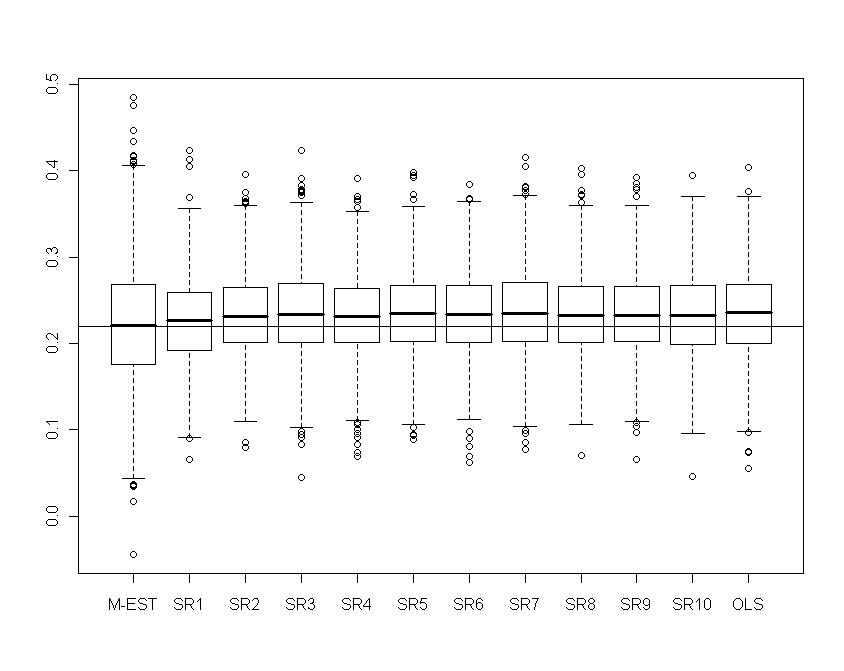 | Figure 17. Portfolio with skew-normal returns: Boxplots of  for several values of shrinkage for several values of shrinkage |
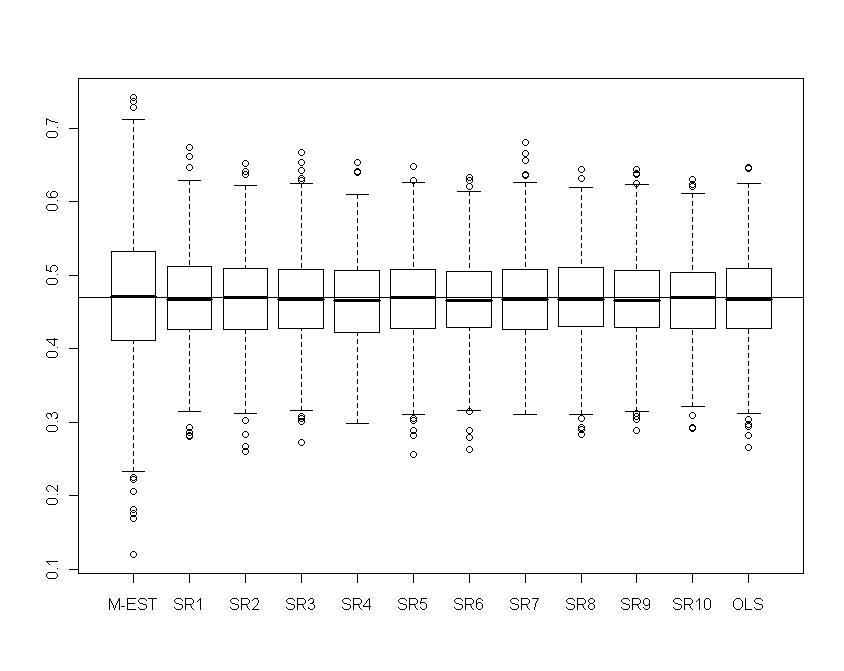 | Figure 18. Portfolio with skew-normal returns: Boxplots of 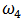 for several values of shrinkage for several values of shrinkage |
|
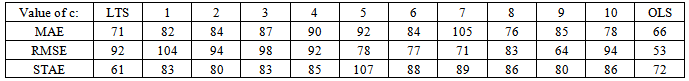
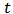 model, using the same parameters as the skew-normal and using 3 degrees of freedom. Small degree of freedom value allows for more outliers. Following Huisman thesis (1999), 3 to 6 degrees of freedom are usual in finalcial data. The optimum value for
model, using the same parameters as the skew-normal and using 3 degrees of freedom. Small degree of freedom value allows for more outliers. Following Huisman thesis (1999), 3 to 6 degrees of freedom are usual in finalcial data. The optimum value for 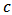 is about 5.
is about 5.
|
7. Conclusions
- In this paper, we have implemented a multivariate version of the shrinkage robust estimators described in Genton and Ronchetti (2008). The aim was to apply the method to the estimation of weights for portfolio optimization. The greatest difficulty was to combine the general method with the constraints which are present in the definition of portfolio optimization. We have seen in Section 6 that the shrinkage constant
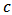 is more instable than in the non-constrained case (Section 5). The origin of the effect is very probably the high instability of the estimation of portfolio weights even with M-estimators. Anyway, the simulations show a optimal shrinkage constant of about 1 for our skew-normal returns and about 5 for our skew-t returns. Location, scale and shape parameters were the same for both laws. We used a skew-t distribution with 3 degrees of freedom, and consequently large outliers were allowed.The Monte Carlo simulations give us only empirical heuristics for actual applications of the robust portfolio allocation. In the future this can be followed by a theoretical study to find more general properties relating asymmetry and shrinkage.
is more instable than in the non-constrained case (Section 5). The origin of the effect is very probably the high instability of the estimation of portfolio weights even with M-estimators. Anyway, the simulations show a optimal shrinkage constant of about 1 for our skew-normal returns and about 5 for our skew-t returns. Location, scale and shape parameters were the same for both laws. We used a skew-t distribution with 3 degrees of freedom, and consequently large outliers were allowed.The Monte Carlo simulations give us only empirical heuristics for actual applications of the robust portfolio allocation. In the future this can be followed by a theoretical study to find more general properties relating asymmetry and shrinkage.ACKNOWLEDGEMENTS
- The core of this work was done while the author was a master student in statistics in the University of Geneva in 2008. The author is very grateful to Prof. Marc Genton and Prof. Elvezio Ronchetti for helpful advises and remarks.
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML