| [1] | M. Nadir, “Etude des propriétés physiques des nanostructures de métaux de transition : CunNim, ” Mémoire de Master, Université A. MIRA – Béjaïa, 2014. |
| [2] | U. S. Okorie, A. N. Ikot, M. C. Onyeaju, and E. O. Chukwuocha, “Bound state solutions of Schrödinger equation with modified Mobius square potential (MMSP) and its thermodynamic properties,” Journal of Molecular Modeling, vol. 24, pp. 289, Aug. 2018. |
| [3] | B. Samia, and S. Salima, “First principles study of the structural, elastic and thermodynamic properties of the cubic perovskite-type SrTiO3,” Modelling, Measurement and Control B, vol. 87, pp. 230-235, Dec. 2018. |
| [4] | S. Aouimer, M. Ameri, D. Bensaid, N. E. Moulay, A. Z. Bouyakoub, F. Z. Boufadi, I. Ameri, and Y. Al-Douri, “The Elastic, Electronic and Thermodynamic Properties of a New Cd Based Full Heusler Compounds — A Theoretical Investigation Using DFT Based FP-LMTO Approach,” ACTA PHYSICA POLONICA A, vol. 136, pp. 127-134, Feb. 2019. |
| [5] | V. Kresin and V. Parkhomenko, “Thermodyanamic Properties of Superconductors With Strong Coupling,” Fizika Tverdogo Tela, vol. 16, pp. 3363, 1974. |
| [6] | M. Xing, B. Li, Z. Yu, and Q. Chen, “Elastic Anisotropic and Thermodynamic Properties of I-4m2-BCN,” ACTA PHYSICA POLONICA A, vol. 129, pp. 1124-1130, Mar. 2016. |
| [7] | R. B. Stephens, “Intrinsic low-temperature thermal properties of glasses,” Physical Review B 13, vol. 852, 1976. |
| [8] | J. Du, R. Devanathan, R. L. Corrales, and W. J. Weber, “First-principles calculations of the electronic structure, phase transition and properties of ZrSiO4 polymorphs,” Computational and Theoretical Chemistry, vol. 987, pp. 62-70, 2012. |
| [9] | I. S. Sedova, L. M. Samorukova, V. A. Glebovitskii, and S. G. Skublov, “Zircon from the Polymigmatites of the Northwestern Ladoga Region: Morphology and Geochemistry,” Geochemistry International, vol. 47, pp. 1050–1066, 2009. |
| [10] | I. Farnan, H. Cho and W. J. Weber, “Quantification of Actinide α-Radiation Damage in Minerals and Ceramics,” Nature, Vol. 445, pp. 190-193, 2007. |
| [11] | J. Chang, Y. Cheng, and M. Fu, “First-principles calculations of thermodynamic properties of superhard orthorhombic β-BC2N,” J. At. Mol. Sci, vol. 1, pp. 243-252, 2010. |
| [12] | Q. Fan, Q. Wei, C. Chai, H. Yan, M. Zhang, Z. Lin,… and D. Zhang, “Structural, mechanical, and electronic properties of P3m1-BCN,” Journal of Physics and Chemistry of Solids, vol. 79, pp. 89-96, 2015. |
| [13] | X. Zhang, Y. Wang, J. Lv, C. Zhu, Q. Li, M. Zhang, ... and Y. Ma, “First-principles structural design of superhard materials, ” The Journal of chemical physics, vol. 138, pp. 114101, 2013. |
| [14] | Q. Fan, Q. Wei, C. Chai, M. Zhang, H. Yan, Z. Zhang,... and D. Zhang, “Elastic and electronic properties of Imm2- and I4¯ m2-BCN,” Computational Materials Science, vol. 97, pp. 6-13, 2015. |
| [15] | S. Q. Wang, “First-principles study of the anisotropic thermal expansion of wurtzite ZnS,” Applied Physics Letters, vol. 88, pp. 061902, 2006. |
| [16] | Y. YU, D. FANG, G. D. ZHAO, and X. L. ZHENG, “AB initio calculation of the thermodynamic properties of wurtzite zns: performance of the lda and gga,” Chalcogenide Letters, vol. 11, pp. 619 – 628, Dec. 2014. |
| [17] | Y. S. Touloukian, “Specific Heat of Non-metallic Elements, Compounds and Mixtures, “Thermophysical Properties of Matter, 1970. |
| [18] | G. Grimvall, “Thermophysical Properties of Materials,” North-Holland, Amsterdam, 1986. |
| [19] | A. Boudali, M. Khodja, B. Amrani, D. Bourbie, K. Amara, and A. Abada, “First-principles study of structural, elastic, electronic, and thermal properties of SrTiO3 perovskite cubic, ” Physics Letters A, vol. 45, pp. 1068-1072, 2009. |
| [20] | J. Kübler, A. R. William, and C. B. Sommers, “Formation and coupling of magnetic moments in Heusler alloys,” Physical Review B, vol. 28, pp.1745, 1983. |
| [21] | K. Trachenko, M. T. Dove, and E. K. H. Salje, “Structural changes in zircon under a-decay irradiation,” PHYSICAL REVIEW B, vol. 65, pp. 180102, May. 2002. |
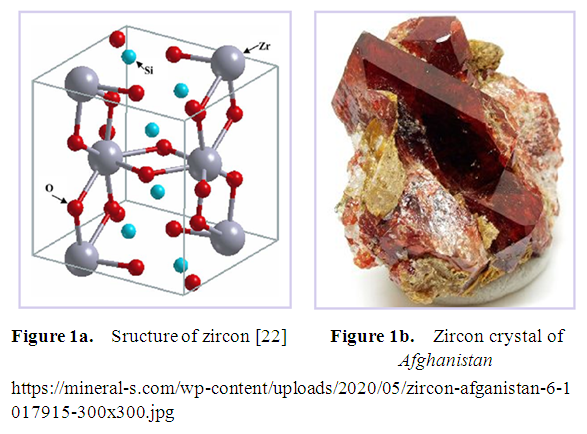
| [22] | R. Terki, G. Bertrand, and H. Aourag, “Full potential investigations of structural and electronic properties of ZrSiO4,” Microelectronic engineering, vol. 81, pp. 514-523, 2005. |
| [23] | K. Trachenko, M. T. Dove, and E. K. H. Salje, “Large swelling and percolation in irradiated zircon,” JOURNAL OF PHYSICS: CONDENSED MATTER, vol. 15, pp. 1-7, Dec. 2002. |
| [24] | J. C. Nipko, and C. K. Loong, “Inelastic neutron scattering from zircon,” Physica B: Condensed Matter, vol. 241, pp. 415-417, 1997. |
| [25] | Y. Luo, and J. C. Ayers, “Experimental measurements of zircon/melt trace-element partition coefficients,” Elsevier Geochimica et Cosmochimica Acta, vol. 73, pp. 3656–3679, Apr. 2009. |
| [26] | N. Daneshvar, M. Maanijou, H. Azizi, and Y. Asahara, “Study of the zircon morphology and internal structures as a tool for constraining magma source: example from granitoid bodies in the northern Sanandaj Sirjan zone (SW Saqqez), ” Geopersia, vol. 8, pp. 245-259, 2018. |
| [27] | Y. Luo, and J. C. Ayers, “Experimental measurements of zircon/melt trace-element partition coefficients,” Geochimica et Cosmochimica Acta, vol. 73, pp. 3656–3679, 2009. |
| [28] | C.B. Grimes, B.E. John, P.B. Kelemen, F.K. Mazdab, J.L. Wooden, M.J. Cheadle, K. Hanghøj, and J.J. Schwartz, “Trace element chemistry of zircons from oceanic crust: A method for distinguishing detrital zircon provenance,” Geology, vol. 35, pp. 643–646, Jul. 2007. |
| [29] | H. Shahbazi, S. Salami, and W. Siebel, “Genetic classification of magmatic rocks from the Alvand plutoniccomplex, Hamedan, western Iran, based on zircon crystal morphology,” Chemie der Erde, vol. 74, pp. 577–584, 2014. |
| [30] | D. Gagnevin, J. S. Daly, Andreas Kronz, “Zircon texture and chemical composition as a guide to magmatic processes and mixing in a granitic environment and coeval volcanic system,” Contrib Mineral Petrol vol. 159 pp. 579–596, 2010. |
| [31] | D. Arya, S. Gupta, S. Kumar, I. Broska, and T. Vaculovic, “Morphology and Chemistry of Zircons from the Paleoproterozoic Cu (±Mo±Au) Hosting Granitoids of Malanjkhand Mine Area, Central India,” JOURNAL GEOLOGICAL SOCIETY OF INDIA, vol. 93, pp. 257-262, Mar. 2019. |
| [32] | A. Gärtner, N. Youbi, M. Villeneuve, A. Sagawe, M. Hofmann, A. Mahmoudi, M. A. Boumehdi, and U. Linnemann, “The zircon evidence of temporally changing sediment transport—the NW Gondwana margin during Cambrian to Devonian time (Aoucert and Smara areas, Moroccan Sahara),” International Journal of Earth Sciences, vol. 106, pp. 1-24, Mar. 2017. |
| [33] | M. Jamshidibadr, “Study of Zircon Crystals: An Implication to Determine of Source and Temperature of Crystallization in Turkeh Dareh Pluton, NW Iran,” Journal of Sciences, Islamic Republic of Iran, vol. 27, pp. 343 – 352, 2016. |
| [34] | G. G. Kenny, L. F. Morales, M. J. Whitehouse, J. A. Petrus, and B. S. Kamber, “The formation of large neoblasts in shocked zircon and their utility in dating impacts,” GEOLOGY, vol. 45, pp. 1003–1006, Nov. 2017. |
| [35] | G. Meinhold, A. Bassis, M. Hinderer, A. Lewin, and J. Berndt, “Detrital zircon provenance of north Gondwana Palaeozoic sandstones from Saudi Arabia,” Geological Magazine, vol. 158, pp. 442–458, 2021. |
| [36] | E. Kovaleva, “Textural Identification of Polycrystalline Magmatic, Tectonically-Deformed, and Shock-Related Zircon Aggregates,” Minerals, vol. 10, pp. 469, May. 2020. |
| [37] | T. Váczi, and L. Nasdala, “Electron-beam-induced annealing of natural zircon: A Raman spectroscopic study,” Phys Chem Minerals, Jun. 2017. |
| [38] | T. Váczi1, L. Nasdala, R. Wirth, M. Mehofer, E. Libowitzky, and T. Häger, “On the breakdown of zircon upon “dry” thermal annealing,” Mineralogy and Petrology, Dec. 2009. |
| [39] | K. BREITER and R. ŠKODA, “Vertical zonality of fractionated granite plutons reflected in zircon chemistry: the Cínovec A-type versus the Beauvoir S-type suite,” GEOLOGICA CARPATHICA, vol. 63, pp. 383—398, Oct. 2012. |
| [40] | V. A. Glebovitsky, I. S. Sedova, S. G. Skublov, L. M. Samorukova, and A. M. Fedoseenko, “Geochemistry of Zircons from Ultrametapmorphic Granitoids in Junction Zone of Aldan Shield and Dzhugdzhur_Stanovoi Fold Region,” Geology Of Ore Deposits, vol. 54, pp. 516–530, 2012. |
| [41] | N. S. Faramarzi, S. Amini, and S. M. Mortazavi, “Geochemical study of zircons and their applications to identify: composition, origin and evolution of rhyolitic parental magma, Hormuz Island (south of Iran),” Journal of Crystallography and Mineraligy, vol. 22, pp. 1-6, Feb. 2015. |
| [42] | H. C. B. Martins, P. P. Simoes, and J. Abreu, “Zircon crystal morphology and internal structures as a tool for constraining magma sources: Examples from northern Portugal Variscan biotite-rich granite plutons,” C. R. Geoscience, vol. 346, pp. 233–243, 2014. |
| [43] | L. AKIN, E. AYDAR, A. K. SCHMITT, H. E. ÇUBUKÇU, “Application of zircon typology method to felsic rocks (Cappadocia, Central Anatolia, Turkey): a zircon crystallization temperature perspective,” Turkish Journal of Earth Sciences, vol. 28, pp. 1-40, 2019. |
| [44] | Z. Salehi, F. Masoudi, M. Razavi, and N. S. Faramarzi, “Estimating of Crystallization Temperature of Mard-Abad (Karaj) Granitic Intrusion Using Mineralogy, Geochemistry and Morphology of Zircon Crystals,” Journal of Sciences, Islamic Republic of Iran, vol. 25, pp. 143 – 155, 2014. |
| [45] | P. E. Montalvo, A. J. Cavosie, C. L. Kirkland, N. J. Evans, B. J. McDonald, C. Talavera, T. M. Erickson, and C. Lugo-Centeno, “Detrital shocked zircon provides first radiometric age constraint (<1472 Ma) for the Santa Fe impact structure, New Mexico, USA,” Geological Society of America Bulletin, vol. 131, pp. 845–863, 2019. |
| [46] | F. Körmann, A. Dick, B. Grabowski, B. Hallstedt, T. Hickel, and J. Neugebauer, “Free energy of bcc iron: Integrated ab initio derivation of vibrational, electronic, and magnetic contributions,” Phys. Rev. B, vol. 78, pp. 033102, Jul. 2008. |
| [47] | L. Kaufman, and H. Bernstein, “Computer calculation of phase diagrams. With special reference to refractory metals,” Refractory Materials. A Series of Monographs. Academic Press Inc; New York, Vol. 4, pp. 344, 1970. |
| [48] | S. Inaba, S. Oda, and K. Morinaga, “Heat capacity of oxide glasses at high temperature region,” Journal of Non-Crystalline Solids, vol. 325, pp. 258-266, Sep. 2003. |
| [49] | A. Gupta, B. T. Kavakbasi, B. Dutta, B. Grabowski, M. Peterlechner, T. Hickel, S. V. Divinski, G. Wilde, and J. Neugebauer, “Low-temperature features in the heat capacity of unary metals and intermetallics for the example of bulk aluminum and Al3Sc,” PHYSICAL REVIEW B, vol. 95, pp. 094307, Mar. 2017. |
| [50] | J. H. Chu, J. G. Analytis, C. Kucharczyk, and I. R. Fisher,” Determination of the phase diagram of the electron-doped superconductor Ba(Fe1−xCox)2As2,” Phys. Rev. B, vol. 79, pp. 014506, Jan. 2009. |
| [51] | K. A. Gschneidner, Jr., and V. K. Pecharsky, “MAGNETOCALORICMATERIALS,” Annual Review of Materials Science, vol. 30 pp. 387-429, Aug. 2000. |
| [52] | M. Khan, K. A. Gschneidner Jr., and V. K. Pecharsky, “Magnetocaloric effects in Er1−xTbxAl2 alloys, ”Journal of Applied Physics, vol.107, pp. 09A904, 2010. |
| [53] | M. Zhang, M. Yu. Efremov, F. Schiettekatte, E. A. Olson, A. T. Kwan, S. L. Lai, T. Wisleder, J. E. Greene, and L. H. Allen, “Size-dependent melting point depression of nanostructures: Nanocalorimetric measurements,” Phys. Rev. B, vol. 62, pp.10548, Oct. 2000. |
| [54] | T. M. Bandhauer, S. Garimella, and T. F. Fuller, “A Critical Review of Thermal Issues in Lithium-Ion Batteries,” Journal of the Electrochemical Society, vol. 158, pp. R1-R25, 2011. |
| [55] | W. Mahmood, M. S. Anwar, and W. Zia, “Experimental determination of heat capacities and their correlation with theoretical predictions,” American Journal of Physics, vol. 79, pp. 1099-1103, 2011. |
| [56] | B. A. Mamedov, E. Eser, H. Koç, and I. M. Askerov, “Accurate Evaluation of the Specific Heat Capacity of Solids and its Application to MgO and ZnO Crystals,” Int J Thermophys, vol. 30, pp. 1048–1054, Jun. 2009. |
| [57] | J. M. Schliesser, and B. F. Woodfield, “Development of a Debye heat capacity model for vibrational modes with a gap in the density of states,” Journal of Physics: Condensed Matter, vol. 27, pp. 285402, 2015. |
| [58] | W. W. Anderson, “An analytic expression approximating the Debye heat capacity function,” AIP Advances, vol. 9, pp. 075108, Jul. 2019. |
| [59] | F. Bernard, S. Kamali-Bernard, and J. Fu, “Approche de modélisation multi-échelle pour la détermination de la chaleur spécifique des matériaux cimentaires,” Academic Journal of Civil Engineering, vol. 35, pp. 164-167, 2009. |
| [60] | W. J. Weber, “Radiation-induced defects and amorphization in zircon,” Journal of Materials Research, vol. 5, pp. 2687 – 2697, Nov. 1990. |
| [61] | J. Ferry and E. Watson, “New thermodynamic models and revised calibrations for the Ti-in-zircon and Zr-in-rutile thermometers,” Contrib. Mineral. Petrol, vol. 154, pp. 429–437, 2007. |
| [62] | R. W. G. Wyckoff, “Crystal Structure,” Wiley, New York, vol. III, 2nd ed., p. 15, 1963. |
| [63] | I.I. Guseinov, and B.A. Mamedov, “Calculation of Integer and Noninteger n-Dimensional Debye Functions Using Binomial Coefficients and Incomplete Gamma Functions,” International Journal of Thermophysics, vol. 28, pp. 1420-1426, Sep. 2007. |
| [64] | J. M. Schliesser, and B. F. Woodfield, “Lattice vacancies responsible for the linear dependence of the low-temperature heat capacity of insulating materials,” Physical Review B, vol. 91, pp. 024109, 2015. |
| [65] | M. Abramowitz, and I. A. Dover “Handbook of Mathematical Functions,” Dover Publications, INC., New Yook, pp. 998, Jun. 1964. |
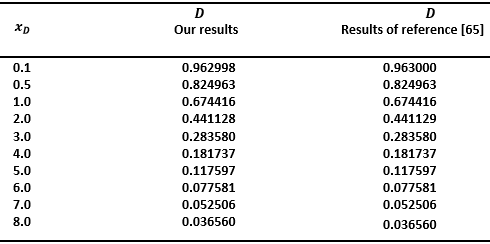
| [66] | B. Fatiha, “Etude des propriétés physiques des matériaux High-k: ZrSiO4, HfSiO4 et ZrGeO4,” Thesis Djillali Liabes University, Algerie, pp. 1-108, Apr. 2017. |



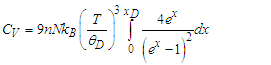
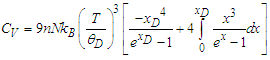
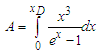
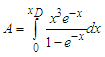
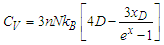
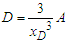
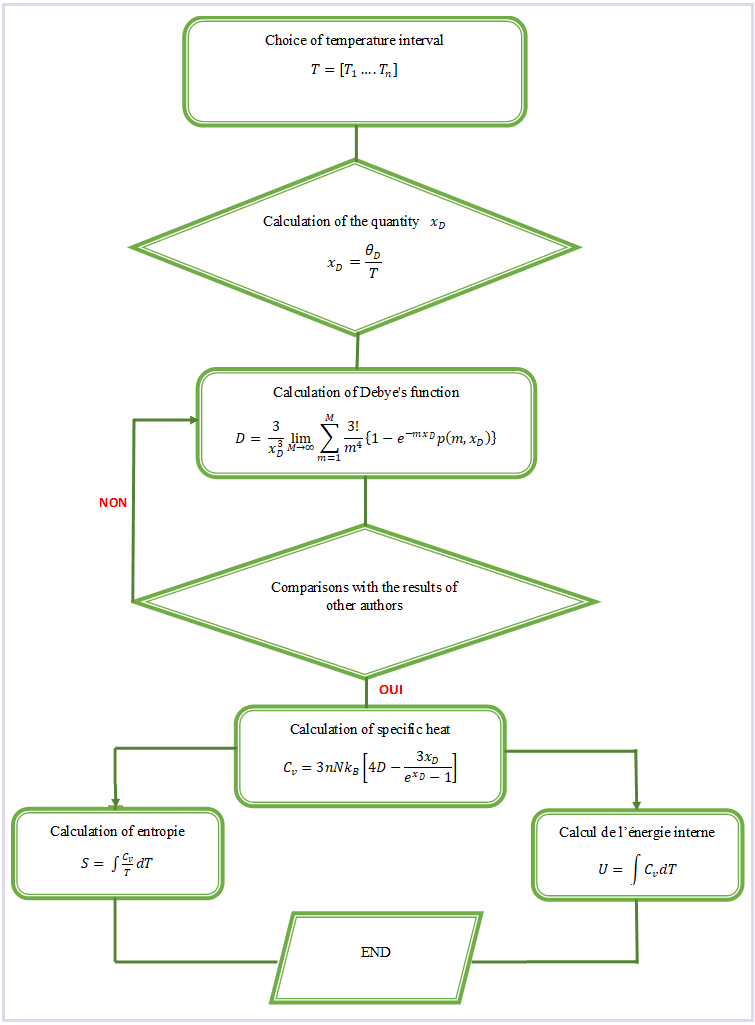
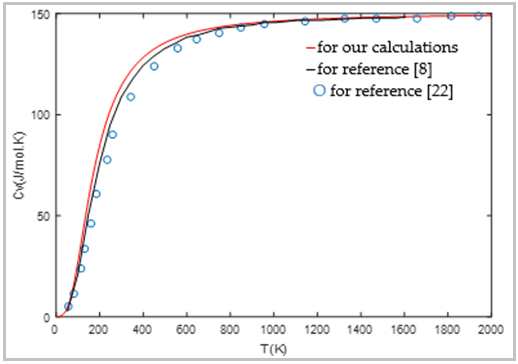
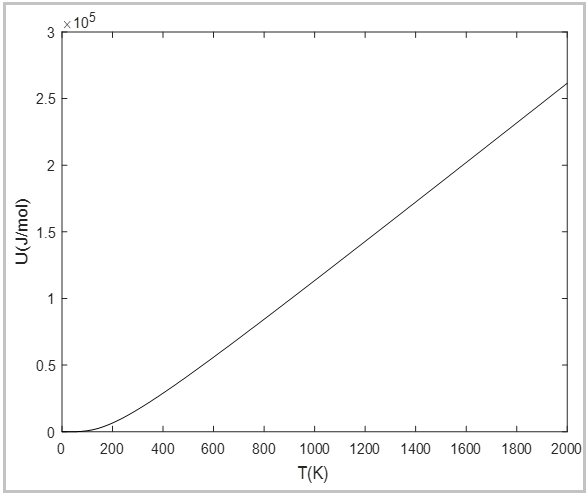
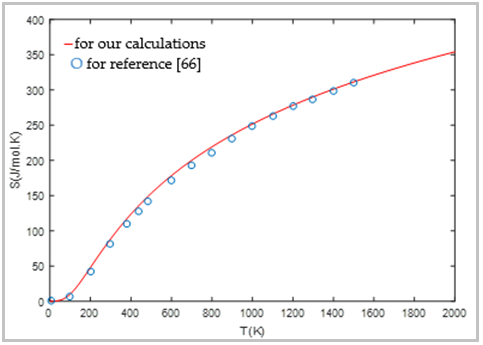
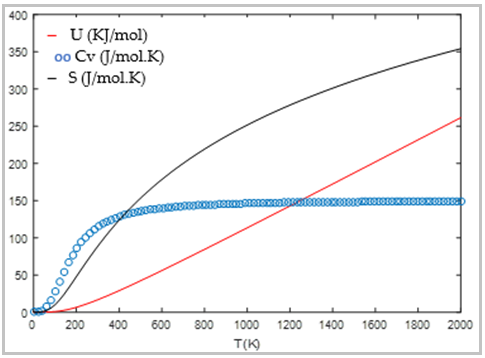
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML