-
Paper Information
- Paper Submission
-
Journal Information
- About This Journal
- Editorial Board
- Current Issue
- Archive
- Author Guidelines
- Contact Us
Modern International Journal of Pure and Applied Mathematics
2017; 1(2): 19-23
doi:10.5923/j.mijpam.20170102.01

First Note on the New Shape of S-convexity
M. R. Pinheiro
IICSE University, DE, USA
Correspondence to: M. R. Pinheiro, IICSE University, DE, USA.
| Email: |  |
Copyright © 2017 Scientific & Academic Publishing. All Rights Reserved.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/

In this note we copy the work we presented on Second Note on the Shape of S-convexity [1], but apply the reasoning to one of the new limiting lines, limiting lines we presented on Summary and Importance of the Results Involving the Definition of S-Convexity [2]. This is about Possibility 1, second part of the definition, that is, the part that deals with negative real functions. We have called it S1 in Summary [2]. The first part has already been dealt with in First Note on the Shape of S-convexity [3]. This paper is about progressing toward the main target: Choosing the best limiting lines amongst our candidates.
Keywords: Analysis, Convexity, Definition,S-convexity, Geometry, Shape
Cite this paper: M. R. Pinheiro, First Note on the New Shape of S-convexity, Modern International Journal of Pure and Applied Mathematics, Vol. 1 No. 2, 2017, pp. 19-23. doi: 10.5923/j.mijpam.20170102.01.
Article Outline
I. Introduction
- In [2], we have decided to keep the name
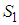 and replace the previous class
and replace the previous class 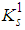 with a new version of it, which would be one of our possible definitions, as for [4].So far, we have:
with a new version of it, which would be one of our possible definitions, as for [4].So far, we have: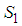 Definition 1. A function
Definition 1. A function 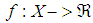 , where
, where 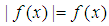 , is told to belong to
, is told to belong to 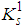 if, for each
if, for each 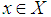 we select, and for all of them, the inequality
we select, and for all of them, the inequality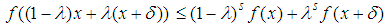
 Definition 2. A function
Definition 2. A function 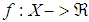 , where
, where  , is told to belong to
, is told to belong to 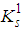 if, for each
if, for each 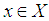 we select, and for all of them, the inequality
we select, and for all of them, the inequality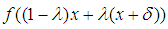
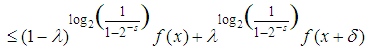
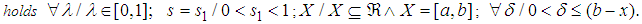 Remark 1. If the inequalities are obeyed in the reverse1 situation by
Remark 1. If the inequalities are obeyed in the reverse1 situation by  , then
, then  is said to be
is said to be  concave.We are now going to concentrate only on the pieces of the definition that we have not yet proven in [3].
concave.We are now going to concentrate only on the pieces of the definition that we have not yet proven in [3].2. Continuity
- We now prove that the function
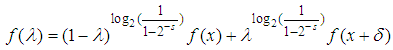 is continuous through a few theorems from Real Analysis.We know that both the sum and the product of two continuous functions are continuous functions (see [5]). Notice that
is continuous through a few theorems from Real Analysis.We know that both the sum and the product of two continuous functions are continuous functions (see [5]). Notice that 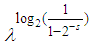 is continuous, given that
is continuous, given that  and
and  .
. 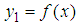 and
and 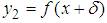 are constants, therefore could be seen as constant functions, which are continuous functions.
are constants, therefore could be seen as constant functions, which are continuous functions. 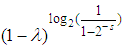 is continuous due to the allowed values for
is continuous due to the allowed values for 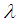 and
and 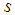 .Notice that
.Notice that 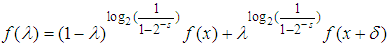 is
is  , that is, is smooth (see [6]).Because the coefficients that form the convexity limiting line use 100% split between the addends and form straight lines and the coefficients that form the
, that is, is smooth (see [6]).Because the coefficients that form the convexity limiting line use 100% split between the addends and form straight lines and the coefficients that form the  convexity limiting line use more than 100% or 100% split between the addends, given that
convexity limiting line use more than 100% or 100% split between the addends, given that 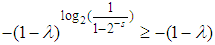 and
and 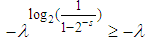 (we are using the negativity of the function here), we know that the limiting line for
(we are using the negativity of the function here), we know that the limiting line for 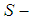 convexity lies always above or over the limiting line for convexity, and contains two points that always belong to both the convexity and the
convexity lies always above or over the limiting line for convexity, and contains two points that always belong to both the convexity and the 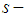 convexity limiting lines (first and last or
convexity limiting lines (first and last or 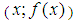 and (
and (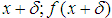 ).We now have then proved, in a definite manner, also in the shape of a paper, that our limiting line for the
).We now have then proved, in a definite manner, also in the shape of a paper, that our limiting line for the 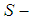 convexity phenomenon is smooth, continuous, and located above or over the limiting line for the convexity phenomenon. Our
convexity phenomenon is smooth, continuous, and located above or over the limiting line for the convexity phenomenon. Our 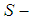 convexity limiting line should also be concave when seen from the limiting convexity line for the same points
convexity limiting line should also be concave when seen from the limiting convexity line for the same points 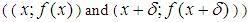 (taking away the cases in which
(taking away the cases in which 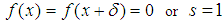 ).
).3. Arc Length
- Arc length is defined as the length along a curve,
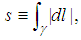 where dl is a differential displacement vector along a curve
where dl is a differential displacement vector along a curve 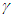 (see [7]).In Cartesian coordinates, that means that the Arc Length of a curve is given by
(see [7]).In Cartesian coordinates, that means that the Arc Length of a curve is given by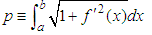 whenever the curve is written in the shape
whenever the curve is written in the shape 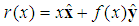 .Our limiting curve for
.Our limiting curve for  convexity could be expressed as a function of
convexity could be expressed as a function of  in the following way:
in the following way: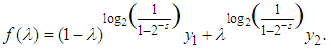 In deriving the above function in terms of
In deriving the above function in terms of 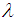 , we get:
, we get: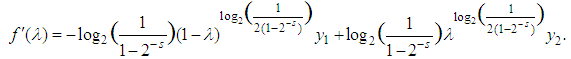 With this, our arc length formula will return:
With this, our arc length formula will return: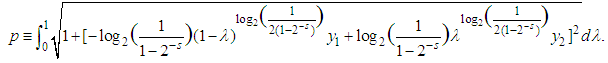 We will make use of a constant function, and we know that every constant function is convex, therefore also
We will make use of a constant function, and we know that every constant function is convex, therefore also 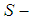 convex (for every allowed value of s), to study the limiting line for
convex (for every allowed value of s), to study the limiting line for 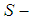 convexity better.We choose
convexity better.We choose 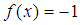 to work with (this function is suitable because
to work with (this function is suitable because 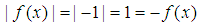 ).We then have:
).We then have: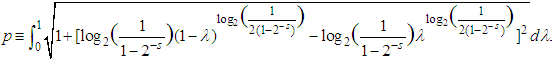 Notice that
Notice that 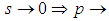 indeterminate and
indeterminate and 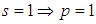 .Notice that 0.25 will become 0.09 when raised to
.Notice that 0.25 will become 0.09 when raised to 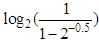 and its supplement through the formula
and its supplement through the formula 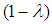 , 0.75, will become 0.6.In convexity, our results would have been 0.25 and 0.75 instead, that is, 64% and 20% less in negativity is gotten with
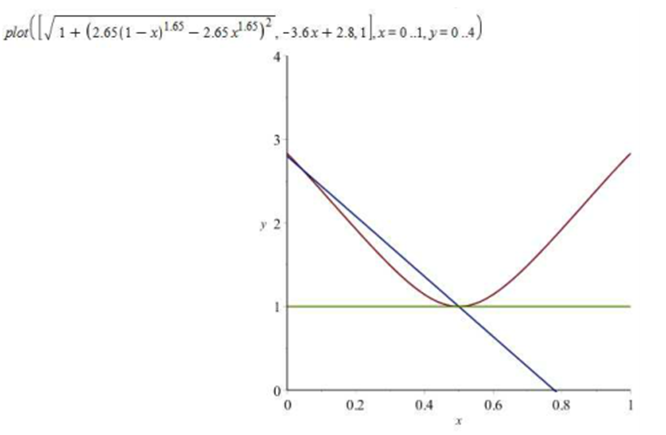
, 0.75, will become 0.6.In convexity, our results would have been 0.25 and 0.75 instead, that is, 64% and 20% less in negativity is gotten with 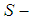 convexity, respectively.We now calculate the area under the curve by hand because Maple could not compute it inside of an acceptable time interval: We notice that the vertex of the graph that represents the function we are interested in is located on (0.5; 1) in both cases. We can then draw a triangle on both sides of the space we are interested in, and find an approximation to our target area. After that, we can subtract an approximation to the piece of the triangle we cannot consider. From eye observation, we can tell that the second leaf is about half of the first, so that whatever we put for the first, we just halve it for the second.
convexity, respectively.We now calculate the area under the curve by hand because Maple could not compute it inside of an acceptable time interval: We notice that the vertex of the graph that represents the function we are interested in is located on (0.5; 1) in both cases. We can then draw a triangle on both sides of the space we are interested in, and find an approximation to our target area. After that, we can subtract an approximation to the piece of the triangle we cannot consider. From eye observation, we can tell that the second leaf is about half of the first, so that whatever we put for the first, we just halve it for the second.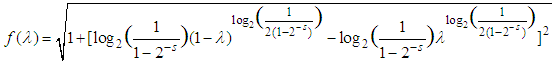
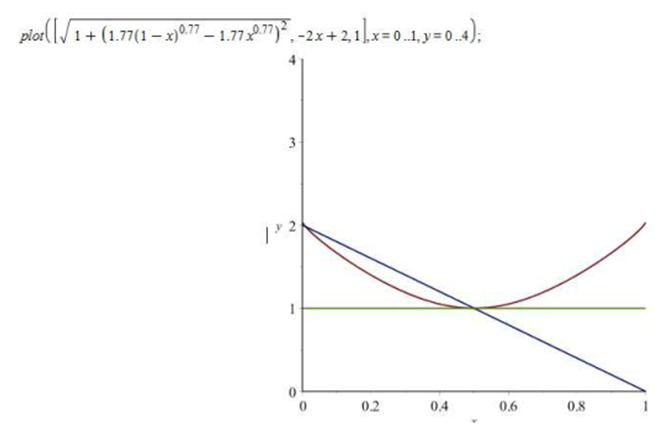 | Figure 1. Maple Plot, s=0.5 |
 | Figure 2. Maple Plot, s=0.25 |
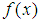 is replaced with -1 in the arc length formula:
is replaced with -1 in the arc length formula:
4. Maximum Height
- The maximum height of the
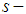 convexity limiting curve is reached when
convexity limiting curve is reached when 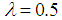 if
if 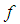 is constant and
is constant and 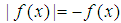 because the first derivative of the function describing the limiting line gives us zero for
because the first derivative of the function describing the limiting line gives us zero for 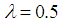 and changes sign from positive to negative.
and changes sign from positive to negative.5. Comparison of Results
- Our calculations in [3] had made use of
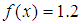 instead of
instead of 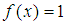 . Today we used Maple and
. Today we used Maple and 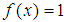 for when we have modulus equating function and exponent being only s. Our results were:
for when we have modulus equating function and exponent being only s. Our results were: When we used exponent
When we used exponent 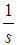 instead for the case in which the modulus does not equate the function, we got, for
instead for the case in which the modulus does not equate the function, we got, for 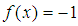 , this time through Maple, the following table:
, this time through Maple, the following table:
6. Conclusions
- Our studies on length were, so far, based on rough approximations. In this paper, we could use Maple to build the table of lengths for two situations, and those were our best matches in terms of algebraic form and all else. We measured length for the case of modulus equating function and exponent s and for the case modulus not equating function and exponent
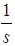 .We have studied alternatives to the exponent
.We have studied alternatives to the exponent 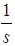 because it seemed that there was non-negligible discrepancy between the case in which the modulus equates the function and the case in which it doesn't.The replacement has been thought of because ideally we would have the same height all the way through for the limiting curve in both the negative and the non-negative case, but such fact was not being verified with the previous definition, as seen on [4].We definitely needed to keep the points where
because it seemed that there was non-negligible discrepancy between the case in which the modulus equates the function and the case in which it doesn't.The replacement has been thought of because ideally we would have the same height all the way through for the limiting curve in both the negative and the non-negative case, but such fact was not being verified with the previous definition, as seen on [4].We definitely needed to keep the points where 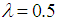 at the same height.That is why our only possible alternative would be the limiting line containing the log, as seen in [4].Upon considering the figures attained for
at the same height.That is why our only possible alternative would be the limiting line containing the log, as seen in [4].Upon considering the figures attained for 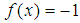 in the system containing the exponent s for the case in which the modulus of the function does not equate the function, and comparing those with the figures we get for
in the system containing the exponent s for the case in which the modulus of the function does not equate the function, and comparing those with the figures we get for 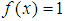 and exponent s, studied here under the light of Maple, we notice that the values are compatible enough.Maple could not calculate the integral for the case in which we have the log, but our rough approximations make us think that the figures are compatible enough and our rough approximations gave us figures that were similar enough for the case in which the exponent was
and exponent s, studied here under the light of Maple, we notice that the values are compatible enough.Maple could not calculate the integral for the case in which we have the log, but our rough approximations make us think that the figures are compatible enough and our rough approximations gave us figures that were similar enough for the case in which the exponent was 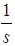 when the results attained with Maple are considered.Our best choice could be
when the results attained with Maple are considered.Our best choice could be 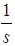 and s because the algebraic form is nicer, the calculations are easier (Maple could calculate for
and s because the algebraic form is nicer, the calculations are easier (Maple could calculate for 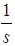 for instance), and the match is instinctive.We started thinking of a new shape because of the discrepancies found in terms of the tables involving both the negative and the non-negative functions for these shapes however.If we consider basic values, such as
for instance), and the match is instinctive.We started thinking of a new shape because of the discrepancies found in terms of the tables involving both the negative and the non-negative functions for these shapes however.If we consider basic values, such as 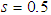 ,
, 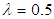 ,
, 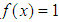 , and
, and 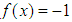 , the results of our calculations show us that we should adopt s and log as exponents instead.We worry about the distance encountered between the limiting line for S-convexity and the line for convexity, as seen in [4].When we write as exponent, we should have
, the results of our calculations show us that we should adopt s and log as exponents instead.We worry about the distance encountered between the limiting line for S-convexity and the line for convexity, as seen in [4].When we write as exponent, we should have 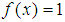 and this distance should be the same as the distance between
and this distance should be the same as the distance between 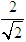 and 1. This distance is approximately 0.41. We can also call it
and 1. This distance is approximately 0.41. We can also call it 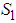 now, thanks to [2]. When we have exponent
now, thanks to [2]. When we have exponent  , we should have
, we should have 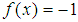 , and the distance should be the same as the distance between
, and the distance should be the same as the distance between 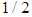 and 1, and that is 0.5.When we write the log as exponent, and consider the negative function, we get 0.41 once more.In this case, the ideal couple is s and log or the New Negative from [4]. However, we must still analyze the case New Positive in full.
and 1, and that is 0.5.When we write the log as exponent, and consider the negative function, we get 0.41 once more.In this case, the ideal couple is s and log or the New Negative from [4]. However, we must still analyze the case New Positive in full.Notes
- 1. Reverse here means `>', not `≥'.2. The first value for Arc Length in the table has been attained through simple substitution in the formula.
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML