1. Introduction

Ceramic-lined cannons have gained attention in recent years[1] due to increased and more sustained combustion gas temperatures used to attain improved cannon performance. Thermal damage of the electroplated chromium used on a cannon bore has become a significant erosion failure mode for today’s gun barrels[2]. The higher elevated-temperature strength of ceramics provides significantly improved resistance to thermal damage in the severe thermo- mechanical environment of cannon firing[3].

Methods of analysis will be generally similar to those described recently by Parker et al.[4], where various combinations of autofrettage and external tension wrapping of steel-lined, carbon-fiber jacketed tubes were studied. Here, the liner-jacket analysis will be for two types of ceramic liner, Si₃N₄ and SiC, and two types of jacket, A723 pressure vessel steel and carbon-epoxy laminate. Emphasis is on composite jacket inner radius stresses that can exceed typical failure strengths and on ceramic liner inner radius stresses that can result in alarmingly small critical crack sizes for brittle fracture. The analysis is for a representative initial residual interface pressure between liner and jacket that would be expected for either shrink fitting or tension wrapping of a liner-jacket vessel.

The work of Malinowski and Magnuki[5] employed parametric discrete optimization techniques to design internal reinforcements of pressure vessels by minimizingthe reinforcement mass considering stress constraints. Following an analytical approach, Banichuk et al.[6] used variational calculus to find the head meridian profile optimal curve in order to maximize the ratio between head volume and mass subjected to stress constraints.

A follow up of this work was provided by Du and Olhoff [7] and Zheng et al.[8], who extended the investigation to three-dimensional structures. However, they considered as objective function the minimization of mean compliance without stress constraints, which is not realistic for pressure vessel design. In fact, the solution of topology optimization considering stress constraints is still an open problem[9-11].

Recently, Blachut and Magnuki[12] published an extensive review about modeling and optimization of pressure vessels where they mention that, in the optimization field, there are still few pieces of work related to pressure vessel design. This work provides a contribution along those lines by presenting an integrated approach in which the entire vessel is considered in the optimization process.

2. Stress Analysis

2.1. Fracture Analysis

Fracture analysis is done to calculate the fracture thickness values at the inner radius of the ceramic lined vessel can be calculated using the formula given below.

Crack Thickness,

2.2. Effect of Jacket

Stresses were calculated for a Silicon-nitride lined vessel with dual jacket is listed in Table 1. Figure 1 shows the results for the C-epoxy jacket, including prestress, firing and total stresses versus radial position, r/a. The prestress is due to the interface pressure at the junction, already discussed. The pressure applied to the vessel inner radius was 500 MPa, based on the 400–700 MPa pressures used in modern cannons. Note that, although the radial prestress does not exceed the C-epoxy compressive strength, when the firing stresses are added, both radial and hoop stresses exceed the respective strength values for C-epoxy.

The total radial stress at the interface is 2300 MPa, compared with 120 MPa compressive strength, and the total jacket hoop stress is 770 MPa, compared with the 600 MPa tensile strength. The problems under consideration are a composite pressure vessel shell which has a liner and a single jacket or a dual jacket. Stress analysis is to be done for the single jacket vessel and dual jacket vessel and the stress variations are to be studied. The wear and erosion is also high in gun barrels. So suitable materials are to be chosen for this application. The different materials taken for this purpose are ceramic materials like Si₃N₄ and SiC for liner, and C-epoxy and A723 Steel for Jacket.

2.3. Effect of Temperature

The effect of moderately elevated temperature on total stresses in a ceramic lined vessel is of interest for cannons and some other applications. Figures 4 and 5 show results for C-epoxy or steel jacked, Si3N4 lined vessels, respectively, at 25°C ambient temperature and after a uniform rise to 125°C. First, note that the ambient results ~25°C; solid lines! are nearly identical for the two vessel ~Fig. 4! the jacket stresses at 125°C are slightly increased, adding to the problem of exceeding the material strength, discussed earlier. However, the liner integrity is improved with the increase in temperature.

The stresses are decreased due to the favorable differential expansion between C-epoxy jacket and ceramic liner. This differential expansion was modeled by an increased 0.13 mm interference between jacket and liner at 125°C, compared with 0.10 mm at 25°C.The effect of temperature on total stresses with a steel liner in Fig. 5 shows that jacket stresses are slightly reduced, but to no advantage in structural integrity because of the adequate strength of steel. However the liner integrity suffers at high temperature, because differential expansion decreases the interference.

3. Results and discussion

3.1. Stress Variation for Dual Jacketed Vessel

Table 1. Variation of Hoop stresse for dual jacketed vessel Radial Location (r/a) Hoop’s Stress (MPa) Prestress Firing Total 1 -928.82 833.34 -95.48 1.1 -848.21 717.63 -130.58 1.2 -786.9 629.63 -157.27 1.3 -739.19 561.14 -172.05 1.33 -726.94 543.55 -183.39 1.33 310.27 543.55 853.82 1.4 289.3 506.8 796.1 1.5 264.27 462.96 727.23 1.6 243.79 427.08 670.87 1.66 233.24 408.6 641.84 1.66 651.44 408.6 1060.04 1.7 633.5 397.35 1030.85 1.8 593.77 372.43 966.2 1.9 560.15 351.34 911.49 2 531.44 333.33 864.77

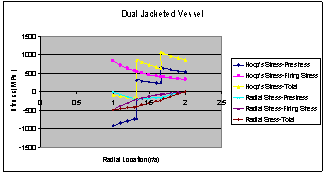Figure 1. Variation of firing & pre-stress for continuous liner and jacket vessels

3.2.Temperature Effect

(a) SiC-C epoxy Vessel:

Table 2. Variation of hoop stress at different temperatures Radial Location (r/a) Hoop’s Stress (MPa) 25°C 125°C 1 281.21 84.41 1.1 213.5 16.7 1.2 161.93 -34.87 1.3 121.8 -75 1.33 111.49 -85.31 1.33 853.82 876.32 1.4 796.1 818.6 1.6 670.87 693.37 1.8 585.02 607.52 1.9 551.89 574.39 2 523.61 546.11

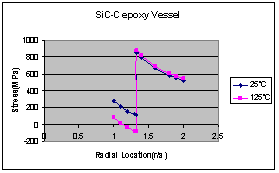Figure 2. Variation of temperature stress for SiC-C epoxy vessel

(b) SiC-A723 Steel Vessel:

Table 3. Variation of hoop stress for SiC-A723 Steel Vessel Radial Location (r/a) Hoop’s Stress (MPa) 25°C 125°C 1 281.21 478.01 1.1 213.5 410.3 1.2 161.93 358.73 1.3 121.8 318.6 1.33 111.49 308.29 1.33 853.82 605.42 1.4 796.1 547.7 1.6 670.87 422.47 1.8 585.02 336.62 1.9 551.89 303.49 2 523.61 275.21

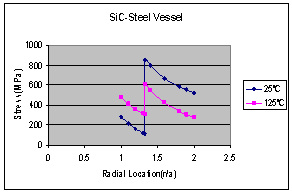Figure 3. Variation of temperature stress for SiC-steel epoxy vessel

3.3. Fracture Analysis

The fracture analysis is performed for the two ceramic materials for single and dual jacketed vessels and tabulated in Table4. It is observed that silicon carbide materials are prone to less crack thickness than silicon nitride materials.

Table 4. Crack thickness for different materials Liner Material Crack Thickness Values (mm) Single Jacket Vessel Dual Jacket Vessel Si3N4 0.01496 0.05582 SiC 0.0066 0.02481

4. Summary & Conclusions

The type of jacket had no significant effect on liner or jacket stresses, because the elastic modulus of the carbon-epoxy considered was close to that of steel. However, the total prestress, firing radial and hoop stresses at the inner radius of the carbon epoxy jack et exceeded typical material strengths in these directions. The result of the fracture analysis is that a ceramic lined pressure vessel with a thin, low modulus, high toughness ceramic liner and a dual-steel interference-fitted jacket would have the best structural integrity of the cases considered. However, it is emphasized that the very small critical crack size for brittle fracture that is inherent in the use of ceramics demands the utmost care in pressure vessel design.