M. Zakaria 1, 2
1Mathematics department, Faculty of Education, El-Shatby 21526, Alexandria University, Alexandria, Egypt
2Department of Mathematics, Faculty of Science, Al-Baha University, P.O. Box 1988 Al-Baha, Kingdom of Saudi Arabia
Correspondence to: M. Zakaria , Mathematics department, Faculty of Education, El-Shatby 21526, Alexandria University, Alexandria, Egypt.
| Email: |  |
Copyright © 2012 Scientific & Academic Publishing. All Rights Reserved.
Abstract
The problem of two dimensional magnetic micropolar generalized thermoelastic medium, in the presence of the combined effect of Hall currents subjected to ramp-type heating, is investigated. The medium is permeated by a strong transverse magnetic field imposed perpendicularly to the displacement plane on the assumption of the induced electric field is neglected. Ohm’s law is modified by the inclusion of two terms, one for the cross product of the current density with the initial magnetic field and the second for the cross product of the velocity with the initial magnetic field. Laplace and exponential Fourier transform techniques are employed to transform the governing partial differential equations to ODE which were solved exactly. Comparisons with previously published work have been conducted and the results are found to be in good agreement. The distributions of the temperature, the displacement, the stress, the microrotation and the current density are obtained. The numerical values of these functions are represented graphically.
Keywords:
Hall Current, Micropolar, Thermoelasticity, Ramp-Type Heating
Cite this paper:
M. Zakaria , "Effect of Hall Current on Generalized Magneto-thermoelasticity Micropolar Solid Subjected to Ramp-type Heating", American Journal of Materials Science, Vol. 1 No. 1, 2011, pp. 26-39. doi: 10.5923/j.materials.20110101.05.
1. Introduction
The linear theory of elasticity is of paramount importance in the stress analysis of steel, which is the commonest engineering structural material. To a lesser extent, linear elasticity describes the mechanical behavior of the other common solid materials, e.g. concrete, wood and coal. However, the theory does not apply to the behavior of many of the new synthetic materials of the elastomer and polymer type, e.g. polymethyl-methacrylate (Perspex), polyethylene and polyvinyl chloride. The linear theory of micropolar elasticity is adequate to represent the behavior of such materials. For ultrasonic waves i.e. for the case of elastic vibrations characterized by high frequencies and small wavelengths, the influence of the body microstructure becomes significant. This influence of microstructure results in the development of new type of waves, not found in the classical theory of elasticity. Metals, polymers, composites, soils, rocks, concrete are typical media with microstructures. More generally, most of the natural and manmade materials including engineering, geological and biological media possess a microstructure. Eringen and Suhubi[1] and Suhubi and Eringen[2] developed the nonlinear theory ofmicro-elastic solids. Later Eringen[3-5] developed a theory for the special class of micro-elastic materials and called it the “linear theory of micropolar elasticity”. Under this theory, solids can undergo macro-deformations and macro - rotations.The classical uncoupled theory of thermoelasticity predicts two phenomena not compatible with physical observations. First, the equation of heat conduction of this theory does not contain any elastic terms, second, the heat equation is of a parabolic type, predicting infinite speeds of propagation for heat waves.Biot[6] introduced the theory of coupled thermoelasticity to overcome the first shortcoming. The governing equations for this theory are coupled, eliminating the first paradox of the classical theory. However, both theories share the second shortcoming since the heat equation for the coupled theory is also parabolic.Two generalizations to the coupled theory were introduced. The first is due to Lord and Shulman[7], who obtained a wave-type heat equation by postulating a new law of heat conduction to replace the classical Fourier’s law. Since the heat equation of this theory is of the wave-type, it automatically ensures finite speeds of propagation for heat and elastic waves. The remaining governing equations for this theory, namely, the equations of motion and constitutive relations, remain the same as those for the coupled and the uncoupled theories. The second generalization is known as the theory of thermoelasticity with two relaxation times, or the theory of temperature-rate-dependent thermoelasticity, and was proposed by Green and Lindsay[8]. It is based on a form of the entropy inequality proposed by Green and Laws[9]. It does not violate Fourier’s law of heat conduction when the body under consideration has a center of symmetry, and it is valid for both isotropic and anisotropic bodies. Ezzat and Youssef[10] studied the generalized magneto-thermoelasticity in a perfectly conducting medium.The foundations of magnetoelasticity were presented by Knopoff[11] and Chadwick[12] and developed by Kaliski and Petykiewicz[13]. An increasing attention is being devoted to the interaction between magnetic field and strain field in a thermoelastic solid due to its many applications in the fields of geophysics, plasma physics and related topics. In all papers quoted above it was assumed that the interactions between the two fields take place by means of the Lorentz forces appearing in the equations of motion and by means of a term entering Ohm’s law and describing the electric field produced by the velocity of a material particle, moving in a magnetic field.Many authors have considered the propagation of electromagneto-thermoelastic waves in an electrically and thermally conducting solid. Paria[14] discussed the propagation of plane magneto-thermoelastic waves in an isotropic unbounded medium under the influence of a uniform thermal field and with a magnetic field acting transversely to the direction of the propagation. Paria used the classical Fourier law of heat conduction, and neglected the electric displacement. Wilson[15] extended Paria’s results by introducing a component of the magnetic field parallel to the direction of the propagation. A comprehensive review of the earlier contributions to the subject can be found in Paria[16]. Among the authors who considered the generalized magneto-thermoelastic equations are Nayfeh and Namat-Nasser[17] who studied the propagation of plane waves in a solid under the influence of an electromagnetic field. They obtained the governing equations in the general case and the solution for some particular cases. Choudhuri[18] extended these results to rotating media. Sherief and Ezzat[19] solved a thermal shock half-space problem using asymptotic expansions. Lately, Rajneesh Kumar and Rupender[20] studied the effect of rotation in magneto-micropolar thermoelastic medium due to mechanical and thermal sources, Rajneesh Kumara and ManjeetSingh[21] solved the effect of rotation and imperfection on reflection and transmission of plane waves in anisotropic generalized thermoelastic media, R. Kumar, Praveen Ailawalia[22] studied moving load response in micropolar thermoelastic medium without energy dissipation possessing cubic symmetry, few attempts have been made to solve two-dimensional problems in this field Moncef Aouadi[23] introduced temperature dependence of an elastic modulus in generalized linear micropolar thermoelasticity.When the magnetic field is very strong, the conductivity will be a tensor and the effect of Hall current cannot be neglected. The conductivity normal to the magnetic field is reduced due to the free spiraling of electrons and ions about the magnetic lines of force before suffering collisions and a current is induced in a direction normal to both the electric and magnetic fields. This phenomenon is called the Hall effect. In all of the above investigations, the effects of Hall current have not been considered.The objective of this paper is to consider two dimensional micropolar generalized magneto-thermoelasticity medium, in the presence of a uniform strong magnetic field acts in y-direction taken into consideration the Hall current effects. This new model is applied to generalization, Lord–Shulman theory and Green–Lindsay theory, as well as to the coupled theory and solved by using Laplace-Fourier transform technique. The formulas of temperature, displacement, stresses, the microrotation and the current density are obtained. The ramp-type heating application is employed to our problem to get the solution in the complete form. The considered variables are presented graphically and comparisons and discussions are made.
2. Basic Equations
We shall consider a generalized magneto - thermoelasticity micropolar solid occupying the region . The governing equations of motion for homogenous, isotropic and elastic solid, when the Hall current effect is taken into account consists of:i. The equations of motion taking into account the Lorentz force have the form
. The governing equations of motion for homogenous, isotropic and elastic solid, when the Hall current effect is taken into account consists of:i. The equations of motion taking into account the Lorentz force have the form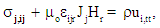 | (1) |
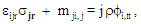 | (2) |
where σij is the force stress tensor, ui is the components of the displacement tensor, φi is the components of the microrotation tensor, mij is the couple stress tensor, ρ is the density, j is the microinertia, εijr is the permutation symbol, µo is the magnetic permeability, Hr is the intensity tensor of magnetic field and Jr is the conduction current density.The above equations are supplemented by the modified Ohm’s law for media with finite conductivity and including the Hall current effect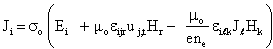 | (3) |
where σo ( = ne e2 te / me ), is the electrical conductivity, e is the charge of an electron, ne is the electron number density, te is the electron collision time, me is the mass of the electron and Ei is the intensity tensor of the electric field.ii. The constitutive equation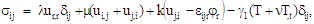 | (4) |
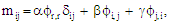 | (5) |
where λ, μ are Lame’s constants, α, β, γ, and k are micropolar constants, T is the absolute temperature of the medium, ν is the constant with dimension of time, called relaxation time, γ1 is the material constant given by γ1 = ( 3 λ+2μ+K)αT, and αT being a coefficient of linear thermal expansion.iii. The generalized equation of heat condition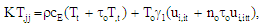 | (6) |
where K is thermal conductivity, cE is the specific heat at constant temperature, To is the uniform temperature, τo is another relaxation time and no is a non-dimensional constant. For L–S theory n0 =1, τo > 0, ν = 0, and for G–L theory n0 = 0, τo > 0, ν > 0.Substituting equations (4), and (5) into equation (5), and (6) we get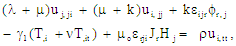 | (7) |
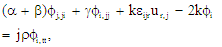 | (8) |
3. Formulation and Solution of the Problem
The rectangular Cartesian system (x, y, z) having origin on the surface x = 0 with x-axis vertical into the medium is introduction. In the following, we restrict our analysis parallel to xz-plane with x 0, ui = (u, 0, w), and φi = (0, φ, 0). A uniform very strong magnetic field of strength Ho is assumed to be applied in the positive y-direction, we also assume that E = 0. Under these assumptions the modified Ohm’s law gives Jy = 0 everywhere in the medium. The current density components Jx and Jz are given by: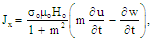 | (9) |
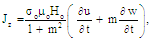 | (10) |
where m (= ωe te) is the Hall parameter and ωe (= e µoHo /me) is the electron frequency,For simplifications we shall use the following non-dimensional variables: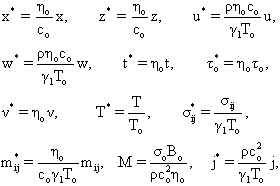 where
where 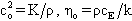 and M is the Hartmann number or magnetic parameter.
and M is the Hartmann number or magnetic parameter.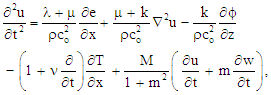 | (11) |
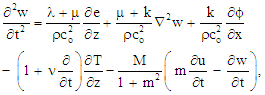 | (12) |
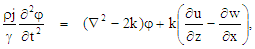 | (13) |
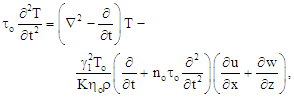 | (14) |
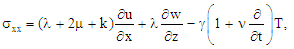 | (15) |
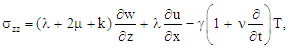 | (16) |
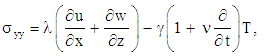 | (17) |
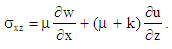 | (18) |
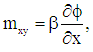 | (19) |
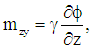 | (20) |
Introducing potential functions Φ(x,z,t), and Ψ(x,z,t) defined by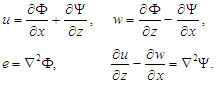 | (21) |
Substituting Eq. (21) into Eqs. (11)–(14) we obtain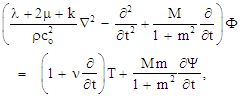 | (22) |
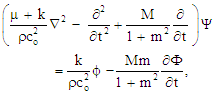 | (23) |
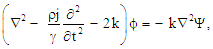 | (24) |
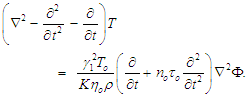 | (25) |
Taking Laplace transform defined by the relation: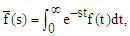 of both sides of (15)-(20) and (22)-(25) using the homogenous initial conditions, we obtain:
of both sides of (15)-(20) and (22)-(25) using the homogenous initial conditions, we obtain: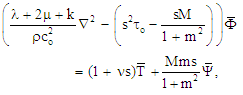 | (26) |
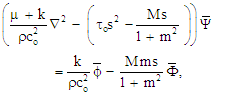 | (27) |
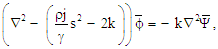 | (28) |
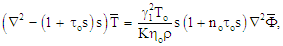 | (29) |
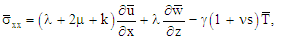 | (30) |
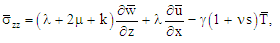 | (31) |
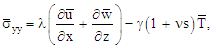 | (32) |
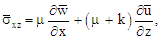 | (33) |
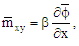 | (34) |
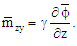 | (35) |
We now use the Fourier transform with respect to the space variable z, defined by Taking the Fourier transform of both sides of (26)–(33)
Taking the Fourier transform of both sides of (26)–(33)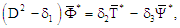 | (34) |
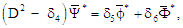 | (35) |
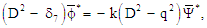 | (36) |
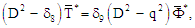 | (37) |
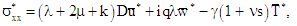 | (38) |
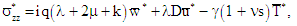 | (39) |
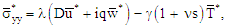 | (40) |
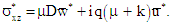 | (41) |
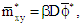 | (42) |
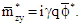 | (43) |
where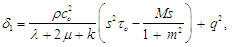
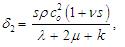
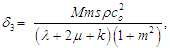
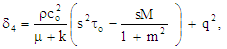
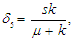
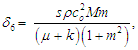
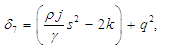
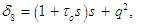
 and the operator D means the derivative with respect to x.On Eqs. (34)-(37) and after some simplification, we arrive at the following eighth order differential equation satisfied by
and the operator D means the derivative with respect to x.On Eqs. (34)-(37) and after some simplification, we arrive at the following eighth order differential equation satisfied by 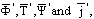
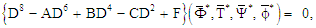 | (44) |
where 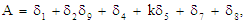
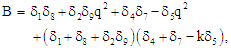
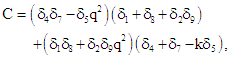
 The solution of the Eq. (44) satisfying the radiation conditions that and tend to zero as x tends to infinity can be written as
The solution of the Eq. (44) satisfying the radiation conditions that and tend to zero as x tends to infinity can be written as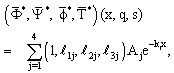 | (45) |
where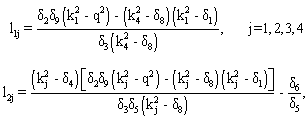
 and kj (j=1,2,3,4) are the characteristic roots of the characteristic equation (36) which is
and kj (j=1,2,3,4) are the characteristic roots of the characteristic equation (36) which is Eq. (21) together with Eq. (45), immediately give
Eq. (21) together with Eq. (45), immediately give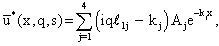 | (46) |
 | (47) |
In order to get the components of stresses, substituting from equations (45)-(47) into equations (38)-(41) we get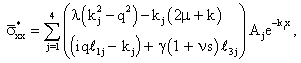 | (48) |
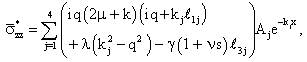 | (49) |
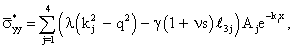 | (50) |
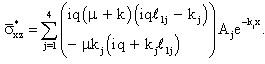 | (51) |
Moreover, the components of couple stresses can be obtained in a similar manner by substituting from (45) into (42) and (43) we get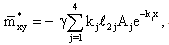 | (52) |
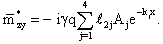 | (53) |
To obtain the components of current density, substituting from equations (46) and (47) into equations (9) and (10) and after taking Laplace-Fourier transform we get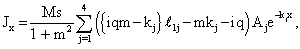 | (54) |
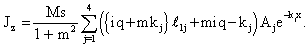 | (55) |
We shall now go to the applications of the problem to evaluate the unknown parameters Aj, j=1,2,3,4.
4. Application
We consider a micropolar thermoelastic material occupying the semi-space region: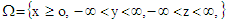 ,let the surface of Ω is traction free and subjected to ramp-type heating, a- Thermal boundary condition.The boundary of the half-space is affected by ramp-type heating, which depends on the coordinate z and the time t of the form
,let the surface of Ω is traction free and subjected to ramp-type heating, a- Thermal boundary condition.The boundary of the half-space is affected by ramp-type heating, which depends on the coordinate z and the time t of the form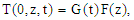 | (56) |
where F(z) is an arbitrary function of z and G(t) is a function defined as Misra et al.,[25]: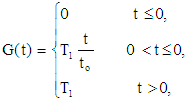 | (57) |
where to indicates the length of time to rise the heat and T1 is constant, this means that the boundary of the half-space, which is initially at rest and has a fixed temperature To, is suddenly raised to a temperature equal to the function G(t)F(z) and maintained at this temperature from then on.Applying the Laplace and Fourier transforms to both sides of Eq. (56), we obtain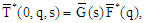 | (58) |
where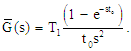 b- Mechanical boundary conditions.Under the assumption that the surface of Ω is traction free we can get the following conditions
b- Mechanical boundary conditions.Under the assumption that the surface of Ω is traction free we can get the following conditions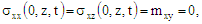 | (59) |
Applying the Laplace and Fourier transforms, we get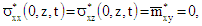 | (59) |
By substituting from equations (58) and (59) into equations (45), (48), (51) and (53), the boundary conditions may be reduced to: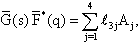 | (60) |
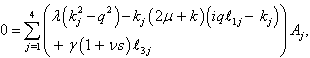 | (61) |
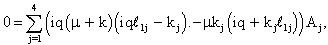 | (62) |
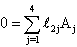 | (63) |
By solving this system of equations for Aj ( j = 1, 2, 3, 4) , we can complete the solution of our problem.
5. Particular Cases
i. If m = 0, in Eqs. (45)–(55), we obtain the components of displacements and tresses in magneto-micropolar generalized thermoelastic without Hall current effect.ii. Taking Hartmann number M = 0, in Eqs. (45)–(55), we obtain the components of displacements and tresses in micropolar generalized thermoelastic iii. Taking n0 =1, τo > 0, ν = 0 in Eqs. (45)–(55), we obtain the corresponding expressions for magneto-micropolar thermoelastic for L–S theory.iv. Taking n0 = 0, τo > 0, ν > 0 in Eqs. (45)–(55), we obtain the corresponding expressions for magneto-micropolar thermoelastic for G–L theory.v. Taking , n0 = 0, τo= 0, ν= 0 we obtain the corresponding expressions for magneto-micropolar coupled thermoelastic medium for C–T theory.
6. Inversion of the Transforms
To obtain the solution of the problem in the physical domain (x, z, t), we have to invert the iterated transforms in Eqs. (45)–(55). These expressions can be formally expressed as functions of x and the parameter of the Fourier and Laplace transforms q and s of the form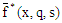 .First, we invert the Fourier transform using the inversion formula given previously. This gives the Laplace transform expression
.First, we invert the Fourier transform using the inversion formula given previously. This gives the Laplace transform expression 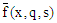 of the function
of the function 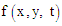 as
as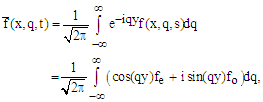 where fe and fo denote the event and the odd parts of the function
where fe and fo denote the event and the odd parts of the function 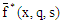 respectively.We shall now outline the numerical inversion method used to find the solution in the physical domain. For fixed values of x, y, and q the function inside braces in the last integral can be considered as a Laplace transform of some function g(t).The inversion formula for the Laplace transform can be written as
respectively.We shall now outline the numerical inversion method used to find the solution in the physical domain. For fixed values of x, y, and q the function inside braces in the last integral can be considered as a Laplace transform of some function g(t).The inversion formula for the Laplace transform can be written as where c is an arbitrary real number greater than all the parts of the singularities
where c is an arbitrary real number greater than all the parts of the singularities . Taking s = c + iy, the above integral takes the form
. Taking s = c + iy, the above integral takes the form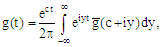 Expanding the function h(t) = exp(-ct)g(t) in a Fourier series in the interval[0, 2L], we obtain the approximate formula.
Expanding the function h(t) = exp(-ct)g(t) in a Fourier series in the interval[0, 2L], we obtain the approximate formula.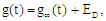 where
where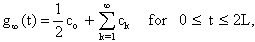 | (64) |
and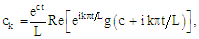 | (65) |
ED, the discretization error, can be made arbitrary small by choosing c large enough.Since the infinite series in Eq. (64) can be summed up to finite number N of terms, the approximate value of g(t) becomes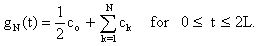 | (66) |
Using the above formula to evaluate g(t), we introduce a truncation error ET which, must be added to the discretization error to produce the total approximation error.Two methods are used to reduce the total error. First the ‘‘Korrecktur’’ method is used to reduce the discretization error. Next, the e algorithm is used to reduce the truncation error and hence to accelerate convergence.The Korrecktur method uses the following formula to evaluate the function g(t):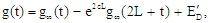 where the discretization error
where the discretization error . Thus, the approximate value of g(t) becomes
. Thus, the approximate value of g(t) becomes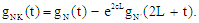 | (67) |
N' is an integer such that N' < N.We shall now describe the e-algorithm that is used to accelerate the convergence of the series in Eq. (66).Let N = 2q + 1 where q is a natural number, and let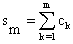 be the sequence of partial sums of Eq. (66). We define the e-sequence by
be the sequence of partial sums of Eq. (66). We define the e-sequence by and
and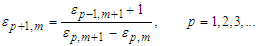 it can be shown that the sequence ε1,1, ε3,1, ε5,1,…, εN,1, converges to f(x,y, t) + ED – co/2 faster than the sequence of partial sums sm (m = 1,2,3,…).The actual procedure used to invert the Laplace transform consists of using Eq. (67) together with the ε-algorithm. The values of c and L are chosen according to the criteria outlined Honig and Hirdes[24].
it can be shown that the sequence ε1,1, ε3,1, ε5,1,…, εN,1, converges to f(x,y, t) + ED – co/2 faster than the sequence of partial sums sm (m = 1,2,3,…).The actual procedure used to invert the Laplace transform consists of using Eq. (67) together with the ε-algorithm. The values of c and L are chosen according to the criteria outlined Honig and Hirdes[24].
7. Numerical Results and Discussion
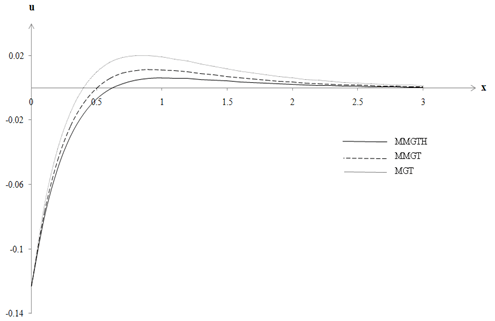
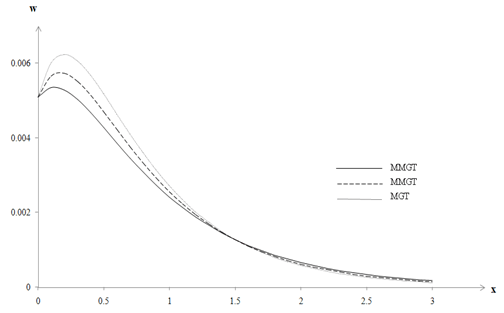
The analysis is conducted for a magnesium crystal-like material. Following reference[25], the values of physical constants areλ= 9.4x1010 Nm-2, μ=4.0x1010 Nm-2, k=1.0x1010 Nm-2, ρ=1.74x103 gm/cm3, γ=0.779x10-9N, j=0.2x10-15 cm2,cE=1.04x103 kgm-3, K*=1.7x102 Jm-1s-1deg-1, To=298 K,ν = 3.68x106Nm-21deg-1, τo=0.02, no=0.05.We consider the following electric constants for our problemσo = 9.36x105Col2/Cal.cm.sec, Ho = 108 Col/cm.secThe computations are carried out for the non-dimensional time t=0.2, strip width a=0.9x103 and on the surface plane y=0. The distribution of non-dimensional temperature T, non-dimensional normal displacement u, non-dimensional transverse displacement w, non-dimensional micro-rotation φ, non-dimensional normal force stress σxx, non-dimensional shearing stresses σxz, non-dimensional couple stress mxy, non-dimensional normal conduction current density field Jx and non-dimensional transverse conduction current density field Jz, with non-dimensional distance x have been shown in Figs. 1–9. Numerical analysis has been carried out by taking x range from 0 to 3. These figures are obtained to illustrate the relative influence of the parameter to, magnetic field parameter (Hartmann number) M and Hall parameter m.The solid line represents magneto-micropolar generalized thermoelastic with Hall current effect MMGTH when M = 0.2, m = 0.6, and large dashes line represents magneto-micropolar generalized thermoelastic without Hall current effect MMGT when M= 0.2, m = 0, while small dotes line represents micropolar generalized thermoelastic MGT for M = 0, m =0. The field quantities, temperature, displacement, force stress, couple stress, and current density fields do not depend only on the state and space variables t , x and z, but also depend on Hall parameter m. It has been observed that, the finite Hall parameter m plays a vital role on the development of temperature, displacement force stress, couple stress, and current density fields. The numerical values for the field temperature are computed for a wide range of values of finite pulse rise-time to in the two situations t > to and t < to.Fig. 1 exhibits the space variation of temperature at t = 0.2 for different values of to and m for the threemodels; magneto-micropolar generalized thermoelastic with Hall current effect MMGTH , magneto-micropolar generalized thermoelastic without Hall current effect MMGT and micropolar generalized thermoelastic MGT in which we observe when to < t in some range of x we have great different values of temperature for the three models in comparing to the case to > t the three models. From this figure we see that the all curves start from two values one at 0.52 when to = 0.1 and the other 0.32 when to = 0.1 then decreases till it tends to zero at x =3, we observe that from this figure the temperature T increases in the case MMGTH in comparison to curve MGT, while decreases in comparison to curves MMGT. The effect of Hall parameter m on the normal displacement u against x is displayed in Fig. 2. It is noticed from the graphic representation of the normal displacement is started from point x = -0.123 for all the cases and then increases up to the point x = 0.7 then decreases till it tends to zero at x =3. From this figure we observe that an increase in Hall parameter m leads to a decrease in the displacement u distribution because the aim of this latter parameter is to produce finite speed of displacement u. Fig. 3 illustrates the influence of the Hall parameter m and Hartmann number M on the transverse displacement w in the medium of generalized magneto-thermoelasticity micropolar solid. Application of magnetic field to MMGTH gives rise to a resistive type force called the Lorentz force. This force has the tendency to slow down the transverse displacement w in the medium. Also, the effects on w become more as the strength of the magnetic field increases, also we record values higher in case MMGTH as compared to the values for case MMGT. It is observed from the decreases in the transverse displacement we presented in Fig. 3.Fig. 4 shows that micro-rotation φ profiles across the medium for different values of the M and m parameters. The models MMGTH, MMGT and MMGT are representation corresponding to M = o.6, m = 1.5, M = 0.6, m = 0 and M = m =0 respectively. It is observed that an increase in Hall parameter m leads to increase in the micro-rotation φ distribution. These behaviors are clear from Fig. 4.Figs. 5, 6 describe the variation of the normal stresses σxx and one of the shearing stresses σxz, the influence of the Hall parameter m affects on the normal stresses by decreasing their values and on the shearing stresses by increasing their values up to the point x = 0.26 then opposite the direction of increasing.Fig. 7 shows that the Variation of couple stress myz increase in the case MMGT in comparison to curve MMGT. The figure indicates that myz records values higher in case MMGTH as compared to the values of case MGT. Figs. 8, 9 describe the variation of two components of density component Jx and Jz respectively; it is evident that the values of both fields are decreased in the MMGTH as compared to the values of the cases MMGT and MGT.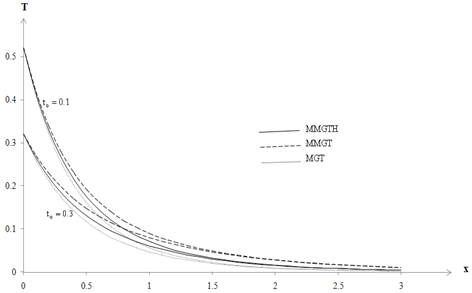 | Figure 1. Variation of temperature distribution T with distance x |
 | Figure 2. Variation of normal displacement u with distance x |
 | Figure 3. Variation of transverse displacement w with distance x |
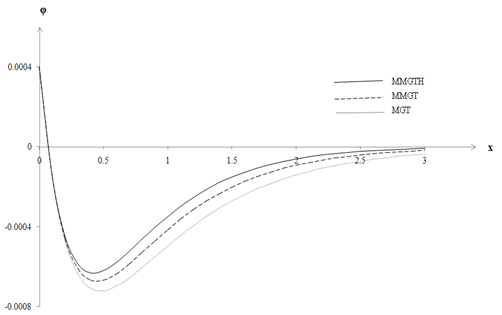 | Figure 4. Variation of micro-rotation φ with distance x |
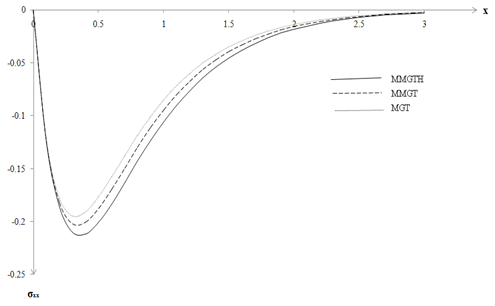 | Figure 5. Variation of normal stresses σxx with distance |
 | Figure 6. Variation of shearing stresses σxx with distance x |
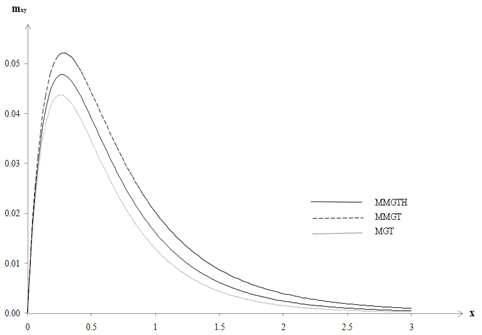 | Figure 7. Variation of couple stresses mxy with distance x |
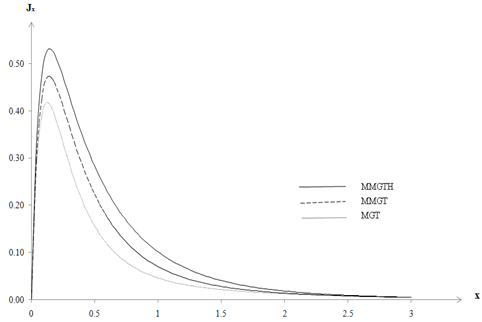 | Figure 8. Variation of normal conduction current density field Jx with distance x |
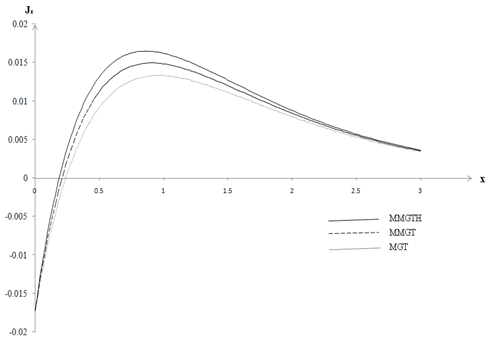 | Figure 9. Variation of transverse conduction current density field Jz with distance x |
References
| [1] | A.C. Eringen, E.S. Suhubi, Non-linear theory of micro-elastic Solids I. Int. J. Eng. Sci. 2(1964), 189–203. |
| [2] | E.S. Suhubi, A.C Eringen, Nonlinear theory of micro-elastic Solids II. Int. J. Eng. Sci. 2(1964), 389–404. |
| [3] | A.C. Eringen, A unified theory of thermo-mechanical materials, Int. J. Eng. Sci. 4(1966), 179–202. |
| [4] | A.C. Eringen, Linear theory of micropolar elasticity, ONR Technical Report No. 29, School of Aeronautics, Aeronautics and Engineering Science. Purdue University, (1965). |
| [5] | A.C. Eringen, Linear theory of micropolar elasticity, J. Mech. 15(1966), 909-923. |
| [6] | M.A. Biot, Thermoelasticity and irreversible thermodynamics. J. Appl. Phys. 27 (1956) 240–253. |
| [7] | H. Lord, Y. Shulman, A generalized dynamical theory of thermoelasticity, J. Mech. Phys. Solid 15 (1967) 299 |
| [8] | A.E. Green, K.A. Lindsay, Thermoelasticity. J. Elasticity 2 (1972), 1–7. |
| [9] | A.E. Green, N. Laws, On the entropy production inequality. Arch. Ration. Mech. Anal. 45(1972), 45–47. |
| [10] | Magdy A. Ezzat , Hamdy M. Youssef, Generalized magneto-thermoelasticity in a perfectly conducting medium, Int. J. Solids and Structures 42 (2005) 6319–6334 |
| [11] | L. Knopoff, The interaction between elastic wave motion and a magnetic field in electrical conductors. J. Geophys. Res. 60(1955), 441–456. |
| [12] | Chadwick, P., 1957. Ninth Int. Congr. Appl. Mech. 7, 143. |
| [13] | S. Kaliski, J. Petykiewicz, Equation of motion coupled with the field of temperature in a magnetic field involving mechanical and electrical relaxation for anisotropic bodies. Proc. Vibr. Probl. (1959)4, 1. |
| [14] | G. Paria, On magneto-thermoelastic plane waves. Proc. Cambr. Phil. Soc. 56 (1962), 527–531. |
| [15] | A. Wilson, Proc. Cambr. Phil. Soc. 59 (1963), 483–488. |
| [16] | G. Paria, Magneto-elasticity and magneto-thermoelasticity. Adv. Appl. Mech. 10 (1967), 73–112. |
| [17] | A.Nayfeh, S. Namat-Nasser, Electromagneto-thermoelastic plane waves in solids relaxation. J. Appl. Mech. E 39 (1972), 108–113. |
| [18] | S. Choudhuri, Electro-magneto-thermoelastic plane waves in rotating media with thermal relaxation. Int. J. Eng. Sci. 22 (1984), 519–530. |
| [19] | H. Sherief, M. Ezzat, A thermal sock problem in magneto-thermoelasticity with thermal relaxation. Int. J. Solids Struct. 33 (1996), 4449–4459. |
| [20] | Rajneesh Kumar, Effect of rotation in magneto-micropolar thermoelastic medium due to mechanical and thermal sources.Chaos, Solitons and Fractals in press |
| [21] | Rajneesh Kumar, Rupender , ManjeetSingh, Effect of rotation and imperfection on reflection and transmission of plane waves in anisotropic generalized thermoelastic media, J. Sound and Vibration, in press |
| [22] | Rajneesh Kumar, Praveen Ailawalia, Moving load response in micropolar thermoelastic medium without energy dissipation possessing cubic symmetry, International Journal of Solids and Structures 44 (2007) 4068–4078 |
| [23] | Moncef Aouadi, Temperature dependence of an elastic modulus in generalized linear micropolar thermoelasticity, ZAMP, .(2006) 1057{1074 |
| [24] | G. Honig, U. Hirdes: A method for the numerical inversion of Laplace transform. J. Comp. Appl. Math. 10 (1984) 113–132. |
| [25] | A. C. Eringen. Plane wave in nonlocal micropolar elasticity. Int J Eng Sci 22 (1984) 1113–1121. |

 . The governing equations of motion for homogenous, isotropic and elastic solid, when the Hall current effect is taken into account consists of:i. The equations of motion taking into account the Lorentz force have the form
. The governing equations of motion for homogenous, isotropic and elastic solid, when the Hall current effect is taken into account consists of:i. The equations of motion taking into account the Lorentz force have the form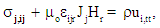
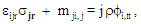
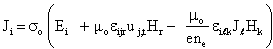
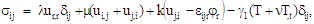
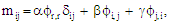
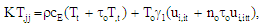
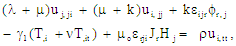
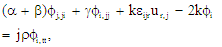
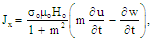
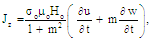
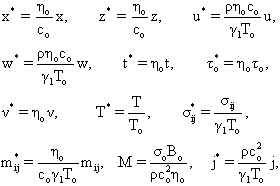 where
where 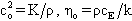 and M is the Hartmann number or magnetic parameter.
and M is the Hartmann number or magnetic parameter.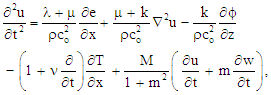
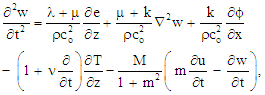
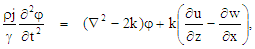
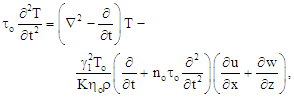
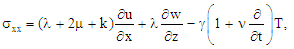
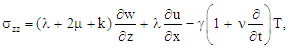
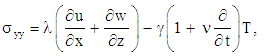
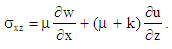
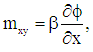
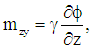
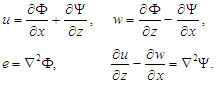
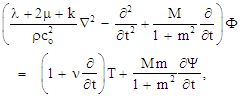
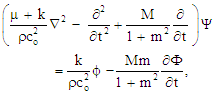
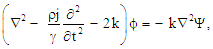
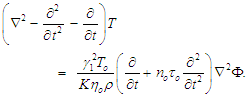
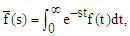 of both sides of (15)-(20) and (22)-(25) using the homogenous initial conditions, we obtain:
of both sides of (15)-(20) and (22)-(25) using the homogenous initial conditions, we obtain: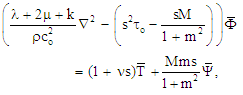
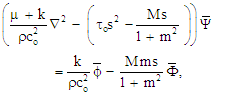
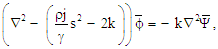
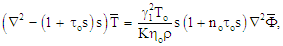
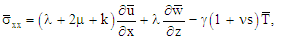
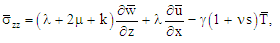
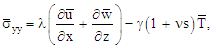
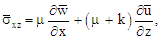
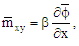
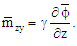
 Taking the Fourier transform of both sides of (26)–(33)
Taking the Fourier transform of both sides of (26)–(33)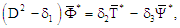
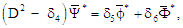
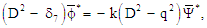
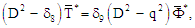
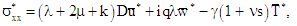
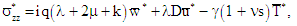
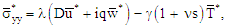
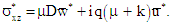
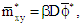
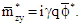
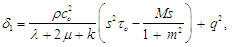
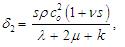
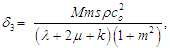
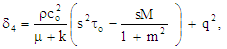
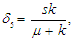
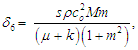
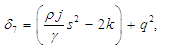
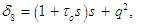
 and the operator D means the derivative with respect to x.On Eqs. (34)-(37) and after some simplification, we arrive at the following eighth order differential equation satisfied by
and the operator D means the derivative with respect to x.On Eqs. (34)-(37) and after some simplification, we arrive at the following eighth order differential equation satisfied by 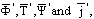
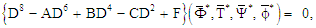
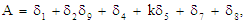
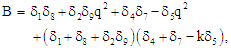
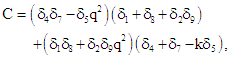
 The solution of the Eq. (44) satisfying the radiation conditions that and tend to zero as x tends to infinity can be written as
The solution of the Eq. (44) satisfying the radiation conditions that and tend to zero as x tends to infinity can be written as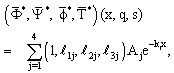
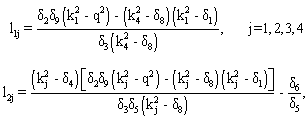
 and kj (j=1,2,3,4) are the characteristic roots of the characteristic equation (36) which is
and kj (j=1,2,3,4) are the characteristic roots of the characteristic equation (36) which is Eq. (21) together with Eq. (45), immediately give
Eq. (21) together with Eq. (45), immediately give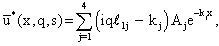

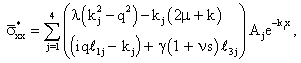
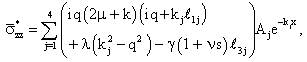
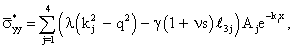
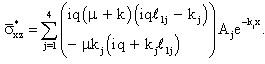
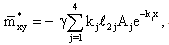
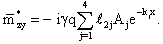
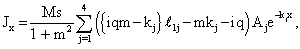
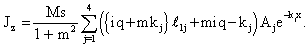
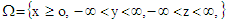 ,let the surface of Ω is traction free and subjected to ramp-type heating, a- Thermal boundary condition.The boundary of the half-space is affected by ramp-type heating, which depends on the coordinate z and the time t of the form
,let the surface of Ω is traction free and subjected to ramp-type heating, a- Thermal boundary condition.The boundary of the half-space is affected by ramp-type heating, which depends on the coordinate z and the time t of the form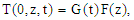
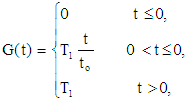
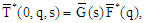
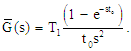 b- Mechanical boundary conditions.Under the assumption that the surface of Ω is traction free we can get the following conditions
b- Mechanical boundary conditions.Under the assumption that the surface of Ω is traction free we can get the following conditions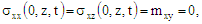
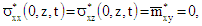
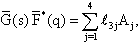
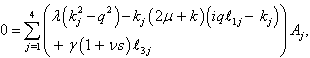
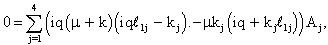
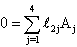
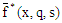 .First, we invert the Fourier transform using the inversion formula given previously. This gives the Laplace transform expression
.First, we invert the Fourier transform using the inversion formula given previously. This gives the Laplace transform expression 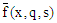 of the function
of the function 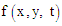 as
as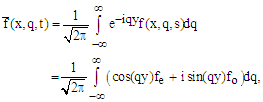 where fe and fo denote the event and the odd parts of the function
where fe and fo denote the event and the odd parts of the function 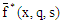 respectively.We shall now outline the numerical inversion method used to find the solution in the physical domain. For fixed values of x, y, and q the function inside braces in the last integral can be considered as a Laplace transform of some function g(t).The inversion formula for the Laplace transform can be written as
respectively.We shall now outline the numerical inversion method used to find the solution in the physical domain. For fixed values of x, y, and q the function inside braces in the last integral can be considered as a Laplace transform of some function g(t).The inversion formula for the Laplace transform can be written as where c is an arbitrary real number greater than all the parts of the singularities
where c is an arbitrary real number greater than all the parts of the singularities . Taking s = c + iy, the above integral takes the form
. Taking s = c + iy, the above integral takes the form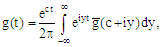 Expanding the function h(t) = exp(-ct)g(t) in a Fourier series in the interval[0, 2L], we obtain the approximate formula.
Expanding the function h(t) = exp(-ct)g(t) in a Fourier series in the interval[0, 2L], we obtain the approximate formula.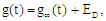 where
where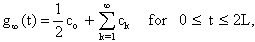
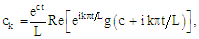
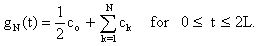
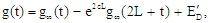 where the discretization error
where the discretization error . Thus, the approximate value of g(t) becomes
. Thus, the approximate value of g(t) becomes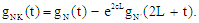
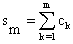 be the sequence of partial sums of Eq. (66). We define the e-sequence by
be the sequence of partial sums of Eq. (66). We define the e-sequence by and
and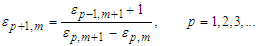 it can be shown that the sequence ε1,1, ε3,1, ε5,1,…, εN,1, converges to f(x,y, t) + ED – co/2 faster than the sequence of partial sums sm (m = 1,2,3,…).The actual procedure used to invert the Laplace transform consists of using Eq. (67) together with the ε-algorithm. The values of c and L are chosen according to the criteria outlined Honig and Hirdes[24].
it can be shown that the sequence ε1,1, ε3,1, ε5,1,…, εN,1, converges to f(x,y, t) + ED – co/2 faster than the sequence of partial sums sm (m = 1,2,3,…).The actual procedure used to invert the Laplace transform consists of using Eq. (67) together with the ε-algorithm. The values of c and L are chosen according to the criteria outlined Honig and Hirdes[24].








 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-Text HTML
Full-Text HTML