Olaniyi Diran Afolayan 1, Murtadha Adekilekun Tijani 2, Monsuru Tunde Akinleye 2
1PhD Student, Department of Civil Engineering, University of Ibadan, Nigeria
2Department of Civil Engineering, Adeleke University, Ede, Nigeria
Correspondence to: Olaniyi Diran Afolayan , PhD Student, Department of Civil Engineering, University of Ibadan, Nigeria.
| Email: |  |
Copyright © 2018 Scientific & Academic Publishing. All Rights Reserved.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/

Abstract
Laterite are soil types with the oxides of iron, aluminum, titanium, zircon, manganese, zinc and Quartz, they very significantly according to their location, climate and depth. Its use as pavement material depends on its index and engineering properties which are obtained experimentally in the laboratory or in-situ. Results on the strength and index properties of laterite as subgrade, sub base and base course material are collected from different geotechnical laboratories indicating the grain size distribution, Atterberg’s limit, Compaction test and the CBR test for the lateritic soil. The obtained equations through the use of SPSS and Least Square regression analysis shows that there exists relations among the engineering properties of laterite with the former producing greater degree of accuracy, more flexibility and higher ease of computation. The application of these statistical tools in determining the engineering properties of lateritic soil will reduce the length of time spent obtaining these properties experimentally; reduction in construction cost and time spent and eliminate errors that are likely to occur during experiments.
Keywords:
Laterite, Stength and Index properties, SPSS, Least-Square Regression
Cite this paper: Olaniyi Diran Afolayan , Murtadha Adekilekun Tijani , Monsuru Tunde Akinleye , Development of Analytical Relationships among Engineering Properties of Lateritic Soil as Pavement Materials, Journal of Civil Engineering Research, Vol. 8 No. 1, 2018, pp. 15-20. doi: 10.5923/j.jce.20180801.03.
1. Introduction
Laterization is a prolonged process of chemical weathering which produces a wide variety in the thickness, grade, chemistry and ore minerals [6]. Laterite can be used for variety of purpose ranging from block building, water supply, waste water treatment, road building etc [2, 10].Soil properties are very important when carrying out construction work of any kind because failure to test the soil upon which the project will be standing might be detrimental to the existence of the project. In the process of carrying out civil engineering projects, it is necessary to carry out various soil tests to determine the soil properties either in the laboratory or on the field (In-situ). Some soil properties like the index properties are readily and easily obtained in the laboratory, however, direct measurements of other properties like the engineering properties including shear strength parameters, hydraulic conductivity, soil water characteristic curves and California Bearing ratios (CBR) are expensive, time consuming and labour intensive. Correlation equations utilize various regression analysis and data mining techniques to extract rules associating basic soil properties with more difficult to measure properties. The concept of the correlation equations has long been applied to estimate soil properties that are difficult to determine. Probably because of the particular difficulty, cost of measurement, and availability of large databases, the most comprehensive research in developing correlation equations has been for the estimation of water retention curve and hydraulic conductivity. Development of correlation equations to estimate soil engineering properties in terms of the soil index properties such as the particle size distribution, organic matter content, bulk density, dry density, specific gravity, void ratio, soil porosity, fines content, and Atterberg limits is now becoming useful [13].There exists a lot of uncertainty in applying correlation equations to other soil conditions different from the soil conditions under which the correlation equations were developed because correlation equations are developed on the basis of a limited database. Thus, there is a need to understand the accuracy and the limit of the correlation equations developed. Previous Work on correlation equations/regression analysisThe development of correlation equations is still an ongoing research topic. The concept was known previously as “surrogate” methods, “rule of Thumb”, “pedo function” [8, 9], “transfer function” [3], and others. With the introduction of the term PTF, it has gained worldwide recognition as a new field in soil science. Three years after its conception, the first paper registered using the term correlation equations is by [12]. A correlation of CBR with plasticity and grading using the concept of suitability index was developed by [7]. In this case, the suitability index is defined as: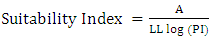 | (1) |
where; A = Percentage passing 2.4mm BS sieve LL = Liquid Limit PI = Plasticity IndexAgarwal and Ghanekar [1] had tried to develop a correlation between CBR values and either liquid limit, plastic limit or plasticity index. However, they failed to found any significant correlation between them. Instead, they did found an improved correlation when they include the optimum moisture content and liquid limit. The correlation is defined as below: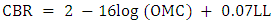 | (2) |
where; OMC = Optimum Moisture Content;LL = Liquid LimitNational Cooperative Highway Research Program [11] of United States of America through the “Guide for Mechanical-Empirical Design of New and Rehabilitated Pavement Structures” had developed some correlations that describe the relationship between soil index properties and CBR values. Two equations were established where one was for non-plastic coarse-grained material and the other was for soils which contains 12% fines and exhibit some plasticity. The best-fitted equation proposed by [11] for clean, coarse-grained soil is shown as below: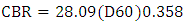 | (3) |
where; D60 = Diameter at 60% passing from grain size distribution (Unit in millimetre).The equation above is limited to D60 values greater than 0.01mm and less than 30mm. For D60 less than 0.01mm, the recommended value of CBR is 5% whereas CBR value of 95% is recommended for D60 greater than 30mm. For plastic, fine-grained soils, the soil index properties chosen to correlate CBR are the percentage passing No.200 U.S. sieve or 0.075mm size sieve and plasticity index. The suggested equation by [11] is shown below: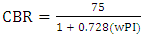 | (4) |
where; w = Percentage passing No.200 U.S. sieve (in decimal), PI = Plasticity Index. Index properties which include; the consistency limits test, water content test, maximum dry density etc. California Bearing Ratio (CBR) is one of the internationally accepted alternative methods of pavement design; it is also employed in soils classification as base and sub-base course materials for their suitability for use in highway design [4].The aim is to establish analytical relationships among Engineering Properties of Lateritic soil as pavement material which thereby provides a quicker and cheaper means of computing the index and strength values of lateritic materials through analytical relations without going through the rigor of the laboratory experiments. In achieving collection of data on soil samples at different borrow pit locations on which various strength and index tests have been carried out. Tests carried out enable their suitability and usability as pavement material and forms the basis of comparison among the through mathematical relations.
2. Methodology
Ÿ Data collection: Data collected for pavement courses on the strength and engineering index properties of lateritic soils as subgrade (14 results were used for the analysis) sub base (12 results employed) and base course (48 results employed) material from quality-controlled laboratories which includes, from Dutum construction company, Ministry of Works and Transport geotechnicallaboratory, Osun and Oyo State, Horizon construction company Osun state, Laboratories and Past project Consultation, where they were carried out in accordance to [5]. The tests are:Ÿ California Bearing Ratio (CBR)Ÿ Particle size analysis (F=% passing sieve 75 microns)Ÿ Atterberg’s LimitŸ Liquid Limit (LL)Ÿ Plastic Limit (PL)Ÿ CompactionŸ Maximum Dry Density (MDD)Ÿ Optimum moisture Content (OMC)Ÿ Analysis of the data by:Use of Least-Square Regression Equation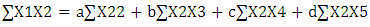 | (1) |
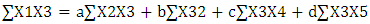 | (2) |
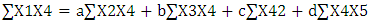 | (3) |
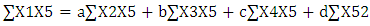 | (4) |
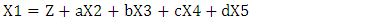 | (5) |
Where, 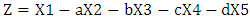 Note, X1=CBR, X2=Liquid limit, X3=Plastic limit, X4=Maximum Dry Density, X5=Optimum moisture content. N.B The number of variables to be determined depends on the forms of equations to be used. For example, CBR against MDD and OMC has three variables so three equations was employed.The equation is
Note, X1=CBR, X2=Liquid limit, X3=Plastic limit, X4=Maximum Dry Density, X5=Optimum moisture content. N.B The number of variables to be determined depends on the forms of equations to be used. For example, CBR against MDD and OMC has three variables so three equations was employed.The equation is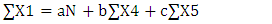 | (6) |
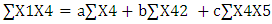 | (7) |
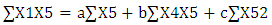 | (8) |
For others, same protocol follows just by interchanging the variable used.Use of SPSS: The data are also imported into SPSS
3. Results
For unsoaked CBR (Base course).Analysis using SPSS as the analyzer gives the followings;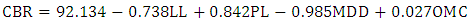 | (1) |
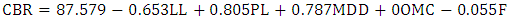 | (2) |
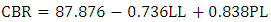 | (3) |
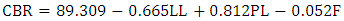 | (4) |
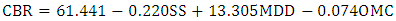 | (5) |
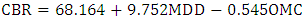 | (6) |
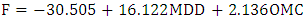 | (7) |
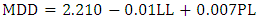 | (8) |
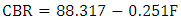 | (9) |
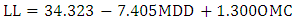 | (10) |
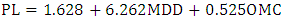 | (11) |
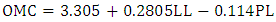 | (12) |
Using the regression formulae;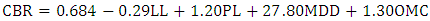 | (13) |
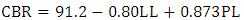 | (14) |
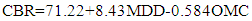 | (15) |
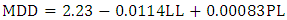 | (16) |
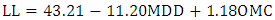 | (17) |
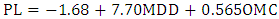 | (18) |
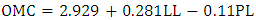 | (19) |
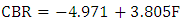 | (20) |
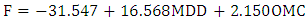 | (21) |
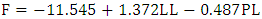 | (22) |
For SubgradeUsing SPSS for the analysis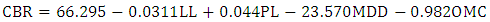 | (23) |
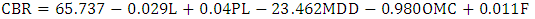 | (24) |
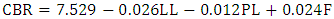 | (25) |
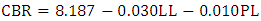 | (26) |
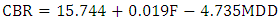 | (27) |
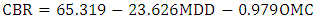 | (28) |
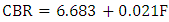 | (29) |
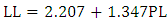 | (30) |
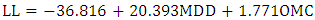 | (31) |
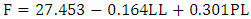 | (32) |
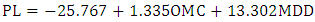 | (33) |
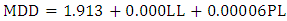 | (34) |
Using the regression formulae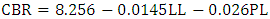 | (35) |
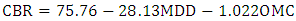 | (36) |
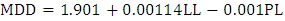 | (37) |
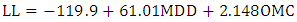 | (38) |
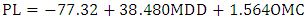 | (39) |
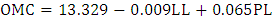 | (40) |
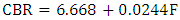 | (41) |
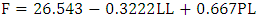 | (42) |
For Sub baseUsing SPSS for the analysis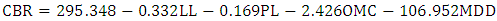 | (43) |
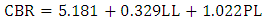 | (44) |
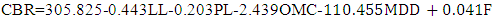 | (45) |
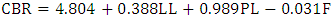 | (46) |
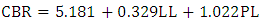 | (47) |
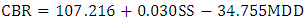 | (48) |
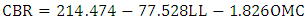 | (49) |
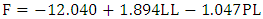 | (50) |
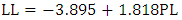 | (51) |
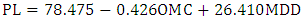 | (52) |
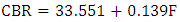 | (53) |
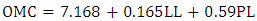 | (54) |
Using the regression formulae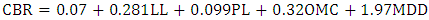 | (55) |
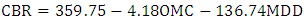 | (56) |
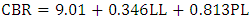 | (57) |
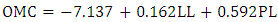 | (58) |
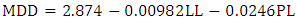 | (59) |
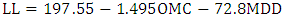 | (60) |
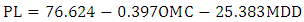 | (61) |
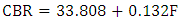 | (62) |
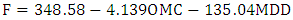 | (63) |
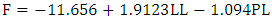 | (64) |
4. Discussions from the Equations
For Base course (From equation 1-22 and Fig. 1-3)Ÿ CBR is inversely proportional to liquid limit, inversely proportional to OMC, directly to MDD and directly proportional to plastic limit.Ÿ Liquid limit is directly proportional to plasticity index. i.e. inversely to Plastic limit and it also varies inversely to MDD and directly to OMCŸ Percent passing sieve 75 microns is inversely proportional to CBR as shown in equation 9 and Fig 1Ÿ Percent passing sieve 75 microns is directly proportional to LL and inversely to PL; so it is directly proportional to plasticity index.Ÿ MDD is directly proportional to PL and inversely to SS and OMC varies inversely to PL.Ÿ OMC varies directly to LL and inverse to PLŸ PL varies directly to both MDD and OMC.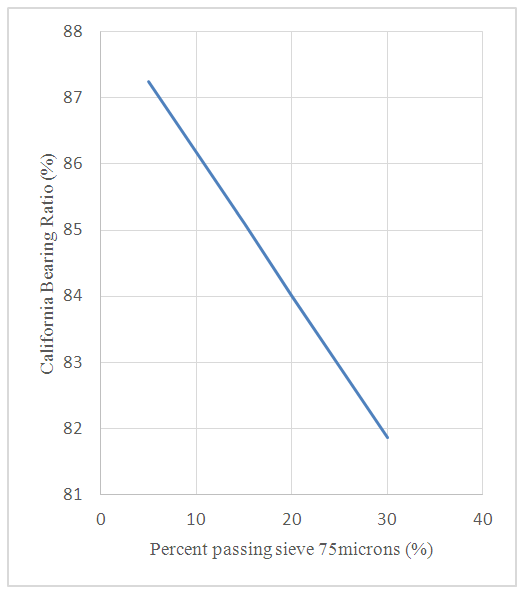 | Figure 1. Showing relations between CBR and F |
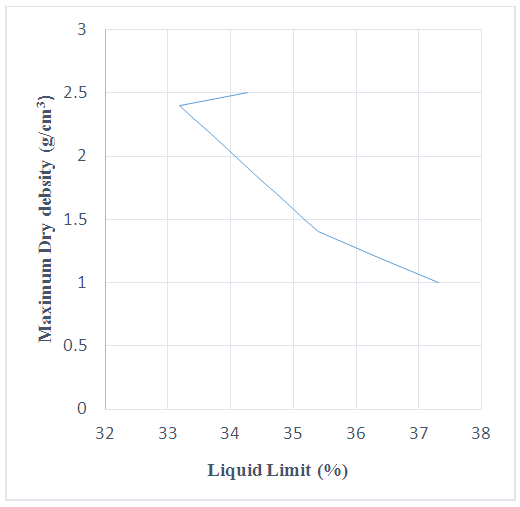 | Figure 2. Showing relations between MDD and LL |
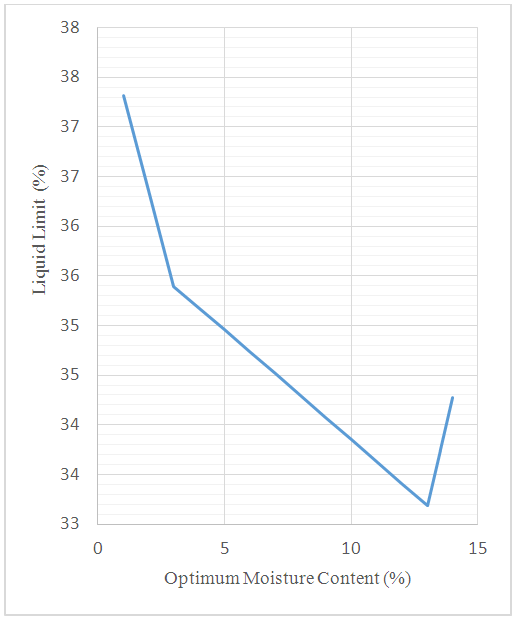 | Figure 3. Showing relations between LL and OMC |
Subgrade (From equation 23-42 and Fig 4)Ÿ CBR varies directly to PL, inversely to LL, MDD and OMC and directly to percent passing sieve 75 microns.Ÿ Percent passing sieve 75 microns varies directly to PL and inverse to LL.Ÿ PL varies directly to OMC and MDDŸ LL varies directly to PL as shown in Fig. 4.0Ÿ MDD varies directly to LL and inversely to PLŸ OMC varies inversely to LL and directly to PL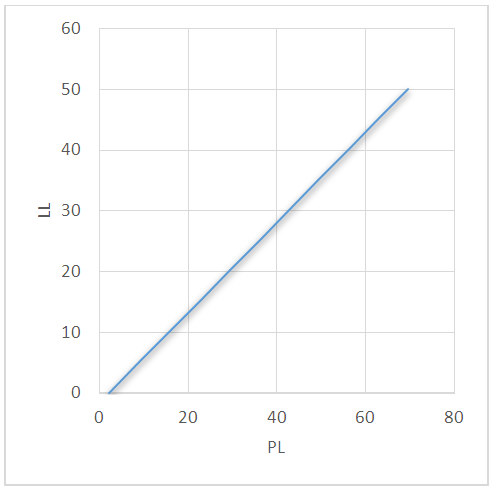 | Figure 4. Showing relations between LL and PL |
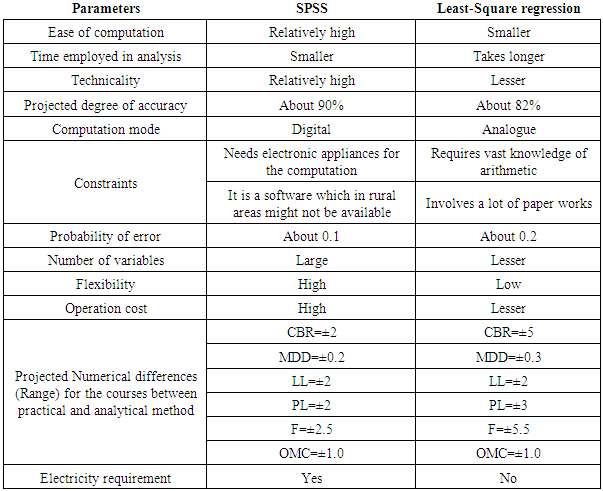
Sub base (From equation 43-64)Ÿ CBR varies inversely to LL, OMC, MDD and directly to percent passing 75 microns sieve and PLŸ Percent passing 75 microns sieve varies directly to LL and inversely to PL, OMC and MDDŸ LL varies directly to PL, inversely to OMC and MDD.Ÿ PL varies inversely to OMC and directly MDDŸ OMC varies directly to both PL and LLŸ MDD varies inversely to both LL and PLTable 1 below shows the operational differences between the two statistical parameter employed in this research work, with SPSS showing the highest degree of accuracy.Table 1. Operational differences between SPSS analysis and Least-square regression analysis
 |
| |
|
5. Conclusions
Ÿ Statistical analysis carried out on the various soil samples shows that there exist correlations among the Engineering Index Properties of the samples.Ÿ Engineering Index Properties of soil samples can be used as a good determinant for the strength characteristics of laterite without going through the rigor of Laboratory experiments.Ÿ SPSS analysis and least square regression method are effective tools for correlation determinant with both giving relatively accurate results with SPSS analysis gives more accurate result compared to least square regression analysis, has more flexibility and higher ease of computations but for smaller construction works it is unadvisable because of the relatively high operational cost and technicality.
6. Recommendations
Ÿ This is to recommend that least square Regression formulae and SPSS analysis can be approximately used to determine the Strength Property (CBR) of a lateritic soil in relation to its Index Properties. Ÿ In order to reduce the probability of error and for further research works, wider range of consultations and number of samples should be used in the analysis, quality controlled laboratory should be consulted for proper efficacy and authenticity of the results to be used and results to be used should not necessarily conform to federal Ministry of Works specifications for roadwork because of its practicability.
References
| [1] | Agarwal, K.B. & Ghanekar, K.D., (1970). Prediction of CBR from Plasticity Characteristics of Soil. Proceeding of 2nd South-east Asian Conference on Soil Engineering, Singapore, Bangkok: Asian Institute of Technology, 571-576. |
| [2] | Bourman, R.P. (1993). Perennial Problems in the study of Laterite: “A Review” Australian Journal of Earth Sciences. 40(4)1387. |
| [3] | Bouma, J. & Van Lanen, H.A.J., (1986). Transfer functions and threshold values: from soil characteristics to land qualities. In: Quantified land evaluation procedures, Proceedings of the international workshop on quantified land evaluation procedures, 27 April - 2 May 1986. Washington, DC. |
| [4] | Braja, M. Das (1998). Principles of Geotechnical Engineering Fourth Edition, California State University. |
| [5] | British Standard Institution (1999). Method of Test for Soil for Civil Engineering Purposes, BS 1377; London British Standard Institution. |
| [6] | Dalvi, Ashok D., Bacon, W. Gordon, Osborne, Robert (2004). The Past and the Future of Nickel Laterite. PDAC 2004, International Convention. |
| [7] | De Graft - Johnson, J.W.S. & Bhatia, H.S., (1969). The Engineering Characteristics of the Lateritic Gravels of Ghana. Proceedings of 7th International Conference on Soil Mechanics and Foundation Engineering, Mexico. August 28-29. Bangkok: Asian Institute of Technology. Vol.2, 13-43. |
| [8] | Kneib, W. & Schroeder, D. (1984). Evaluation of the pedofunction plant-production in Schleswig-Holstein. Zeitschrift fur Pflanzenernahrung und Bodenkunde 147, 425-434. |
| [9] | Lamb, J. & Kneib, W., (1981). Zur quantitativen Erfassung und Bewertung von Pedofunktionen. Mitteilungen der Deutschen Bodenkundlichen Gesellschaft 32, 695-711. |
| [10] | McAtamney, C.F. (1996). “Constructed Wetlands for Waste Water Treatment” Use of Laterite in the Bed medium in Phosphorus and Heavy metal Removal”. Hydrobiologia (Springer Netherlands) 340: 329. |
| [11] | National Cooperative Highway Research Program, (2001). Appendix CC- I. Correlation of CBR Values with Soil Index Guide for Mechanistic and Empirical- Design for New and Rehabilitated Pavement Structures, Final Document. In: Properties. West University Avenue Champaign, Illinois: Ara, In. |
| [12] | Petach, M.C., Wagenet, R.J. and Degloria, S.D., (1991). Regional water-flow and pesticide leaching using simulations with spatially distributed data. Geoderma 48, 245-269. |
| [13] | Young MDB, Gowing JW, Hatibu N., Mahoo, H.M.F. and Payton, R.W. (1999). Assessment and development of pedotransfer functions for semi- arid sub-Saharan Africa. Physics and Chemistry of the Earth Part B-Hydrology, Oceans and Atmosphere 24, 845-849. |



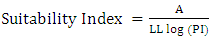
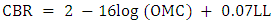
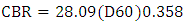
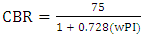
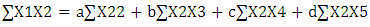
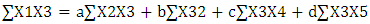
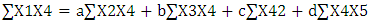
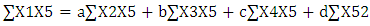
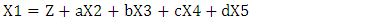
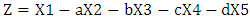 Note, X1=CBR, X2=Liquid limit, X3=Plastic limit, X4=Maximum Dry Density, X5=Optimum moisture content. N.B The number of variables to be determined depends on the forms of equations to be used. For example, CBR against MDD and OMC has three variables so three equations was employed.The equation is
Note, X1=CBR, X2=Liquid limit, X3=Plastic limit, X4=Maximum Dry Density, X5=Optimum moisture content. N.B The number of variables to be determined depends on the forms of equations to be used. For example, CBR against MDD and OMC has three variables so three equations was employed.The equation is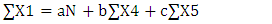
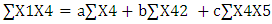
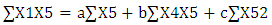
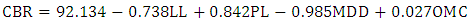
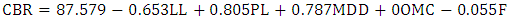
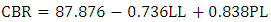
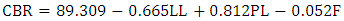
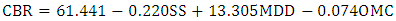
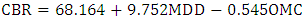
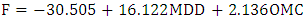
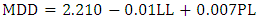
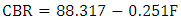
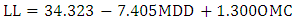
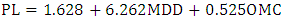
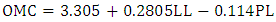
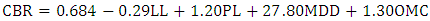
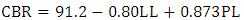
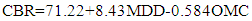
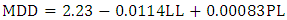
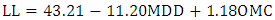
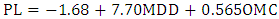
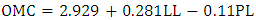
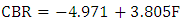
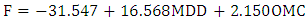
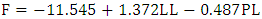
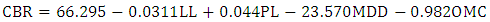
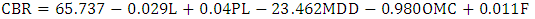
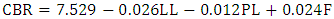
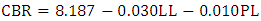
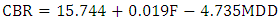
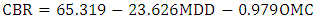
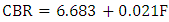
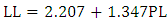
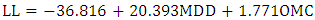
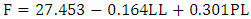
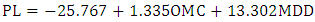
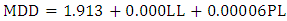
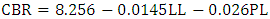
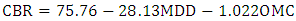
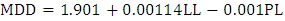
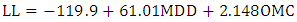
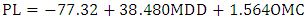
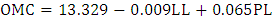
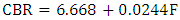
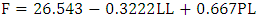
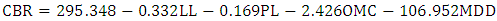
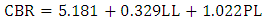
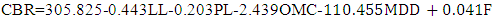
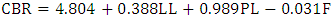
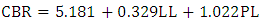
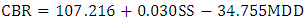
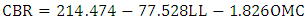
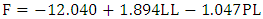
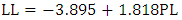
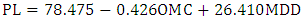
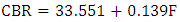
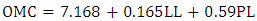
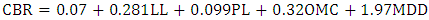
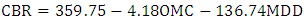
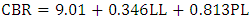
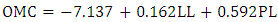
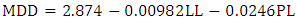
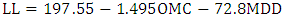
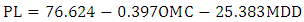
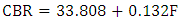
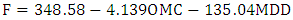
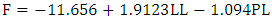




 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML