Yan Rui-Xin, Shen Yan-Jun
School of Architecture and Civil Engineering, Xi'an University of Science and Technology, Xi'an, China
Correspondence to: Shen Yan-Jun, School of Architecture and Civil Engineering, Xi'an University of Science and Technology, Xi'an, China.
| Email: |  |
Copyright © 2015 Scientific & Academic Publishing. All Rights Reserved.
Abstract
The rock mass classification system, BQ (basic quality) system, is the only national system that is suitable to most kinds of rock engineering in China. It uses two main aspects of the rock mass, that is the intact uniaxial compressive strength (UCS) and the rock intactness index (KV), to obtain the rock mass basic quality BQ. The index KV could be measured by the acoustic wave method; however, because this method is an indirect approach to characterize the rock mass structure, misfits often occur between the test results and the actual rock mass characteristics. Therefore, a new parameter KGSI expressed by the GSI (geological strength index) is proposed to replace the original KV, and a new method is presented to express the value of BQ as a function of the UCS and the KGSI. Then, the underground excavations at five hydropower stations are chosen as examples to study the relationship between the revised BQ and the each of the international systems (RMR, Q or RMi), In addition, the applicability of the RMR, Q, or RMi system is discussed for China’s rock characteristics and geological conditions, and suggestions are given for improving the revised BQ system.
Keywords:
Revised BQ system, KGSI, International rock mass classification systems
Cite this paper: Yan Rui-Xin, Shen Yan-Jun, Correlation of Revised BQ System in China and the International Rock Mass Classification Systems, Journal of Civil Engineering Research, Vol. 5 No. 2, 2015, pp. 33-38. doi: 10.5923/j.jce.20150502.03.
1. Introduction
BQ system (a China’s national standard - GB 50218-94 Standard for engineering classification of rock masses, 1994) [1], is the only national code which is suitable to most kinds of rock engineering in China. This system is developed based on lots of rock engineering cases in China and also referred to many domestic and international rock mass classification systems. Two steps need to be used the BQ system for rock mass classification. Firstly, two common characteristics that represent rock engineering, intact rock strength index and rock integrity index are selected to determine the basic quality (BQ) of rock mass. Then, three more detailed correction factors in the rock engineering, the groundwater condition, the orientation of joints related to the excavation, and the in-situ stress state, are considered to acquire the final rock mass quality [BQ]. This approach has been highly recognized and widely used in many rock projects in China.The two quantitative indexes, namely, the intact uniaxial compressive strength (UCS) and the rock integrity index (Kv) are used in the basic quality calculation BQ. It is relatively simple to decide the UCS value directly by means of the intact un-drained rock uniaxial compressive strength σci or the point-load strength index IS(50). And the Kv value can be obtained from the rock mass acoustic P-wave velocity Vpm and the intact rock velocity Vpr by following equation: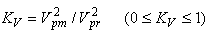 | (1) |
The rock mass acoustic velocity can be obtained from the in situ measurement, while the intact rock velocity can be measured from the core samples.The acoustic velocity of the rock mass is not only related to the rock composition and structure characteristics, but also associated to the discontinuity of the rock mass, cementation of the joints, and the groundwater conditions. Therefore, the index Kv is regarded as a comprehensive assessment to the rock mass integrity.However, it is difficult to ensure that all the rock mass can be tested by the acoustic wave in engineering applications. Moreover, the measurement results may be not able to accurately reflect the real rock integrity situations. In certain cases, the KV value even can be greater than 1. For example, in a thin-bedded sedimentary rock, the in-situ tectonic compression may cause the measured results of the velocity (Vpm) to be very high, yet the core sample results (Vpr) may show a low value due to the stress unloading. On the other hand, the velocity of a water saturated rock is greater than that when the rock is in the dry condition (Abe and Saito, 1976; Shao et al., 2010; Torok and Vasarhelyi, 2010; Yang and King, 1986) [2-5]. For those rocks with high water content, the water content of the core sample could be reduced obviously and lead to the value of Vpr less than the Vpm. Therefore, it is necessary to optimize the quantification expression of the rock integrity index Kv. In this paper, a new index KGSI is proposed based on the GSI chart to replace the former one Kv. The value KGSI can be obtained by means of the GSI chart, so that a revised and quantitative equation of BQ is established to evaluate the rock mass quality.During engineering application, it is emphasized to compare the BQ system to the common international systems (e.g., RMR, Q, RMi). It is also recommended that at least two systems should be used in each rock project in China. However, due to the different parameters, the evaluation results in the two methods are probably inconsistent. The correlations of the BQ system and the common-used international systems are also different for different rock projects in China. It is necessary to study the correlations of the BQ system to those international systems and determine the applicability of different systems in views of China’s rock characteristics and geological conditions.
2. The BQ System in China
2.1. The Basic Quality of Rock Mass (BQ)
In China, 103 domestic rock engineering cases including 30 metallurgical projects, 24 hydropower projects, 21 national defending projects, 13 railway projects, 8 coal mine projects, 6 civil engineering projects and 1 civil air defense project are chosen as statistical samples in the BQ system. The aforementioned two indexes, the UCS and the KV, are used as the evaluation variables, and a linear regression equation is obtained as following,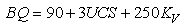 | (2) |
Where, 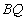 is the basic quality value of rock mass, the UCS is the intact unaxial compressive strength, MPa; and the KV is the rock intactness index.Two constraint conditions must be considered during the use of the BQ system. (1) when UCS>90KV+30, it should use the equation UCS=90KV+30 for the BQ calculation. (2) when UCS KV >0.4+0.04UCS, it should use the equation KV =0.4+0.04UCS and the value UCS to determine the BQ.The BQ system classifies rock masses as 5 categories. Each class and the value of
is the basic quality value of rock mass, the UCS is the intact unaxial compressive strength, MPa; and the KV is the rock intactness index.Two constraint conditions must be considered during the use of the BQ system. (1) when UCS>90KV+30, it should use the equation UCS=90KV+30 for the BQ calculation. (2) when UCS KV >0.4+0.04UCS, it should use the equation KV =0.4+0.04UCS and the value UCS to determine the BQ.The BQ system classifies rock masses as 5 categories. Each class and the value of 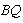 in the 5 categories are listed in the following: Class I,
in the 5 categories are listed in the following: Class I, 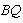 > 550, Class II,
> 550, Class II, 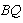 = 550-451; Class III,
= 550-451; Class III, 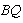 = 450-351; Class IV,
= 450-351; Class IV, 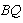 = 350-251 and Class V,
= 350-251 and Class V, 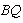 < 250.
< 250.
2.2. The Main Correction Factors of the BQ System
Considering the influence of the external geological conditions and the excavation feature to the rock mass quality, three coefficients (i.e., the groundwater condition parameter K1, the orientation parameter of joints related to the excavation K2 and the in-situ stress condition parameter K3) are used to revise the basic value BQ in Eq. (2). The rock mass quality [BQ] can then be expressed as (GB 50218-94 Standard for engineering classification of rock masses, 1994):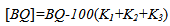 | (3) |
Where, [BQ] is the corrected value of the BQ, and K1-K3 are coefficients factors and listed in Table.1.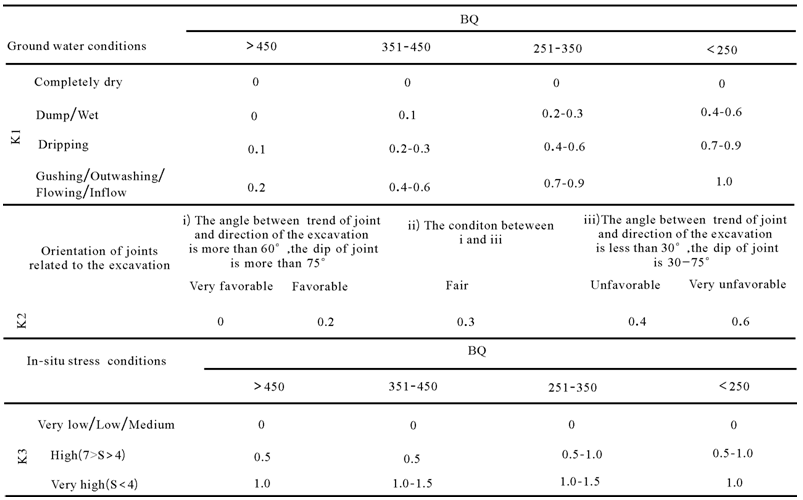 | Table 1. Three correlation coefficients 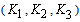 in the BQ system (GB 50218-94 Standard for engineering classification of rock masses, 1994) in the BQ system (GB 50218-94 Standard for engineering classification of rock masses, 1994) |
3. New Proposed Rock Integrity Index Kgsi Based on the Gsi
As the above discussion, the rock integrity index KV is difficult to measure accurately in practice, and the acoustic wave results also exist large dispersion, which may not directly and precisely reflect the actual rock mass characteristics. This is most likely to have the misfit between the predicted result from the BQ method and the actual rock mass characteristics.In order to improve the BQ system, it needs to use a direct method to characterize rock mass properties and replace the indirect acoustic wave method for rock mass characteristics. At present, the GSI chart is a widely used tool to indicate the rock mass characteristics. The main purpose of the rock integrity index (KV) in the BQ system is to quantitatively express the rock mass characteristics, and it is the wholly same to the original intention of the GSI system. Therefore, this consistence provides an important theoretical foundation for establishing the relation of the two methods.However, as one of the two parameters in the basic BQ equation, the rock integrity index KV is so important in the BQ system that the original equation would be no longer suitable for application if the KV is replaced by the GSI value. This would destroy totally the core thought of the BQ system. Moreover, even we use the GSI to replace the KV value; it is too difficult to build a new BQ equation, which needs many engineering cases to prove its applicability. However, it is possible to find a correlation between the KV and the GSI value by using plenty of in-situ tests. The tests on the correlation of the KV and the GSI were done in different excavation sections of the Layer III-V in the underground opening in Dagangshan hydropower station of China, 106 boreholes were assigned to measure the acoustic velocities and the corresponding GSI value. Finally, 83 comparison values were obtained after discarding those outliers. In addition, measurement data in the adits of Dagangshan hydropower station and in two other large-scale underground excavations in China were also collected to analyze the KV-GSI correlation (as Figure 1). The fitting equation can be expressed by the S-shaped growth function as below: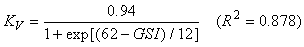 | (4) |
As we known, for the same kind of rock, the ratio of the deformation modulus of the rock mass to the one of the intact rock is approximately equal to the square of their wave velocity ratio as: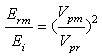 | (5) |
It should be particularly mentioned that Hoek [6] firstly suggested the following equation using the expression of the most popular tool of GSI as the in-situ measured rock mass deformation modulus data from China and Taiwan,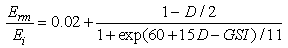 | (6) |
Where, Erm/Ei is the deformation modulus ratio of the rock mass and the intact rock, and D is the disturbance factor of the excavation.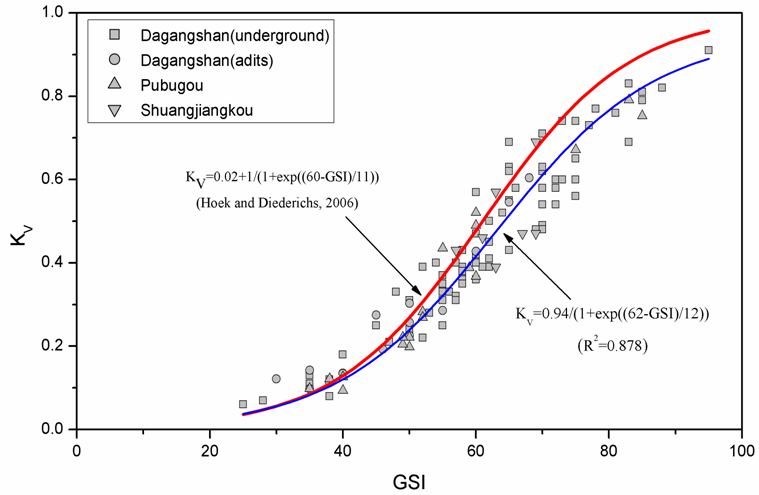 | Figure 1. The correlation of the KV and the GSI value by the curve-fitting (blue line) and the Hoek-Diedenichs’s recommendation (red line, Hoek and Diederichs, 2006a) |
The controlled blasting (smooth blasting, pre-split blasting, et.al) is normally used in the excavation to control the profile of the chambers. Therefore, the corresponding index D should be zero in Hoek’s equation. Hence, Eq. (6) can be simplified as following form when the disturbance factor D = 0.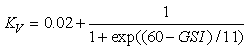 | (7) |
There is a good consistency between the simplified equation (Eq.7) and the fitting equation (Eq.4), except that the values in Eq.4 are slightly smaller than the ones obtained from Eq.6 (Fig.1). This may be caused by the fact that disturbance factor still has influence in the kv in the controlled blasting method. Therefore, we establish a new integrity index/factor, KGSI to replace the original KV as a comprehensive correlation of Eqs. (4) and (7). The following equation is used for the BQ system as.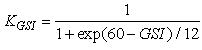 | (8) |
4. The Comparative Analysis of the Rock Mass Quality Results in the Revised BQ and Other Sustems
Now several underground excavations or adits in five hydropower stations in China: ① Diversion tunnels at Jinping II hydropower station site, ② Exploration adits PD09 at Shuangjiangkou hydropower station site③ Exploration adits PD204 at Nuozhadu hydropower station site, ④ Exploration adits PD02, PD14 at Laxiwa hydropower station site and ⑤ Powerhouse at Dagangshan hydropower station site) are chosen as examples to study the relationship between the BQ system and the commonly-used ones (RMR, Q and RMi [7-10]). Furthermore, the applicable conditions of the RMR, Q and RMi systems are also discussed in view of the unique rock characteristics and geological conditions in China.According to the most widely adopted procedure in the world, the rock mass is usually divided into 5 classes from Class-I (very Good) to Class-V (very Poor). The numbers of statistical samples are 38 (Jinping II), 38 (Laxiwa), 35 (Shuangjiangkou), 51(Nuozhadu) and 25(Dagangshan) respectively. Based on the category standards of the four systems, three charts for the sample distributing comparisons ([BQ]Rev-RMR, [BQ]Rev -Q and [BQ]Rev -RMi) are established in all five projects. Because the RMi system uses a parameter 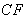 to express the very high stress condition (S<5), its representation is totally different from the parameter SL in the normal stress condition (S>5). In order to maintain the comparability of the results in the RMi system, all of the samples which are located in the very high stress conditions (Jinping II and Shuangjiangkou hydropower stations) are not listed in the [BQ]Rev -RMi chart.Some conclusions can be drawn by the above comparable charts of the revised BQ and the commonly-used systems (RMR, Q, RMi) as follows (as Figure 2):(1) The BQ system has relatively strong correlations with all of the commonly-used systems, but its correlations with the RMR and RMi systems are better.(2) The correlation between the revised BQ and the RMR system on the good rock mass quality condition is apparently better than that on the poor conditions. In addition, the sample category results given by the RMR are generally one grade higher than those in the revised BQ system on the poor conditions (i.e., Class IV-V). It should be noted that the sample categories obtained by the RMR still keep good relationships with those given by the BQ system on the very high stress conditions (i.e., in Jinping II and Shuangjiangkou) even though the in-situ stress parameters are not considered in the RMR system .(3) The correlation of the revised BQ and the Q system is very poor on the very high stress conditions. The category results of the samples by the revised BQ system is generally 1 to 2 grades higher than those by the Q system. However, the correlations are relatively good on the medium stress level conditions, such as in the Laxiwa, Nuozhadu and Dagangshan hydropower stations.(4) The correlation between the revised BQ and the RMi is, in general, good on the medium stress conditions. However, for the Laxiwa hydropower station (with the good integrity rocks), the category results of the samples by the revised BQ system is generally 1 grade higher than that by the RMi system. On the contrast, the result by the revised BQ system is 1 grade lower than that by the RMi system for the poor integrity rocks at the Dagangshan hydropower station.
to express the very high stress condition (S<5), its representation is totally different from the parameter SL in the normal stress condition (S>5). In order to maintain the comparability of the results in the RMi system, all of the samples which are located in the very high stress conditions (Jinping II and Shuangjiangkou hydropower stations) are not listed in the [BQ]Rev -RMi chart.Some conclusions can be drawn by the above comparable charts of the revised BQ and the commonly-used systems (RMR, Q, RMi) as follows (as Figure 2):(1) The BQ system has relatively strong correlations with all of the commonly-used systems, but its correlations with the RMR and RMi systems are better.(2) The correlation between the revised BQ and the RMR system on the good rock mass quality condition is apparently better than that on the poor conditions. In addition, the sample category results given by the RMR are generally one grade higher than those in the revised BQ system on the poor conditions (i.e., Class IV-V). It should be noted that the sample categories obtained by the RMR still keep good relationships with those given by the BQ system on the very high stress conditions (i.e., in Jinping II and Shuangjiangkou) even though the in-situ stress parameters are not considered in the RMR system .(3) The correlation of the revised BQ and the Q system is very poor on the very high stress conditions. The category results of the samples by the revised BQ system is generally 1 to 2 grades higher than those by the Q system. However, the correlations are relatively good on the medium stress level conditions, such as in the Laxiwa, Nuozhadu and Dagangshan hydropower stations.(4) The correlation between the revised BQ and the RMi is, in general, good on the medium stress conditions. However, for the Laxiwa hydropower station (with the good integrity rocks), the category results of the samples by the revised BQ system is generally 1 grade higher than that by the RMi system. On the contrast, the result by the revised BQ system is 1 grade lower than that by the RMi system for the poor integrity rocks at the Dagangshan hydropower station.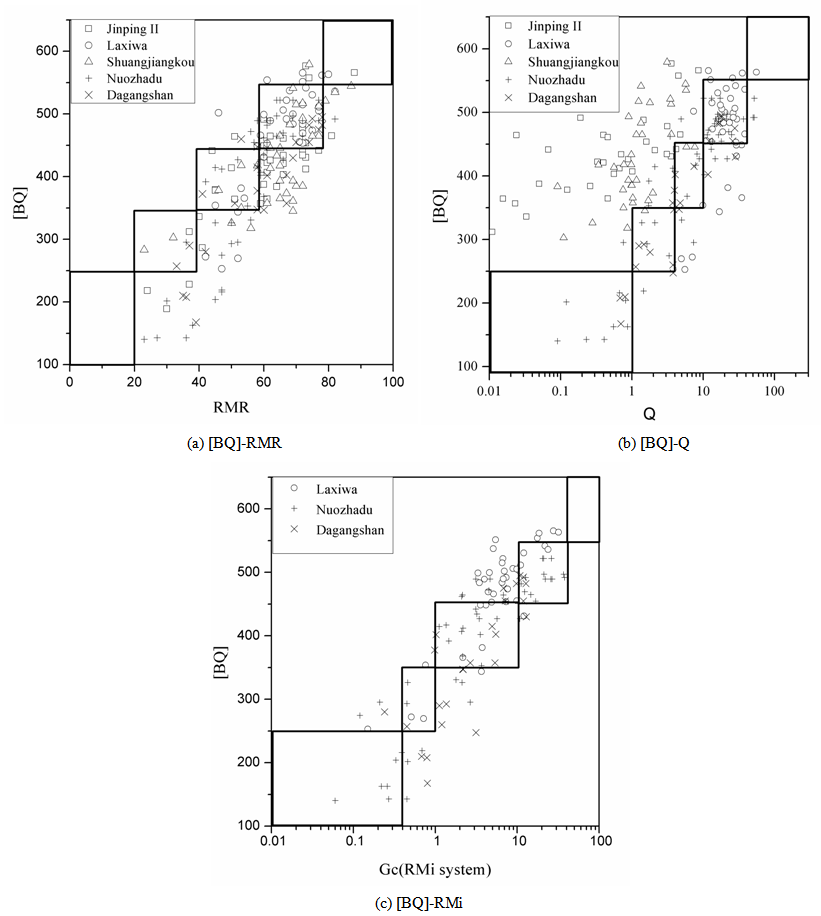 | Figure 2. The samples distributing comparison charts between the revised BQ and the international systems (RMR, Q, and RMi) in five projects |
4.1. Discussion on the Statistical Correlation Results
Based on the statistical results in the correlations of the revised BQ system and the common-used ones, a further applicable discussion of these commonly-used systems (RMR, Q, RMi) is performed in view of the unique rock characteristics and geological conditions in China. (1) The conclusion of the sample category results by the RMR system having one grade higher than those by the revised BQ system on the poor conditions has been proved in several projects in China. And the reasons might be explained in general by Bieniawski’s study (Bieniawski, 1989) on the relationship between the GSI and the RMR: 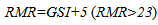 | (9) |
Regardless of the UCS values the category always belongs to Class-V. Yet for the rock mass quality in ranges of 18<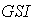 <35, the category by the RMR system should be classified as Class-IV following the Eq. (9). Considering that the BQ(or revised BQ) system is derived from the statistical results in many rock engineering projects and its applicability have been validated in China, this concludes that the RMR system is not applicable on the status of the poor rock mass quality in China.This fact that the categories given by the RMR system have good relationships with those by the revised BQ system in very high stress conditions implies that the correlation coefficient K3 tends to be risky. Therefore, it is necessary to increase the value K3 in the very high stress conditions (
<35, the category by the RMR system should be classified as Class-IV following the Eq. (9). Considering that the BQ(or revised BQ) system is derived from the statistical results in many rock engineering projects and its applicability have been validated in China, this concludes that the RMR system is not applicable on the status of the poor rock mass quality in China.This fact that the categories given by the RMR system have good relationships with those by the revised BQ system in very high stress conditions implies that the correlation coefficient K3 tends to be risky. Therefore, it is necessary to increase the value K3 in the very high stress conditions (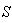 <5).(2) The correlations of the revised BQ system and the Q system are relatively good in the medium stress level conditions, even in the status of the poor rock mass quality. It has been proved that the revised BQ system is quite inappropriate to assess the rock mass quality on the very high stress conditions (S<5). For example, during the construction of the Jinping II hydropower underground station, the original rock mass quality evaluated by the BQ system was relatively good as the category of Class-II. However, due to the influence of ultra-high in-situ stress the rock bursts had happened many times and resulted in the reduction in the rock mass quality. The rock mass quality, if assessed by the Q system, should be Class-IV or Class-IV, which is suitable to the actual conditions. Therefore, the Q system is recommended as the preferred tool to assess the rock mass quality in very high in-situ stress conditions.(3) The correlation of the BQ and the RMi is discussed only in the normal stress conditions. Some misfits appear in individual project, for instance, in the Laxiwa hydropower station. The uniaxial compressive strength in this project is relatively high (
<5).(2) The correlations of the revised BQ system and the Q system are relatively good in the medium stress level conditions, even in the status of the poor rock mass quality. It has been proved that the revised BQ system is quite inappropriate to assess the rock mass quality on the very high stress conditions (S<5). For example, during the construction of the Jinping II hydropower underground station, the original rock mass quality evaluated by the BQ system was relatively good as the category of Class-II. However, due to the influence of ultra-high in-situ stress the rock bursts had happened many times and resulted in the reduction in the rock mass quality. The rock mass quality, if assessed by the Q system, should be Class-IV or Class-IV, which is suitable to the actual conditions. Therefore, the Q system is recommended as the preferred tool to assess the rock mass quality in very high in-situ stress conditions.(3) The correlation of the BQ and the RMi is discussed only in the normal stress conditions. Some misfits appear in individual project, for instance, in the Laxiwa hydropower station. The uniaxial compressive strength in this project is relatively high (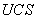 = 95-110MPa), most of rock mass qualities belong to the good or very good level. As the in-situ survey, the value of GSI = 55-60, it means the value of KGSI = 0.54-0.57 and the rock mass belong to Class-II as the revised BQ system. However, the value of GC are from 14.3 to 18.7 by the RMi system, it means that the rock mass belongs to Class-II.From the above example, the sensitivity to the integrity degree in the revised BQ system is higher than that in the RMi system for those high strength rocks (UCS>100MPa). Cindering the economy and the safety in the underground engineering, the RMi system is not so appropriate to evaluate those hard rocks with the extreme integrity degree (very good case and very poor case).(4) In order to assure the accuracy of the rock mass classification in the condition of ultrahigh in-situ stress, the correlation coefficient K3 in the revised BQ system is suggested to increase to 2.0 for the Class I-II, Class V and 2.0-2.5 for the Class III-IV. Moreover, the applied conditions should be changed from
= 95-110MPa), most of rock mass qualities belong to the good or very good level. As the in-situ survey, the value of GSI = 55-60, it means the value of KGSI = 0.54-0.57 and the rock mass belong to Class-II as the revised BQ system. However, the value of GC are from 14.3 to 18.7 by the RMi system, it means that the rock mass belongs to Class-II.From the above example, the sensitivity to the integrity degree in the revised BQ system is higher than that in the RMi system for those high strength rocks (UCS>100MPa). Cindering the economy and the safety in the underground engineering, the RMi system is not so appropriate to evaluate those hard rocks with the extreme integrity degree (very good case and very poor case).(4) In order to assure the accuracy of the rock mass classification in the condition of ultrahigh in-situ stress, the correlation coefficient K3 in the revised BQ system is suggested to increase to 2.0 for the Class I-II, Class V and 2.0-2.5 for the Class III-IV. Moreover, the applied conditions should be changed from 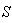 <4 to
<4 to 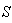 <5.
<5.
5. Conclusions
(1) In view of the drawback of the integrity index KV, by means of the empirical relations between GSI and KV, a new parameter KGSI expressed by the GSI is proposed to replace the original KV. The GSI – KGSI correlation expression is also established to obtain the value KGSI conveniently. It should be noted that this chart can be only suitable to those tunnels where a slight disturbance appears to the surrounding rocks.(2) The underground excavations in five hydropower stations in China are chosen to study the relationship between the BQ and the other systems. The RMR is not applicable on the status of the poor rock mass quality (Class IV-V), while the Q is suitable to evaluate all kinds of the rock mass characteristics on the normal stress conditions. And the RMi is not very appropriate to evaluate the hard rocks (for UCS > 100MPa) with the extreme integrity degrees. Furthermore, the Q is recommended as the preferred method in the three systems for assessing to the rock mass in the very high in-situ stress condition.
References
| [1] | The National Standards compilation group of People′s Republic of China, 1994. GB 50218–94 Standard for engineering classification of rock masses.Beijing:China Planning Press, 1-22. (in Chinese) |
| [2] | Abe, M., Saito, T., 1976. Effect of water content in cracks on the elastic wave velocity in rock. Nippon Kogyo Kaishi/Journal of the Mining and Metallurgical Institute of Japan 92, 73-78. |
| [3] | Shao, M., Li, L., Li, Z., 2010. Elastic wave velocity and mechanical properties of sandstone under different water contents at Longyou grottoes. Yanshilixue Yu Gongcheng Xuebao/Chinese Journal of Rock Mechanics and Engineering 29, 3514-3518. |
| [4] | Torok, A., Vasarhelyi, B., 2010. The influence of fabric and water content on selected rock mechanical parameters of travertine, examples from Hungary. Eng Geol 115, 237-245. |
| [5] | Yang, H.J.P., King, M.S., 1986. Study of elastic wave velocities in dry and water-saturated, regularly-jointed rock masses. International journal of rock mechanics and mining sciences & geomechanics abstracts 23, 277-280. |
| [6] | Hoek, E., Diederichs, M.S., 2006b. Empirical estimation of rock mass modulus. Int J Rock Mech Min 43, 203-215. |
| [7] | Bieniawski, Z.T., 1973. Engineering classifications of jointed rock masses. Trans S Afr Inst Civ Eng 15(12), 335-344. |
| [8] | Bieniawski, Z.T., 1989. Engineering rock mass classification. New York : Wiley Interscience. |
| [9] | Barton, N., Lien, R., Lunde, J., 1974. Engineering classification of rock masses for the design of tunnel support. Rock Mech Rock Eng 6, 189-236. |
| [10] | Palmstrom, A., 1996a. Characterizing rock masses by the RMi for use in practical rock engineering .1. The development of the Rock Mass index (RMi). Tunn Undergr Sp Tech 11, 175-188. |

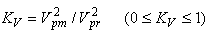
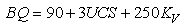
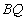 is the basic quality value of rock mass, the UCS is the intact unaxial compressive strength, MPa; and the KV is the rock intactness index.Two constraint conditions must be considered during the use of the BQ system. (1) when UCS>90KV+30, it should use the equation UCS=90KV+30 for the BQ calculation. (2) when UCS KV >0.4+0.04UCS, it should use the equation KV =0.4+0.04UCS and the value UCS to determine the BQ.The BQ system classifies rock masses as 5 categories. Each class and the value of
is the basic quality value of rock mass, the UCS is the intact unaxial compressive strength, MPa; and the KV is the rock intactness index.Two constraint conditions must be considered during the use of the BQ system. (1) when UCS>90KV+30, it should use the equation UCS=90KV+30 for the BQ calculation. (2) when UCS KV >0.4+0.04UCS, it should use the equation KV =0.4+0.04UCS and the value UCS to determine the BQ.The BQ system classifies rock masses as 5 categories. Each class and the value of 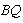 in the 5 categories are listed in the following: Class I,
in the 5 categories are listed in the following: Class I, 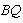 > 550, Class II,
> 550, Class II, 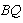 = 550-451; Class III,
= 550-451; Class III, 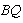 = 450-351; Class IV,
= 450-351; Class IV, 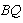 = 350-251 and Class V,
= 350-251 and Class V, 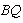 < 250.
< 250.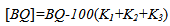

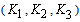 in the BQ system (GB 50218-94 Standard for engineering classification of rock masses, 1994)
in the BQ system (GB 50218-94 Standard for engineering classification of rock masses, 1994)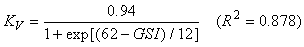
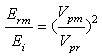
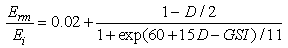

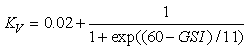
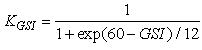
 to express the very high stress condition (S<5), its representation is totally different from the parameter SL in the normal stress condition (S>5). In order to maintain the comparability of the results in the RMi system, all of the samples which are located in the very high stress conditions (Jinping II and Shuangjiangkou hydropower stations) are not listed in the [BQ]Rev -RMi chart.Some conclusions can be drawn by the above comparable charts of the revised BQ and the commonly-used systems (RMR, Q, RMi) as follows (as Figure 2):(1) The BQ system has relatively strong correlations with all of the commonly-used systems, but its correlations with the RMR and RMi systems are better.(2) The correlation between the revised BQ and the RMR system on the good rock mass quality condition is apparently better than that on the poor conditions. In addition, the sample category results given by the RMR are generally one grade higher than those in the revised BQ system on the poor conditions (i.e., Class IV-V). It should be noted that the sample categories obtained by the RMR still keep good relationships with those given by the BQ system on the very high stress conditions (i.e., in Jinping II and Shuangjiangkou) even though the in-situ stress parameters are not considered in the RMR system .(3) The correlation of the revised BQ and the Q system is very poor on the very high stress conditions. The category results of the samples by the revised BQ system is generally 1 to 2 grades higher than those by the Q system. However, the correlations are relatively good on the medium stress level conditions, such as in the Laxiwa, Nuozhadu and Dagangshan hydropower stations.(4) The correlation between the revised BQ and the RMi is, in general, good on the medium stress conditions. However, for the Laxiwa hydropower station (with the good integrity rocks), the category results of the samples by the revised BQ system is generally 1 grade higher than that by the RMi system. On the contrast, the result by the revised BQ system is 1 grade lower than that by the RMi system for the poor integrity rocks at the Dagangshan hydropower station.
to express the very high stress condition (S<5), its representation is totally different from the parameter SL in the normal stress condition (S>5). In order to maintain the comparability of the results in the RMi system, all of the samples which are located in the very high stress conditions (Jinping II and Shuangjiangkou hydropower stations) are not listed in the [BQ]Rev -RMi chart.Some conclusions can be drawn by the above comparable charts of the revised BQ and the commonly-used systems (RMR, Q, RMi) as follows (as Figure 2):(1) The BQ system has relatively strong correlations with all of the commonly-used systems, but its correlations with the RMR and RMi systems are better.(2) The correlation between the revised BQ and the RMR system on the good rock mass quality condition is apparently better than that on the poor conditions. In addition, the sample category results given by the RMR are generally one grade higher than those in the revised BQ system on the poor conditions (i.e., Class IV-V). It should be noted that the sample categories obtained by the RMR still keep good relationships with those given by the BQ system on the very high stress conditions (i.e., in Jinping II and Shuangjiangkou) even though the in-situ stress parameters are not considered in the RMR system .(3) The correlation of the revised BQ and the Q system is very poor on the very high stress conditions. The category results of the samples by the revised BQ system is generally 1 to 2 grades higher than those by the Q system. However, the correlations are relatively good on the medium stress level conditions, such as in the Laxiwa, Nuozhadu and Dagangshan hydropower stations.(4) The correlation between the revised BQ and the RMi is, in general, good on the medium stress conditions. However, for the Laxiwa hydropower station (with the good integrity rocks), the category results of the samples by the revised BQ system is generally 1 grade higher than that by the RMi system. On the contrast, the result by the revised BQ system is 1 grade lower than that by the RMi system for the poor integrity rocks at the Dagangshan hydropower station.
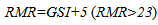
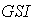 <35, the category by the RMR system should be classified as Class-IV following the Eq. (9). Considering that the BQ(or revised BQ) system is derived from the statistical results in many rock engineering projects and its applicability have been validated in China, this concludes that the RMR system is not applicable on the status of the poor rock mass quality in China.This fact that the categories given by the RMR system have good relationships with those by the revised BQ system in very high stress conditions implies that the correlation coefficient K3 tends to be risky. Therefore, it is necessary to increase the value K3 in the very high stress conditions (
<35, the category by the RMR system should be classified as Class-IV following the Eq. (9). Considering that the BQ(or revised BQ) system is derived from the statistical results in many rock engineering projects and its applicability have been validated in China, this concludes that the RMR system is not applicable on the status of the poor rock mass quality in China.This fact that the categories given by the RMR system have good relationships with those by the revised BQ system in very high stress conditions implies that the correlation coefficient K3 tends to be risky. Therefore, it is necessary to increase the value K3 in the very high stress conditions (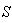 <5).(2) The correlations of the revised BQ system and the Q system are relatively good in the medium stress level conditions, even in the status of the poor rock mass quality. It has been proved that the revised BQ system is quite inappropriate to assess the rock mass quality on the very high stress conditions (S<5). For example, during the construction of the Jinping II hydropower underground station, the original rock mass quality evaluated by the BQ system was relatively good as the category of Class-II. However, due to the influence of ultra-high in-situ stress the rock bursts had happened many times and resulted in the reduction in the rock mass quality. The rock mass quality, if assessed by the Q system, should be Class-IV or Class-IV, which is suitable to the actual conditions. Therefore, the Q system is recommended as the preferred tool to assess the rock mass quality in very high in-situ stress conditions.(3) The correlation of the BQ and the RMi is discussed only in the normal stress conditions. Some misfits appear in individual project, for instance, in the Laxiwa hydropower station. The uniaxial compressive strength in this project is relatively high (
<5).(2) The correlations of the revised BQ system and the Q system are relatively good in the medium stress level conditions, even in the status of the poor rock mass quality. It has been proved that the revised BQ system is quite inappropriate to assess the rock mass quality on the very high stress conditions (S<5). For example, during the construction of the Jinping II hydropower underground station, the original rock mass quality evaluated by the BQ system was relatively good as the category of Class-II. However, due to the influence of ultra-high in-situ stress the rock bursts had happened many times and resulted in the reduction in the rock mass quality. The rock mass quality, if assessed by the Q system, should be Class-IV or Class-IV, which is suitable to the actual conditions. Therefore, the Q system is recommended as the preferred tool to assess the rock mass quality in very high in-situ stress conditions.(3) The correlation of the BQ and the RMi is discussed only in the normal stress conditions. Some misfits appear in individual project, for instance, in the Laxiwa hydropower station. The uniaxial compressive strength in this project is relatively high (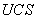 = 95-110MPa), most of rock mass qualities belong to the good or very good level. As the in-situ survey, the value of GSI = 55-60, it means the value of KGSI = 0.54-0.57 and the rock mass belong to Class-II as the revised BQ system. However, the value of GC are from 14.3 to 18.7 by the RMi system, it means that the rock mass belongs to Class-II.From the above example, the sensitivity to the integrity degree in the revised BQ system is higher than that in the RMi system for those high strength rocks (UCS>100MPa). Cindering the economy and the safety in the underground engineering, the RMi system is not so appropriate to evaluate those hard rocks with the extreme integrity degree (very good case and very poor case).(4) In order to assure the accuracy of the rock mass classification in the condition of ultrahigh in-situ stress, the correlation coefficient K3 in the revised BQ system is suggested to increase to 2.0 for the Class I-II, Class V and 2.0-2.5 for the Class III-IV. Moreover, the applied conditions should be changed from
= 95-110MPa), most of rock mass qualities belong to the good or very good level. As the in-situ survey, the value of GSI = 55-60, it means the value of KGSI = 0.54-0.57 and the rock mass belong to Class-II as the revised BQ system. However, the value of GC are from 14.3 to 18.7 by the RMi system, it means that the rock mass belongs to Class-II.From the above example, the sensitivity to the integrity degree in the revised BQ system is higher than that in the RMi system for those high strength rocks (UCS>100MPa). Cindering the economy and the safety in the underground engineering, the RMi system is not so appropriate to evaluate those hard rocks with the extreme integrity degree (very good case and very poor case).(4) In order to assure the accuracy of the rock mass classification in the condition of ultrahigh in-situ stress, the correlation coefficient K3 in the revised BQ system is suggested to increase to 2.0 for the Class I-II, Class V and 2.0-2.5 for the Class III-IV. Moreover, the applied conditions should be changed from 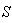 <4 to
<4 to 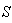 <5.
<5. Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML