1. Introduction

Indian Railways is one of the most popular and busiest modes of transportation in India and connects numerous cities, towns, states, districts, and regions. The capacity of a line is expressed in the number of trains in both directions that can be run on a given section of the line. Line capacity of a section represents the maximum number of trains can be passed over the section from 00:00 hrs to 24:00 hrs. The highest speed of freight trains varies from 60 km/hr to 75 km/hr depending on their axle load. Indian Railways' rolling stock consisted of 76,608 passenger coaches, 2, 93,077 freight wagons and 12,729 locomotives as per record of March 2020.

Based on literature review, it has been observed that 1. A less number of approaches are observed to measure network capacity of mix traffic 2. Impact of various parameters on railroad capacity in mix traffic is not sufficiently analysed. 3. No noticeable study has been carried out to manage the capacity of networks through improved operations in the circumstance of our country.

2. Literature Review

Xiaoming Xu et. al studied for balanced train time tabling with optimized velocity for single line. This model is used for non constant train velocity. [13]. Sameni, Melody Khadem, and Arash Moradi gave three categories of capacity assessment methods as analytical, optimization and simulation [9]. Ranolds et. al developed a statistical method to model traversal times in the variable-speed model [8]. By Zhang, Xin, and Lei Nie. an iterative approximation method proposed to reduce the computational time [16]. Chen, Angyang, et al. provided more transport services, it is necessary to add as many trains as possible in a section to increase capacity [2]. Yang Yuxiang, et al. suggested eleven types of delay events are classified, and a detailed analysis of delay distribution for each classification is presented [15]. The aim of researcher Yan, Fei, Nikola Besinovic, and Rob MP Goverde is to find an efficient, regular and robust timetable that utilizes the infrastructure capacity as good as possible [14]. Pouryousef, Hamed, Pasi Lautala, and David Watkins says that his model can provide a conflict free and compressed timetable out of initial schedule and works on single or multiple track corridors with directional or bi directional operations [6]. According to tang, Jia, et al. high speed rail service design should facilitate passenger engagement in various activities and improvement of their travel experience [10]. As per Pouryousef, Hammed, Pasi Lautala, and Thomas white, the capacity methods are commonly divided into analytical and simulation methods, but his paper also introduces a third, “combined simulation and analytical” category [7]. The method of computation of capacity in single-track railway lines is different from double or more track ones [1]. Pengling Wang et al. gave many different things including locomotive utilization, yard capacity, crew availability, track structure, and traffic levels all play a role and combine to determine railing efficiency [5]. Peng-Sheng You et.al developed heuristic algorithm and worked on train scheduling with parallel track [4]. T wan Dollevoet et. al. provided maximum allowable delay to measure the optimum result and developed a framework for optimizing the train scheduling [11]. Multi objective train scheduling model gave by Xiang Li et. al for focused on the lowering the fuel consumption cost [12]. Janusz Woch [18] presented the new way of methods for transportation network optimization who are based on the fact that the dependency of intersection capacity from traffic assignment. Proposed method is deep analyze the conditional capacities, that gives the good estimation of the network bottlenecks, and in the next steps, and the bottlenecks remove in order the most serious bottlenecks. This is proved that such method of network optimization is proper only.

According to author Mittal, Manoj, and Anand Kishore Chaturvedi that the analytical model are useful in planning phase and can evaluate the railway capacity of entire network of railway by efficient utilization of existing resources [17].

3. Definition of Railway Capacity

UIC consider only the schedule and but not the rolling stock, infrastructure, or the quality of service. However, both the rolling stock and the infrastructure are entirely included because they are important parameters for the timetable, while the quality is described by the stability, the number of trains, the average speed and the heterogeneity. In 2004 the International Union of Railways (UIC) re defined railway capacity ‘UIC 2004’. On the basis of available railway infrastructure and schedule, railway capacity can be measured. It is difficult to define because of several dependent parameters as like numerous of trains, average speed, heterogeneity and stability. Figure 1 shows that capacity is a balanced mix of the number of trains, consistency of the timetable, level of average speed and variety of the operation. It may be possible to meet market demand for a high average speed with higher variations as like a mix of fast Intercity Express, Intercity and slower Regional trains serving all stations. However, the result of having high average speed and high asymmetry is that it is not possible to operate multiple trains with a high stability. If there is market demand to operate more trains, it may be necessary to have fever mixed operations.

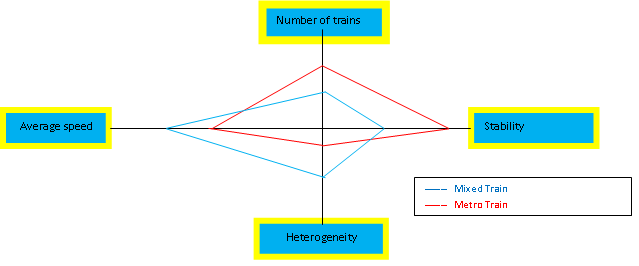Figure 1. Re defined railway capacity UIC 2004

3.1. Calculation of Line Capacity by Scott’s Formula

(1) Line capacity in theoretically calculated by the Scott’s formula which expresses the line capacity in terms of train per day in both directions.

Line capacity (C) = [A / (T+t)].Z

Where,

A = 1440 (Minutes per day)

T = running time of ruling train on the ruling section in the minutes

t = Time between the arrival and departure of two opposite or same direction train in a station in minutes

Z = Efficiency factor (The 70% factor is an efficiency factor, which accounts for the impossibility of regular distribution of train over the day, as it is not possible to run trains strictly one after the other, which is assumed while arriving at the theoretical line capacity.)

Ruling section = Governing section is the section between those two stations of the line with the longest running time of the ruling trains. The ruling trains normally are the slowest moving goods trains.

(2) To calculate the line capacity of a single line section (Line capacity) single line = C/2.

3.2. Railway Capacity and Train Speed

Railway capacity is always depends on the speed of the train. The availability of track (allocating time of track) is based on speed of trains. Track allocating is the length of track when train is moving on the track, it is known as blocking track and available free track is known as unblocking track. If train speed is higher than blocking time of section and track will be lower and vice verse. According to Indian railway rules AGC 03.07.2014, Maximum length of train 750 m and average length of coach 25m. Various speed pattern of trains are given in table 1. The train length should not exceed the loop line length 750 m. Hence passenger train can have maximum 24 coaches. It varies from 12 to 24 coaches based on the route and train type while wagons of goods train will be 11 to 15m in length (BOX, BOXN, BOXN-HL etc.)

Table 1. Pattern of Trains

Generally wagons in one rack can be from 40 to 58 maximum based on the length of the boxes and also goods trains should not exceed the loop line length 750m. The length of train may be categorized in three types 750m, 600m and 450m as per number of bogies with various speed of trains. The range of speed may be varying from 30km/hr to 150 km/hr with the difference of 15 km/h. The Length of block section assumed is 750m in our case study at the entry of the station. Hence crossing time has calculated in given table 2.

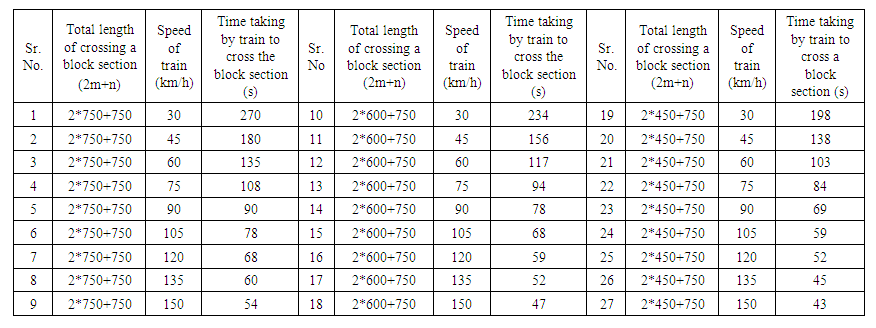Table 2. Travelling time of block section

4. Models for Analysis of Train Capacity for Variable Speed Trains

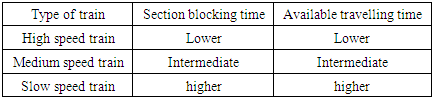
Four models is given below-

a. Higher speed train Vs blocking time of track: the track blocking time (t) is inversely proportional to speed (s) of train. If train has high speed then track blocking time will be low. When the train leaves the section completely, the blocking time is over and another train can enter to the same section after all relevant signalling equipment reset to their normal state. Hence, Blocking time of track (t) ∝ 1 / Speed of train (s) (particular section) Train travelling time (T) ∝ 1 / Speed of train (S) (from origin to destination)

b. Medium speed train Vs blocking time of track: Blocking time of track will lie between blocking time of slow speed train and higher speed train.

c. Slow speed train Vs blocking time of track: Blocking time of track will be more compare to higher and medium speed train.

d. Zero speed train Vs Blocking time of track: Blocking time of train will be infinite if train stayed on the track and not stayed any platform or crossing i.e. train is not in operating condition.

5. Case Study for Analysis of Rail Network Capacity

Analysis of railway network capacity has been done for Indian railway from Sawai Madhopur SWM) junction to RamganjMandi (RMA) Junction. Real data of passenger train has taken for this purpose in table 3.

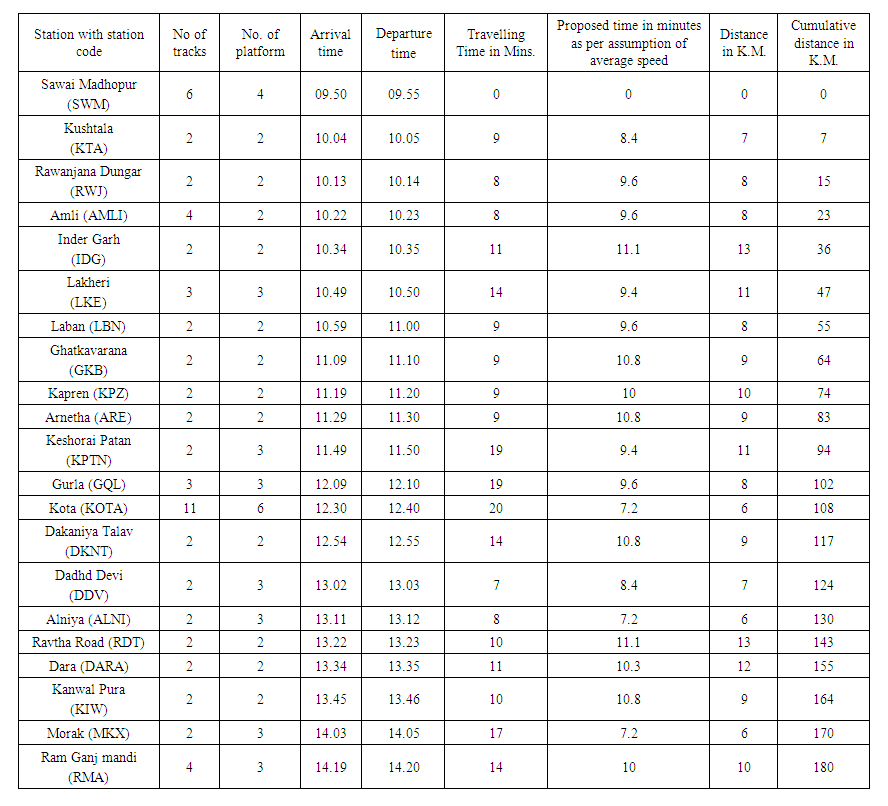Table 3. Existing schedule of passenger train from junction SWM to junction RMA (from open source)

5.1. Literature Gap

1. Analysis has been done in the past on the basis of whole route not done on the basis of intermediate stations between origin and destination.

2. In the previous study didn’t analysis the average speed between the intermediate stations and not compared to average speed of whole route.

3. In the past it was not observed that the fluctuation of actual average speed with compare to whole route average speed.

5.2. Research Objective

1. To find out the average running speed of whole route without considering the dwell time.

2. To find out actual average speed between intermediate stations.

3. To compare the route average speed with average speed of intermediate stations.

4. To find out the route of intermediate stations where actual average speed of intermediate station is lesser than average speed of whole route. This is known as bottleneck route.

5. To compare existing actual average speed & route average speed with proposed average speed.

6. For block section, time taken by the train to cross the block section (TB).

TB = (2m+n) / V

Where,

Length of train = m

Length of block section = n

6. Heuristic Algorithm

6.1. Assumption of Speed (Proposed)

Following assumption has taken in our study, all are given below (see table 3.):-

1. If D < 10 then V_P = 50 Km/h

2. If D = 10 then V_P = 60 Km/h

3. Neglect all obstacles during running and not Included the dwell time in our study.

4. If 10 < D ≤ 20 then V_P = 70 Km/h

5. If 20 < D then V_P =100 Km/h

Where, Average speed of whole route = V

Actual average speed of Intermediate stations = V_A

Proposed Average speed = V_P

Distance (In K.M.) = D

Proposed avg. speed of Intermediate stations = V_P

6.2. Analysis of Various Speeds of Train between Intermediate Stations and Whole Route (See Table 3)

1. For existing, If V_A < V Bottleneck, otherwise not.

2. For proposed, V< V_P and V_A < V_P

3. V_Ai = D_i / T_i

4. V = (1/n) ∑VA_i

5. V = (1/n) ∑D_i / ∑T_i (i =1…..n)

6. Index I = V_A / V_P ,

If I ≥ 1 feasible (F), otherwise Infeasible (I)

6.3. Marked Symbol is Given in Evaluation Table 4

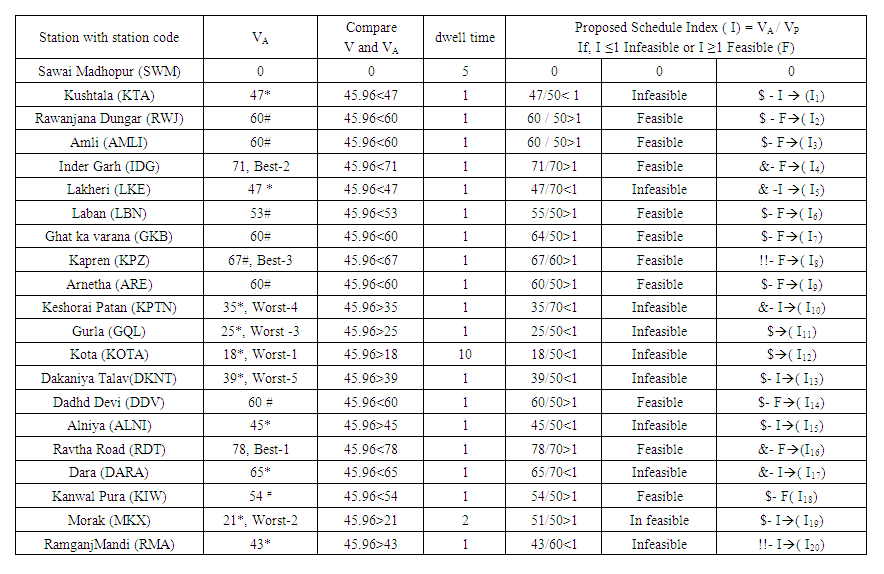Table 4. Analysis of existing and proposed schedule of passenger train from junction SWM to junction RMA

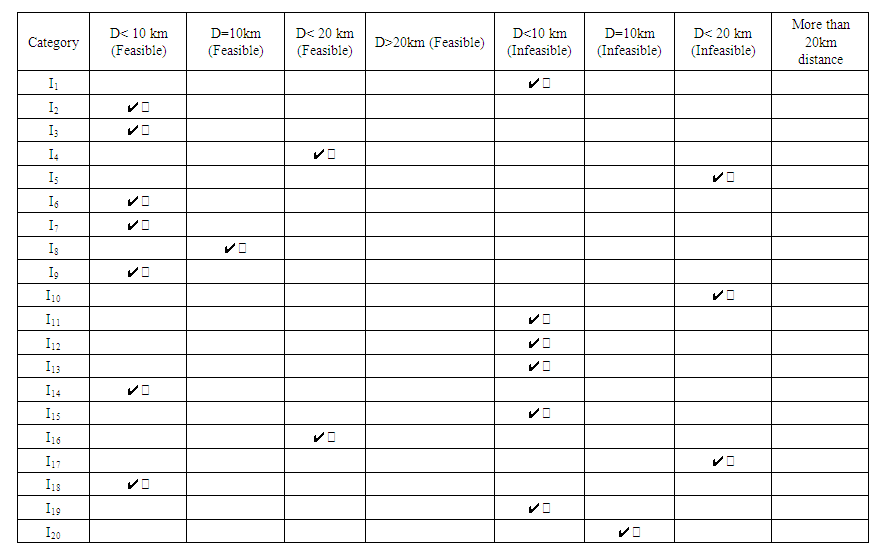Table 5. Analysis of existing schedule for slow speed train

6.4. Existing and Proposed Analysis is Given Below

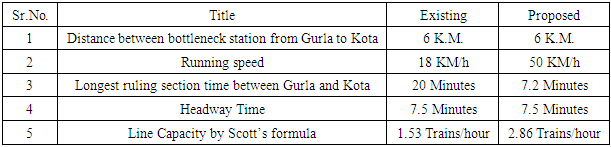
Most bottleneck route identified from Gurla to Kota. An outcome of existing and proposed analysis by Scott’s formula is given in table 6 and figure 2 & figure 3.

Table 6. Existing and proposed analysis

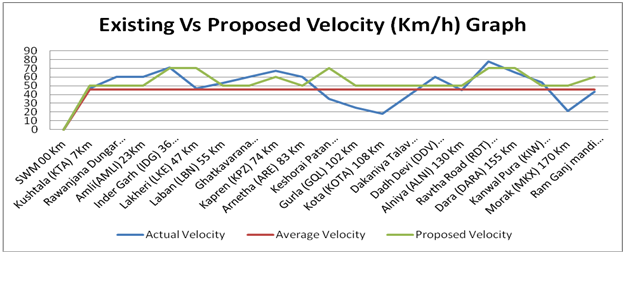Figure 2. Existing vs proposed velocity (Km/h)- Distance graph

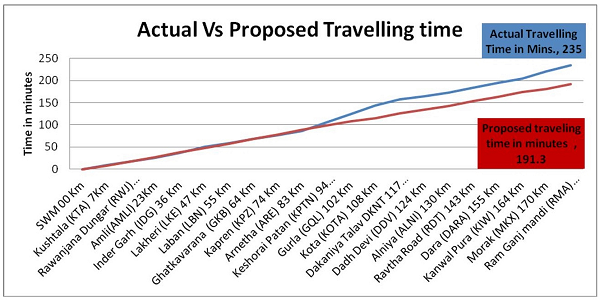Figure 3. Existing vs. proposed time (Minutes)- Distance (Km) graph

7. Result

The Analysis done in this paper shown in graph and the result obtained after comparing the proposed average speed of train with the existing whole route average speed & actual average speed of intermediate stations. Train running time on the route from SWM to RMN has reduced 43.7 minutes. Track availability will increase by 18.6% by reducing train running time. In India total 13169 passenger trains are running daily on all routes (as per Indian Railways records) in which 3572 slow trains are running daily. Hence the capacity of the railway network will increase by 18.6% for the 27.12% running the slow trains.

8. Conclusions

This paper examines a significant number of research papers and explains the idea of rail capacity and increasing railway network capacity for slow trains. It is observed that this study can eliminate the possibility of delay of train. It focuses on each section of railway track. In future, various uncertainties can be studied using various simulation models for complex route and prevention for train delay and accidents can be done.