-
Paper Information
- Paper Submission
-
Journal Information
- About This Journal
- Editorial Board
- Current Issue
- Archive
- Author Guidelines
- Contact Us
International Journal of Theoretical and Mathematical Physics
p-ISSN: 2167-6844 e-ISSN: 2167-6852
2021; 11(2): 67-70
doi:10.5923/j.ijtmp.20211102.02
Received: Mar. 19, 2021; Accepted: Apr. 7, 2021; Published: Apr. 15, 2021

Quantum Gate Based on Graphene Quantum Dot Modeling
Asmaa Mo. S.1, Ali M. Kh.2, Zynab A.1, Shimaa S. A.1
1Department of Physics, Al-Azhar University (Girls' Branch), Egypt
2Department of Physics, Al-Azhar University, Egypt
Correspondence to: Asmaa Mo. S., Department of Physics, Al-Azhar University (Girls' Branch), Egypt.
| Email: |  |
Copyright © 2021 The Author(s). Published by Scientific & Academic Publishing.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/

We propose here a model Hamiltonian of double quantum dots with the armchair boundary to produce a theoretical study of a quantum computer. Then we solve it using Dirac fermions equations. Solving the Hamiltonian model and investigate the exchange interactions between two electrons captured in the double dots. Then we investigate the effect of different parameters on exchange interaction. We have found the dependence of the exchange interaction for various potential barrier height and barrier thickness between double dots. Our result shows that; the change of exchange interaction under the effect of this parameter leads to studying coherence time for this model and get the smallest value of switching time. Changing of exchange interaction is accompanied by a transition of electrons between different states. This reality can examine this model as a quantum gate for quantum information.
Keywords: Quantum dot, Graphen, Qubit, Exchange interaction
Cite this paper: Asmaa Mo. S., Ali M. Kh., Zynab A., Shimaa S. A., Quantum Gate Based on Graphene Quantum Dot Modeling, International Journal of Theoretical and Mathematical Physics, Vol. 11 No. 2, 2021, pp. 67-70. doi: 10.5923/j.ijtmp.20211102.02.
1. Introduction
- A quantum dot based on graphene nanoribbon GNR [1,2] is the best candidate as a basic unit for quantum computers [3,4].This computer that works depends on quantum mechanics phenomena [5] such as superposition and entanglement [6], where the spin of electrons acts as a quantum bit (qubit) [7-10].Several types of research worked on how to model quantum computers depend on the spin of the electron and preserved the coherent time [11] of that electron compared to gate time to do its operation. Since the main sources of spin De-coherence are the spin of nucleus and orbit interaction [12-14], carbon material overcomes these difficulties [15], but the valley degeneracy [16] represents another problem. GNR introduces the ability to get a model of double quantum dot where the valley degeneracy is left and, coherence time of the electron's spin is long enough to transfer information, on the contrary; this property is lost in semiconductor material [17,18].Here we introduce a theoretical model of graphene nanoribbon (GNB) double quantum dot of armchair boundary condition each of them confined single electron acts as a spin qubit. We allowed them to a couple through exchange coupling. Using the Dirac equation and Heisenberg exchange model we can determine the exchange coupling, since studying quantum gate occur through studying exchange coupling. Finally, we examine the effect of a different variable on exchange coupling and calculate the switching time related to this model.
2. Theiortical Quantum Dot Model
- Consider a model of two neighboring quantum dots constructed on graphene nanoribbon (GNB) with armchair boundary conditions whose length L and width W fig. (1). separated them by a potential barrier with thickness d; each dot has dimensional length l along the y-axis. A single electron captured on each dot with an electric potential V (y) along y-direction [19], the two spin qubits are coupled between dots through barrier 2 (thickness d) via exchange coupling and the coupling controlled by barrier voltage that represented by low energy effective Hamiltonians:
 Where J (t) is the exchange coupling constant between the two spins S(1) and S(2).The left and right barriers should be controlled to control the Klein tunneling effect.
Where J (t) is the exchange coupling constant between the two spins S(1) and S(2).The left and right barriers should be controlled to control the Klein tunneling effect.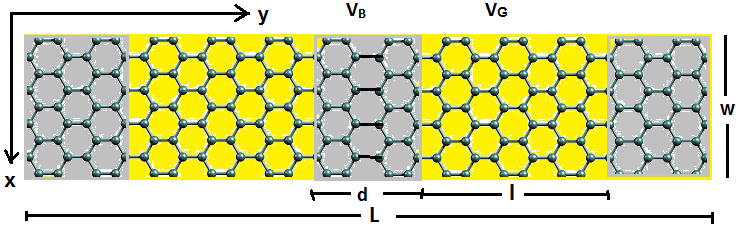 | Figure (1). Structured of double quantum dot of GNB with armchair edge separated by barrier with thickness d |
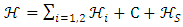 | (1) |
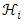 is the single-particle Hamiltonian for a Dirac particle can be defined by Dirac equation [20].
is the single-particle Hamiltonian for a Dirac particle can be defined by Dirac equation [20].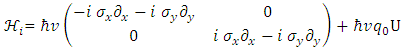 | (2) |
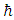 is Planck’s constant,
is Planck’s constant, 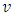 is the Fermi velocity of graphene, σx, σy are Pauli matrices for the pseudospin describing two sublattices of graphene A and B, ∂x and ∂y are partial derivatives is the charge of an electron,
is the Fermi velocity of graphene, σx, σy are Pauli matrices for the pseudospin describing two sublattices of graphene A and B, ∂x and ∂y are partial derivatives is the charge of an electron, 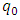 is the quantization ground state wave vector in x- direction which is
is the quantization ground state wave vector in x- direction which is 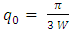 Where W is the quantum dot width, and U is the electrical confining energy in units of
Where W is the quantum dot width, and U is the electrical confining energy in units of 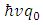 along the y-axis which is given by:
along the y-axis which is given by: 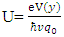 Where V(y) is confinement potential in y- directionC is the Coulomb interaction in two-dimension given by:
Where V(y) is confinement potential in y- directionC is the Coulomb interaction in two-dimension given by: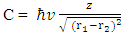 Where
Where 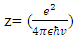 is a dimensionless Coulomb parameter, z =1.43 for graphene on quartz substrate [23].The four-component wave function describes this system given by:
is a dimensionless Coulomb parameter, z =1.43 for graphene on quartz substrate [23].The four-component wave function describes this system given by: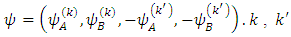 related to the two valleys in the Brillion zone of graphene, The solution of eq. (1) gives an electron wave as:
related to the two valleys in the Brillion zone of graphene, The solution of eq. (1) gives an electron wave as: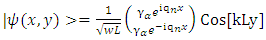 | (3) |
 refer to the two components of the pseudospin. The basis vectors for the two-component pseudo spinor are:
refer to the two components of the pseudospin. The basis vectors for the two-component pseudo spinor are: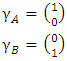 The total wave function of coupled electrons must be anti-symmetric can be written as the product of the spatial wave function and the corresponding two-particle spinor for the singlet (triplet) state.
The total wave function of coupled electrons must be anti-symmetric can be written as the product of the spatial wave function and the corresponding two-particle spinor for the singlet (triplet) state.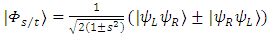 | (4) |
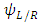 denotes a four-component single-particle wave function located at the left (right) quantum dot, and S
denotes a four-component single-particle wave function located at the left (right) quantum dot, and S 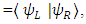 which is the overlap between them.Diagonalizable of S yield:
which is the overlap between them.Diagonalizable of S yield: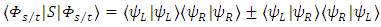 | (5) |
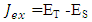 from the Schrodinger equation:
from the Schrodinger equation: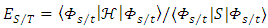 | (6) |
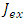 analytical and result:
analytical and result: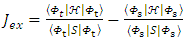 | (7) |
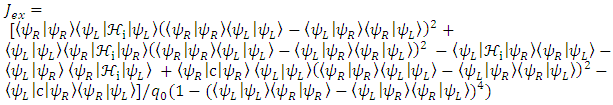 | (8) |
3. Results
- a) The effect of barrier height
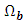 on exchange coupling JexWe represent the ribbon of width W= 0.4
on exchange coupling JexWe represent the ribbon of width W= 0.4 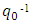 and its length L= 16
and its length L= 16 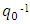 , the length of the dot is l= 4
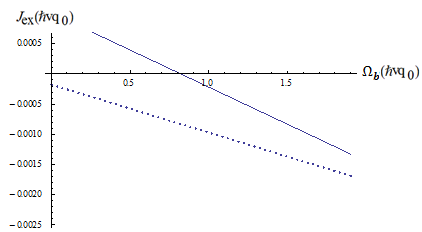
, the length of the dot is l= 4 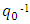 , where q0= 1/20 (nm)-1.We examine the change of exchange coupling at different barrier heights between two electrons in the double quantum dot. Using equation (8), we found that at small barrier height, for barrier thickness d=2 q0-1 fig. (2-dot line) The exchange coupling Jex starting as a positive value, reaches to maximum value
, where q0= 1/20 (nm)-1.We examine the change of exchange coupling at different barrier heights between two electrons in the double quantum dot. Using equation (8), we found that at small barrier height, for barrier thickness d=2 q0-1 fig. (2-dot line) The exchange coupling Jex starting as a positive value, reaches to maximum value 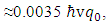 and as the barrier height increase, Jex decrease and change its sign to negative. For d=4 q0-1 fig. (2-solid line) The exchange coupling is always negative for small and large barrier height. For small barrier height and small barrier thickness, the system behaves like a single quantum dot and coupling between electrons in a strong favored singlet state with positive Jex. As the potential increase or as large barrier thickness d, the double quantum dot becomes dominant and we see negative Jex according to transition from singlet to triplet, The transition from antiferromagnetic (J > 0) to ferromagnetic (J < 0), at
and as the barrier height increase, Jex decrease and change its sign to negative. For d=4 q0-1 fig. (2-solid line) The exchange coupling is always negative for small and large barrier height. For small barrier height and small barrier thickness, the system behaves like a single quantum dot and coupling between electrons in a strong favored singlet state with positive Jex. As the potential increase or as large barrier thickness d, the double quantum dot becomes dominant and we see negative Jex according to transition from singlet to triplet, The transition from antiferromagnetic (J > 0) to ferromagnetic (J < 0), at 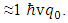 The value of Jex for short and long d is nearly close to each other as the barrier height reaches the value GNB ≈ 2
The value of Jex for short and long d is nearly close to each other as the barrier height reaches the value GNB ≈ 2 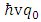 . this phenomenon is a special character for GNB whereas the barrier is high the Klien tunneling occurs. The same result is obtained if we change the length of dots l=2 q0-1 at barrier thickness d=1.2 q0-1 and d=2 q0-1 fig. (3).
. this phenomenon is a special character for GNB whereas the barrier is high the Klien tunneling occurs. The same result is obtained if we change the length of dots l=2 q0-1 at barrier thickness d=1.2 q0-1 and d=2 q0-1 fig. (3).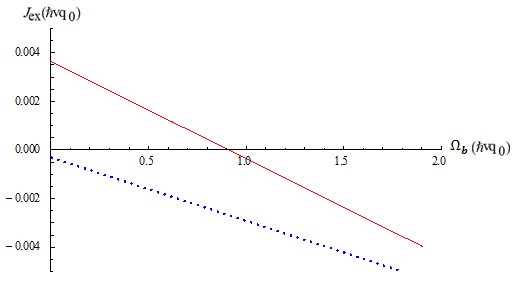 | Figure (2). The exchange coupling 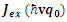 as a function of barrier height as a function of barrier height 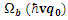 at l= 4 q0-1, q0=1/20 (nm)-1 and d=2 q0-1 (solid line), d=4 q0-1 (dot line) at l= 4 q0-1, q0=1/20 (nm)-1 and d=2 q0-1 (solid line), d=4 q0-1 (dot line) |
 | Figure (3). The exchange coupling 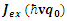 as a function of barrier height as a function of barrier height 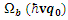 at l= 2 q0-1, q0=1/20 (nm)-1 and d=1.2 q0-1 (solid line), d=2 q0-1 (dot line) at l= 2 q0-1, q0=1/20 (nm)-1 and d=1.2 q0-1 (solid line), d=2 q0-1 (dot line) |
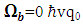 at l=4q0-1 fig. (4-blue dot line) The exchange coupling Jex started positively at about 0.0002
at l=4q0-1 fig. (4-blue dot line) The exchange coupling Jex started positively at about 0.0002 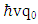 then decrease and change its sign into negative at d ≈ 4q0-1 corresponding to a transition from singlet to the triplet state. For small distance d, the system considers as a single dot and two electrons arranged in the singlet state. As the inter-distance increase, the double dot system dominant and triplet state exists. For barrier height greater than
then decrease and change its sign into negative at d ≈ 4q0-1 corresponding to a transition from singlet to the triplet state. For small distance d, the system considers as a single dot and two electrons arranged in the singlet state. As the inter-distance increase, the double dot system dominant and triplet state exists. For barrier height greater than 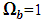 (critical value) fig. (4) (red and green); the exchange interaction always negative, and the value of Jex increase first as d increase until reaching the critical value of d; about 4 q0-1; then start to decrease again. The value of Jex for
(critical value) fig. (4) (red and green); the exchange interaction always negative, and the value of Jex increase first as d increase until reaching the critical value of d; about 4 q0-1; then start to decrease again. The value of Jex for 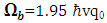 is greater in the negative direction than that for
is greater in the negative direction than that for 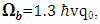 for triplet state, this may be related to Klein tunneling properties in graphene.
for triplet state, this may be related to Klein tunneling properties in graphene.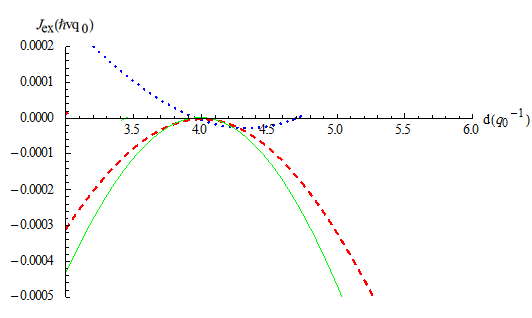 | Figure (4). The exchange coupling 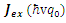 as a function of inter- dot distance d (q0-1) at l= 4 q0-1, q0=1/20 (nm)-1 for as a function of inter- dot distance d (q0-1) at l= 4 q0-1, q0=1/20 (nm)-1 for 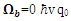 (blue), (blue), 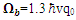 (red), and for (red), and for 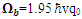 (green) (green) |
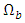 with d= 5q0-1. For barrier height greater than the critical value, the figure represents a negative value of Jex according to triplet state and Jex increase exponential as the dot length increase but the minimum value of Jex is greater for
with d= 5q0-1. For barrier height greater than the critical value, the figure represents a negative value of Jex according to triplet state and Jex increase exponential as the dot length increase but the minimum value of Jex is greater for 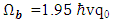 than that for
than that for 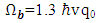 where overlap between wave functions of electrons increases by Klein tunneling of Dirac particle this gives a special character for a qubit in GNB than qubit in other systems [21].
where overlap between wave functions of electrons increases by Klein tunneling of Dirac particle this gives a special character for a qubit in GNB than qubit in other systems [21].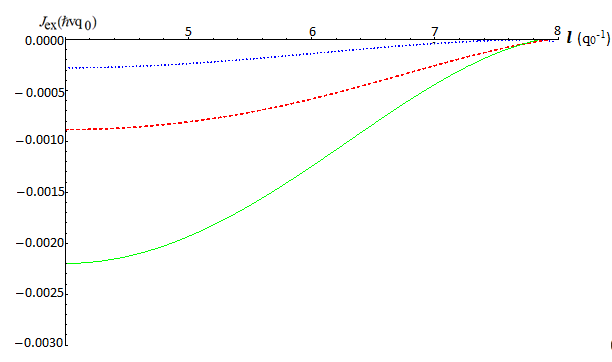 | Figure (5). The exchange coupling 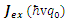 as a function of confining length l (q0-1) at d= 5 q0-1, q0=1/20 (nm)-1 for as a function of confining length l (q0-1) at d= 5 q0-1, q0=1/20 (nm)-1 for 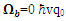 (blue), (blue), 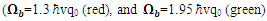 |
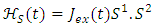 The exchange interaction allows representing the unitary time evolution between the 1-th and 2-th spins with the operator:
The exchange interaction allows representing the unitary time evolution between the 1-th and 2-th spins with the operator: 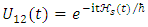 , corresponding to the "swap" operator. The combination of this gate with one qubit rotation gate obtain a universal quantum gate UXOR which can use for programming any desired quantum computation. Thus the calculation of quantum XOR can be reduced by calculation of exchange coupling Jex(t) and how can be controlled experimentally using external parameter [22].
, corresponding to the "swap" operator. The combination of this gate with one qubit rotation gate obtain a universal quantum gate UXOR which can use for programming any desired quantum computation. Thus the calculation of quantum XOR can be reduced by calculation of exchange coupling Jex(t) and how can be controlled experimentally using external parameter [22].4. Conclusions
- As we Show; we have introduced a theoretical model of the double qubits on the GNB quantum dot, we solve the Dirac equation for the model and get the exchange interaction Jex between two qubits. We have examined the value of Jex at different parameters such as barrier height between double quantum dot, barrier thickness, and dot width to study the effect of each one on Jex. The results represent a transition of exchange interaction from singlet to triplet state by increasing barrier height between double quantum dots as in fig. (2) and (3). This transition can also get under the effect of barrier thickness figure (4-blue dot line). This transition we can use to transfer information in quantum computers. The transfer of information is an essential step for computation. Also, we get the maximum value of Jex≈
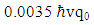 ≈ 0.11515 meV which is greater than that of GaAs [23,24] (in GaAs is around 0.1 mV) and exceed the value of Jex in other models. The corresponding value of switching time is about
≈ 0.11515 meV which is greater than that of GaAs [23,24] (in GaAs is around 0.1 mV) and exceed the value of Jex in other models. The corresponding value of switching time is about 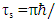 Jex [6] ≈.018ns. The validity of the quantum gate comes from the long coherence time to switching time. Since the coherence time for graphene is greater than that for GaAs [22,25] and we get the smallest value of τs, so we found that the graphene qubit is better than that of GaAs. This factor is a fundamental condition for working a spin as a qubit.
Jex [6] ≈.018ns. The validity of the quantum gate comes from the long coherence time to switching time. Since the coherence time for graphene is greater than that for GaAs [22,25] and we get the smallest value of τs, so we found that the graphene qubit is better than that of GaAs. This factor is a fundamental condition for working a spin as a qubit. Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML