-
Paper Information
- Paper Submission
-
Journal Information
- About This Journal
- Editorial Board
- Current Issue
- Archive
- Author Guidelines
- Contact Us
International Journal of Materials Engineering
p-ISSN: 2166-5389 e-ISSN: 2166-5400
2018; 8(6): 162-166
doi:10.5923/j.ijme.20180806.05

Comparison of Two Methods to Determine the Bending Modulus of Elasticity of Wood
Lucas José Marini1, André Luís Christoforo2, Francisco Antonio Rocco Lahr3
1Department of Materials Engineering (SMM), São Carlos School of Engineering, University of São Paulo (USP), São Carlos, SP, Brazil
2Centre for Innovation and Technology in Composites (CITeC), Department of Civil Engineering (DECiv), Federal University of São Carlos (UFSCar), São Carlos, Brazil
3Department Structural Engineering (SET), São Carlos School of Engineering, University of São Paulo (USP), São Carlos, SP, Brazil
Correspondence to: Lucas José Marini, Department of Materials Engineering (SMM), São Carlos School of Engineering, University of São Paulo (USP), São Carlos, SP, Brazil.
| Email: |  |
Copyright © 2018 The Author(s). Published by Scientific & Academic Publishing.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/

The modulus of elasticity is among the main properties required for the dimensioning of a wood structure. It can be calculated using different test methods, the most commonly used are the 3 and 4 points bending tests. The Brazilian standard, ABNT NBR 7190: 1997, recommends the 3-point static bending test. Studies about the influence of testing methods to determine the bending modulus of elasticity are always relevant, since can improve its reliability. Thus, the aim of this study is to examine the bending modulus of elasticity of samples of nine Brazilian wood species: Cambará Rosa (Erisma uncinatum Warm), Caxeta (Tabebuia cassinoides), Tatajuba (Bagassa guianensis), Itaúba (Mezilaurus itauba), Branquilho (Termilalia spp), Cedro Amazonense (Cedrelinga catenaeformis Ducke), Roxinho (Peltogyne spp.), Cumaru (Dipteryx odorata) and Cambará (Gochnatia polymorpha). The results of the confidence intervals have showed that the moduli of elasticity for both test methods were equivalent at 5% significance level.
Keywords: Tropical species, Bending test, 3 and 4 points, Characterization, Analysis of variance (ANOVA)
Cite this paper: Lucas José Marini, André Luís Christoforo, Francisco Antonio Rocco Lahr, Comparison of Two Methods to Determine the Bending Modulus of Elasticity of Wood, International Journal of Materials Engineering , Vol. 8 No. 6, 2018, pp. 162-166. doi: 10.5923/j.ijme.20180806.05.
1. Introduction
- Wood is a natural and renewable resource, used in several sectors, mainly in the construction sector as structural and finishing elements [1-4]. It is a heterogeneous material, constituted by various types of cells, with varied chemical, physical and mechanical properties [5]. In addition, wood presents good resistance/weight ratio, when compared to other materials such as steel and concrete [6].For the rational application of this material it is important to know its physical and mechanical properties [7, 8]. In Brazil, ABNT NBR 7190: 1997 (Design of Timber Structures) [9] provides guidelines for the design of structures and for the characterization of wood. In Appendix B: "Determination of wood properties for structural design", the standard recommends the 3-point static bending test, which is restricted to small and defect-free specimens.Several data banks on wooden properties of different species contain mechanical characteristics of which the bending modulus of elasticity, compression, tensile, among other properties. The bending modulus of elasticity can be obtained by different test method, the more commonly used are the 3 and 4 points bending tests [10, 11].In [12] it was analysed the influence of testing methods (three and four points bending) and the moisture content to determine the bending modulus of elasticity, the limit of proportionality (LOP) and the elastic potential (PE). Results showed that the difference in the modulus of elasticity values measured in three and four points bending is statistically significantly affect by the moisture content, while the LOP and PE was not affected. It was performed a study [13] to verify the influence of the length of wood specimens on the modulus of elasticity obtained in compression parallel to the grain (Ec0). It were adopted 1:3, 1:4 and 1:5 ratios between the side of the cross section (50mm) and the length of the specimens (150, 200 and 250 mm). The Ec0 values are statistically equivalent to the 5% statistical significance level, showing that the changing the lengths of the specimens did not affect this property. In addition another study was conducted to determine the influence of testing methods on the modulus of elasticity of the wood [11]. Based on ANBT NBR 7190:1997 and international standards, it was examined the bending modulus of elasticity of three wood species Canelão (Nectranda membranacea), Corymbia (Corymbia citriodora) and Angelim (Angelim sp). The results for Canelão and Angelim showed statistical equivalence between the methods at 5% significance level. However, for Corymbia species, statistical differences of results for bending tests with three and four points were observed. Although the current Brazilian standards not addressed the four-point static bending tests, there are a number of papers that have evaluated it. In France [10] it was performed a study to make an analytic formula of a crossing coefficient between three and four point bending modulus of elasticity following the French standards. It has verified that the three points bending tests underestimated about 19% the values of modulus of elasticity relative to the four points test. However the relative difference between these two bending tests was not continuous following the wood density. Other relevant study [14] have examined two methods of calculation to obtain the bending modulus of elasticity in wooden specimens of Paricá (Shizolobium amazonicum) with some defects created: a simplified, adapted from ABNT NBR 7190: 1997, and another based on the least square method. Results of the confidence intervals showed that de modulus of elasticity for both ways of calculation were equivalent for non-defective specimens. For the defective pieces the alternative methodology for the modulus of elasticity presented significantly different results from those of Brazilian standard adaptation.In this context, this study aims to analyze the influence of different bending methods (3 and 4 load points) in the determination of longitudinal modulus of elasticity in specimens of nine Brazilian wood species.
2. Material and Methods
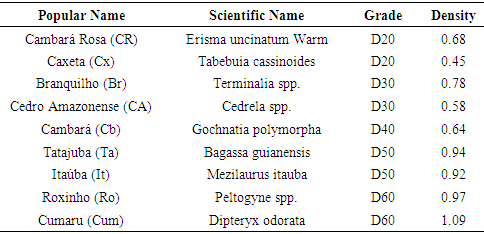
- This study was conducted at the Wood and Timber Structures Laboratory (LaMEM), Department of Structural Engineering (SET), São Carlos School of Engineering (EESC), University of São Paulo (USP). The analysis of variance (ANOVA) was used to investigate the influence of the testing methods (static bending at 3 and 4 points) in the determination of the modulus of elasticity considering wood species of Cambará Rosa (CR), Caxeta (Cx), Tatajuba (Ta), Itaúba (It), Branquilho (Br), Cedro Amazonense (CA), Roxinho (Ro), Cumaru (Cum) and Cambará (Cb). It should be noted that the species of wood selected cover all strength grading (grade) of the hardwood of the Brazilian standard ABNT NBR 7190 (1997) (Table 1), which contributes to a greater comprehension of the results obtained in the present research.
|
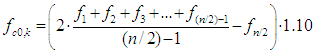 | (1) |
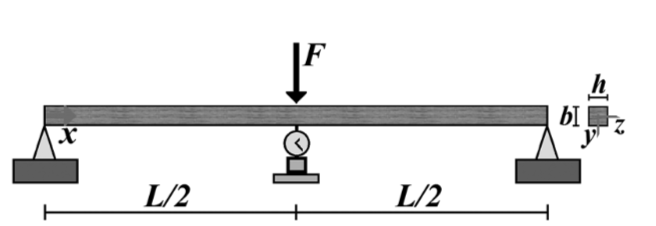 | Figure 1. Three point bending tests model |
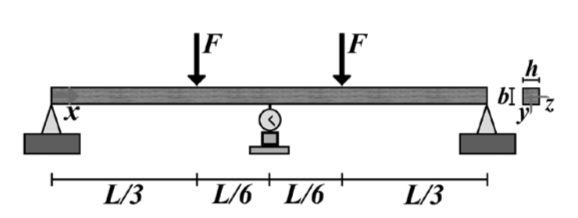 | Figure 2. Four point bending tests model |
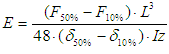 | (2) |
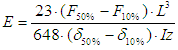 | (3) |
3. Results and Discussion
- Figures 3 and 4 show the mean values, the confidence intervals (95% confidence) and the variation coefficients (CV) of the modulus of elasticity obtained by both test methods and for each species of wood evaluated.
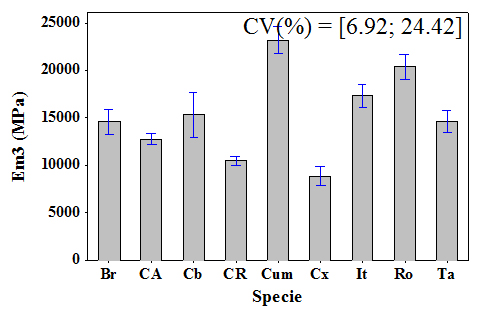 | Figure 3. Elasticity modulus obtained from three points bending test |
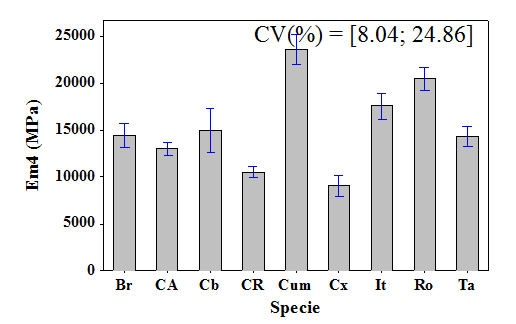 | Figure 4. Elasticity modulus obtained from four points bending test |
|
4. Conclusions
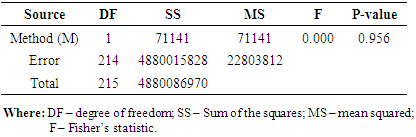
- The aim of this paper was to determine the influence of testing methods to determine the bending modulus of elasticity of nine Brazilian woods species. These species cover all strength grade of the hardwood of the Brazilian standard ABNT NBR 7190 [9]. According to the procedures adopted, it is possible to infer the following conclusions:• For static bending tests with 3 and 4 points, results of E were statistically equivalent for all the nine species studied (P-value = 0.956); • It is suggested to study other species of wood and consider other moisture content values.
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML