André Luis Christoforo1, Tiago Hendrigo de Almeida2, Diego Henrique de Almeida3, Júlio César dos Santos4, Tulio Hallak Panzera4, Francisco Antonio Rocco Lahr5
1Centre for Innovation and Technology in Composites – CITeC, Department of Civil Engineering (DECiv), Federal University of São Carlos, São Carlos, Brazil
2Department of Materials (SMM), São Paulo University (EESC/USP), São Carlos, Brazil
3Pitágoras Faculty, Department of Engineering, Poços de Caldas Campus, Poços de Caldas, Brazil
4Centre for Innovation and Technology in Composites (CITeC), Department of Mechanical Engineering, Federal University of São João del-Rei, São João del-Rei, Brazil
5Department of Structural Engineering (SET), São Paulo University (EESC/USP), São Carlos, Brazil
Correspondence to: André Luis Christoforo, Centre for Innovation and Technology in Composites – CITeC, Department of Civil Engineering (DECiv), Federal University of São Carlos, São Carlos, Brazil.
| Email: |  |
Copyright © 2016 Scientific & Academic Publishing. All Rights Reserved.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/

Abstract
The knowledge about anisotropy of wood possibility more security design of structures and structural members designed. Therefore, there are little quantity of results knowledge about shrinkage and anisotropy coefficients of Wood species, motivating the development of new researches. The aim of research is evaluate the possibility of estimate the full shrinkage (radial; tangential; volumetric), anisotropy coefficient and shrinkage coefficient (radial; tangential; volumetric), using polynomial regression models (linear; quadratic; cubic) and exponential, by density of five Wood species (Cedro Doce [C20]; Canafístula [C30]; Angelim Araroba [C40]; Mandioqueira [C50]; Angelim Vermelho [C60]), properly classifieds in five strength classes for dicotyledonous according Brazilian Standard ABNT NBR 7190:1997. The better results were from tangential and volumetric retractions both using exponentials functions, justified by lower values of variation coefficients, with coefficients values of determination adjusted ranging from 51 and 54%. Even considered significant to the regressions for the tangential and volumetric shrinkage by analysis of variance, but with adjusted coefficients of determination lower than 70%, news researches should realized with other Wood species, enabling find general expressions for estimating such properties using the knowledge of the density.
Keywords:
Wood, Density, Anisotropy coefficient, Strength classes
Cite this paper: André Luis Christoforo, Tiago Hendrigo de Almeida, Diego Henrique de Almeida, Júlio César dos Santos, Tulio Hallak Panzera, Francisco Antonio Rocco Lahr, Shrinkage for Some Wood Species Estimated by Density, International Journal of Materials Engineering , Vol. 6 No. 2, 2016, pp. 23-27. doi: 10.5923/j.ijme.20160602.01.
1. Introduction
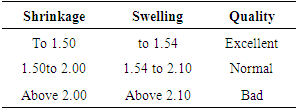
Wood is a material with great variability in their mechanical and physical properties, being the study and knowledge of these properties essential to its best processing [1, 2], providing rational use, whether in industry or in civil construction [3, 4].Studies with Paricá Wood specie (Schizolobiumamazonicum Herb.) and Cumaru Wood specie (Dipterixodorata) present different values for mechanical and physical properties, classifying it according Brazilian standard ABNT NBR 7190:1997 [5] at belonging to strength classes C20 and C60 of the dicotyledonous, respectively [6-8].Researches with different positions, sizes and grain directions of the specimens were realizes to determine more information to mechanical and physical properties of the wood [9-12].The density of wood is one of the main physical properties of wood, because with it is possible to estimate their mechanical properties [13, 14]. Classifying the Wood species according to their densities is also possible to plan programs for drying wood to minimize the problems of this process [15].The anisotropy property of the wood is very important to studies of their characteristics and is related with their moisture content. The dimensions of the wood undergo variations according to the increase or decrease in moisture content. Below the fibers saturation point can determine the swelling and shrinkage of the wood only increasing or decreasing moisture content, respectively [14, 17]. The Brazilian standard ABNT BR 7190:1997 [5] recommended 25% for moisture content of reference for fiber saturation point.The anisotropy coefficient (ratio between shrinkage or swelling in tangential and radial directions, respectively), is an important parameter that indicates the best application of wood specie relating it to their drying quality (Table 1).Table 1. Anisotropy coefficient and wood quality [18]
 |
| |
|
The aim of this research is to get more information about wood anisotropy, using polynomial (linear; quadratic; cubic) and exponential regression models, estimate the full shrinkage, anisotropy coefficient and shrinkage coefficient by density of five Wood specie, classifieds in five strength classes for dicotyledonous according Brazilian standard ABNT NBR 7190:1997 [5].
2. Material and Methods
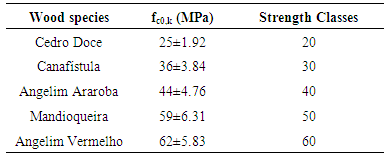
The research was carried outat Wood and Timber Structures Laboratory (LaMEM), São Carlos School of Engineering (EESC), University of SãoPaulo (USP), Brazil.The density (ρ12%), full shrinkage (radial [RS]; tangential [TS]; volumetric [VS]), inverse anisotropy coefficient (1/AC), anisotropy coefficient (AC) and shrinkage coefficient (radial [RSC]; tangential [TSC]; volumetric [VSC]) of Wood species were obtained according ABNT NBR 7190:1997 standard, using moisture content at the fiber saturation point (PS) for shrinkage coefficients calculation.The Wood species used in this research were Cedro Doce (Cedrela sp.), Canafístula (Cassia ferrugínea), Angelim Araroba (Vataireopsisararoba), Mandioqueira (Qualeasp.) e Angelim Vermelho (Diniziaexcelsa). Were used 12 specimens for Wood species to obtain the above cited properties and plus 12 for testing of compression parallel to the grain to obtain the characteristic strength value (fc0,k), responsible for defining the strength classes (Table 2).Table 2. Strength classes of Wood species used according ABNT NBR 7190:1997 Standard
 |
| |
|
The regression models for fullshrinkages, anisotropy coefficients and shrinkage coefficients at function of density were exponential (Equation 1) and linear (Equation 2), quadratic (Equation 3) and cubic (Equation 4) polynomial. The aim of regression models find the values for coefficients of functions [a, b, c and d] presented in Equations 1, 2, 3 and 4 according methods of least square fundaments (lower residue). The adjusts were realized for each property and according obtained values by specimens for five Wood species, totaling 60 specimens for each answer.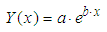 | (1) |
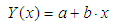 | (2) |
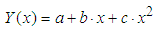 | (3) |
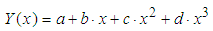 | (4) |
The significance and quality of stipulated adjusts were evaluated by variance analysis (ANOVA) of regression, with level of significance of 5% (α), having as null hypothesis (H0) the no-significance of regression by adjusted coefficients and significance of regression as alternative hypothesis (H1). P-value less the level of significance implies reject the null hypothesis, otherwise accepted the answer.
3. Results and Discussion
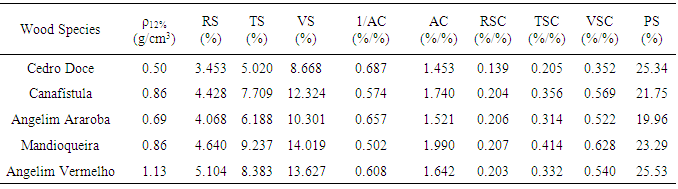
Table 3 present the results of mean values for each property for five wood species studied in this research.Table 3. Mean values of wood species properties
 |
| |
|
Cedro Doce Wood specie had the lowest density, shrinkage volumetric and radial, tangential and volumetric shrinkage coefficient. This result is consistent, therefore, Wood species with more density has more dimensional variation, because it has more wood per unit volume [19, 20].According Tables 1 and 3, Cedro Doce Wood specie present excellent quality, because present shrinkage anisotropy coefficient less than 1.5 can be used in furnitures, miters, sports equipment and other uses. The other Wood species studied are classified as normal quality, because present shrinkage anisotropy coefficient greater than 1.5 and less than 2.0, can be used in shelves, tables and cupboard [17, 18]. The shrinkage anisotropy coefficient found to Mandioqueira Wood specie (1.990) is equal the value determined to Cedro Marinheiro Wood specie (Guareatrichilioides L. (Meliaceae)), satisfactory result, because the two species belong to the C50 dicotyledonous strength classes [17].The mean value of volumetric shrinkage for Bracatinga Wood Specie (Mimosa scabrella Benthan) is 7.65%, less than the values found for the woods studied in this research [21].The Eucalypt Wood specie (Eucalyptus benthamii Matenet Cambage) have radial shrinkage, tangential shrinkage and anisotropy coefficient equal to 5.91%, 13.87% and 2.36, respectively [22]. The anisotropy coefficients of the Wood species used in this research are betters, with greater opportunities for more refined uses. The mean value fiber saturation point found to all Wood species were equal to 23.17%, very close to the 25% recommended by Brazilian standard ABNT NBR 7190:1997 [5].Table 4 to 10 presents the results of ANOVA regression models for seven studied properties. The label NS is no-significance of adjusted coefficient in the model (P-value > 0.05).Table 4. Results of regression models for radial shrinkage (RS)
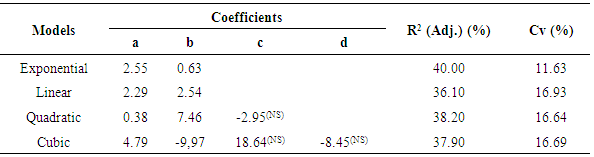 |
| |
|
Table 5. Results of regression models for tangential shrinkage (TS)
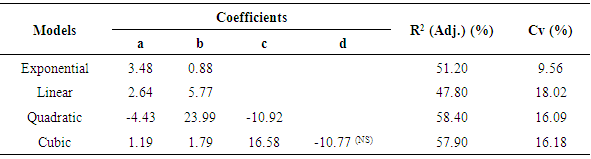 |
| |
|
Table 6. Results of regression models for volumetric shrinkage (VS)
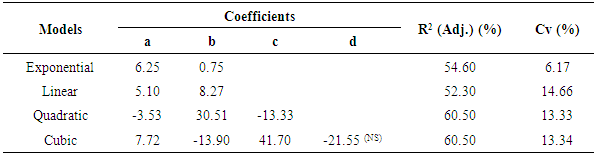 |
| |
|
Table 7. Results of regression models for inverse anisotropy coefficient (1/AC)
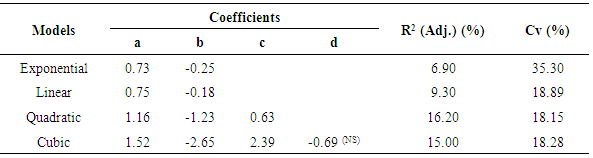 |
| |
|
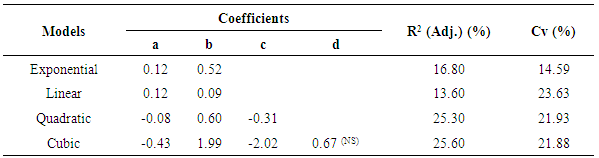
Table 8. Results of regression models for radial shrinkage coefficient (RSC)
 |
| |
|
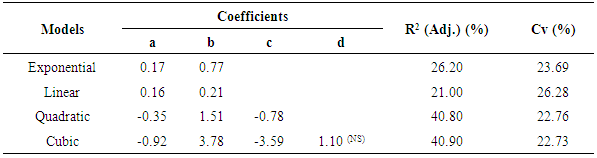
Table 9. Results of regression models for tangential shrinkage coefficient (TSC)
 |
| |
|
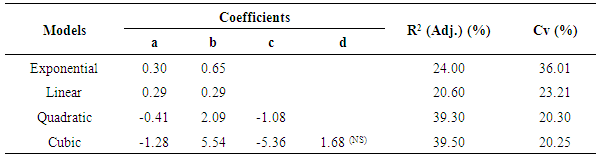
Table 10. Results of regression models for volumetric shrinkage coefficient (VSC)
 |
| |
|
The results of determination coefficients adjusted R²(Adj.) showed the possibility of estimative of tangential and volumetric shrinkages by density.For tangential and volumetric shrinkages, of linear adjusts for quadratics observed increases in R²(Adj.) value and reduction in variation coefficient of regression, and with cubic polynomial, ANOVA present no-significance in the results, equal occur for others properties. However, the variation coefficient of adjusts using exponential functions presents lower results of R²(Adj.) compared to variation coefficient of linear and quadratic polynomial functions, but presents variation coefficients well below derived of polynomials, showing be the exponentials models the betters among the evaluated, for the greater representation.For exponential models, the determination coefficients adjusted were below to 70% according with Montgomery [23], the representation of adjusted model present R²(Adj.) upper to 70%. The results indicate the feasibility of estimate of tangential and volumetric shrinkages by density, however, need more researches with other Wood species.
4. Conclusions
The Cedro Doce Wood specie presented excellent quality in relation to anisotropy coefficient and others Wood species studies were classifieds at normal quality.The results of determination coefficients adjusted R²(Adj.) showed the possibility of estimative of tangential and volumetric shrinkages by density.Even considered significant to the regressions for the tangential and volumetric shrinkage by analysis of variance, but with adjusted coefficients of determination lower than 70%, news researches should realized with other Wood species, enabling find general expressions for estimating such properties using the knowledge of the density.
References
| [1] | Gonçalves, M. T. T. Processamento da madeira. 1 ed. Bauru: Unesp/Bauru, 2000. |
| [2] | Teixeira, C. R.; Casanova, C. A. M.; Ferreira Filho, D.; Fernandes, J. A.; Balzaretti, N. M. Influência da anisotropia da madeira no desgaste das ferramentas diamantadas. In: VICOBEF – Congresso Brasileiro de Engenharia de Fabricação, Brasil, Associação Brasileira de Engenharia e Ciências Mecânicas, 2011. |
| [3] | Calil Junior, C.; Lahr, F. A. R.; Dias, A. A. Dimensionamento de elementos estruturais de madeira. 1 ed. Barueri: Manole Ltda, 2003. |
| [4] | Calil Junior, C.; Molina, J. C.Coberturas em estruturas de madeira: exemplos de cálculo. 1 ed. Pini: São Paulo, 2010. |
| [5] | Associação Brasileira de Normas Técnicas. ABNT NBR 7190: Projeto de estruturas de madeira. Rio de Janeiro: ABNT, 1997 |
| [6] | Almeida, D. H.; Scaliante, R. M.; Macedo, L. B.; Macêdo, A. N.; Dias, A. A.; Christoforo, A. L.; Calil Junior, C. Caracterização completa da madeira da espécie amazônica Paricá (Schizolobium amazonicum HERB) em peças de dimensões estruturais. Revista Árvore, v.37, p.1175-1181, 2013. |
| [7] | Almeida, D. H.; Cavalheiro, R. S.; Scaliante, R. M.; Christoforo A. L.; Calil Junior, C.; Lahr, F. A. R.Full characterization of strength properties of Schizolobiumamazonicum Wood for timber structures. International Journal of Engineering & Technology, v.37, p.97-100, 2013. |
| [8] | Almeida, D. H.; Chahud, E.;Ferro, F. S.; Poleto, S. F. S.; Almeida, T. H.; Christoforo, A. L.; Lahr, F. A. R. Physical and mechanical properties of Dipterixodorata (Aublet) Willd. Advanced Materials Research, v.1025, p.46-49, 2014. |
| [9] | Ferro, F. S.; Icimoto, F. H.; Almeida, D. H.; Christoforo, A. L.; Lahr, F. A. R. Verifications of test conditions to determine the compression modulus of elasticity of wood. International Journal of Agriculture and Forestry, v.3, p.66-70, 2013. |
| [10] | Icimoto, F. H.; Ferro, F. S.; Almeida, D. H.; Christoforo, A. L.; Lahr, F. A. R. Influence of the wood specimen position on calculus of the bending modulus of elasticity. International Journal of Materials Engineering, v.3, p.41-46, 2013. |
| [11] | Bertolini, M. S.; Silva, D. A. L.; Souza, A. M.; Calil Neto, C.; Lahr, F. A. R. Influência do comprimento de corpos-de-prova na obtenção do módulo de elasticidade pela compressão paralela às fibras. In: XIII EBRAMEM – Encontro Brasileiro em Madeiras e em Estruturas de Madeira, Brasil, Instituto Brasileiro de Madeiras e de Estruturas de Madeira, 2012. |
| [12] | Almeida, D. H.; Almeida, T. H.; Molina, J. C.; Ferro, F.S.; Christoforo, A. L.; Lahr, F. A. R. Embedment strength of Pinus sp. wood to metal pins. Advanced Materials Research, v.884, p.653-656, 2014. |
| [13] | Almeida, D. H.; Scaliante, R. M.; Christoforo, A. L.; Varanda, L. D.; Lahr, F. A. R.; Dias, A. A.; Calil Junior, C. Tenacidade da madeira como função da densidade aparente. Revista Árvore, v.38, p.203-207, 2014. |
| [14] | Logsdon, N. B.; Calil Junior, C. Influência da umidade nas propriedades de resistência e rigidez da madeira. Cadernos de Engenharia de Estruturas, n.18, p.77-107, 2002. |
| [15] | Silveira, L. H. C.; Rezende, A. V.; Vale, A. T.Teor de umidade e densidade básica da madeira de nove espécies comerciais amazônicas. Acta Amazonica, v.43, p.179-184, 2013. |
| [16] | Logsdon, N. B. Influência da umidade nas propriedades de resistência e rigidez da madeira. Tese (Doutorado em Engenharia de Estruturas) - Escola de Engenharia de São Carlos, Universidade de São Paulo, 1998. |
| [17] | Logsdon, N. B.; Finger, Z.; Penna, E. S. Caracterização físico-mecânica da madeira de Cedro-marinheiro, GuareatrichiliidesL. (Meliaceae). Scientia Forestalis, v.36, p.43-51, 2008. |
| [18] | Logsdon, N. B.; Penna, E. S. Análise comparativa entre os coeficientes de anisotropia dimensional da madeira, no inchamento e na retração. Agricultura Tropical. v.8, n.1, 2004. |
| [19] | Vital, B. R.; Trugilho, P. F. Variação dimensional e uso da madeira de Eucalyptus. Informe Agropecuário, v.18, n.186, p.57-61, 1997. |
| [20] | Tsoumis, G.Science and technology of wood: structure, properties and utilization. New York: Van NastrndReinold, 1991. |
| [21] | Quartaroli, L.; Kuritza, J.; Rancatti, H.; Machado, G. O; Hillig, E. Propriedades físicas da madeira de Bracatinga (Mimosa scrabella Bentham) de ocorrência na região de Irati, PR. In: II Seminário de Atualização Florestal e XI Semana de Estudos Florestais, Brasil, Universidade do Centro do Paraná, 2010. |
| [22] | Müller, B. V.; Rocha, M. P.; Cunha, A. B.; Klitzke, R. J.; Nicoletti, M. F. Avaliação das principais propriedades físicas e mecânicas da madeira de Eucalyptus benthamii Maiden et Cambage. Floram: Floresta e Ambiente, v.21, p.535-542, 2014. |
| [23] | Montgomery, D. C. Design and analysis of experiments. Arizona: John WIley @ Sons Inc., 2005. |


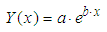
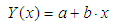
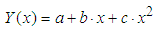
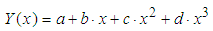
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML








