-
Paper Information
- Previous Paper
- Paper Submission
-
Journal Information
- About This Journal
- Editorial Board
- Current Issue
- Archive
- Author Guidelines
- Contact Us
International Journal of Materials Engineering
p-ISSN: 2166-5389 e-ISSN: 2166-5400
2016; 6(1): 15-21
doi:10.5923/j.ijme.20160601.03

Numerical Study of Finite Fracture Growth in an Epoxy Resin
André Luis Christoforo1, Márcio Eduardo Silveira2, Avelino Manuel da Silva Dias3, Victor Almeida De Araujo4, Francisco Antonio Rocco Lahr5
1Department of Civil Engineering, Federal University of São Carlos (UFSCar), São Carlos, Brazil
2Department of Mechanical Engineering, Federal University of São João del-Rei (UFSJ), São João del-Rei, Brazil
3Department Mechanical Engineering, Federal University of Rio Grande do Norte (UFRN), Natal, Brazil
4Research Group LIGNO of UNESP-Itapeva, Department of Forest Sciences, School of Agriculture Luiz de Queiroz of University of São Paulo (ESALQ/USP), Piracicaba, Brazil
5Department of Structural Engineering, Engineering School of São Carlos (EESC/USP), São Carlos, Brazil
Correspondence to: André Luis Christoforo, Department of Civil Engineering, Federal University of São Carlos (UFSCar), São Carlos, Brazil.
| Email: |  |
Copyright © 2016 Scientific & Academic Publishing. All Rights Reserved.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/

In the last decades, the study of fracture mechanics has been increase as tool capable to analyze the mechanical strength for structures without the occurrence of failure. The presence of small cracks can reduce the structural strength of the component enabling, in some cases, the collapse of the structure under a lower stress than the structural ultimate strength. Currently, the use of numerical methodologies, as finite element method (FEM), that address the problem of fracture in structural components to representing the macroscopic regime of the structure has been also used becoming increasing. The goal of the article, through the analysis of fracture and grounds of FEM, determine the fracture growth for a polymeric material (epoxy resin). Through ASTM E399 were established experimental procedures for obtaining fracture toughness (GC), the mechanical fracture bending SE (B) testing was choose, for its simplicity of design and manufacture. Three different numerical models were used to analyze the behavior the material and the fracture process. The results obtained from the numerical simulation were close to the literature results, confirming the effectiveness of the numerical tool used in predicting fracture phenomena in the material and its evolution associated with the loads application.
Keywords: Fracture, Finite Element Method, Epoxy resin
Cite this paper: André Luis Christoforo, Márcio Eduardo Silveira, Avelino Manuel da Silva Dias, Victor Almeida De Araujo, Francisco Antonio Rocco Lahr, Numerical Study of Finite Fracture Growth in an Epoxy Resin, International Journal of Materials Engineering , Vol. 6 No. 1, 2016, pp. 15-21. doi: 10.5923/j.ijme.20160601.03.
Article Outline
1. Introduction
- In the last decades, the fracture mechanics field has undoubtedly prevented a substantial number of structural flaws. If it is correctly applied, the fracture mechanics inhibits the flaws from the negligence during the project and of that due to the implementation of new projects with the utilization, or not, of new materials, which produce an unexpected and unwanted result in their behavior [1]. In these recent decades, new methods approach the problem of these structural failures through the numerical models with macroscopic organization of the structure [2-5]. More recently, discrete models of finite elements have been used along with the concepts of fracture mechanics, continuum mechanics and finite fracture mechanics by the evaluation of the structure damage [3, 6-8]. These approaches have been developed for both the analysis of structural flaws in ceramics, metals, polymers and, more recently, the composite materials. However, the correct incorporation of mechanical and phenomenological aspects inherent to failure mechanisms become dominant for success and effectiveness of such predictive methodologies applicable to numerical analysis of the mechanical integrity of a wide class of structural components and different material types [3, 9]. In this regard, over the years, the finite element method (FEM) has established itself as a simulate and study the nucleation and crack growth in the structures. The FEM include simple procedures for extrapolation of the stress intensity factor and the development of special elements (decohesion elements) for cracks to represent the failure region, as well as the stress in the crack’s front or tip [3, 6-10].This study aimed to evaluate the behavior of cracks or areas prone to damage in a polymeric material (epoxy resin) through different numerical models enabled for the study of fracture problems. It is noteworthy that the epoxy resin is a commonly used material as matrix phase in the development of various composites [4]. It was used a commercial software of finite elements, and three different analysis for study development were used [10]. Thus it is intended to contribute to the understanding of numerical techniques for determining of the behavior of process failures, enabling an improvement in the safety of structural projects.
2. Material and Methods
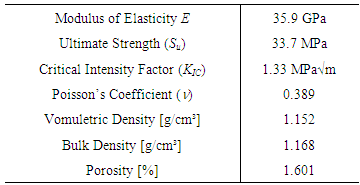
- Table 1 presents the data obtained in the literature for the mechanical behavior of commercial epoxy resin. This raw material has long been used as matrix phase of particulate composites and, in particular, the laminated composites of glass fiber, aramid fiber and carbon fiber. The latter composite material has been extremely used in the aerospace industry due to its excellent relation between mechanical strength and its weight (density). However, due to the mechanical characteristics of the matrix and of disperse phase, this composite has a fragile mechanical behavior. Therefore, it is possible to apply the mechanical concepts of linear elastic fracture to then study the other damage numerical models available on finite element solver [10].
|
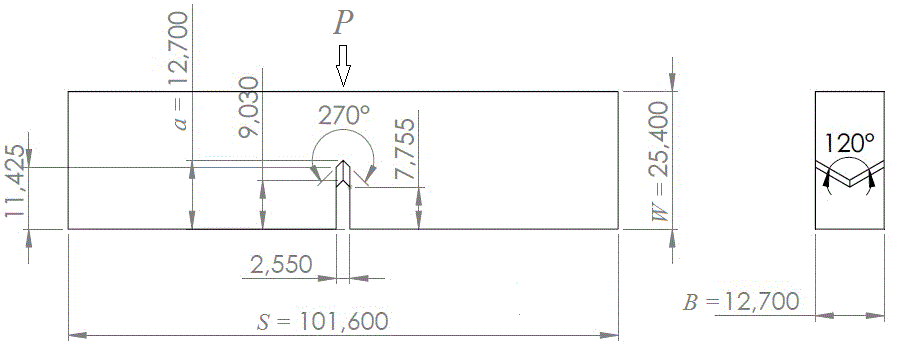 | Figure 1. Illustration of the SE (B) specimen with dimensions in millimeters [13] |
2.1. Linear Elastic Fracture Mechanics Model (LEFM)
- As can be seen in Medeiros et. al. [3], the concept of linear elastic fracture mechanics prescribes, for the tests with SE (B) specimens, some relationships between the mechanical properties of the material and geometrical properties of the specimen. In addition, as a condition for validation of this test, there is the need to ensure the existence of a plane condition of deformations at the crack tip, and these conditions are evaluated by Equation 1 [1, 3]. In this numerical simulation, KQ value corresponds to the critical stress intensity fator KIC material [13, 15].
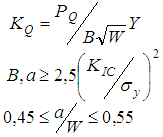 | (1) |
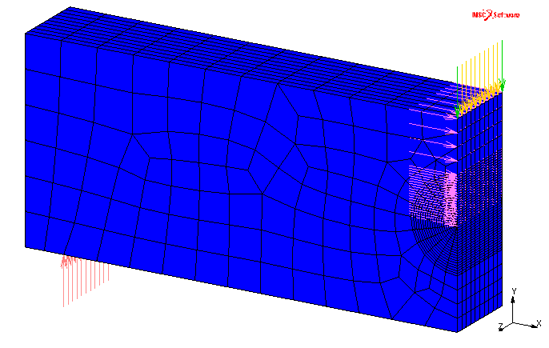 | Figure 2. 3D mesh of numerical model of SE (B) specimen considering ½ symmetry. 3.550 solid elements (3D) with eight nodes were used |
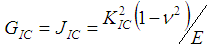 | (1) |
2.2. Cracking Strain Model
- To incorporate the numerical simulation to some mechanisms to examine fracture process, initially, constitutive relationship based on classic models of continuum mechanics was used, Figure 3, which was also known as cracking strain model [16, 17]. This model assumes an elastic behavior to the breaking point of studied material or critical cracking stress (σcr). After reaching this limit, it is estimated that its damage is characterized by a cracking strain of the material in the normal direction to the maximum principal stress (Rankine theory) and the material is replaced by an orthotropic behavior (references). This model allows the incorporation of a reduction behavior in the strength of the structure after the formation of first crack, described by a parameter of tension-softening modulus (ES).
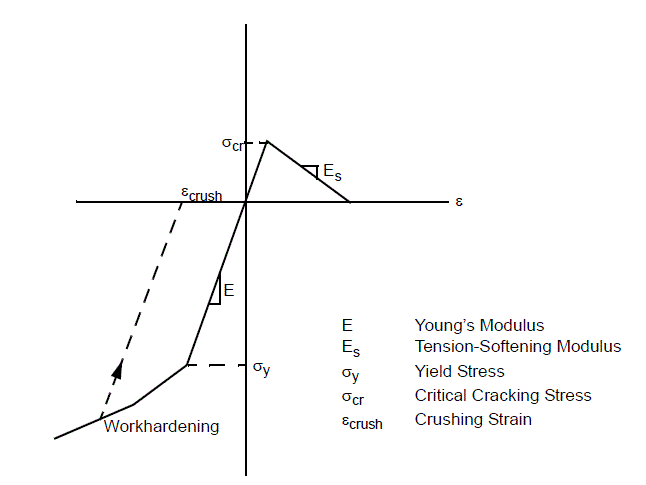 | Figure 3. Uniaxial Stress-Strain Diagram for Cracking Strain Model [10] |
2.3. Virtual Crack Closure Technique Model (VCCT)
- Another way to study the crack growth is the direct approach, or fracture mechanics approach. This approach directly utilizes fracture mechanics criteria in which the strain energy release rates (G`s) are computed and compared to the corresponding critical valor, for example, fracture toughness in mode I (GIC). Recently, the virtual crack closure technique is the most popular and powerful tool to approximately computes G`s values, specially, because VCCT is simple, efficient and robust in analyzing any crack growth problems [8]. Instead of the fact that these analyses almost have a very expensive computational cost.Figure 4 shows the 3D mesh used in this analysis, which is able to represents the SE (B) specimen, whereas their dimensions were shown in Figure 1. Since this analysis was adopted a procedure of automatic generation of meshes during the simulation (automesh), it was opted a first rough mesh, with four hundred 3D solid elements of eight nodes. In addition, the specimen was modeled without taking advantage of its symmetry due to the use of automesh to refine the crack front. In order to a better numerical control during the processing [18], the external load was applied through a prescribed displacement applied in the middle of the span (y). The value of this prescribed displacement was obtained through the first model used here, i.e., through the simulation of fracture mechanics model. Finally, as the crack growth criterion, it was used the value of toughness fracture (GIC) for epoxy resin obtained through the Equation (2). It was used a hundred increments and no convergence difficult has been found during the crack growth analyses.
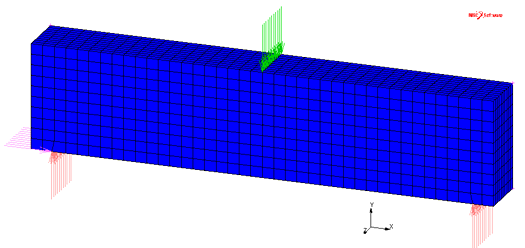 | Figure 4. 3D mesh initially used in VCCT analysis for the numerical model for specimen SE (B) with 400 3D solid elements with 8 nodes |
3. Results and Discussions
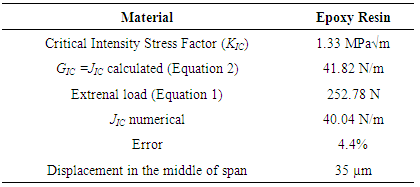
- Table 2 shows the results obtained by numerical simulation using the mechanical model of linear elastic fracture showed in Figure 2. The external load applied in bending specimen SE (B) was P = 252.78 N.
|
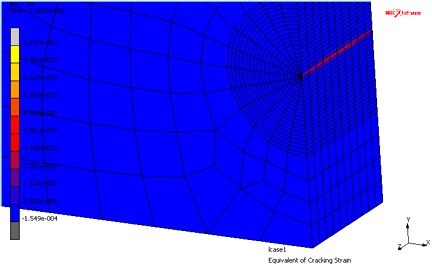 | Figure 5. Numerical distribution of equivalent cracking strain in front of crack |
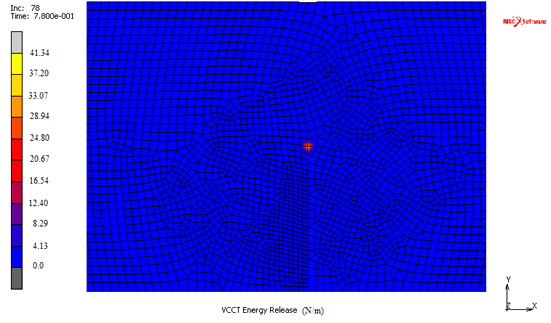 | Figure 6. Zoom of the mesh in front of the crack for the VCCT model of the epoxy resin |
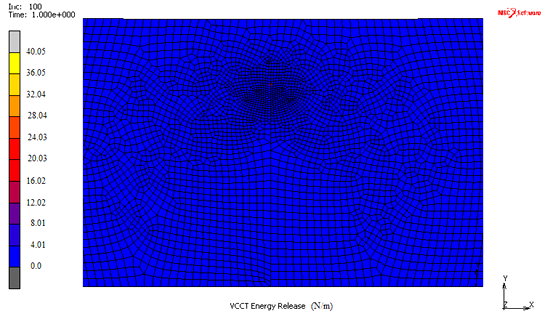 | Figure 7. Zoom of the mesh in front of the crack for the VCCT model of the epoxy resin in the end of test |
4. Conclusions
- A major advantage of the adoption of numerical analysis is in its low cost, but with great precision, speed and reliability, in addition to reduce the spent time in the experimental tests performance of the same style. However, these analyses require a high knowledge of the concepts related to the methods and analytical and numerical models [21]. Therefore, this research aimed to study the fracture processes in epoxy resin through its numerical simulation. In this study, three different numerical models were adopted to evaluate a fracture mechanics test defined by ASTM E399 standard [13]. The selected test was the bending at three points SE (B) due to its ease of numerical modeling and because it is widely used in the analysis of ceramic materials, polymers, and recently laminated composites. The first simulated model was the linear elastic fracture mechanics, through the calculation of the Critical Stress Intensity factor (KIC) of the epoxy resin found in the literature (Table 1). In the numerical analysis, the integral J was used to evaluate the model, whereas it is the parameter adopted by the finite elements software [10]. The numerical model was able to represent the SE (B) test, as well as reproduce the singularity in the region in front of the crack. The difference between the numerical value of JIC and experimental was 4.4%. This analysis showed quickly, because the material presented a linear elastic behavior. However, its main limitations are due to the attention in the generation of the numerical singularity in front of the crack.The second tested model was of cracking strain model. In the numerical simulation of SE (B) was possible to verify that this analysis represented the mechanical behavior of the experimental test, whereas the region in front of the crack reached the breaking point when they were given in the maximum load defined by the linear elastic fracture mechanics (Figure 4).The last model tested was the VCCT with the utilization of the automesh process during the simulation. This model showed the crack growth with more precision, which may be used to simulate the nucleation process of this crack. The initial mesh can be relatively rough, considering that the numerical processing with automesh establishes the necessary conditions for the representation of the singularity in front of the crack. However, the high computational spent in this model makes its use somewhat restricted, if it is compared to previous models.Finally, all analyzed models were able to represent the mechanical behavior of specimen in the bending test SE(B). Despite the specialized literature presents several studies using different models of finite element in numerical simulations [5], rarely is found a same work about the comparison of failure mechanisms studies. Comparing the three studied models in termos of difficulties in the mesh generation and computational costs, it can be concluded that the diffuse crack model was able to simulate the mechanical behavior of the test SE(B) and to capture the characteristics of the formation of cracks during these conventional tests of fracture mechanics. This model also presented a low computational cost when is compared with the powerful VCCT model. Therefore, it is recommended to adopt this model in bi modularity in the first step in the structural design process to identify areas prone to nucleation and growth of cracks. Then, an upgrade could occur in the simulation including the VCCT model, but restricting and directing in the critical areas defined in the simulation with the bi modularity model. The importance of the LEFM model would limit to validate the studies in the cases when there were difficulties in the obtaining mechanical properties derived from complex mechanical tests [20].
ACKNOWLEDGEMENTS
- The authors recognize the National Council for Scientific and Technological Development (CNPq) for financial support through process 460943/2014-6. One of them also thanks for the CAPES (Higher Coordination for Scientific and Educational Training), through process BEX 6569-14.
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML