Babatunde Bolasodun1, Olubukola Rufai1, Alan Nesbitt2, Richard Day3
1Department of Metallurgical and Materials Engineering, University of Lagos, Nigeria
2National Composites Certification and Evaluation Facility, School of Materials, University of Manchester, M1 7HS, United Kingdom
3Glyndwr University, Mold Road, Wrexham, LL11 2AW, Wales
Correspondence to: Babatunde Bolasodun, Department of Metallurgical and Materials Engineering, University of Lagos, Nigeria.
| Email: |  |
Copyright © 2014 Scientific & Academic Publishing. All Rights Reserved.
Abstract
Comparative studies were carried out on the cure kinetics of an epoxy resin Araldite LY 5052 and a hardener, 4 4’ Diaminodiphenyl sulfone. Differential scanning calorimetry and a microwave heating calorimeter were used to monitor the curing kinetics of conventionally and microwave cured epoxy samples. These studies were carried out under isothermal conditions using four different temperatures. There was a significant increase in the fractional conversion of the microwave cured samples compared to the conventionally cured samples. The curing reactions for samples cured using microwave heating took place over a smaller temperature range. Higher reaction rates were observed in the samples cured using microwave heating.During the isothermal curing, higher K1 and K2 kinetic parameters were observed in microwave cured samples. A lower K1 / K2 ratio was observed in microwave curing than in conventional curing. This is attributed to the enhancement of the catalytic reaction over the non-catalytic reaction by the microwave radiation which occurs as a result of the high activity of the [OH] group.
Keywords:
Isothermal curing, Epoxy, Microwave heated calorimeter, Cure kinetics
Cite this paper: Babatunde Bolasodun, Olubukola Rufai, Alan Nesbitt, Richard Day, Comparison of the Isothermal Cure Kinetics of Araldite LY 5052 / 4 4’ DDS Epoxy System Using a Differential Scanning Calorimetry and a Microwave Heated Calorimeter, International Journal of Materials Engineering , Vol. 4 No. 4, 2014, pp. 148-165. doi: 10.5923/j.ijme.20140404.05.
1. Introduction
An epoxy is a thermosetting resin which usually starts out as a liquid and is converted into a solid by a chemical reaction only. When cured, epoxies become irreversibly rigid. An epoxy based polymer is mechanically strong, and in its solid form, it is chemically resistant to degradation. They are also highly adhesive during conversion from liquid to solid. These properties when put together, make epoxy materials very versatile [1].Epoxy systems are made up of two essential components, a resin and a hardener. Sometimes there is a third component which is an accelerator. The resin component is the epoxy while the hardener is what the epoxy reacts with chemically and it is often a type of amine. When the epoxy and amine are added together and mixed in a specific way and then heated, they will react chemically and link together irreversibly. When the full reaction is complete, the resulting product is a rigid plastic polymer material [1].Due to increasing applications of epoxy materials as high performance structural adhesive systems in the aerospace and the microelectronic industries, the demand for epoxies is on the rise. This rise has lead to the exploration of faster and more efficient methods of manufacturing epoxy materials [2].Although thermal curing increases the rate at which the material cures and lowers the time taken for it to cure, it is limited by the fact that for any given system, the maximum rate of reaction has an optimum temperature. If a resin or a material is heated to a temperature higher than its optimum temperature, there would not be an increase in its reaction rate, but instead the material would start to degrade thermally [2].Alternatives to thermal curing which can accelerate the rate of reaction, reduce the cure time or provide a more energy efficient method for curing epoxy resins have been looked into. These alternatives were the use of ultraviolent light, electron beams, gamma rays and microwave energy [2, 3].Ultraviolet light has a poor ability to penetrate into the material. It also has a restricted close rate. Due to this, application of ultraviolet light in curing materials is only possible in very limited circumstances [2, 3].Gamma rays are usually delivered from naturally radiating sources such as cobalt-60, but there are several health and environmental concerns which are associated with the radiation hazards from gamma rays. Due to these concerns, they are hardly used [2].Curing with electron beams has proven to be an efficient and a quick method of curing, however, its disadvantage is that high costs are involved with the operation using this method [2, 3].Microwave heating uses the ability of some liquids and solids to convert electromagnetic energy into heat. It is based on the principle that a material can be heated by applying energy to it in the form of high frequency electromagnetic waves. An electromagnetic radiation consists of an electric field whose plane is perpendicular to the plane of a magnetic field. The microwave heating effect originates from the interaction of the electric field component of the microwaves with charged particles in the materials. A current will be induced through the material if the charged particles are able to move through the electric field. If the particles can not move because they are bonded to the material, they will simply rearrange themselves in phase with the electric field. This mechanism is called dielectric polarisation [4]. Any increase or decrease in the microwave input power leads to a corresponding increase or decrease in the temperature of the material undergoing cure. Unlike conventional heating which heats the material being processed, along with the walls of the oven and the air surrounding the process material, microwave heating affects only the material being processed. This makes microwave heating a more energy efficient method of heating materials being processed, and this translates into lower production costs for microwave heating [3]. This article presents the results of the kinetic studies on the conventional and microwave cure of Araldite LY5052 / 4 4’ DDS epoxy system.
2. Experimental Procedure
2.1. Materials
This research was carried out at the National Composites Certification and Evaluation Facility, Manchester. Araldite LY 5052 was the epoxy resin used for this research. It was supplied by Hexcel, UK. Araldite LY 5052 has an epoxide weight equivalent of 148.33. The hardener used for this study was 4 4’ Diaminodiphenyl sulfone. It was supplied by Sigma-Aldrich, UK.
2.2. Curing Procedure
A PerkinElmer Pyris 1 DSC machine was used for the thermal curing of the Araldite LY 5052 / 4 4’ DDS epoxy system. Samples weighing between 2-5 mg were placed in aluminium sample pans; and were cured in a nitrogen atmosphere at isothermal temperatures of 170, 180, 190 and 200℃. The samples were held at these temperatures for 300, 240, 200 and 200 minutes respectively.A microwave calorimeter shown in figure 1 was used to carry out isothermal microwave curing of the samples. The microwave curing was carried out in a cylindrical brass, single mode cavity operating in a TE111 mode which was designed to give maximum field strength at the centre of gravity. A Hewlitt-Packard 8720ET was used to generate microwaves at a frequency of 2.45GHz; which were then amplified to a maximum power of 30 W with a solid state amplifier. An Anritsu power meter was used to measure the transmitted and reflected powers. The power required for heating the sample was calculated by subtracting the transmitted and reflected powers from the amplifier power. This power is similar to the heat flow to the DSC cell in the sense that there will be a decrease in the microwave power during exothermic reaction, while an endothermic reaction will require an increase in the microwave power.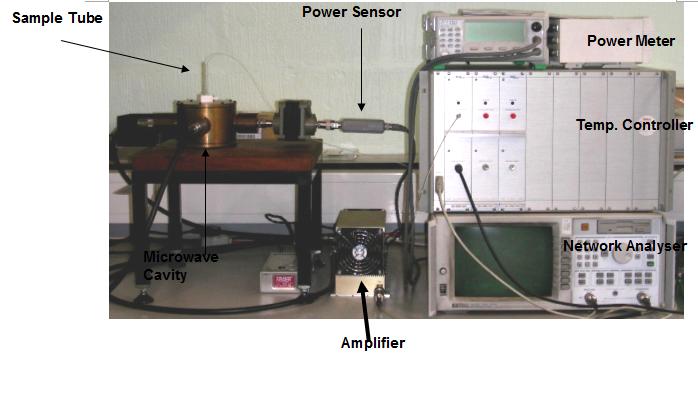 | Figure 1. Microwave Heated Calorimeter used for Isothermal cure |
 | Figure 2. A Perkin- Elmer Pyris 1 power compensated DSC |
For microwave curing, epoxy resin samples weighing between 0.3-0.5g were poured in glass test tubes and placed in the centre of the cavity. A flouroptic thermometer was inserted in a 1.5 mm diameter glass tube placed in a test tube.
3. Results and Discussion
3.1. Kinetic Analysis
Epoxy resin curing is an exothermic reaction. For an exothermic reaction, if we assume that [5]1) During curing the exothermic heat generated is proportional to the number of double bonds which have reacted in the system.2) That maximum conversion is attained when all the bonds that can react have reacted3) The rate of reaction during cure is directly proportional to the rate of heat generation.At time t, is becomes possible to determine the degree of conversion α, and the reaction rate 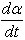 at time t [15]. This can be determined by the following expressions.
at time t [15]. This can be determined by the following expressions.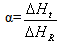 | (1) |
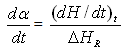 | (2) |
Where 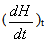 is the rate of heat generation and is directly related to the calorimetric signal at time t, and ΔHR is the total reaction heat associated with the complete conversion of all the reactive groups , and ΔHt is the heat released until time t. This can be obtained directly by integrating the calorimetric signal
is the rate of heat generation and is directly related to the calorimetric signal at time t, and ΔHR is the total reaction heat associated with the complete conversion of all the reactive groups , and ΔHt is the heat released until time t. This can be obtained directly by integrating the calorimetric signal 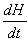 until the time t [15].
until the time t [15].
3.2. Modelling of Cure Kinetics
A crucial step in the study of cure kinetics by DSC is fitting of the reaction rate profile obtained from either isothermal or dynamic experiments, to a kinetic model. There are essentially two forms of kinetic models used to describe thermoset curing reactions; empirical and mechanistic models [6]. Due to the complexity of the mechanistic models, the empirical kinetic method is more preferable for practical and comparison purposes. In empirical models, the whole reaction is considered as a single kinetic process, regardless of the different reactive processes or the different stages evolving within the system [5].Empirical models are based on the assumption that the over all reaction rate, 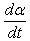 can be expressed as [5]:
can be expressed as [5]: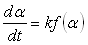 | (3) |
where α is fractional conversion, f(α) is a function of fractional conversion, and k is the rate constant which obeys the Arrhenius relationship: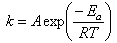 | (4) |
where A is pre-exponential rate constant (i.e. also known as the collision frequency factor), Ea is the activation energy, R is the gas constant, and T is the absolute temperature.The simplest empirical model corresponds to an nth-order equation: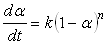 | (5) |
where n is the reaction order. This model is applied to a curing system showing no autocatalytic phenomena and no complexity in the reaction mechanism [7]. In the case where the curing system exhibits autocatalytic effect, i.e. a maximum of the reaction rate is observed at some point other than the beginning of the reaction in an isothermal cure, the kinetic model cannot be applied and consequently, the so-called autocatalytic model must be used. For an autocatalytic reaction in which the initial reaction rate is zero, the following equation is applied [8]. 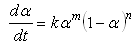 | (6) |
If the initial reaction rate is not zero, the autocatalytic model is given by [134]: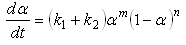 | (7) |
where m and n are the reaction orders.K1 is the rate constant associated with the non-catalytic reaction, while K2 is the rate constant associated with the autocatalytic reactions. K1 and K2 usually obey the Arrhenius relation. K1 and K2 are functions of temperature. m and n represent the reaction orders. m indicates how much of the curing reaction follows the autocatalytic path, while n indicates how much of the curing reactions follow the non-catalytic path. The introduction of these four parameters enable us to obtain a good fit to the experiment data. The values of m and n are found to vary from experiment to experiment. It is quite usual to assign a value of m + n = 2 when using the autocatalytic model. Some researchers have used this value [9] whereas others allowed it to float and the reaction orders were adjusted until the best optimisation of the kinetic parameters were obtained and the value of m + n were reported to vary, for instance, from 1.0 [10] to 7.2 [11]. The autocatalytic model has been widely used by many researchers to study the reaction kinetics of various thermoset polymers including epoxy resins [7, 12], and urethane acrylate resins [13].Although the empirical models are based on the isothermal experiments, several authors [5, 7] have demonstrated that these models are also valid in dynamic experiments with a simple modification of the rate equation (Equation 5.6) and that;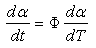 | (8) |
where Φ is the linear heating rate.
3.3. Determination of Appropriate Stoichiometric Molar Ratio
In order to choose the appropriate stoichiometric amine / epoxy (A/E) ratio value to be used for this research, Araldite LY 5052 / 4 4’ DDS epoxy systems were prepared with amine / epoxy stoichiometric ratios of 0.5, 0.6, 0.7, 0.8, 0.9, 1.0, 1.1, 1.2. DSC scans were carried out on these systems. The systems were heated at 10 K min-1 from 30 to 350℃ in order to cure the sample. It was then cooled back to 0℃, and reheated at a heating rate of 10 K min-1 in order to determine the glass transition temperature value. The epoxy amine / epoxy ratio which gave the highest Tg was 0.8 and was subsequently used for this research.Similar samples of Araldite LY 5052 / 4 4’ DDS epoxy system with amine / epoxy ratios of 0.5, 0.6, 0.7, 0.8, 0.9, 1.0, 1.1, 1.2 were prepared for microwave curing. A heating rate of 10 K min-1 was used to cure the sample from 30 to 310℃, and allowed to cool. Pieces of the cured samples were extracted from the microwave sample tube, and a DSC scan was carried out on the extracted piece from 30 to 350℃ at a heating rate of 10 K min-1 in order to determine its glass transition temperature.Both plots in figure 3 show a increase in Tg values as the Amine / Epoxy ratio increases up to a maximum, and then the Tg starts to decrease. The highest Tg values of conventional and microwave heated samples occur at different stoichiometric ratios. The highest Tg value of the microwave cured sample occurs at a higher Amine / Epoxy Molar ratio than conventional heating. This is an early indication that the curing mechanisms for conventional and microwave curing are different.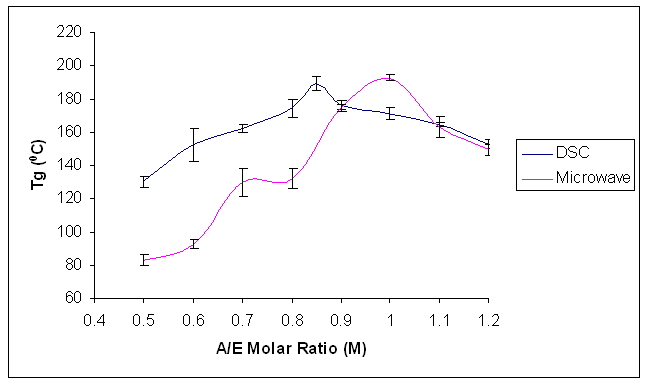 | Figure 3. Plot of Glass Transition against Molar ratio for different stoichiometric ratios for both conventional and microwave cured samples of Araldite LY 5052 / 4 4’ DDS epoxy system |
 | Figure 4. Typical DSC thermograms for Araldite LY 5052 / 4 4’ DDS epoxy system system with an amine / epoxy ratio of 0.85 at different heating rates using conventional DSC |
Figure 5 shows the reaction rate increasing as the reaction temperature increases. The increase in the reaction temperature causes a distinct distribution of molecular speeds and energies followed by an increase in the average value of energy from the reactant molecules. As a result, a larger number of molecules have enough activation energy to surpass the activation barrier, and this leads to an increase in the reaction rate [14-16].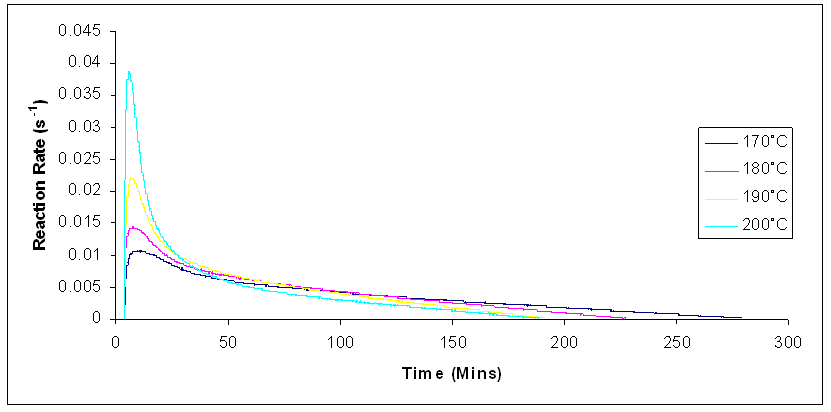 | Figure 5. Reaction rates for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system system with an amine / epoxy ratio of 0.85 using conventional heating |
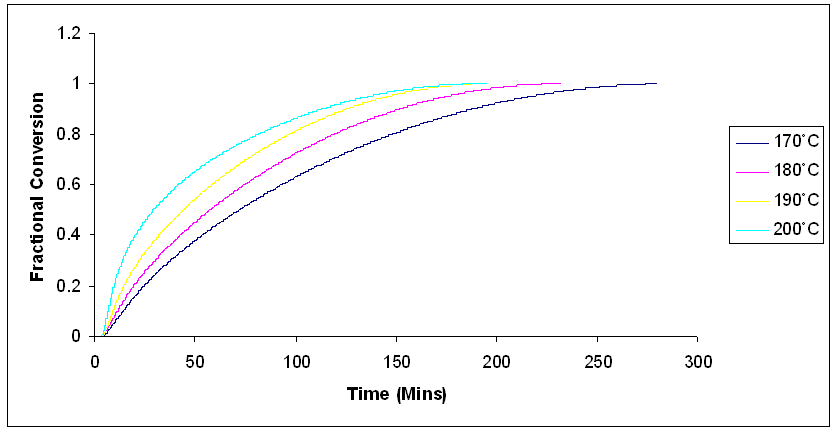 | Figure 6. Fractional conversion for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 0.85 using conventional heating at different isothermal temperatures |
A plot of fractional conversion against reaction rate for all the heating rates is shown in the figure 7. Typical changes are found to occur in the reaction rate over the whole range of conversion. For isothermal cure, the reaction rate increases and it quickly reaches a maximum at the early stages of the reaction, in the fractional conversion range of 0 – 0.15. Beyond this range, the reaction rate started to decrease. This decrease is ascribed to the increase in the viscosity of the reaction medium as the curing material gelled [17]. There was a significant reduction in molecular mobility of the reactants at this stage. The reaction became diffusion controlled, and it eventually stopped. It was also observed that the reaction rate increased as the curing temperature increased. Also, at higher temperatures, the time taken to attain full cure becomes lower. | Figure 7. Plot of Reaction rate against Fractional Conversion for the curing reaction of Araldite LY 5052 / 4 4’ DDS epoxy system system with an amine / epoxy ratio of 0.85 at different isothermal temperatures |
Typical DSC thermograms for the cure of Araldite LY 5052 / 4 4’ DDS epoxy system with amine / epoxy ratio of 1.0 are shown in figure 8. 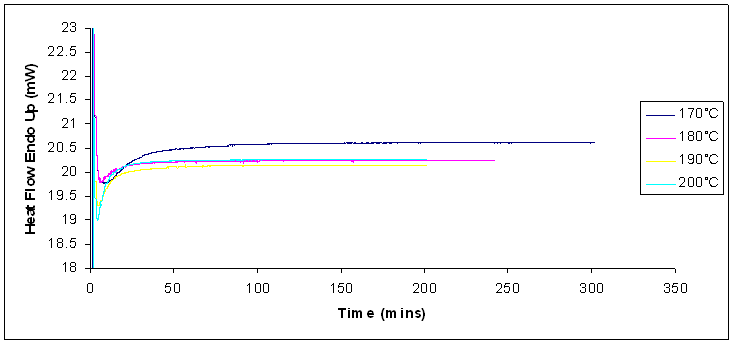 | Figure 8. DSC thermograms for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 obtained from conventional DSC at different heating rates |
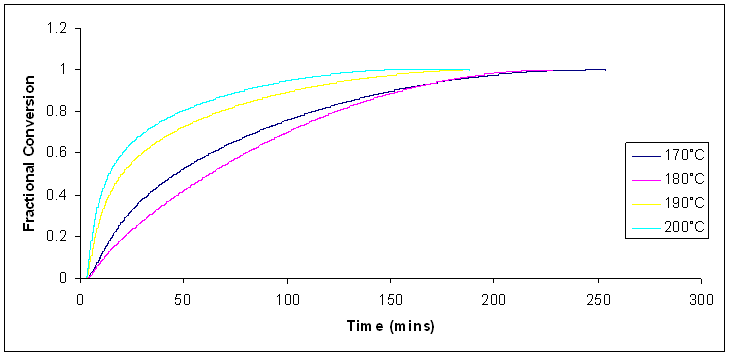 | Figure 9. Fractional conversion for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 at different heating rates using conventional heating |
Figure 10 shows the reaction rate increasing as the reaction temperature increases. As with figure 5, the increase in the reaction temperature causes a distinct distribution of molecular speeds and energies followed by an increase in the average value of energy from the reactant molecules. As a result, a larger number of molecules have enough activation energy to surpass the activation barrier, and this leads to an increase in the reaction rate [14-16].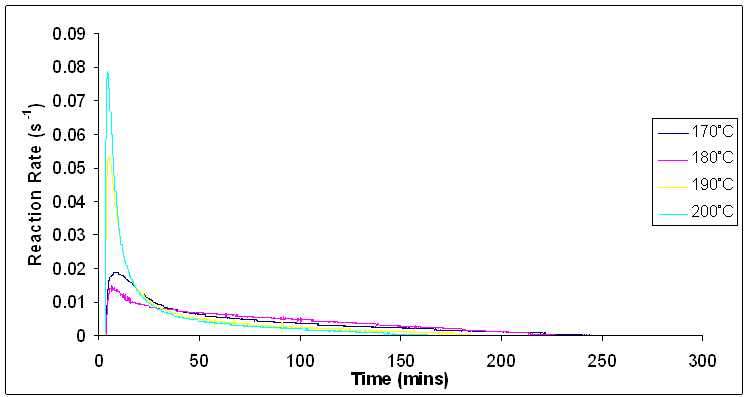 | Figure 10. Reaction rates for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 at different heating rates using conventional heating |
From figure 11, typical changes are found to occur in the reaction rate over the whole range of conversion. For isothermal cure, the reaction rate increases and it quickly reaches a maximum at the early stages of the reaction, in the fractional conversion range of 0 – 0.15. Beyond this range, the reaction rate started to decrease. This decrease is ascribed to the increase in the viscosity of the reaction medium as the curing material gelled [17]. There was a significant reduction in molecular mobility of the reactants at this stage. The reaction became diffusion controlled, and it eventually stopped. It was also observed that the reaction rate increased as the curing temperature increased. Also, at higher temperatures, the time taken to attain full cure becomes lower.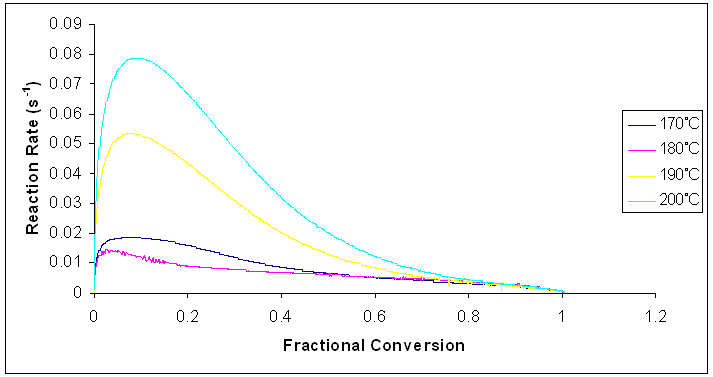 | Figure 11. Rate of reaction against fractional conversion for the curing reaction of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 at different heating rates |
3.4. Isothermal Microwave Curing
A microwave-heated calorimeter was used to cure the samples with microwave heating using the same isothermal conditions as for conventional heating. The temperature dependence of the fractional conversion and the reaction rates at different temperatures are shown in figures 12 and 13 below.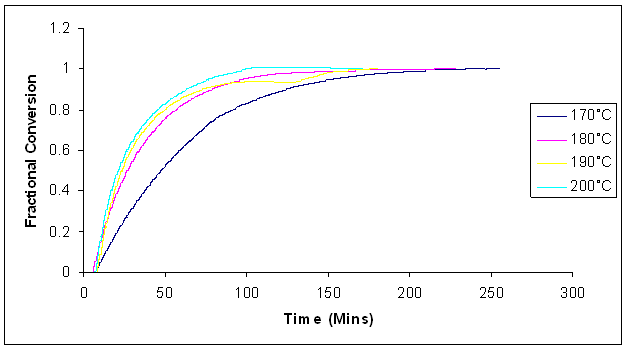 | Figure 12. Fractional conversion of dynamic cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 0.85 at different isothermal temperatures using microwave heating |
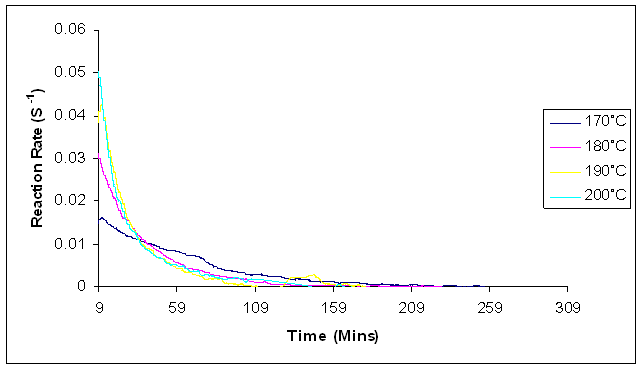 | Figure 13. Plot of Reaction rates against temperature for dynamic cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 0.85 at different isothermal temperatures using microwave heating |
Figure 14 shows a plot of fractional conversion against reaction rate for all the isothermal temperatures. Typical changes are found to occur in the reaction rate over the whole range of conversion. Just as in conventional curing, the reaction rate increases and it quickly reaches a maximum at the early stages of the reaction within the fractional conversion range of 0 – 0.15 after which the reaction rate started to decrease. This decrease is ascribed to the increase in the viscosity of the reaction medium as the curing material gelled [17]. The reaction rate increased as the curing temperature increased. The curing times are lower at higher temperatures.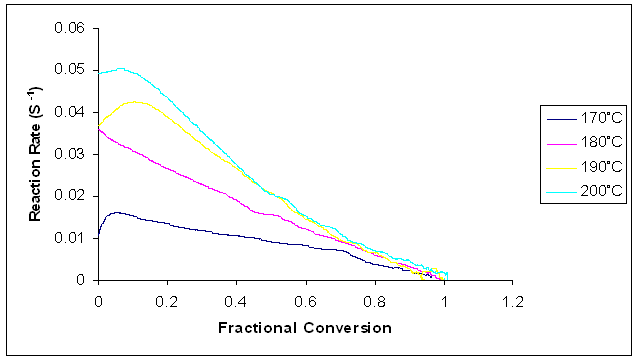 | Figure 14. Plot of reaction rates against fractional conversion for the microwave cure reaction of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 0.85 at different isothermal temperatures |
Figures 15 and 16 shows the plots of the temperature dependence of the fractional conversion and the reaction rates of cured microwave cured samples of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0.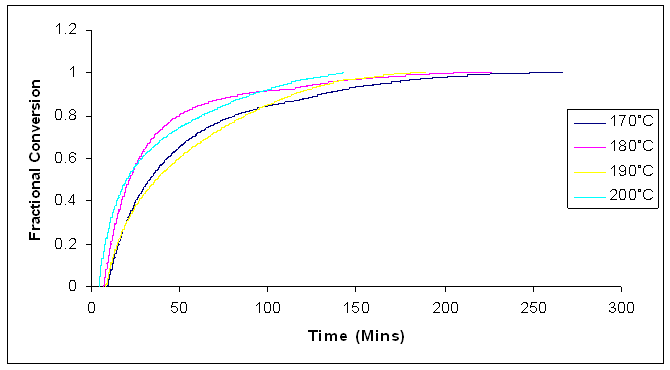 | Figure 15. Fractional conversion for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 at different isothermal temperatures using microwave heating |
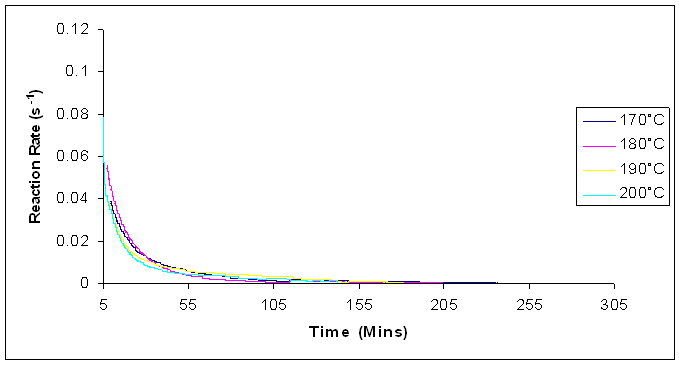 | Figure 16. Reaction rates for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 at different isothermal temperatures using microwave heating |
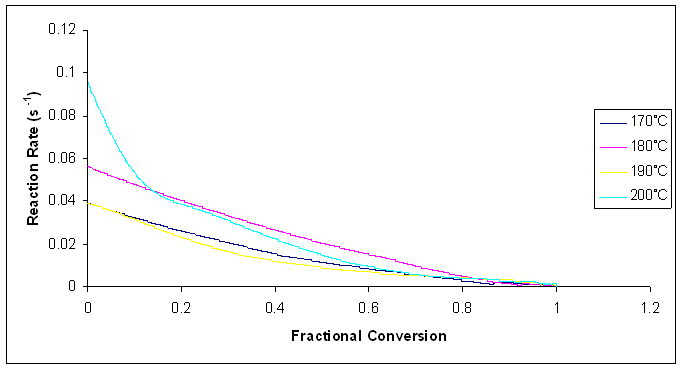 | Figure 17. Plot of reaction rates against fractional conversion for the microwave cure reaction of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 at different isothermal temperatures |
Plots of the time dependence of fractional conversion and reaction rate for the microwave and conventional curing of Araldite LY 5052 / 4 4 DDS epoxy system are shown in the figures 18 and 19 below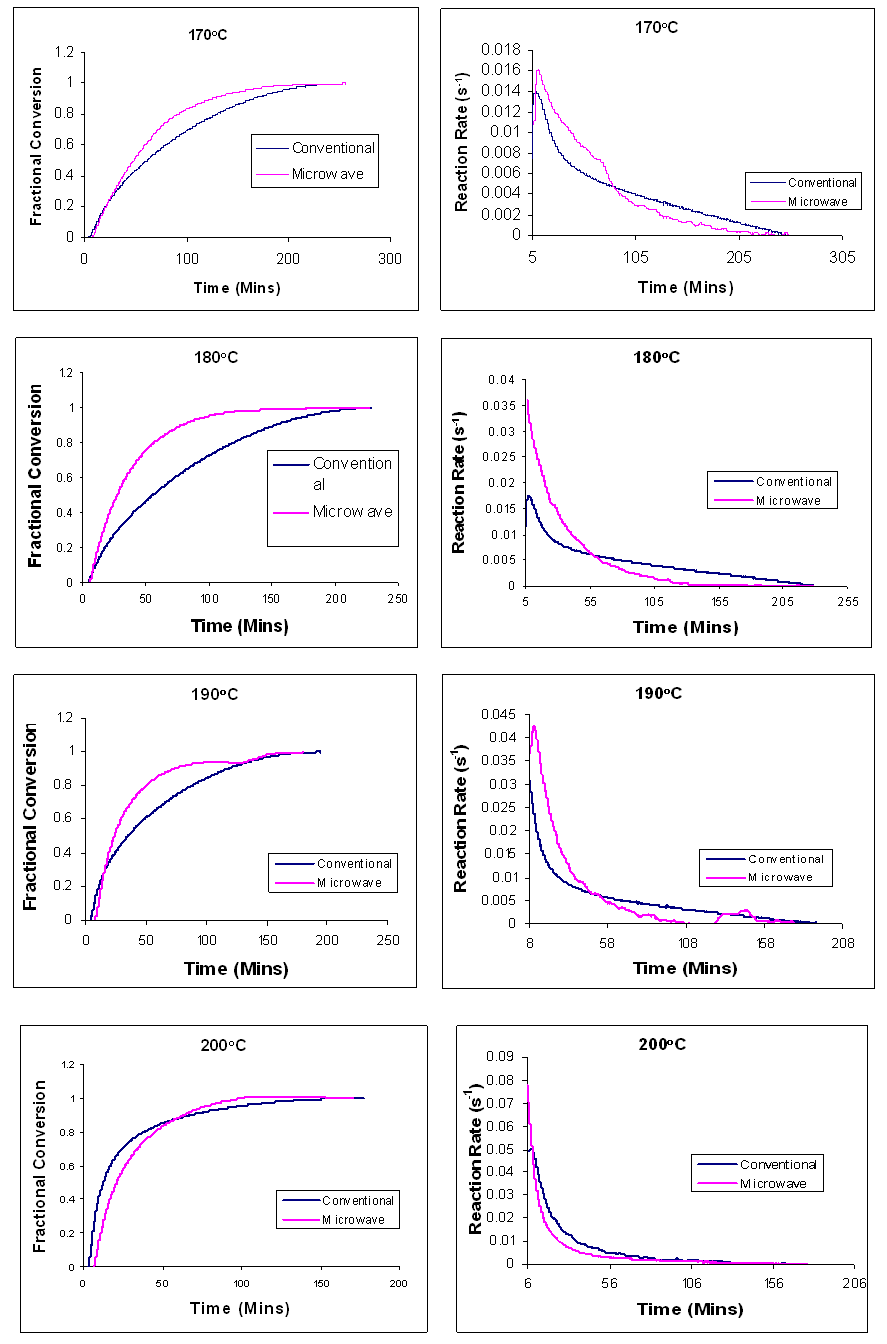 | Figure 18. Time dependence of the fractional conversion (right) and the reaction rate (right) for Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 0.85 under conventional and microwave curing, at different isothermal temperatures |
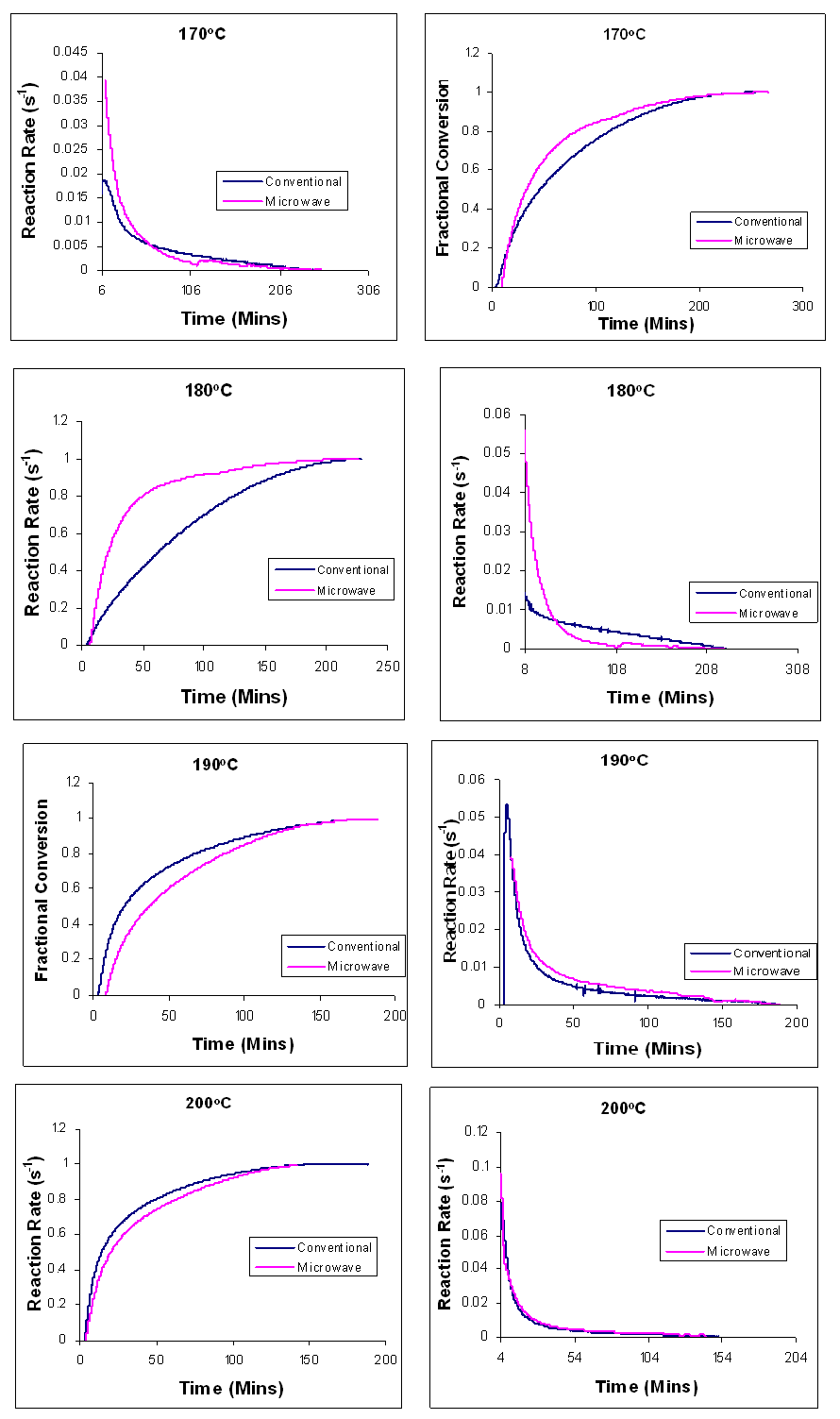 | Figure 19. Time dependence of the fractional conversion (left), and reaction rate (right) for the curing of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 under conventional and microwave curing, at different isothermal temperatures |
Figures 18 and 19 show the comparison of the time dependence of the fractional conversion and the reaction rates at different heating rates for both conventional and microwave cured Araldite LY 5052 / 4 4’ DDS epoxy system. The microwave heated samples had a higher peak rate of reaction than the conventionally cured samples. When compared to the conventionally cured samples, there was a significant increase in the reaction rate of the microwave cured samples. For all the heating rates, higher reaction rates were observed in microwave cured samples. As explained earlier during the dynamic curing of both epoxy systems, These differences between the conventional and microwave cured systems are all as a result of an improved efficiency in the transfer of energy for the microwave heating because microwave heating involves a direct interaction with the molecules with the electromagnetic field causing heat to be generated internally throughout the volume of the material [18], unlike conventional heating where energy is transferred from the surface of the material into the material via conduction or convection. Polymer molecules are heated in the microwave field directly as a result of the relaxation of the dipole polarization along the electromagnetic field. While conventional heating requires the entire molecule to first be heated, Microwave heating enable the reactive polar molecules to selectively absorb the microwave, and this enhances the reaction [18]. The higher fractional conversion for the microwave cured samples can be as a result of an increase in the reactant mobility after gelation. This is as a result of the induced polarization of the polymer and monomer molecules along the applied electromagnetic field [13], enabling more reactants to be consumed to form a more rigid network.
3.5. Modelling of Cure Kinetics
The reaction rate profile obtained from both the conventional and microwave isothermal cured samples were fitted to a kinetic model. Figures 20 and 21 compares the reaction rate profile of both conventionally cured (Left) and microwave cured (Right) Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 0.85 obtained experimentally and the reaction rate profile predicted by the autocatalytic model.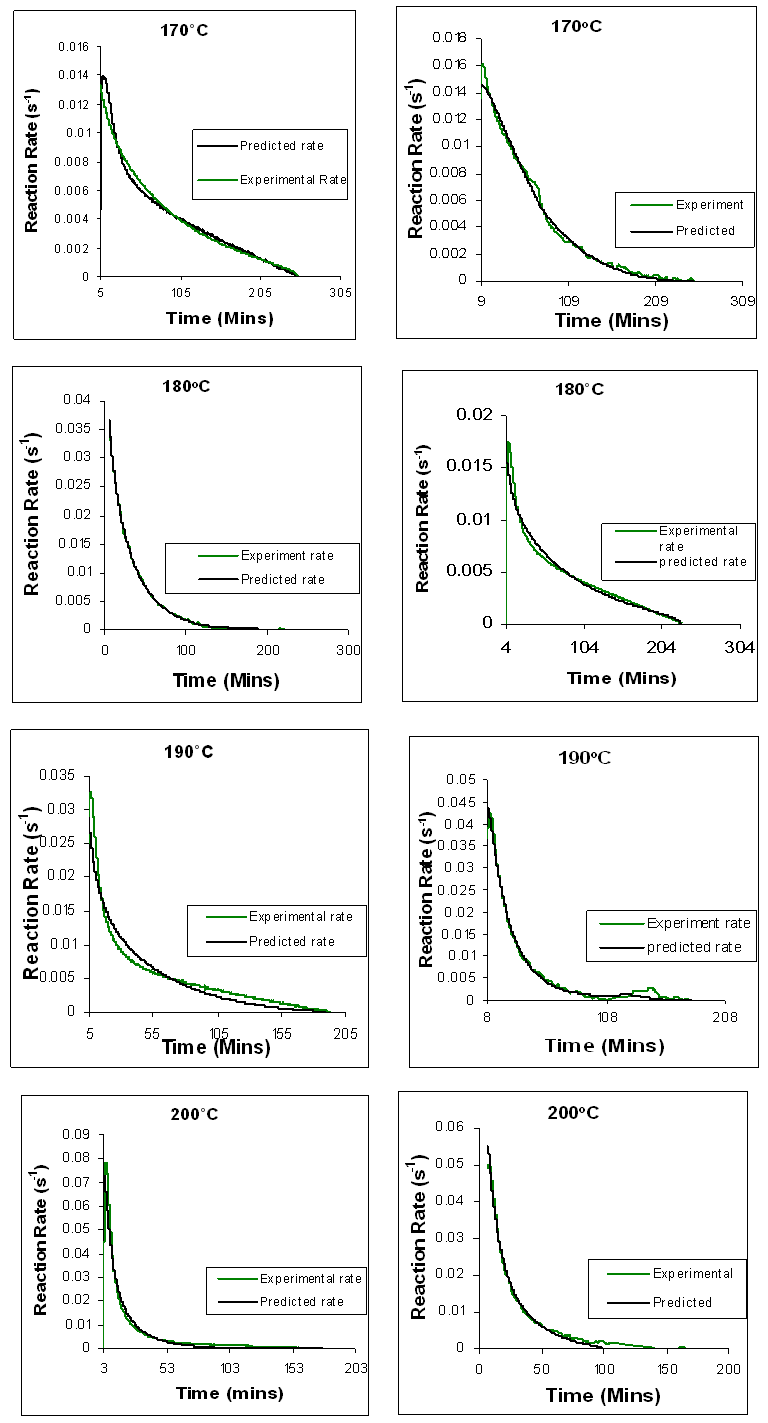 | Figure 20. Comparison between time dependence of experimental reaction rate curves and the curves predicted by means of autocatalytic adjustment for conventional heating of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 0.85 on the left, and microwave heating on the right |
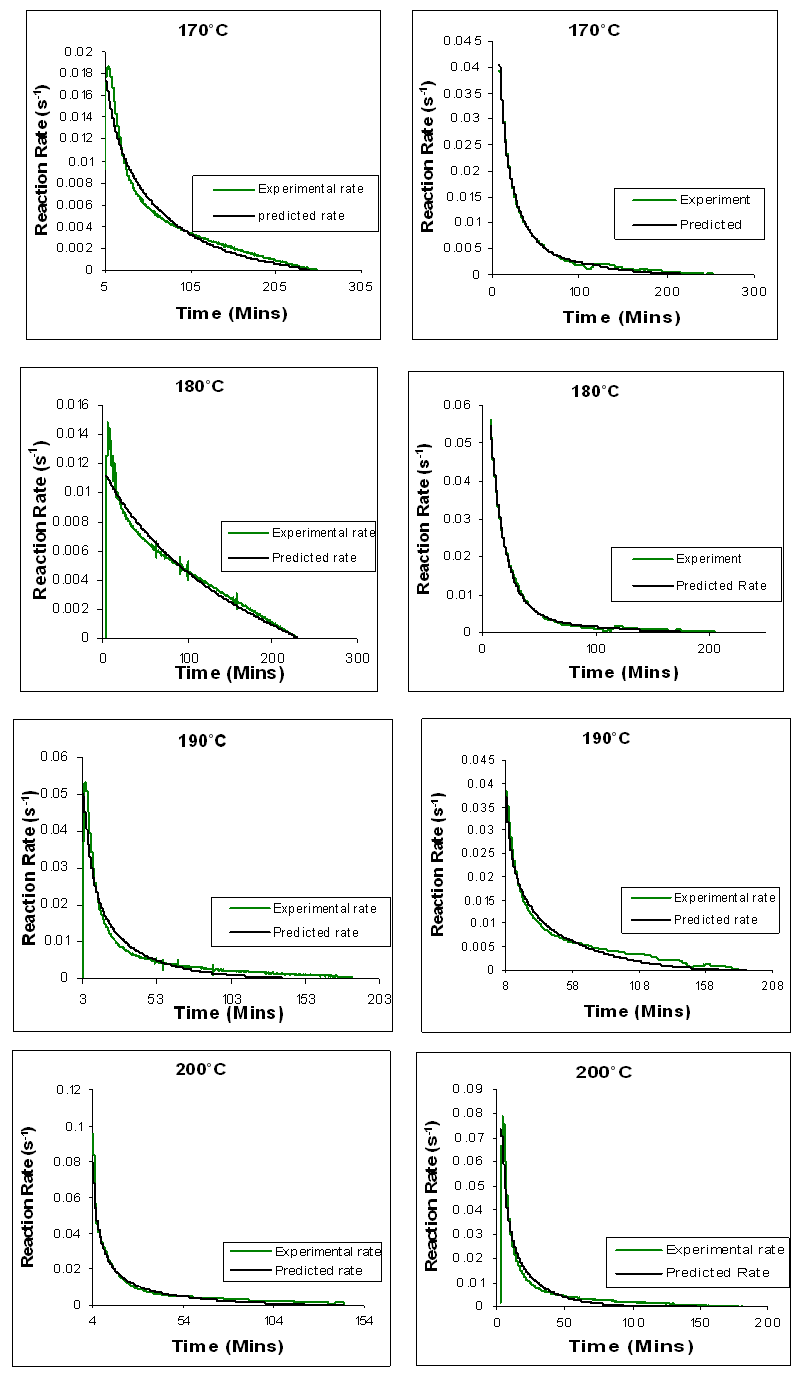 | Figure 21. Comparison between time dependence of experimental reaction rate curves and the curves predicted by means of autocatalytic adjustment for conventional heating of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 on the left, and microwave heating on the right |
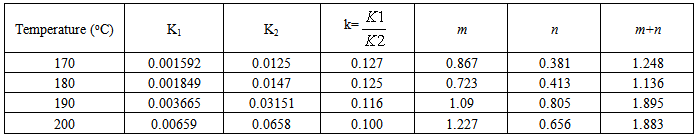
From figures 20 and 21, we observe that the result of the mathematical simulations compare well with experimental results. There are good agreements between the experimental and the model results, proving that the autocatalytic model is able to predict the curing path of the epoxy system.The kinetic parameters obtained from fitting the autocatalytic model with the experimental reaction rate are tabulated in tables 1 to 4 below. Table 1. Kinetic parameters for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine epoxy ratio of 0.85 using conventional heating
 |
| |
|
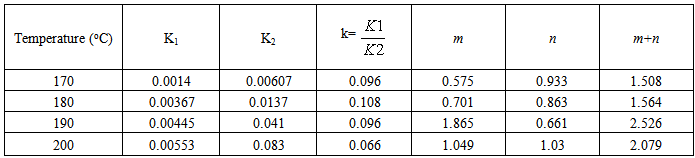
Table 2. Kinetic parameters for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 0.85 using microwave heating
 |
| |
|
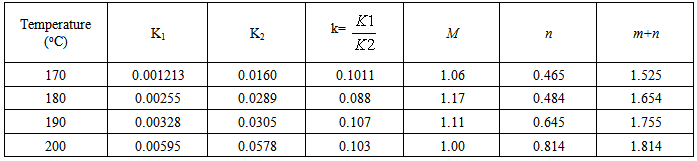
Table 3. Kinetic parameters for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 using conventional heating
 |
| |
|
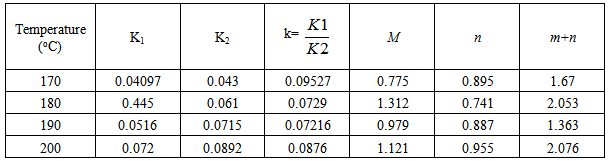
Table 4. Kinetic parameters for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 using microwave heating
 |
| |
|
Tables 1 to 4 display the kinetic parameters obtained for the isothermal curing of both the microwave and the conventional curing of Araldite LY 5052 / 4 4’ DDS and Araldite DLS 772 / 4 4’DDS epoxy systems. It is observed that K1 and K2 values increased with increasing temperature. The absolute values of K1 and K2 are greater in microwave cure than the values of K1 and K2 obtained from conventional cure. For all the systems, the K2 values are much larger than the K1 values. The ratio of K1 / K2 was larger in conventional curing than in microwave curing. Miyalovic et al attributed the lower values of K1 / K2 in the microwave cure to the enhancement of the catalytic reaction of the epoxy system more than the non catalytic reaction by microwave radiation [19]. This phenomenon was explained by the high activity of the [OH] group in the microwave field.The reaction orders m and n are independent of temperature for both conventional and microwave heating. However, for all the epoxy systems, the microwave cured samples have a higher reaction order (m+n) than conventionally cured samples. This means that the microwave cured samples have a higher reaction rate [16]The values of k = K1 / K2 for both conventional and microwave cured samples were less than unity for both Araldite LY 5052 / 4 4 DDS and Araldite DLS 772 / 4 4’ DDS epoxy systems. This is an indication that both systems were characterized by a negative substitution effect. There was a higher rate of reaction of the primary amine with the epoxy group compared to the reaction of the secondary amine with the epoxy group [20].Plots of rate constant (K1 and K2) against temperature for both microwave and conventional curing of both epoxy systems are displayed in figures 22 to 25. Activation energy values obtained are tabulated in table 5.Table 5. K1 and K2 activation energy values obtained from figures for both conventional and microwave heating for both Araldite LY 5052 / 4 4’ DDS and Araldite DLS 772 / 4 4’ DDS epoxy systems
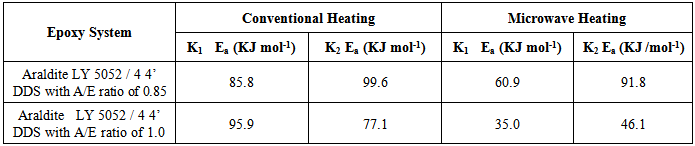 |
| |
|
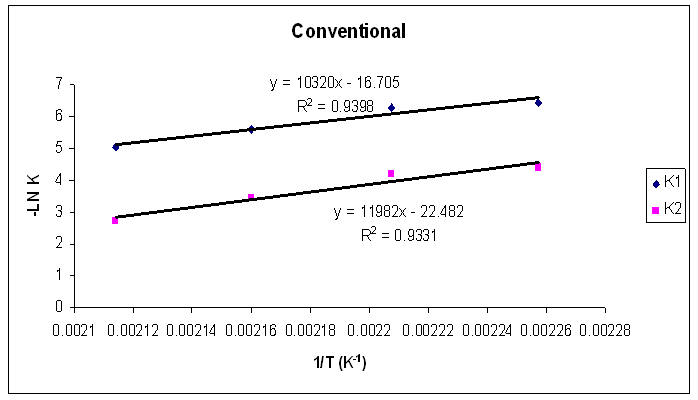 | Figure 22. Rate constants of curing reaction against temperature for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 0.85 under conventional curing |
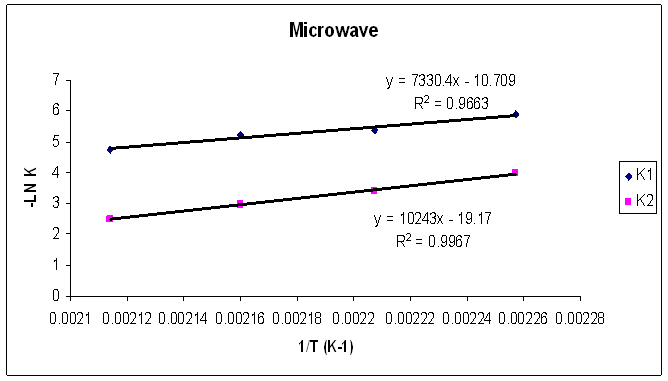 | Figure 23. Rate constants of curing reaction against temperature for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 0.85 under microwave curing |
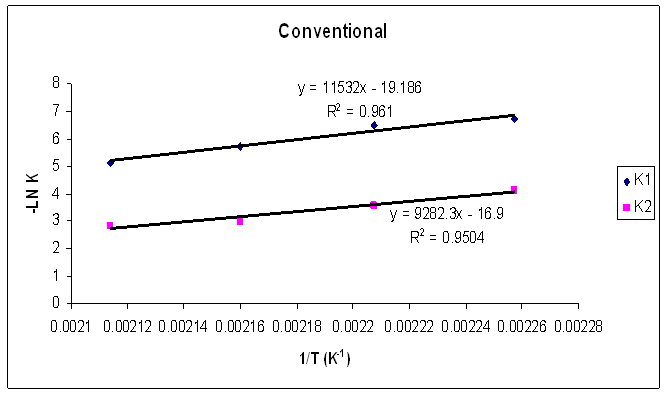 | Figure 24. Rate constants of curing reaction against temperature for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 under conventional curing |
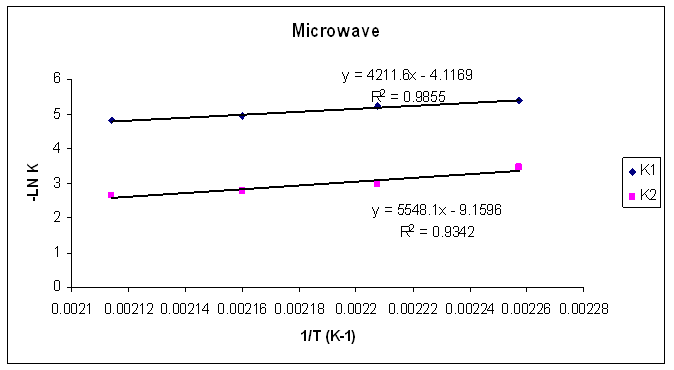 | Figure 25. Rate constants of curing reaction against temperature for isothermal cure of Araldite LY 5052 / 4 4’ DDS epoxy system with an amine / epoxy ratio of 1.0 under microwave curing |
4. Conclusions
The results of the experiments show that for isothermal curing, there was a significant increase in the fractional conversion of the microwave cured samples compared to the conventionally cured samples. The curing reactions for the microwave cured samples took place over a smaller temperature range; and Higher reaction rates were observed in the samples cured using microwave heating.Also, higher K1 and K2 kinetic parameters were observed in microwave cured samples. A lower K1 / K2 ratio was observed in microwave curing than in conventional curing. This is attributed to the enhancement of the catalytic reaction over the non-catalytic reaction by the microwave radiation which occurs as a result of the high activity of the [OH] group.
References
| [1] | http://www.azom.com/details.asp?ArticleID=1098. [cited 2010 October 06]. |
| [2] | Boey, F.Y.C. and B.H. Yap, Microwave curing of an epoxy-amine system: effect of curing agent on the glass-transition temperature. Polymer testing, 2001. 20(8): p. 837-845. |
| [3] | Ayats, J.C., MICROWAVE CURING OF A. |
| [4] | Metaxas, A.C. and R.J. Meredith, Industrial Microwave Heating. 1988: Peter Peregrinus Ltd. |
| [5] | Salla, J.M. and X. Ramis, Comparative study of the cure kinetics of an unsaturated polyester resin using different procedures. Polymer Engineering and Science, 1996. 36(6): p. 835-851. |
| [6] | Halley, P.J. and M.E. Mackay, Chemorheology of thermosets-an overview. Polymer Engineering and Science, 1996. 36(5): p. 593-609. |
| [7] | Karkanas, P.I., I.K. Partridge, and D. Attwood, Modelling the cure of a commercial epoxy resin for applications in resin transfer moulding. Polymer International, 1996. 41(2): p. 183-191. |
| [8] | Kenny, J.M., Determination of autocatalytic kinetic model parameters describing thermoset cure. Journal of Applied Polymer Science, 1994. 51(4): p. 761-764. |
| [9] | Ivankovi, M. cacute, and N. zcaron, DSC study on simultaneous interpenetrating polymer network formation of epoxy resin and unsaturated polyester. Journal of Applied Polymer Science, 2002. 83(12): p. 2689-2698. |
| [10] | de la Caba, K., et al., Comparative study by DSC and FTIR techniques of an unsaturated polyester resin cured at different temperatures. Polymer International, 1998. 45(4): p. 333-338. |
| [11] | Vilas, J.L., et al., Unsaturated polyester resins cure: Kinetic, rheologic, and mechanical dynamical analysis. I. Cure kinetics by DSC and TSR. Journal of Applied Polymer Science, 2001. 79(3): p. 447-457. |
| [12] | Zhou, S. and M.C. Hawley, A study of microwave reaction rate enhancement effect in adhesive bonding of polymers and composites. Composite Structures, 2003. 61(4): p. 303-309. |
| [13] | Sarrionandia, M., et al., Analysis of kinetic parameters of an urethane-acrylate resin for pultrusion process. Journal of Applied Polymer Science, 2000. 77(2): p. 355-362. |
| [14] | Stutz, H., Lifetime assessment of epoxies by the kinetics of thermal degradation. Journal of Applied Polymer Science, 2004. 91(3): p. 1881-1886. |
| [15] | Berglund, L. and J. Kenny, Processing science for high performance thermoset composites. Sampe Journal, 1991. 27: p. 27-37. |
| [16] | Costa, M., L. Pardini, and M. Rezende, Influence of Aromatic Hardeners in the cure kinetics. Materials Research, 2005. 8: p. 65-70. |
| [17] | Marand, E., K.R. Baker, and J.D. Graybeal, Comparison of reaction mechanisms of epoxy resins undergoing thermal and microwave cure from in situ measurements of microwave dielectric properties and infrared spectroscopy. Macromolecules, 1992. 25(8): p. 2243-2252. |
| [18] | Wei, J., M.C. Hawley, and M.T. Demeuse, Kinetics modeling and time-temperature-transformation diagram of microwave and thermal cure of epoxy resins. Polymer Engineering and Science, 1995. 35(6): p. 461-470. |
| [19] | Mijovic, J. and J. Wijaya, Comparative calorimetric study of epoxy cure by microwave vs thermal energy. Macromolecules, 1990. 23(15): p. 3671-3674. |
| [20] | Hill, D.J.T., G.A. George, and D.G. Rogers, A systematic study of the microwave and thermal cure kinetics of the DGEBA/DDS and DGEBA/DDM epoxy-amine resin systems. Polymers for Advanced Technologies, 2002. 13(5): p. 353-362. |
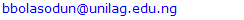


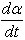 at time t [15]. This can be determined by the following expressions.
at time t [15]. This can be determined by the following expressions.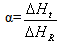
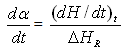
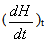 is the rate of heat generation and is directly related to the calorimetric signal at time t, and ΔHR is the total reaction heat associated with the complete conversion of all the reactive groups , and ΔHt is the heat released until time t. This can be obtained directly by integrating the calorimetric signal
is the rate of heat generation and is directly related to the calorimetric signal at time t, and ΔHR is the total reaction heat associated with the complete conversion of all the reactive groups , and ΔHt is the heat released until time t. This can be obtained directly by integrating the calorimetric signal 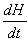 until the time t [15].
until the time t [15].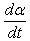 can be expressed as [5]:
can be expressed as [5]: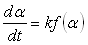
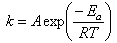
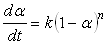
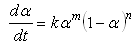
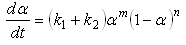
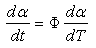















 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML











