1. Introduction

In the quantum information and computation theory, quantum entanglement, a non-classical correlation, is the key resource and a most remarkable feature. Quantum entanglement was first introduced by Einstein, Podolsky and Rosen (EPR)[1] and also Schrödinger[2]. There was a question in[1] by EPR, whether quantum mechanics is local and complete theory or not? In this context, Bell[3] has given a very significant result : the well known Bell’s Inequality and the consequent features of quantum mechanics are usually called non-local theory. It is accepted that quantum entanglement is responsible for the non-locality of quantum mechanics and the performances of so many information theoretic tasks like Teleportation, Dense coding, Cloning and many others[4-6]. Hence characterization and quantification of quantum entanglement are the most important tasks of quantum information and computation theory.

But there exist non-classical correlations other than entanglement for a composite systems. Quantum entanglement is quantifiable. Practical application of it demands quantification. Von-Neumann Entropy, entropy of entanglement for pure state entanglement[7], Entanglement of formation, Distillability[8,9,10,11,12,13,14], and Concurrence[15,16] are the very useful measures of quantum entanglement – a non-classical correlation.

Now the most popular measure introduced by Olliver and Zurek[17] and separately by Hendersen and Vedral[18] is quantum discord- which is much better than the other measures and can find out the non-classical correlations even in separable states. Quantum discord brought as an information theoretic measure of the ‘quantumness’ of correlations[19] and is used to determine some results in thermodynamics[20]. Characterizing correlations in terms of its quantum discord, it is proved that classical correlations leads to completely positive reduced dynamics and the induced maps can be completely non-positive when quantum correlations is present[21] and completely positive(CP) maps arise exclusively from the class of separable states with vanishing quantum discord[22]. Use of quantum discord for the characterization of correlations present in the quantum computational model DQC1, introduced by Knill and Laflamme reveals that non-zero values of discord indicates non-classical correlations whenever there is no entanglement between the two parts[23]. A large amount of discord is found but no entanglement in the experiment by the implementation of DQC1 in an all-optical architecture[24]. Also in the DQC1 model, it is proved that a non-zero quantum discord implies a non-zero shift under locally non-effective unitary operations(LNUs)[25]. In the dissipative dynamics of two-qubit quantum discord under Markovian environments, comparison of the dynamics of entanglement with that of quantum discord was made and shown that the entanglement suddenly disappears in all cases where quantum discord vanishes only in the asymptotic limit as the individual decoherence of the qubits, also in finite temperature. Which concludes that quantum discord is more robust than the entanglement against decoherence so that quantum algorithms depending on the correlation ‘quantum discord’ may be more robust than those based on quantum entanglement[26]. Study of quantum discord for two-qubit states gives that for separable states, the entanglement of formation always vanishes but discord does not vanish implies the superiority of quantum discord[27].For finding the monotonic nature of quantum discord ,a few discussion on incomparability and majorization is required. The monotonic nature of quantum discord through incomparability is also observed here.But our present discussion, we are concentrating in the difficulties in the quantification of quantum discord.

2. Concept of Quantum Discord

Now we know that a bipartite quantum state has both classical and quantum correlations. An information theoretic measure of a bipartite quantum state is ‘quantum mutual information’.

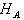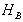I. Quantum mutual information : Let the two parts are A and B and their corresponding Hilbert spaces are and respectively. We consider a density operator 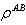in 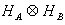of the composite bipartite system AB, and 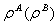the density operators of part A(B) respectively, then the quantum mutual information is defined as:

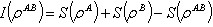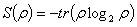where is the von Neumann entropy.

Mutual information is the maximum amount of information that A can securely send to B if a composite correlated quantum state is used as the key for a one-time pad cryptographic system[28]. Quantum mutual information is the sum of classical correlation 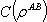and quantum correlation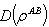, that is,

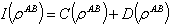This quantum part 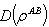is called quantum discord.

II.Quantum discord:

Now, the mutual information may be written as 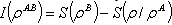where 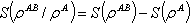denotes quantum conditional entropy.

Let the projection operators 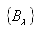represents a von Neumann measurement for subsystem B only, then the conditional density operator 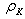associated with the measurement result K is

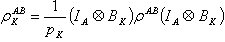where the probability

.

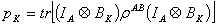Then the quantum conditional entropy with respect to this measurement is given by

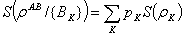And the associated quantum mutual information of this measurement is defined as

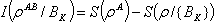Classical correlation is given by[17,18,27,29]

.

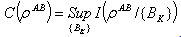Calculating 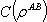is difficult because it can be obtained by taking maximum over all possible measurement of B. If however, we can find 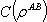then quantum discord is found by.

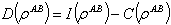Review of incomparability under deterministic LOCC

Entanglement transformation is a very fundamental problem in quantum information. Here we deal with the question that, if be a pure bipartite state then is it possible to transform 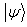to another state 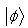by using LOCC?

I.Concept of Majorization:

Majorization[4] resolves the question. Let 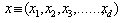and 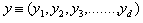are real d-dimensional vectors. Then x is majorized by y (equivalently y majorizes x), written as 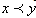, if for each k in the range 1,2,3,….,d,

,

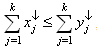Where equality holds for k=d, and where the 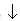indicates that the components are in decreasing order. Let be the state of the first obtained by taking trace on second party and 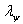be the vector of eigen values of 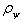. Then

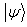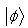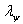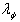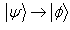Theorem[5]: transforms to using LOCC if and only if is majorized by or iff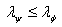 where 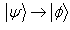indicates that 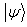transforms to .

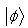II. Incomparability:

If 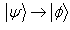is not possible with probability one under LOCC then we denote this by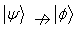. But it may possible that 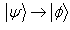under LOCC with probability one. If for a pair of pure bipartite state (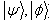, 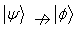and 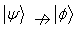both happens then we call (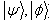as a pair of incomparable states.

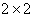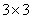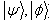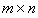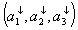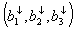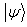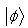In systems, there do not exist incomparable pair of states. But in system incomparable pair of states exist. For the criterion of incomparability for a pair of pure entangled states of systems where min{m,n}=3, we have the following way. Let and are the Schmidt vectors corresponding to the states and respectively and

.

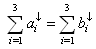Then it can be obtained from Nielsen’s criterion that 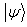and 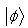are incomparable if and only if either 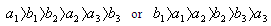. All the above studies is for the deterministic transformation.

3. Analitical Approach in Quantification Procedure of Discord

It is briefly discussed and completely explained[30] that for two-qubit X-states quantum discord can be found. The method applied for finding quantum discord has required the use of the von-Neuman measurements for the subsystem B as

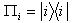where is the projector for the subsystem B for the basis 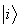and .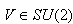

I.von-Neuman measurements in

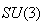Here we are emphasizing on a bipartite three-qubit system. So here the von-Neuman measurement for the subsystem B is B_i = VП_iV^†, i =0,1,2.

Where 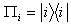is the projector for the subsystem B for the basis 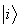and .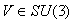

Any element in 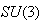can be expressed as

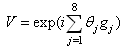Where 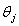are real numbers and

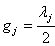where 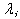’s are the Gell-Mann matrices.

We see that

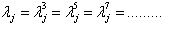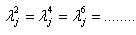and , for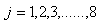

These yields the expression of V in[30] as

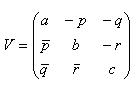Where

and

.

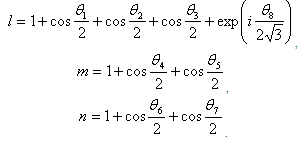Then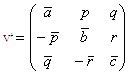

Now von-Neumann measurement for subsystem B are

B_i= VП_iV^† , i=0,1,2.

Where .

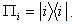B₀, B₁, B₂ are expressed as

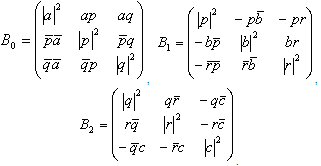II. Quantification of Discord:

Let us consider an example to clarify such concept for bipartite three-qubit system by taking an arbitrary state

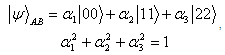Then

and the expression for 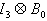is found as

So for calculating the ensemble for the state 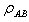, we know that

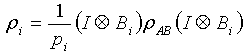and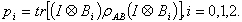

Here we get the

.

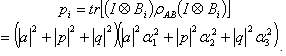Hence the eigen values of 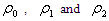, are 1,0,0,0,0,0,0,0,0.These gives

.

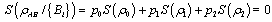The classical correlation coefficient becomes

.

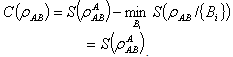So the quantum discord

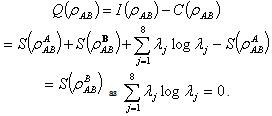For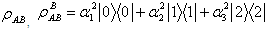

yields

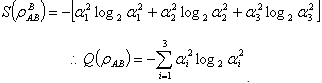And so for bipartite qudit systems, we have

,

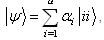we get

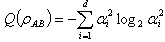Which is von-Neumann Entropy of the reduced system of .

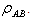Monotonicity of Quantum discord under deterministic incomparability

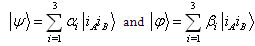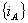In this section our attempt to observe the monotonic nature of quantum discord under deterministic incomparability LOCC. For this consider where and 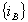are the orthogonal basis of the respective Hilbert spaces 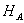and 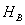. Now the observations on the analytic expression of quantum discord it is really established the fact Discord 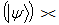Discord 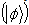according to the numerical values of 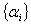and 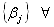i=1,2,3. So in general we have no such stick monotonic nature of the quantum discord of the two incomparable pairs.

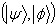4. Conclusions

Quantum discord is more powerful feature for realizing the non-locality aspect of quantum mechanics than quantum entanglement because some separable states have non-zero quantum discord. In this paper our aim is to find out the mathematical difficulties in the calculating procedure of Quantum discord. We observe that even in 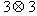, the large expressions of elements of the matrix are really hard to handle. So it obstacles us for finding the eigen values of the matrices. The next big problem is due to the optimization occurred in the expression of the Quantum discord. So finding the general expression of Quantum discord in this above mathematical process is really a great challenge to the people. Though many tight bounds have been discovered and theoretical works have been done the analytic expression for this discord in higher dimension is not yet found.