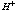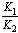-
Paper Information
- Paper Submission
-
Journal Information
- About This Journal
- Editorial Board
- Current Issue
- Archive
- Author Guidelines
- Contact Us
Food and Public Health
p-ISSN: 2162-9412 e-ISSN: 2162-8440
2012; 2(3): 65-68
doi:10.5923/j.fph.20120203.01
Molal Solubility, Dissociation, Association and Solvation Parameters for Saturated O -Chlorobenzoic Acid Solutions in Various Solvents at 298.15 K
Esam A. Gomaa
Chemistry Department, Faculty of Science, Mansoura University, Mansoura, 35516, Egypt
Correspondence to: Esam A. Gomaa, Chemistry Department, Faculty of Science, Mansoura University, Mansoura, 35516, Egypt.
| Email: |  |
Copyright © 2012 Scientific & Academic Publishing. All Rights Reserved.
The molal solubility for saturated solutions of O -chlorobenzoic acid at 298.15 K in various solvents was determined. The solvents used are, water (W), ethanol (Et), dimethylsulphoxide (DMSO), acetonitrile (AN), methanol (Me), 1-4, dioxane (Di) and N , N-dimethylformamide (DMF).From the experimental data for solubility, pH , densities, the different volumes, free energies, dissociation constants, association constants and solvation parameters were estimated. Also the free energies of dissociation and association were also evaluated. Other solvation parameters like the solvation numbers were cited here to help explaining the solubility trend.This work give a lot of data for the solubility of orthochlorobenzoic acid which help the biologist for using it as food preserver .The results were also discussed.
Keywords: Keywords Solubility, Dissociation Constants, Association Constants, Different Volumes, Solvation Parameters, Free Energies ,Orthochlorobenzoic Acid
Cite this paper: Esam A. Gomaa, Molal Solubility, Dissociation, Association and Solvation Parameters for Saturated O -Chlorobenzoic Acid Solutions in Various Solvents at 298.15 K, Food and Public Health, Vol. 2 No. 3, 2012, pp. 65-68. doi: 10.5923/j.fph.20120203.01.
1. Introduction
- The solubility of an electrolyte is influenced by a wide range of factors , including ion association ,variation in ionic activity coefficients , complexation and temperature. Solubility is an equilibrium property enable to thermodynamic parameters through the standard state free energy. Ion pairing can occur in dilute solutions for many electrolytes, particularly these with multivalent ions and for all electrolytes in concentrated solutions. Ion pairing is generally more pronounced in non-aqueous solvents which have lower dielectric constants than water. In effect, the ion pairs represent a reservoir of electrolyte in the solution and increase the solubility.The complexityof the system increases for unsymmetrical electrolytes or in mixed electrolyte systems[1].Bjerrum[2] proposed that the motion of ions would be coupled when the energy of attraction between them exceeded the thermal energy. For solely columbic interactions his theory predicts a distance within which the electrostatic attraction between ions is greater than 2kT. Which will be sufficient to couple the motions of the ions. The treatment takes account of only electrostatic interactions and neglects the molecularity of solvent, Nevertheless in low concentration interactions between ions and solvent molecules resulting in ion pair formation. The three commonly assumed structures are the first in which the ion retains their Individual solvation shells, and so is separated by two solvent molecules. The second in which the ions share some part of their solvation shells so are separated by one molecule and the third where the ions are in contact and share a common solvation shell.The presence of species such creates an experimental difficulty; the different techniques will have different sensitivities to the species present. Thus the conductance will see on the dissociated ions and the presence of ion pairs is determined by difference from experimental molar conductance and that expected for strong electrolyte[3].The formation of complexes (complexation) provides a route to increased solubility. Several equivalent representations of the speciation in these systems have been used[4].Many publications have appeared on the behaviour of weak acids in anhydrous solvents. Interesting work has been done by Kolthoff et al.[5,6]. Aleksandrov et al.[7,8] studied the dissociation of salicylic acid in butane-2-one. Kreshkov et al.[9] studied the dissociation of amino acids (as weak) acids) in mixtures of formic and ethylmethylketone and in mixtures of acetic acid-ethylmethylketone. Gomaa et al.[10] studied association, dissociation and hydrogen bonding of salicylic acid in water-N,N- dimethylformamide mixtures from solubility measurements.The aim of this work is to evaluate the solubilities of O-chlorobenzoic acid in different solvents and discuss in detail the solvation parameters for the solubility process for O-chloro benzoic acid which is one of the most widely used preservation materials .O-chloro benzoic acid has the advantage of low cost, ease of in- corporation into products. Lack of colour and relatively low toxicity.Also knowing the other factors affecting the solubility is very important here. Is the electrostatic energy play important role in the solubility or not.Many authors like Bjerrum and other reported that the electrostatic energy plays important role in the solvation energy. In this work more effort was done to explain the main factors affecting the solubility of orthochlorobenzoic acid in different solvents[11]
2. Experimental
- The O-chlorobenzoic acid 98 % m.p type Cambarian Chemicals was used. The solvents, ethanol (ET), dimethylsulphoxide (DMSO), acetonitrile (AN), methanol (Me), 1-4 dioxane (Di) and dimethylformamide (DMF) were supplied from Merck. The saturated solutions of O-chlorobenzoic acid were prepared by dissolving it in the solvents used. The solutions were saturated with N2 gas in closed test tubes. The tubes were placed in a shaking water bath of the type assistant for a period of four days, followed by another two days without shaking to reach the necessary equilibrium. The solubility of O-chlorobenzoic acid in each solution was determined gravimetrically by taking 1 ml of the saturated solution and subjecting it to complete evaporation using small aluminium disks heated by an infrared lamp. The pH readings of the saturated solutions were measured using a pH-meter of the type Tacussel/Minis 5000. The densities were measured by using weighing bottle 1 ml and analytical balance (4 digits) of the type Mettler Toledo DA.
3. Results and Discussion
- Determination of the dissociation constants of organic acids in aqueous and non aqueous solutions by different methods like condutometric method during a period of 100 years was discussed. From time of pioneering works of Arrhenius and Ostwald the conductometric method provide simple method for the determination of the dissociation constants of organic solvents in water. This method is based on the assumption that strong electrolytes are completely dissociated while weak electrolytes attain this state only at infinite dilution. At fixed temperature, an equilibrium exists in solutions of weak electrolytes between ions and undissocited molecules to which the law of mass action and the Ostwald dilution law can be applied. Therefore complete study need more work for this field which is the target of this work.The calculated molal solubilities (m) for O-chlorobenzoic acid saturated solutions were given in Table (1) from at least three average measurements. Also the measured densities and pH values of the acid used are also listed in Table (1).The molar volumes (VM) of benzoic acid were obtained by dividing the molar mass by the densities and their values are listed in Table (2). The packing density as reported by Kim et al.[11] and Gomaa et al.[12], i.e. the relation between Van der Waals volume (VW) and the molar volume (VM) of relatively large molecules (above 40) was found to be a constant value and equal to 0.661.
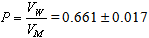 | (1) |
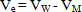 | (2) |
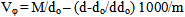 | (3) |
|
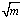 [17].Kass values were calculated[18] from the ratios of association constant to dissociation constant (i.e., K1/K2) for the dimers of O-chlorobenzoic acid which form a complex ion (
[17].Kass values were calculated[18] from the ratios of association constant to dissociation constant (i.e., K1/K2) for the dimers of O-chlorobenzoic acid which form a complex ion (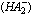 and hydrogen ion (H+), and the values of K` (where K` is the dissociation constant of the associated acid complex, H2A2) are given by the following equations
and hydrogen ion (H+), and the values of K` (where K` is the dissociation constant of the associated acid complex, H2A2) are given by the following equations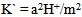 | (4) |
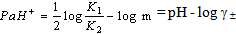 | (5) |
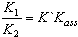 | (6) |
|
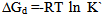 | (7) |
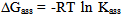 | (8) |
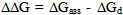 | (9) |
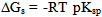 | (10) |
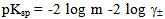 | (11) |
|
|
References
| [1] | Apelblat , Alexander, .J. Molecular Liquids,95(2002)99-145. |
| [2] | Barthel,J ,Wachter,R. and Gores,H.-J., inModern Aspects of Electrochemistry,Coway ,B.E., and Bockris,J.O`M, Editors,Vol.13,pp.1- 179. Plenum Publ.Corp., New York, 1979. |
| [3] | Barthel,J.M.G.,Krienke,H. and Kunz,W.,”Physical Chemistry of Electrolyte Solutions , Modern Aspects”., Springer,Darmstadt,1998. |
| [4] | Bockris,J.O`M . and Reddy, A.K.N.,” Modern Electrochemistry”, Plenum Press,New York,1970. |
| [5] | Kolthoff, I.M. and Chantooni, M.K., J. Am. Chem. Soc., 87(1965).4428. |
| [6] | Kolthoff, I.M., Chantooni, M.K. and Bhownik, S., J. Am. Chem. Soc., 90(1968) 123. |
| [7] | Aleksandrov, V.V., Zudochkina, A.I., and Sandovinchaya, L.P., Zh. Fiz. Khim., 52(1978) 1295 . |
| [8] | Alekandrov, V.V., and Burakhovich, Fizicheskaya Khimiya Rastrorov (Physical Chemistry in Solutions), Nauka, Moscow, 1972, p. 154. |
| [9] | Kreshkov, A.P., Tanganor, B.B., Yarovenko, A.N. and Batoreva, T. Kh., Zh. Fiz. Khim., 54(1980) 105. |
| [10] | Gomaa, E.A., Mousa, M.A. and El-Khouly, A.A., Thermochimica Acta, 89(1985) 133-139. |
| [11] | Kim, J.T., Cecal, A., Born, H.J. and Gomaa, E.A., Z. Physikalische Chemic, Neue Folge, 110(1978) 209-227. |
| [12] | El-Khouly, A.A., Gomaa, E.A. and Abou-El-Leef, S., Bull. Of Electrochemistry, 19(2003) 153-164. |
| [13] | El-Khouly, A.A., Gomaa, E.A., Abou El-Leef, S., Bull. Of electrochemistry, 19(2003) 193-202. |
| [14] | Oswal, S.L., Desai, J.S., Ijardar, S.P. and Jain, D.M., journal of Mol. Liquids, 144(2009) 108-114 . |
| [15] | Dorota Bobicz, Waclaw Grzybkowski and Andrzej Lwandowski, J. of Mol. Liquids, 105(2003).93-104. |
| [16] | Marcus, Y. “The properties of solvents”, Wiley, London, 1998. |
| [17] | Moelwyn-Hughes, E.A., “Physikalische Chemie”, George Thieme Verlag, Stuttgart, 1970, p. 489. |
| [18] | Gomaa, E.A., Mousa, M.A. and El-Khouly, Thermochim. Acta, 89(1985).133-139. |
| [19] | Gomaa, Esam, A., Thermochim. Acta, 120(1987).183-190. |
| [20] | Bondi, A., J. Phys. Chem., 68 (1964) 441. |
| [21] | Gomaa,E.A and Al-Jahdalli,B.M.,American Journal of Condensed Matter Physics,2(2012).16-21 . |
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML