1. Introduction

The Angolan reality proposes an increasing need to improve the quality of mathematics training from a basic to higher education level, as an alternative to strengthen the careers that will boost the social and economical development.

In this sense, the contextualization problems, the logical interpretation of the mathematical contents and its application in solving concrete problems of life are currently topics of high reflection and methodological discussion.

It has been observed in the high school as well as in the higher pedagogical institutes where mathematics is studied in Angola a reduced number of students.

There is therefore, a need to enhance the process of teaching and learning of mathematics, by using active methodologies, a system of procedures and technological resources aimed at achieving higher levels of contents contextualization and motivation of students from the initial stages of mathematical training to higher levels.

However, teachers who must fulfill this task do not have suitable training, from a teaching, pedagogical and technologically standpoint. The mathematical communication styles, methods and teaching aids used in class do not take into account the participation of students in the interpretation of the results, and its application in solving specific problems of life.

These demands, coupled with the rapid scientific and technological development currently happening in the world, constantly raise a number of problems that occur both in the schools and in everyday life.

Therefore, teaching that stimulates academic activities of students and develops logical and interpretive thought by introducing educational models to visualize the significance of the emerging results of solving mathematical problems, with higher levels of contextualization, would help students to observe the mathematical reality of their environments.

This would equip them to face new challenges as well as interpret the multiple problems for which they must find solutions, [1] and [2].

In addition taking into account several factors: the level of the logical and interpretative development of each of the students before the need to address specific situations of the life; the previous knowledge that would serve as the basis to assimilate new contents; the guidance provided by the teacher to improve the interpretation procedures, as well as the degree of significance it may have for students, principally on their professional training.

2. Aim of the Paper

In the high school and university process of mathematics training in Angola, manifest shortcomings in the logical reasoning required to solve mathematical problems in correspondence with the needs of practical application; insistent use of traditional methods of teaching and learning, low students’ motivation in mathematics careers; limited contextualization of the contents, as well as a limited use of software in the interpretation of the results, in the graphic representation, among other applications.

The above aspects clearly show the need to improve the quality of mathematics training in these education levels.

In this context, teachers and researchers in mathematical areas have as universal problem, to find ways to ensure the new generations face challenges and solve the many problems that have to find solutions [3-5].

Our prospective is to construct knowledge that can be translated into strategies to improve the dynamic of mathematical training in Angola; increase the level of contents contextualization as well as students’ motivation to study mathematics careers.

The aforementioned arguments allowed us to assume that the aim of this paper is to approach the Angolan context of mathematical training, using criteria issued by teachers, high school and university students surrounding the applied questionnaires.

To fulfill the stated aim is necessary to note that there are different factors to consider in the development of logical reasoning, but authors like: [6-8] consider that if the cognitive structures are not prepared to incorporate new content, it is difficult to give to the students, tools that allow them to develop their logical thought.

This thought form constitutes a fundamental premise, especially if we consider that mathematics is a sequential science, the understanding of content requires furthering bases related to previous content, which contributes to students’ motivation in the appropriation of new content.

Therefore, professor not always understands that their role has changed; their role is to teach students to develop their logical reasoning.

For these reasons is important to characterize the level of responsibility of the teacher in preparing the necessary cognitive structures of the students to introduce new contents, [9].

3. Didactic Aspects to Raise the Level of the Students' Motivation in the Mathematics Careers

At all educational levels the mathematics learning of students is a task that presents many difficulties. Reason about this, teachers are called to create new methodological alternatives to motivate their students to study this science, [10] and [11].

With the mentioned alternatives the student will be willing to learn mathematics, whenever the teachers reveal its meaning in the solution of concrete problems of the life and profession.

Considering the mathematics learning as a process heavily influenced by logical reasoning, from the practical point of view it makes a teacher a tireless researcher of the alternative mechanisms that enable to attract the students to the study of the mathematical contents.

In this case, the teaching and learning should become an active process, constructive and facing the constant motivation of the students to the contents that makes part of their career. [12], [13], [24], [25], [26] and [31].

Therefore the teacher as facilitator should select methods, procedures, techniques and teaching aids that enable the student to feel like an active part of the teaching and learning process, aspect that contribute to raise the students’ motivation to the activities of their professional formation.

According to authors like [14-16] in the teaching and learning of mathematics, is necessary that the teacher has clear that this process involve research, reasoning, communication, contextual knowledge as well as a correct interpretation of the results.

Teachers have also to understand that the dynamics of mathematics training has changed significantly with the development of science and modern technology, [17].

In this sense is necessary to persist in the contextualization processes of contents, exercises and problems addressed by the appropriate use of software to help simulate concrete situations of the life, [18] and [19].

The previous considerations help to reveal the interdisciplinary studies as a balancing of the teaching and learning process whenever the contextualization of content is achieved.

We agree with [20], to substantiate the need for the teachers to know the subject they teach, as well as to make available the mathematical tools to the students to model and analyze typical phenomena of their specialty and other branches of the science.

Another important aspect to consider is the deep knowledge of students’ personality, their aspirations, goals, and limitations. The teacher also have to know the problems surrounding each of the students, their family customs and traditions, beliefs, fears and interests with respect to mathematics.

Therefore to increase the levels of students’ motivation towards studying mathematics careers, teachers have the task of clarifying students the enormous potential that science offers in the human and social development, in the economical development of the country, in the national security, in the development of modern technologies, among other potentialities.

4. Economic Reality and Proposal for Improving the Process of Angolan Mathematical Training

The Angolan economy is changing as the knowledge replaces physical capital as a source of current and future wealth. This process is being propelled by petroleum production, diamonds extraction, among other natural resources.

However, the social and economical crisis that affects the world, the rapid scientific and technological advances that characterize the twenty-first century, where we highlighted the areas of informatics, biotechnology, microelectronics, astrology, pharmaceutical sciences and other educational innovations, prompt the need to change our way of life, of work, our economical production and social thought.

Hence, there is a need to strengthen mathematical training that is offered at all levels of education, whether in public or private institutions.

This will enable the students to develop a way of thinking that allows them to apply previous knowledge in solving specific situations, current and future, and to understand the role that mathematics plays in the development process of human, social and economic thinking.

Nowadays, the less motivation of the high school and university students to study mathematics careers is worrying in Angola.

The majority of students resort to careers related to economics, informatics, agriculture, civil engineering and hydraulics, given the current need for food production, construction and reconstruction of new schools, hospitals, banks, airports, bridges, roads, among others social public works.

However, the limited focus of the potential training of mathematics, as a main support of the engineering careers, constitutes another barrier faced by students due to insufficient professional guidance.

On the other hand, the insufficient mathematics teachers that the country has, demonstrate little interest in the contextualization of contents, exercises, problems, as well as in its interpretation process.

The former requires not only the ability to mobilize and coordinate theoretical knowledge but also the ability to handle specific situations of life, skills that can be progressively developed from the basic stages to a professional training.

The question that is raised in the dynamics of mathematical training in Angola is to know if the degree in Mathematics is simply to increase the number of graduates or if also has to train teachers who can actively contribute to strengthening the basic education, the development of higher education, as well as to the economical development.

There is a need to reduce the high failure rates observed not only in mathematics, but in all those subjects that require the mathematical contents as an indispensable tool for its understanding and development.

This is an old dilemma, stemming from primary school to the university, given the limited practical approach to mathematics and its importance in solving concrete problems of daily and professional life.

For example, when someone uses a phone, a credit card, electronic devices, calculators, computers, surfs the Internet, listens to a CD, watches a DVD, drives a car, takes a plane, boat or train, are they aware that what makes these devices work normally is mathematics?

However, if the responsible institutions for the mathematical training of the younger generation, aimed to raise the levels of contextualization in order to demonstrate the practical application of this science in solving professional problems, are not committed to the issue in question, they in reality contribute to the risk of forming misfit teachers.

Authors such as: [1-9], have made significant contributions aimed at improving the process of mathematical training in the higher education.

In general, they agree on the necessity of strengthening the process of mathematical education through the use of active teaching methods, as well as the development of logical thinking skills through problem solving.

However, the educational demands and social requirements of the rapid scientific and technological development of the twenty-first century reveals inconsistencies in the conception of improving the process of mathematical training.

There is excessive emphasis on solving mathematical problems. It is essential that this process be changed to one that systematically emphasizes the contextualization of the contents, the interpretation of problems and the practical application of the results in solving concrete problems of daily and professional life.

This need is based on the analytical and interpretive limitations of the students in the hour of revealing the practical significance of the results in a particular.

Another limitation observed in the dynamics of mathematical training in Angola, mainly in the high school and higher education, is the insufficient use of mathematical software for graph functions, solve complex problems, test the results, and interpret them among other applications. [17]

This situation limits the appropriate content and visibility of the progress of science and modern technology in the mathematical field.

To modify this situation, Angola needs to increase the training of teachers with master and doctoral degrees to benefit from scientific and technological progresses and motivate a greater proportion of their young people to study math careers.

In line with the issues mentioned above, to further strengthen the process of mathematical training in Angola and the dynamics of the interpretative formation of this science, it is necessary to consider the following:

• The interpretation of mathematical problems and solutions are favored by the wealth of prior knowledge and experience of the subject (student, teacher and researcher).

• The process of interpreting mathematical problems and their solutions cannot be a mechanical work but must be logical, conscious of the results and the impact it can have on the society transformation.

• The conduction of the interpretive formation process in the mathematical field must be centered on the problematization, contextualization, dialogic interaction, as well as the high degree of responsibility for the allocation of new meanings and significance.

To support the mentioned aspects is necessary to consider the formal logic and dialectical logic as one of the constituents of the cognitive system of the students and teachers.

Its recognition is what allows us to establish the basis of logical reasoning, as a premise for the construction not only of mathematical knowledge.

4.1. The Process of Mathematical Training in Angolan Higher Education: Historical Trends and Graduation Structure

Angola is a country located in Southern Africa, bordered to the North by the Republic of Congo; Zambia to the East, Democratic Republic of Congo to the Northeast; Namibia to the South and West by the Atlantic Ocean.

It was a Portuguese colony for nearly five centuries and occupies an area of 1, 246, 700 square kilometers.

Angola has an estimated 24 million inhabitants. It became independent on November 11th, 1975.

After a bloody independence, the country met another period of internal conflict that ended on April 4th, 2002.

Nowadays, it is a multilingual country and the official language, Portuguese, is used in formal learning and teaching.

However, due to the cultural diversity there is a need to encourage the citizens to gain an appreciation of the national dialects.

This strategy is currently being implementing in the school system. The most important dialects that are already been implemented in the educational system in Angola are: Umbundu, Kimbundu, Kikongo, Tchokwe, Ngangela, Kwanhama, Fyote, and Nhaneka.

To reveal the historical trends of the mathematical training process in higher education and the dynamics interpretive training of this science, the behavior of the following indicators were examined:

• Mathematical requirements according to a professional profile.

• Contextualization of mathematical contents, in correspondence with the professional application.

• Interpretation of the results in correspondence with the need of its application.

The analysis of the behavior of the mentioned indicators enabled the authors to reveal three stages in the process of mathematical training in the Angolan higher education and the dynamics of interpretive formation of this science.

Consequently, we considered all aspects related to the dynamics of interpretive training of the mathematical contents and its application in solving social and professional problems, with the implementation of the higher education in 1962.

The transformations that currently verify the dynamics of the mathematics training in the Angolan higher education, whose aims are focused on improving the quality of citizenship education; promote an authentic construction of mathematical knowledge in the diversity and complexity of epistemological and methodological approaches generated on the social, economical and technological needs of development.

4.2. First Stage. Recognition of Mathematical Training in the Higher Education (1978-1988)

The recognition of the importance of professional training, particularly in mathematics, was understood by the educational reforms planned in 1977 and approved in 1978 as a strategic alternative, due to the critical situation of dependency that existed in the field of education.

These reforms were intended, to restructure the Angolan education system; university extension, as well as to understand and to solve problems that affects directly and indirectly the social and economical progress of various regions of the country.

To this end, the fundamental principles to destroy the old traditions inherited from the colonial Portuguese University, characterized by a form of teaching and learning relatively dissociated to the Angolan context, where prevailed high levels of decontextualization of the contents, and a weak mathematical training, a situation that considerably delayed the understanding of the role and significance of mathematics in the process of social and economical development of various regions of the country.

However, since the ends of 1979 to the middle of 1988, the process of mathematical training, has gained new dimensions that have motivated the researchers.

The small university community began to understand the role of the mathematics in the process of social and economical development; in the administrative management; in the optimization of the natural resources; in the national reconstruction as well as in the strengthening process of other careers.

For this purpose, methods and strategies were developed due to the shortage of qualified personnel that did not have full independence for the demand of such time.

As a result of this problem, programs and curricula used clearly reflected typical aspects of the colonial Portuguese University, a situation that dragged for several decades.

Therefore, at this stage, the process of mathematical training in the higher education was deficient compared to other international experiences.

The mathematical contents taught in the various careers, were not contextualized to the professional profile.

The mentioned contents have presented high levels of inaccuracies and limited possibilities of generalization to solve concrete situations of the life.

The interpretation that was attaining from the mathematical problems and its results did not satisfy the needs of practical application to solve specific problems of the life. It has been focused primarily on reproduction and memorization.

4.3. Second Stage. The Role of Mathematical Contextualization in Higher Angolan Education. (1989-2002)

The resolution of the Central Committee of the Angolan Government on the reformulation of the educational system, [27], emphasizes the importance of education as a key to social development and reveals the need to prepare a new citizen as a prerequisite for the social innovation.

The strategies and methodologies used in previous years were reviewed, with an aim to identify deficient areas and the prospect of further refining the process of mathematical training in higher education and dynamic interpretation formation of this science. Substantial changes were made to the plans and programs of study.

However, the changes made to strengthen this process have not yet achieved full satisfaction because they had not taken into consideration the objective and subjective aspects of the hermeneutical process.

The previously disclosed, involves not only an abstract appreciation of the process, exempt of contextualized problems and interpretative results; it is also necessary to put into action the essential relationships between theory, practice and social context.

The entire dynamic of mathematical training needs to emphasize the use of epistemological, methodological and technological approaches that enable students to see mathematics as an attractive science; enjoy their learning potential and to understand the role it plays in the developmental process of human thought and its economic impact.

Therefore, in actuality the process of mathematical studies in higher education and the dynamics of interpretative training of this science began to reveal new indicators, despite evident limitations consistent in math requirements under the professional profile. These indicators revealed aspects that displayed an increase in the contextualization of mathematical contents taught in different careers and the need to raise levels of appropriate procedures for solving problems and interpretation of results according to the practical application.

4.4. Stage Three. Systemization of the Process of Mathematical Training in Higher Education (2003 - Present)

In the school year 2003-2007, a new dynamic in the process of mathematical training in Angola was implemented. In the Superior Institute of Educational Sciences of Huambo, this dynamic allowed the transition in the time required to attain a mathematics degree from 5 to 4 years.

With the implementation of these transformations, the process became an integrated system [23] and involved the establishment of new relationships between the different disciplines that make up the curriculum. This process allowed the elimination of some disciplines and the inclusion of others such that the integration guaranteed a new quality, with an aim to strengthen the process of mathematical training.

In this case, the authors postulate that to contribute the ongoing transformations of this process, it requires the reorganization of the teaching-learning process as a whole. This reorganization would entail restructuring of planned objectives and curricula, contextualization of the contents, diversifying the teaching methods and techniques as well as the integration of teaching aids.

Today, one of the most criticized aspects of teachers and researchers in mathematical education in Angola is evidently the application and the role of this science to social and economic problems experienced by citizens. However, tutorial encouragement is lacking, a form interpretation, which demonstrates the mathematical experiences of the environment in which the students are developed.

In designing the curriculum for a degree in Mathematics, taking into consideration the above trends of scientific, technological and methodological development, conceived by strengthening the dynamics of mathematical training, it is essential to establish a relationship between the content and its application to real life. It is also necessary to deepen the objectivity of mathematical knowledge and an understanding of how to extend it in solving problems of other branches of science.

However, the issued statements do not pass from paper to action, causing as a result students to delay in adapting to the scientific, technological and methodological changes that occur constantly in the world.

The developed research, either by national and foreign authors, has an aim to strengthen the dynamics of mathematics training; the development of logical thinking and interpretive university students, such as, [29], and [30].

However, epistemological and methodological analysis of the contributions made by these authors, has allowed one to appreciate that there are still aspects that need to be argued and reinterpreted in terms of achieving higher levels of understanding the mathematical content, its applications, and strengthening the dynamics of the formation of this interpretive science.

Therefore, characterization of the defined stages, lead to specify that the main historical trends of the mathematical training process in the Angolan higher education, and the interpretative dynamics of this science start from:

• Educational concepts that emphasize reproduction, memorization of the contents, methods, procedures, troubleshooting; insufficient focus on the demands of math in accordance of the professional profile in the formation; conceptions that recognize the need for integration of methods, teaching aids and the amount of the mathematical content as required by the profession.

• Epistemological and methodological approaches that make high emphasis on problem solving, an approach that takes into account the students' participation, as the center of the teaching-learning process; responsible for their own process of scientific and technological formation, in tune with the needs of practical application.

5. Structure of Graduation in Higher Angolan Education

In Angola, higher education is taught in public and private schools and covers Bachelors, Masters and doctoral degrees. These institutions perform one or more of the following activities: teaching, scientific and humanistic research, technological studies, university extension, environmental preservation and cultural diffusion.

The Bachelorette (Bacharel) corresponds to short-cycle courses, with a duration of three years, which aims to enable the student to acquire basic scientific knowledge in a respective professional domain.

Degree studies are developed over a period of four years due to the results of ongoing educational reforms throughout the country aimed with the objective of the acquisition of knowledge, basic practices and skills development for professional performance.

The post-graduation has two categories: post-academic graduation and post-professional graduation.

The post-academic graduation has two levels:

Masters with 2 to 3 years duration, its main objective is to enrich the technical and professional competence of graduates.

Doctorates are attained in a period between 4 to 5 years and aims to provide a scientific, technological and humanistic education and broadens the scope of learning for graduates of degrees and Masters.

The post-graduation includes professional specialization.

Specialization is for courses lasting at least one year and has as an objective to enhance the technical-professional graduate.

6. Methodological Aspects

We used the historical-logical method, document analysis and stratified random sampling technique.

The procedure used in preparing the questions, fits the Likert style and attributed to a scale ranging from 1 to 5, the value corresponding to an appropriate response, depending on the level of knowledge that the subject (teachers and students) has about the topic.

7. Context and Sample

Given the need to strengthen the process of mathematical training in Angola, the characterization of its current state, is carried out through questionnaires applied to the students and teachers.

To select the sample we applied a stratified random sampling technique already used by [30]. Our stratum is defined by 40 students from high school institutes, 50 pursuing a Bachelor’s degree in Mathematics and 20 university Mathematics teachers.

The participants were selected randomly during the period of February 2011until June of 2014.

The activities were implemented in a regular teaching context administered to 12^th grade students of the high school institute of Huambo, as well as first year students of the Higher Pedagogical institute of the above mentioned province.

7.1. Questionnaires Applied to the Students

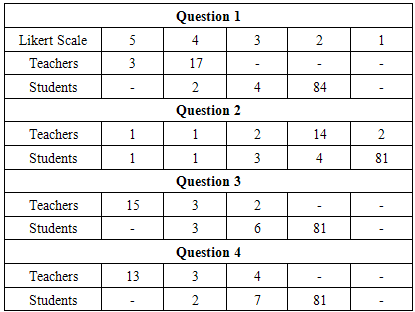
The aim of implementing the survey to the stratum is to characterize the process of mathematical training and to explain the potential that this science has in solving concrete problems of the society.

This survey is part of a doctoral research on interpretive training in Higher Mathematics, [16] to help raise the quality of teaching and learning process in Angola. In this process, we ensured that these surveys were anonymous.

Therefore, the subjects were instructed to mark with an (x) the square that corresponds to their answer. (As shown in the data table 1).

Question 1.

Are the methods and procedures used by the teacher, appropriate in helping you to play a leading role in the interpretation of the results?

Question 2

Does the teacher employ the use of software in the process of solving complex problems, graphing functions, verifying results and its interpretation?

Question 3

Do the activities performed by the teacher in the class motivate you to pursue a career in mathematics?

Question 4

Are the exercises and problems addressed in the class contextualized to your profession?

7.2. Questionnaires Applied to the Teachers

Question 1

Do your methods, techniques and procedures allow the students to play a leading role in the interpretation of the results?

Question 2

Do you employ the use of software in the process of solving complex problems, graphing functions, verifying the results and its interpretation?

Question 3

Do the activities that you perform in the class contribute to yours students’ motivation in pursuing a career in mathematics?

Question 4

Are the exercises and problems addressed in the class contextualized to the students’ profession?

8. Data and Its Analysis

After tabulating the data, we proceeded to its interpretation, according to the criteria of the assumed scale: Very frequent (5), Quite frequent (4), Frequent (3), Infrequent (2), Never (1).

As we can observe in the table 1, 85% of the surveyed teachers agree that the use of active methodologies in the teaching and learning process of mathematics, contributes to increase students’ participation in strengthening of the mathematical training process.

Table 1. Data

Nevertheless, 93% of students require the active use of methodologies that take into account their participation, as a center of the teaching and learning process.

70% of teachers consider the employment of software irrelevant in the mathematical problem solving process, graphical representation, verification and interpretation of results, due to the limited domain and technological focus given to the mathematics teaching.

90% of students expressed that they did not know the usefulness of these tools, due to the excessive use of traditional methods and teaching aids.

75% of teachers reveal that the lack of motivation of students to study mathematical careers is due to the limited basic preparation, insufficient appreciation of mathematics epistemology, its roots and perspectives as an indispensable tool for the students and mathematics teachers.

They also reveal that this situation has contributed significantly to awaken concern to study the process of mathematical communication, understanding, interpretation and practical application of this science.

However, 90% of students said that is due to the abstract nature of mathematics, it is difficult to apply it in solving practical life problems.

65% of teachers considered that it is necessary to improve the process of mathematical training through the contextualization of contents, problems and results.

In this case, 90% of students expressed the need for higher levels of contextualization in order to qualitatively strengthen the process of mathematical training in Angola.

9. Results and Discussion

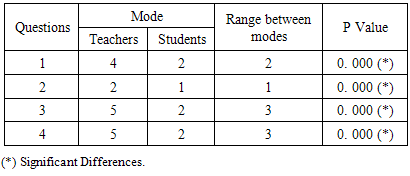
To evaluate the results of the research carried out, it is imperative to comprehend the problems in the dynamics of Angolan mathematical training, as well as the role of contextual interpretation.

To corroborate the results, we applied the non parametric test of Mann-Whitney and Kolmogorov-Smirnov for two independent samples: a group of teachers and a group of students.

The responses were analyzed from both groups in relation to the above mentioned questionnaires.

The results of the statistical analysis in table 2 indicate that the perception of students and teachers differs significantly with p values = 0.000 in all cases.

Table 2. Summary of the data

The research also showed a great contradiction in the responses of the teachers and students which explains the wide variation of modes in questions 3 and 4. Therefore showing a correlation between the responses of these questions in that if the content is not contextualized it does not contribute to the students´ motivation.

In order to find evidence which would further corroborate the analysis and interpretation of the obtained results, we selected 20 experts. Among them, 4 belong to the Catholic University of Angola - Luanda, 3 are from Jean Piaget University - Benguela, 4 belong to the high school Institute - Huambo and 8 are from the Higher Pedagogical Institute of Huambo.

In this process the experts were given a copy of the applied questionnaires, as well as the results.

95% of the experts were in agreement of the responses regarding the need to strengthen the process of mathematical training in Angola, by using active methodologies in the teaching and learning process and software.

They also agreed that it is necessary to raise the level of students’ motivation in mathematical careers, through an appropriate contextualization of the contents, problems, results and its application in solving specific problems of life.

For data processing, we used the IBM SPSS Statistics 20, with which we obtained the statistical position and dispersion that allowed us to summarize the information which demonstrates that, the aim of this research was fulfilled.

10. Conclusions

The solution of the problems detected in the dynamics of Angolan mathematical training requires profound transformations so that students can raise their levels of understanding of the role which mathematics plays in the development process of human thought as well as in social transformation.

The analysis of the historical background process of mathematical training, allowed us to appreciate that there are still aspects that need to be argued and reinterpreted, with the hope of achieving higher levels essentially in the contextualization of mathematical contents and its applications.

The present investigation allowed us to observe that in the different provinces of Angola, there exists a low motivation among students to study mathematical careers, due to the limited practical focus of mathematics and its transcendence to solve concrete problems of life.

ACKNOWLEDGMENTS

The direction of Scientific & Academic Publishing (USA) for reviewing the paper and the decision taken to socialize the main results obtained during this research.