-
Paper Information
- Next Paper
- Previous Paper
- Paper Submission
-
Journal Information
- About This Journal
- Editorial Board
- Current Issue
- Archive
- Author Guidelines
- Contact Us
Education
p-ISSN: 2162-9463 e-ISSN: 2162-8467
2012; 2(3): 37-40
doi: 10.5923/j.edu.20120203.03
Conductometric Studies of Calcium Ions with Kryptofix 221 in Mixed MeOH-DMF Solvents at Different Temperatures
Esam A. Gomaa 1, B.A. Al-Jahdali 2
1Chemistry Department, Faculty of Science, Mansoura University, 35516- Mansoura, Egypt
2Chemistry Department, Faculty of Applied Science, Umm Al- Qura University, Saudi Arabia
Correspondence to: Esam A. Gomaa , Chemistry Department, Faculty of Science, Mansoura University, 35516- Mansoura, Egypt.
| Email: |  |
Copyright © 2012 Scientific & Academic Publishing. All Rights Reserved.
On using conductometric technique, the apparent association constant (KA) of Ca (NO3)2 were measured in mixed MeOH-DMF mixed solvents at 0, 20, 40 , 60 ,80 and 100% MeOH (by volume) and different temperatures (298.15, 303.15, 308.15 and 313.15K) in absence and presence of . Kryptofix- 221 [4, 7, 13, 16, 21- pentaoxa-1.10-diazo-bicyclo (8,8,5) tricosane]. From the experimental results, the molar conductivities (∧) were calculated and limiting molar conductivities (∧0) were calculated by using Shedlovsky and Fouss – Kraus extrapolation methods. The molar solvated (∨), Van der Waals (∨w) and electrostriction (∨e) were evaluated. The association constants KA for Ca(NO3)2 were calculated by using simple equation derived from Ostwald, Arrhenius and Fuoss-Shedlovsky theories. From the association constants the different thermodynamic parameters were evaluated and the results obtained were discussed. The free energy of association (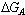 ), free energy necessary for complexation (
), free energy necessary for complexation (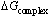 ) and enthalpy (
) and enthalpy ( ) were determined. It was concluded that the maximum complexation between calcium ions and Kryptofix-221 were by using 80% and 100%. MeOH in the mixtures. Where as the least complexing ability at 40% and 60% by volume of MeOH .This work give data can help for the analytical determination of calcium in blood and water samples.
) were determined. It was concluded that the maximum complexation between calcium ions and Kryptofix-221 were by using 80% and 100%. MeOH in the mixtures. Where as the least complexing ability at 40% and 60% by volume of MeOH .This work give data can help for the analytical determination of calcium in blood and water samples.
Keywords: Association Constants, Activity Coefficients, Different Volumes, Free Energies of Association, Solvation Enthalpies, Calcium , Kryptofix 221
1. Introduction
- Calcium is an essential element in living organism.It plays an important role in the metabolism of nitrogen in some plants, where a deficiency of calcium leads to poor absorption of nitrogen. Lack of calcium nutrition leads to a reduction in the number and size of chloroplants.Cacium in the most abundant inorganic element in the higher animals, and is located principally in the bones and teeth as apatite, a calcium mineral.Bllod is also a huge reservoir of calcium in animals. Calcium is distributed throughout all tissues where it has special roles in controlling g nerve impulse transmission, muscle action, blood cotting and cell permeability. Calcium deficiency is exhibited by the outset of rickets, failure of blood-clotting, nervous disorder andconvulsive muscular contractions. Vitamin D greatly improves the absorbability of calcium ion. Large intakes of calcium lead to excessive calcification and kidney stones. Almost all natural waters, including seawater, contain either or both calcium carbonate and calcium sulphate.Many organisms concentrate calcium in their shells or skeletons. For example calcium carbonate is formed in shells of oysters. Calcium has significant applications in the production of alloys with lead and aluminium and in industrial products of metals from its oxides.It is also used as deoxidizer with iron, steel and its alloys.[1,2]. The main target is to data help for calcium analysis.The association of salt solutions were studied by Barthel [3] and Deepa[4]. The ion solvent interactions were explained in different electrolytes and polymers using conductivity, relaxation and FTIR measurements1.Schori et al.[5,6] used conductivity as an electrical method for studying complexation of Na+ with some crown ethers in DMF and in diemthoxyethane solutions.Different Fuoss theories, Fuoss-Shedlovsky, Fuoss-Kraus and Fuoss-Debye theories[7,8]were used for the estimation of the association constants (KA) for symmetric electrolytes (1:1 electrolytes).We apply here simple equation for determining KA for asymmetric 1:2 salts in solutions based on Fuoss-Shedlovsky and Ostwald law[8]. Evaluation of individual ionic molar volumes[9], preferential solvation of drugs[10] partial molar volumes[11], ligands used for selective metal extraction[12], ionic salvation[13], potntiometric study[14] and specific salvation[15] are some trends of this area of physical inorganic chemistry.
2. Experimental
- Kryptofix 221 (Fig.1) was supplied from Fluka Co., whereas Ca(NO3)2 was supplied from BDH. The water content of Ca(NO3)2 was determined by using (Mettler DL-18) Karl-Fisher titrator and it was found to be less than ±0.01%. methanol (MeOH) and N,N-dimethylformamide (DMF) used were from BDH. All the conductometric titrations were manipulated using 1x10-3 mol/l Ca(NO3)2 and 1x10-4 molal of Kryptofix 221 as stock solutions. The specific conductivity (KS) measurements were achieved at different temperatures using a conductometer of the type YSI model-35 connected with an ultra thermostatic water bath of the type Kottermann-4130.
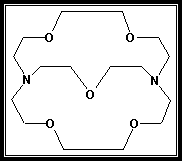 | Figure 1. Kryptofix-221 [4,7,13,16,21-pentaoxa-1.10-diazo-bicyclo [8,8, 5] tricosane] |
3. Results and Discussion
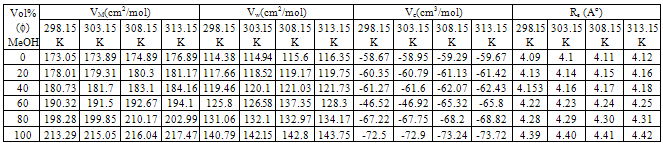
- The molar volumes of Ca(NO3)2 stork solution (VM) were calculated by dividing the molecular weight of the salt by the measured densities. From the packing density (P) as reported by Kim[16,17], i.e., the relation between Van der Waals volume (VW) and the molar volume (VM) for large molecules (M.W. above 40) the Van der Waals volumes for Ca(NO3)2 were evaluated (equation 1)[16,17].
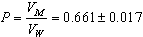 | (1) |
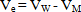 | (2) |
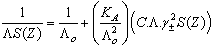 | (3) |
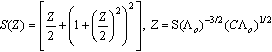
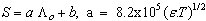
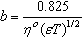 ε is the dielectric constant, ηo is the viscosity of the solvent, T absolute temperature and S(Z) is the Fuoss-Shedlovsky factor.With the use of equation (3) for 1: 2 electrolytes, small values are obtained for KA . Therefore another simple equation can be applied by following equations (4-8)
ε is the dielectric constant, ηo is the viscosity of the solvent, T absolute temperature and S(Z) is the Fuoss-Shedlovsky factor.With the use of equation (3) for 1: 2 electrolytes, small values are obtained for KA . Therefore another simple equation can be applied by following equations (4-8)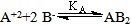 | (4) |
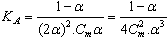 | (5) |
 | (6) |
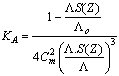 | (7) |
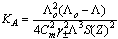 | (8) |
 ,
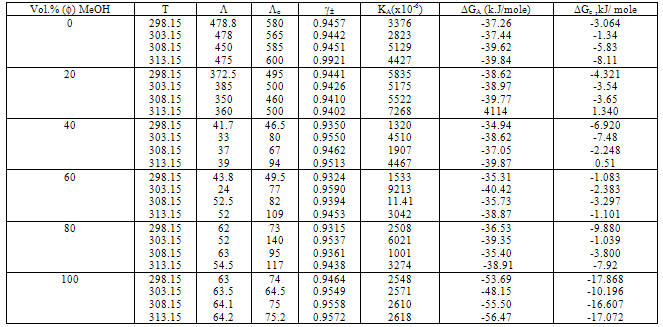
,  o for Ca(NO3)2 solutions were evaluated at concentration equal 8x10-5 as inflection point and zero concentration, respectively.KA association for Ca(NO3)2 in absence and presence of Kryptofix 221 were evaluated and their values are given in Tables 2 and 3, knowing that S(Z) factor for all solutions were found to be approximately one.The Gibbs free energies of association were calculated by applying equation (9) [20-23] in absences and presences of Kryptofix 221 in mixed MeOH-DMF solvents at different temperatures and their values are shown also in Tables (2) and (3).
o for Ca(NO3)2 solutions were evaluated at concentration equal 8x10-5 as inflection point and zero concentration, respectively.KA association for Ca(NO3)2 in absence and presence of Kryptofix 221 were evaluated and their values are given in Tables 2 and 3, knowing that S(Z) factor for all solutions were found to be approximately one.The Gibbs free energies of association were calculated by applying equation (9) [20-23] in absences and presences of Kryptofix 221 in mixed MeOH-DMF solvents at different temperatures and their values are shown also in Tables (2) and (3).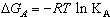 | (9) |
|
|
|
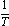 with slope equal
with slope equal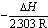 . The evaluated data were listed in Table (4) in absence and presence of ligand.It was concluded from the given tables that the maximum complexation between calcium ions and Kryptofix-221 were by using 80% and 100%. MeOH in the mixtures. Where as the least complexing ability at 40% and 60% by volume of MeOH. This behaviour was indicated from ΔGC and ΔH values cited in Tables 3 and 4, respectively.
. The evaluated data were listed in Table (4) in absence and presence of ligand.It was concluded from the given tables that the maximum complexation between calcium ions and Kryptofix-221 were by using 80% and 100%. MeOH in the mixtures. Where as the least complexing ability at 40% and 60% by volume of MeOH. This behaviour was indicated from ΔGC and ΔH values cited in Tables 3 and 4, respectively. 4. Conclusions
- We tried in this work to give data that can help for biological and analytical determination of calcium ions in blood,tissues,water and industrial samples.The free energy values for calcium ions are great in 80% MeOH-DMF and pure MeOH, whereas the conductance values are great in 20 % MeOH-DMF and pure DMF.These data explains the ion solvent interaction trend in the same order. All the thermodynamic parameters for calcium ions are greater in presence of Krytofix 221 than in absence of it, favouring more complexing character and ease of detection on using Kryptofix221. The ligand used can be extracted by chloroform and by evaporating the solvent the solid can be obtained for further use.
References
| [1] | Wikipedia,the free encyclopedia. |
| [2] | www.springerlink.com |
| [3] | Barthel, J. Krienke, H., Holovlao, M.F., Kapke, V.I. and Protsykevick, i., Cond. Mat. Phys., 3, 637 (2000). |
| [4] | Deepa, M., Agnihorty, S.A. Gupta, D., Chondra, R., Electrochimica Acta, 49, 373 (2004). |
| [5] | Schori, E., Jagur, J., Grodzinski, Z., and Shporer, M.; J. Am. Chem. Soc., 93, 7133 (1971). |
| [6] | Schori, E., and Jagur, J. and Grodzinski, Z.,Isr. J. Chem. Soc., 11, 243 (1973). |
| [7] | Covington, A.K., and Dickinson, T., “Physical Chemistry of Orgnaic Solvent Systems”, Plenum Press, London (1973). |
| [8] | Fuoss, R.M. and Accasina, F., “Electrolyte Conductance”, Interscience, New York (1959). |
| [9] | Gy.Jakli, Journal of Chemical Thermodynamics, 40, 770 (2008). |
| [10] | Yizhak Marcus, Journal Molecular Liquids,140 ,61(2008). |
| [11] | K.Rajagopal. S.Edwin Gladson, Journal of Chemical Thermodynamics. , 43,852 (2011). |
| [12] | Sk. Musharaf Ali. Dilip K. Maity, Sulagno De, M. R,Shenoi,. K. Deslination, 232 181(2008). |
| [13] | Hongwei Qlas,Helin Luan, Zhiming Zhou,Lixue Bi, Wen Yao,Jimei Li and Chang Chen, Journal of Molecular Structure, 885,89-96 (2008). |
| [14] | Ewa Kamenska-Pictrowicz, Janusz – Stangret, Joanna Szymanska–Cyblaka, Spectrochimica Acta, Part A, 60, 1 (2007). |
| [15] | Albert J.Fry, L. Kraig Steffen, Journal of Electranalytical Chemistry, 638,218(2010). |
| [16] | Kim, J.I., J. Phys. Chem., 82, 191 (1978). |
| [17] | Kim, J.I., and Gomaa, E.A., Bull. Soc. Chim. Belg., 90, 391 (1981). |
| [18] | El-Khouly, A.A., Gomaa, Esam, A. and Abou El-Leef, S., Bull. of Electrochemistry, 19, 193-202 (2003). |
| [19] | Dash, N.U., and Pasupalala, N.N., Ind. J. Chem., 36, 88 (1997). |
| [20] | Gomaa, Esam, A., Thermochim. Acta, 120, 183-190 (1987). |
| [21] | Gomaa, Esam,A., Science and technology, 2,51 (2012). |
| [22] | Gomaa, E.A. and Al-Jahdalli,B.M.,American Journal of Condensed Matter Physcics.,2, 16 (2012). |
| [23] | Gomaa, E.,A., and Al-Jahdalli,B.M.,American Journal of Fluid Dynamics.,1,4 (2011). |
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-Text HTML
Full-Text HTML