M. M. Rakhimova, N. Z. Yusupov, T. M. Nurmatov, J. A. Davlatshoeva, L. Kvyatkovskaya, A. R. Bakhtibekova, M. Bobonazarov
The Research Institute of the Tajik National University, Rudaki aven.,17, Dushanbe, 734025, Republic of Tajikistan
Correspondence to: M. M. Rakhimova, The Research Institute of the Tajik National University, Rudaki aven.,17, Dushanbe, 734025, Republic of Tajikistan.
| Email: |  |
Copyright © 2012 Scientific & Academic Publishing. All Rights Reserved.
Abstract
Method oksidimetryis a highly sensitive, low-cost, easy-to of the experiment and was used extensively in the study of complex transition metal anions of monobasic organic acids in the acid pH range. In the presence of polybasic acids or multi-ligand, when the probability of formation of heterovalent coordination compounds, complexes with protonated complex composition, mixed ligands require new approaches and methods of thermodynamic calculations. Proposed oxidative function and the principle of equilibrium modeling, which allows to study the processes of formation of complexes of any complexity in the whole pH range, as well as using computer programs to accelerate the calculations and improve their accuracy. The possibility of using oxidative function and principle of modeling for the study of complex systems with multi-ligand: Fe (II)-Fe (III)-hydroxybenzoic (salicylic) acid-water. Drawn up on the basis of the experimental data model is the model parameters: structure, stability, the existence, degree of accumulation (mole fractions) formed salicylate coordination compounds of iron. Shown that the most stable are binuclear coordination compounds and heterovalent complex - FeIIIFeIIL2ОН0. Model parameters allowed a clear distinction between the domain of existence, domination and isolation of a stable solution with a high degree of accumulation of biologically active complexes of practical interest. So they can be ready.
Keywords:
Coordination Compounds, Oxidimetry, Oxidative Capacity
Cite this paper: M. M. Rakhimova, N. Z. Yusupov, T. M. Nurmatov, J. A. Davlatshoeva, L. Kvyatkovskaya, A. R. Bakhtibekova, M. Bobonazarov, Salicylate Coordination Compounds of Iron, Models of Their Formation, American Journal of Chemistry, Vol. 3 No. 2, 2013, pp. 23-29. doi: 10.5923/j.chemistry.20130302.01.
1. Introduction
Preparations based on hydroxybenzoic (salicylic acid) and its derivatives are a group of non-steroidal anti-inflammatory drugs (NSAIDs), which are extensive and diverse in chemical structure group of drugs that are widely used in clinical practice. Salicylic acid derivatives (salicylates) entered into medical practice in the late XIX century, and are commonly used to date. Derivatives such as acetylsalicylic acid (aspirin), sodium salicylate, salicylamide, methyl salicylate, used as painkillers (analgesics), fever (antipyretics) and antithrombotics (antiplatelet agents) means[1-3]. According to the latest derivative of salicylic acid can be considered as bioregulators that are synthesized by the body and perform its protective function and boost the immune system[4,5]. Having enumerated effective properties, these drugs have some side effects. They have a damaging effect on the mucous membrane of the gastrointestinal tract, violate the kidneys.In the literature, there is evidence that the coordination compounds of some metals, especially the 3-d transition, with salicylate ions have increased biological activity. For example, complexes of iron and calcium with aspirin does not cause side effects, less is irritating to the mucous membrane of the stomach and intestines. In addition, salicylates exist in the stomach, intestines, blood plasma and other organs[6,7], that is, for the living organism are homogeneous.In this connection, the actual task of chemists, physicians and pharmacists is to develop new, improved methods for the synthesis of known drugs (including coordination compounds of transition metals) with salicylic acid and its derivatives, as well as highly targeted search for therapeutic agents in a highly biologically activity combined with low toxicity and less severe side effects. Effectively address these problems contribute to new approaches in the experiment, methods of calculation of the thermodynamic parameters and software.Therefore, the aim of the present work was to study the complexation of iron with salicylic acid anions that have useful biological properties, using the oxidation potential of Nicholas Clark[8,9] and the proposed oxidative function and principle of modeling of chemical equilibria.
2. Exprimental
In determining the oxidative capacity of the plant is used as described in[9]. EMF measured three galvanic cells without wrapping. They were made up as follows: redox and silver chloride to measure EMF chain silver chloride and glass to determine the pH, platinum and glass to control the first and second readings of chains.EMF of galvanic cells was measured by EV-74 ion meter with an accuracy of ± 1 ÷ 3 mV. Chloride electrode potential values and the value of υ = 2.303 RT/F at required temperatures were taken from the reference[10]. Platinum electrodes were polarized before the experiments in a 5% solution of sulfuric acid. Glass electrodes brand ESP-63/07 and ESP-43-07 to restore the function of the hydrogen pre-reated with steam, and then kept in 0.1 N hydrochloric acid. The slope of the E - pH was 58-59 mV, which corresponds to its theoretical value. The pH value in the solutions was controlled by a glass electrode in the calibration curve composed by the values of pH standard buffer solutions.Measurementaccuracy was ±0.05of the pH units.In the experiments, as the initial perchlorate salts used metals having the least complex-forming ability[9]. The salts were obtained by the method described in[10]. Concentration of iron (III), iron (II), sodium hydroxide used acids were determined by appropriate methods[11-13]. Perchloric acid HClO4 brand "chemically pure" was used without purification. NaClO4 salt was purified by recrystallization of a saturated aqueous solution. Equimolar mixture of iron perchlorate in two oxidation states prepared in 1 M perchloric acid, and their total concentration of the restoration of Fe (III) to Fe (II) with the gearbox Johnson amalgamated zinc[14].According to the theory of the method of oxidimetry composition formed in the system of coordination compounds is determined by analysis of the experimental curves of the oxidation potential of one of the parameters: pH, PCo, PCR, PChl and p (C0 = Cr) at constant rest. From the slopes of the curves are determined by the number of atoms of the oxidized and reduced forms, the total number of ligands and coordinated hydroxyl groups is expected to be formed complexes. The general equation of the oxidation potential output in view of the complexes and their formation constants are then calculated by the method of approximation. As shown by the large accumulation of experimental and theoretical data on the study of complex formation in the redox systems the original theory of the method of oxidative potential matches only the simplest systems with transition metals and ligands in monobasic acidic pH, where there is no education heterovalent coordination compounds. With increasing pH where increases possibility of mixed-, and polynuclear coordination compounds heterovalent theory had to be expanded and enhanced with new improved approaches for thermodynamic calculations that take into account the probability of formation of complexes of all possible compositions. Theory was developed by Professor Yusupov, for quick and accurate calculations using sophisticated computer programs they offered a new oxidative function.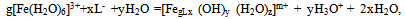 | (1) |
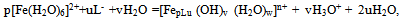 | (2) |
where: g - the number ofatoms ofFe (III)in the complex; x-number of acidoligandsandL-acid residue; y-number of coordinatedhydroxyl groups; z-number of coordinatedwater molecules; m-charge of the coordination compound ofFe (III); p-number of atoms ofFe (II)in the complex; u-number of coordinated ligandsL-; v-ordinated a numberof hydroxyl groups; w-the number of coordinatedwater moleculesin complexes ofdivalent iron; n - charge of thecoordination compound ofFe (II).The values of x, m, u, n otherrelated as follows: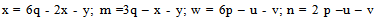 | (3) |
The balance between the metal ions in aqueous solutions is given by: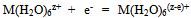 | (4) |
The general equation of the oxidation potential φ, when formed heterovalent, mixed-complexes is: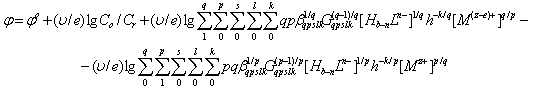 | (5) |
where: φ0-standard oxidation potential, C0 is the total concentration of the oxidized form of the metal, Cr - total concentration of the reduced form of the metal; υ = 2.303 RT / F; q-number of atoms in the oxidized form (nuclear complex compound), k-number of protonated groups in the complex , x is the number of groups of A b-, y-number of coordinated hydroxyl groups, r - the number of coordinated water molecules, m - charge of coordination compound of oxidized metal, p - the number of atoms of the reduced form of the metal (the nuclear complex compound), l - the number of groups in the protonated complex, u - A number of coordinated ligands, ν - the number of coordinated hydroxyl groups, w - the number of coordinated water molecules, n - charge of coordination compound of the reduced form of the metal. The water activity of the investigated dilute solutions is constant and is taken to be unity.The experimental dependence of the oxidation potential of the pH according to the equation (4) Calculate the experimental oxidative function: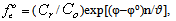 | (6) |
and then plotted on the pH of fe. The number of linear segments on the curve indicates the number of formed coordination compounds, and their length - the interval of existence of the complexes on the pH scale.Theoretical oxidative function is the amount of: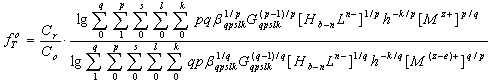 | (7) |
where all the symbols correspond to the notation of (1-5) Each amount represents a portion of the equation some coordination compounds in accordance with the inclination angles (coefficients) of the experimental curves, which are determined by an analysis of their values derived oxidative capacity in one of the specified parameters. The true values of the formation constants of complexes are theoretical values iteration oxidative function for experimental computer program Excel. Calculations are considered complete when the theoretical and experimental dependences of oxidative function of pH as the same, which usually occurs after 8-10 approximations. Thus, oxidative function allows you to determine the composition of coordination compounds, make design work to determine the constants of the formation of complexes with less time consuming and more reliable data.Calculations of the formation constants of coordination compounds easier to perform using chemical equilibrium models - matrices with the numerical values of basic particles, is expected to be complex, the reactions of their formation. To do this, you first need to create a common table (stoichiometric matrix) all equilibria with the numerical values of the slopes of the φ - pH, φ - pCo, φ - pCr , and φ - pCL experimental curves redox. Instead of presenting a large number of uniform rectilinear curves with different slopes, drawn stoichiometric matrix for the entire range of the process of complex on the pH scale (Table 1). Then, from the data in this table is based chemical model, which are introduced in the form of columns basic particles: the numerical values of the nuclear complexes of the oxidized and reduced forms of the metal, the number of coordinated ligands and the number of protons in them, hydroxyl groups - g, p, s, l and k, respectively, which are determined by comparing the slopes of the experimental curves and the partial derivativesof the general equation of oxidation potential (5) on the concentration variables. For example, we have following for the dependence of φ - pH: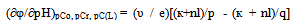 | (8) |
If the main acid is b, then from equation (8) we obtain (n +1) partial derivatives(n = 0, 1, 2, ...), to allow an assessment in coordination compound of the total number of proton proton-donor ligand-H b-n L n-1. However, to determine the equilibrium shapes of ionization products acid should be considered a partial derivative of the oxidation potential relative to PC L: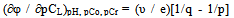 | (9) |
In terms of the concentration of the oxidized and reduced forms of the metal partial derivative becomes: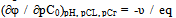 | (10) |
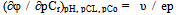 | (11) |
The numerical values of basic particles: g, p, s, l and k from the table of chemical model used to write subscripts complexation constants to simplify the representation of the composition of coordination compounds. According to a set of numbers q, p, s, l and k we obtain the equations of each line separate the linear region, the sum of which provides a general theoretical expression of oxidative function of the studied system.All further calculations to determine the formation constants of coordination compounds made us with an oxidizing function described above and the computer program Excel. Then, to calculate the degree of accumulation (mole fractions) of the complexes formed from the general formula Ni = ni/∑nij. On the basis of this expression, the accumulation of degree can be expressed as: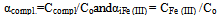 | (12) |
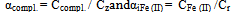 | (13) |
where, αi - mole fractions of equilibrium reactions of complex shapes of components, and the CFe(III) and C Fe (II) the equilibrium concentration of free iron ions. The calculated values of the stability constants of the complexes, the values of the maximum mole fractions (degrees of accumulation), the interval of their existence on the pH scale, the fragments of the equation for the oxidation potential of each individual and the complex are recorded in a separate table of model parameters.
3. Results and Discussion
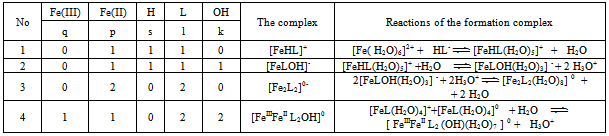
For a system of Fe (II)-Fe (III) - salicylic acid-water, the from one of the φ experimental curves of the oxidation potential parameters: pH.pCo, pCr,pCHL and p (C0 = Cr) at constant all other (concentration conditions are shown in the tables). As example here is presented dependencyφ from pH (Fig. 1).Analysis of the data held in view of the fact that for salicylate (o-hydroxybenzoic – NOC6N4 COOH) acid in the acidic pH region dominated protonated form of the ligand, HL- , and with increasing pH – L2-.According to the theory of the method carried out a joint analysis of the slopes of these relationships and their experimental values compiled stoichiometric matrix (Table 1). Comparison of the experimental slope of the curve (3, 4, 5 and 6 columns of Table 1) Partial oxidation potential of equations (6)-(9) in the variables allowed the calculation of the numerical values of basic particles,a table of themodelof the formation of the complexes (Table 2) and determine theirtentative composition:[FeHL]2+,[FeНL(ОН)]+, [Fe2(НL)2]4+,[Fe2L2(ОН)2]0,[FeIIIFeIIL2ОН]0,[Fe2L2(ОН)4]2-,[FeНL]+,[FeL(ОН)]-,[Fe2L2]0 .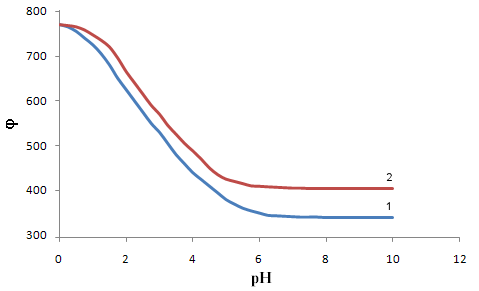 | Figure 1. The dependence ofthe oxidation potentialof φ on pHfor the system: Fe (III)-Fe (II)-salicylic acid-waterat a temperature of298.16K,ionic strengthof the solution I = 1.0;СFe(III) = СFe(II) = 1•10-3: (1) CНL =1•10-1mol / l; (2) CНL =1•10-1mol / l |
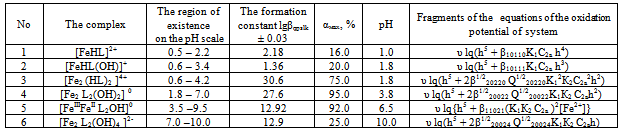
Thus, it was found that in addition to heterovalent coordination compound Fe (III) forms five complexes of different composition, and Fe (II) – threecomplexes.At acidic pH, which is dominated by protonated ligand HL-formed complex of ferric iron[FeHL]2+, then the mixedligands (with hydroxyl group) and binuclear. Andbinuclearcomplex forms and ferrous iron. Perhaps this is due to thefact that in the presence of certain metals ability of salicylic acid to the formation of dimer-type 9 cycles ksantenona increases. These complexes exist in a fairly large range, from 2 to 6 pH units. As the pH increases the likelihood of coordination compounds with the deprotonated ligand L 2-.Given the composition of the compounds (Table1) for known values g,p,s,l,k,n and[HL-] = K1K2C2ah-и[L2-] = K1K2C2ah-2, revealing the sum expression in equation (1) we get the general equation of the oxidation potential of Fe (III) -Fe (II)-salicylic acid-water:φ = φ0 + υ lq{h5 + β01110K1C2ah4 +2β1/202020P1/202020K1К2 C2ah3+ β01011K1K2C2ah4+ β11021(K1К2 C2a )2[Fe3+]} - υ lq{h5 + β10110K1C2ah4+β10111K1C2a h3 +2β1/220220 Q1/220220K12К2C2a2h2 +2β1/220022Q1/220022K1К2C2ah2+2β1/220024 Q1/220024K1К2 C2ah + β11021(K1К2 C2a )2[Fe2+]} This expression is the sum of the individual equations of the linear parts of the experimental curve φ-pH corresponding to each image of the complex and is used to find the approximate and exact values of their formation constants. Should note one feature of these systems.Strong ability salicylate ions to coordinate and form complexes significantly inhibits the hydrolysis of iron, so that the process of complex flows to pH 12.Modelling Education salicylate complexes of iron (Table 2) includes a number of basic particles, the composition formed coordination compounds and the equations of equilibrium with the material balance. This model is ready for the settlement of the stability constants of complexes with the use of oxidative function[15], the iteration method described above. Then, the equations (11 and 12) determine the degree of accumulation, which are the maximum mole fraction of the complex and fill the table model parameters complexes with fragments of redox equations used in their calculation (Table 3).The estimated model parameters (Table 4) showed thatsalicylate binuclear complexesFe (III) of[Fe2 (HL) 2] 4 +, [Fe2L2(ОН)2]0, are the most stable (lgβ 30.68 and 27.62 ), is dominated by a large range of pH(Fig.2, curves 4 and5), their degree of accumulation varies from 75.0 to 95.0%.These optionsmake the synthesis of these systems are easily realizable. In addition, the data in Table 3 shows that, with the number of hydroxyl groups in the complex decreases its stability, which is apparently due to the weakening of the link between the complexingagent and the ligand, as well as steric hindrance.Table 1. The experimental values of the slope of the oxidation potential of the concentrationVariables (stoichiometric matrix) for the Fe (II)-Fe (III)-salicylic acid-water at a temperatureof 298.16 K,ionicstrengthI = 0.5; СFe(III) = СFe(II) = 1•10-3 and СL = 1• 10-2
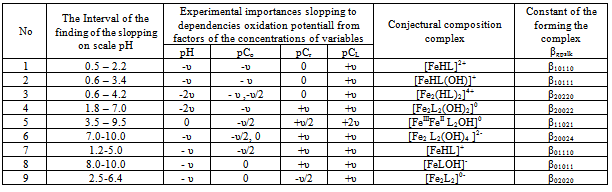 |
| |
|
Table 2. Chemical model of salicylate coordination compounds Fe (III) at 298.16 K, ionic strengthI = 0.5; СFe(III) = СFe(II) = 1•10-3and СL = 1• 10-2 mol / l
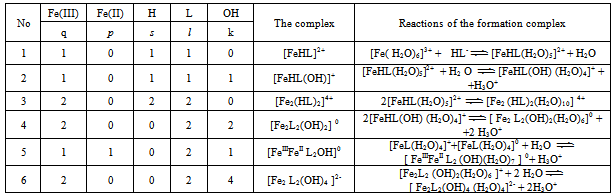 |
| |
|
Table 3. Chemical model of salicylate coordination compounds Fe (II) at 298.16 K, ionic strength I = 0.5; СFe(III) = СFe(II) =1•10-3 and СL = 1• 10-2 mol / l
 |
| |
|
Table 4. Model parameters of the salicylate complexes of Fe (III) at298.16 K, ionic strength I = 0.5; СFe(III) = СFe(II) = 1•10-4and СL = 1• 10-2mol / l
 |
| |
|
Table 5. Model parameters of the salicylate coordination compounds Fe (II)at 298.16 K, ionic strength I = 0.5; СFe(III) = СFe(II)= 1•10-3andСL = 1• 10-2mol / l
 |
| |
|
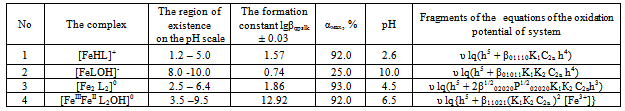
Heterovalent complex-[FeIIIFeIIL2ОН]0,which has a maximum degree of accumulation of 92.0%, there is a large range of pH (3.5 to 9.5)(Fig.2, curve6)and is stable (lgβ = 12.92).From the literature it is known that salicylate iron complexes exhibit biological activity, so the calculated model parameters of stable complexes (binuclear and heterovalent) were used to determine the optimal conditions for their separation from the solution. Tried and tested systems have been tested in the treatment bath salts with the addition of an extract of rose petals. The results showed that salicylate heterovalent iron complex is more active and raises a treatment efficiency of the components of salt, but also enhances their specific activity[16].Salicylate complexes of divalent iron[FeНL]+, [FeL(ОН)]-, [Fe2L2]0 (Table 5) is the least stable (apparently due to the oxidation of the metal), though their considerable accumulation (25.0, 92.0 and 93.0%). Their area of existence on scale pH was clearly defined from diagram of the distribution (Fig.3)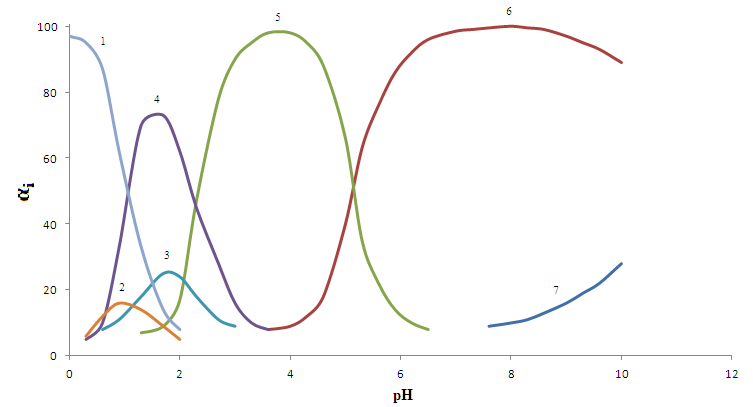 | Figure 2. Distribution diagram of the salicylatecomplexesFe (III)in the system: Fe(III)- Fe(II)-Н2L-Н2Оat298.16K,СFe(III) = СFe(II) = 1•10-4; CНL =1•10-2 and I =1,0 mol/l: (1)Fe3+; (2)[FeНL]2+; (3) [FeНLОН]+;(4)[Fe2(НL)2]4+; (5) [Fe2L2(OH)2]0; (6)[FeIIIFeIIL2OH]0-; (7) [Fe2L2(OH)4]2- |
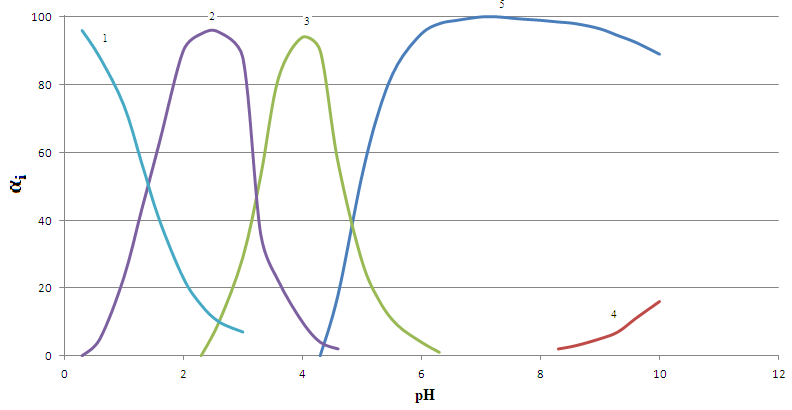 | Figure 3. Distribution diagram of the salicylatecomplexesFe (III)in the system: Fe(III)- Fe(II)-Н2L-Н2О at298.16K,СFe(III) = СFe(II) = 1•10-4; CНL =1•10-2 and I =1,0 mol/l: (1)Fe2+; (2) FeНL]+; (3) [Fe2L2]0; (4) [FeLОН]-; (5) [FeIIIFeIIL2OH]0- |
Stability binuclear above mononuclear and hydroxyl group, also makes coordination compound less stable, as discussed above.
4. Conclusions
Method of oxidation potential using the proposed c oxidative function and the principle of equilibrium modeling systems define the composition, stability, the existence, degree of accumulation of salicylate coordination compounds of iron:[FeHL]2+,[FeНL(ОН)]+,[Fe2(НL)2]4+, [Fe2L2(ОН)2]0 ,[FeIIIFeIIL2ОН]0,[Fe2L2(ОН)4]2-,[FeНL]+,[FeL(ОН)]-, [Fe2 L2]0.The possibility of using the principle of oxidative function modeling in redox systems hydroxybenzoic acid, which speeds up the calculation and increase their accuracy. The estimated model parameters complexes allowed a clear distinction between the domain of existence and stable, highly complex accumulation of fluid. Therefore, the model parameters can be ready reference tool for the directed synthesis and implementation of the various thermodynamic calculations.
References
| [1] | Kovalenko V.N., Compendium - 2005 – Drugs, Krasnodar, Russia: Morion, 2005, p. 1920 |
| [2] | Lisina S. V., 2006, Synthesis and pharmacological activity of new derivatives of salicylic acid and aspirin as potential drugs,: Success of modern natural science, 11, 95-96 |
| [3] | Lisina S.V., 2007, Studies antipyretic activity of new derivatives of salicylic acid : Bull. of the Volgograd State Medical University, Russia, 2, 66-69 |
| [4] | Brel A.K., 2008, Antimicrobial activity of derivatives of salicylic acid ,: Success of modern natural science, 5, 80-85 |
| [5] | Lisina S.V., 2008, Synthesis and antipyretic activity of new derivatives of salicylic acid,: Chemical Pharmaceutical Journal, V. 42, No. 10, 27-29 |
| [6] | Criss E.E., Metal coordination compounds in medicine, Kiev, Ukraine: NaukovaDumka, 1986, p.216 |
| [7] | Grigorieva A.S., 2000,Optimization pharmacotherapeutic activity biometalscomplexation with NSAIDs,: Trace elements in medicine, V. 2, No. 1, 17-22 |
| [8] | Clark W.M., Oxidation-Reduction Potentials of Organic Systems. Baltimore, USA: The Williams and Wilkins Company, 1960, p.584 |
| [9] | Nicholsky B.P., Palichevskiy V.V., Pendin A.A., YakubovKH.M.,Oksredmetriya. Leningrad, USSR: Khimiya, 1975, p.304 |
| [10] | Volkov A.B., ZHarskiy I.M., Large chemical directory . Minsk, Belarus: Belarusian Printing House. 2003, p.38 |
| [11] | KoryakinYu.V., Angelov I.I., Pure chemical reagents. Moskow, USSR: Khimiya, 1974, p.406 |
| [12] | Suslennikova V.M., Kisilyova E.K., Guide to preparing standard solution. Leningrad, USSR: Khimiya, 1968, p. 45 |
| [13] | Schwarzenbach G., Flashka G., Complexometric titration. Moskow,USSR: Khimiya, 1970, p.355 |
| [14] | Laitinen G.A., Chemical analysis. Zakarenok L.Z. Transl. Moskow, Russia: Khimiya, 2003, p.134 |
| [15] | Yusupov Z.N., The method for determining the composition and formation constants of coordination compounds . TJ Patent 295. Dec. 16.1997 |
| [16] | Yusupov N.Z., Rakhimova M.M., Yusupov J.Z., Yusupov KH.Z., Nurmatov T.M., Khasanova K.G., Ismatov F., Preparation of therapeutic salt baths with bio. TJ Patent № 513. Febr. 01.2012 |

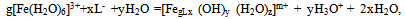
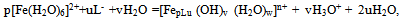
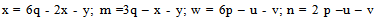
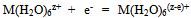
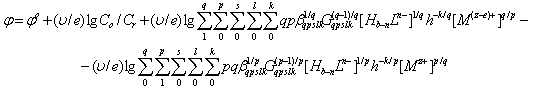
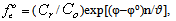
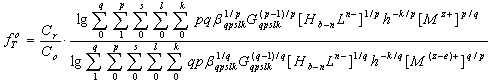
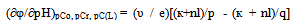
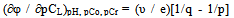
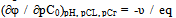
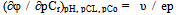
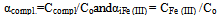


 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML




