Ari A. Mohammed, Hameed T. Abdulla, Alan S. Said Ahmad
Department of Physics, Faculty of Science, University of Zakho, Duhok, Iraq
Correspondence to: Ari A. Mohammed, Department of Physics, Faculty of Science, University of Zakho, Duhok, Iraq.
| Email: |  |
Copyright © 2016 Scientific & Academic Publishing. All Rights Reserved.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/

Abstract
Craters occur when an objects like Asteroids, Comets and Meteorites hits onto the surfaces of Moon and Planets and their impact has became a major geological process. The objective of the project is to investigate how the size of a Meteorite and Asteroid on the Moon and Planets is related to the size of impact crater. We started with a two round stone of different mass and size covered slightly with a layer of a viscous mud fluid as a fragmentation were dropped at variable height onto the flour. However, we have used a layer of sprinkles as a mineral diversity of the surface impacted. We have measured the diameter, depth and the Ejecta distance of the crater each time, there were three trails for each stone drop height. The results shows that, the crater diameter and the crater depth increased as the height and mass of the ball drop increased in addition of increasing Ejecta distance.
Keywords:
Impact crater, Diameter, Asteroid, Planet, Moon, Comets, Meteorites
Cite this paper: Ari A. Mohammed, Hameed T. Abdulla, Alan S. Said Ahmad, How the Size of a Meteorite and Asteroid on the Moon and Planets is Related to the Size of the Crater, International Journal of Astronomy, Vol. 5 No. 2, 2016, pp. 13-18. doi: 10.5923/j.astronomy.20160502.01.
1. Introduction
Craters form when an object with high velocity like Asteroids, Comets and Meteorites strikes the surface of a Moon and Planets. Craters are almost circular, excavated holes in shape made by impact events and are the most common features on many Planets and Satellites. Material from the surface is thrown from the impact area to form a ring of material called Ejecta. This ejecta is distributed outward from the crater’s center on to the Planet’s surface as Debris [1, 2]. Craters are much less visible on earth than on the moon because earth’s atmosphere create weathering and erosion that wears the craters away. The very thin atmosphere on the moon, as well as the lack of weather, preserves the craters that form for long periods of time. The depth and diameter of the crater depends on the mass and velocity of the meteorite. A faster traveling or more massive meteorite will have more kinetic energy, so it will leave a larger crater [3-8].The size of a crater is naturally going to be related to the energy of the impacting object like an asteroid. As the energy of the asteroid increases, it releases more energy on impact, making a bigger crater. However, it turns out that the relationship is not linear [4]. However, the size of a crater made during an impact depends not only on the mass and velocity of the impactor, but also on the amount of kinetic energy possessed by the impacting object. Energy comes in different forms and is associated with motion is called Kinetic energy and can be calculated from the equation: KE = ½ mv2Where m is the mass of the object and v is the velocity of the object.Kinetic energy is proportional to the square of velocity, if the velocity of an object doubles then the kinetic energy increases by a factor of four. However, kinetic energy is proportional to the mass, so as the ball falls its kinetic energy increases however, it is the gravitational force that accelerates the ball causing the velocity to increase, the increase in velocity also increases the kinetic energy [5].During impact, the kinetic energy of an asteroid is transferred to the target surface, breaking up rock and moving the particles around. The mechanical energy of an object is the sum of its potential energy (PE) and kinetic energy (KE), Energy can also be associated with the gravitational force called gravitational potential energy. Asteroid and Meteorite in deep interplanetary space gain its kinetic energy (KE) and loses its potential energy (PE) as it falls toward the surface of the celestial object [6]. Since the beginning energy is all potential energy PE equals to the final energy is all kinetic energy KE at the point where the object from space smashes with a celestial body’s surface therefore, the impact velocity of an object can be calculated by conservation of energy [7].V = (2gh) ½Where h represent height and the gravitational constant g is the acceleration of the object due to gravity. This acceleration is about 9.8 m\s on earth. Impact Crater: Mechanics and its Formation:The whole impact crater process can be described as a type of explosion, in which the initial kinetic energy of the projectile does work on the target point to make up a hole called the Crater as well as heating the material of both projectile and target. However, the crater is much larger than the original projectile, the process in a high velocity impact can be divided into three main steps, these steps are Contact and Compression (Shock wave generation), Excavation Growth and Modification stage [1].i. Contact and Compression step:In this step the moving projectile makes contact with the ground surface, a shock wave propagates through the projectile and into the target. This results in a redistribution of the projectiles initial kinetic energy into kinetic and internal energy of all colliding material. The internal energy heats the material both the projectile and target. If the projectile is small, typically a few meters or less in size behave differently in passing through the atmosphere, they loss most or all of their original velocity and kinetic energy in the atmosphere through disintegration and ablation, and they strike the ground at speeds of no more than a few hundred meters per second. In such a low velocity impact, the projectile penetrates only a short distance into the target depending on its velocity and the nature of the target material, and the projectile’s momentum excavates a pit that is slightly larger than the projectile itself. The projectile survives, more or less intact and much of it is found in the bottom of the pit. Such pit, sometimes called penetration craters, are typically less than a few tens of meters in diameter [9].ii. Excavation Growth:After the contact and compression step forms in the target it expand away from the impact site, compressing and accelerating the material it encounters. The projectile is surrounded by a roughly hemispherical envelope of shock wave that expand rapidly through the target rock. Because the projectile has penetrated a finite distance into the target, the center of this hemisphere actually lies within the original target rock at appoint bellow the original ground surface. The depth and diameter of the excavation crater are determined by the properties of the target, which may include density, strength and acceleration of gravity [1, 10-12].iii. Modification stage:When the excavation stage ends the modification stage begins immediately, the modification produce the final crater shape. The immediate part of the modification stage during which the major impact related changes occur, lasts only slightly longer than the excavation stage, less than a minute for a small structure, a few minutes for a large one. However, the modification stage has no clearly marked end, and the modification process of uplift and collapse movement, isostatic uplift, erosion, and sedimentation. The mechanical style of modification depends on the gravity field of the planet and the strength of near surface rocks [1, 13-15].
2. Method of the Projects Work
In this project the material we have been using consist of two Round stone of different mass and size, Flour in a Tupperware container, Cocoa powder, small sprinkles, mud fluid, along hollow plastic tube, a tape measure, a meter stick, a ruler, Vernier calipers.We have hold the long plastic tube fixed to the vertical side with one end resting on the edge of the Tupperware container as shown in fig.1. We put a layer of sprinkles on the surface of flour is to act as a mineral diversity of the surface impacted as shown in fig. 2. Then we put a layer of a cocoa powder on the layer of sprinkles to be like a real surface of moon and planets as shown in fig. 3. We have made a mud fluid and then we put the stone on it until the stone covered slightly with a layer of viscous mud fluid is to act as a fragmentation when we drop it on the flour as shown in fig. 4. At the end of the tube we have hold the stone and drop it freely without any external forces and strikes the flour as shown in fig. 5. Then the ball skip across the flour and create an impact crater and we used tape measure to measure the distance of the ball drop before hitting the flour. Also we measure the depth, diameter of the crater and the ejecta distance for each ball drop at different height by using Vernier calipers. | Figure 1. Tape measure fixed with plastic tube to the vertical side |
 | Figure 2. A layer of Sprinkles on the surface of flour |
 | Figure 3. A layer of Coco over the surface of Sprinkles |
 | Figure 4. Round stone covered slightly with a layer of viscous mud fluid |
 | Figure 5. Multi craters formed |
3. Result and Discussion
In results, During testing balls we have been using two round stone of different mass and size, the first stone has a diameter of (23 mm) and has the mass of (26 g), the second stone has a diameter of (36.8 mm) and has the mass of (75.8 g). By using the first stone which is small, we have found that as the velocity of the ball increased the crater diameter increased. However, by using the second stone which is bigger than the first stone in mass and size also shows crater diameter increased as the velocity of the ball increased so that whenever the size and mass of the ball increased the crater diameter will increase as shown in fig. 6(a, b) respectively. However, this process is same for the crater depth as the velocity of the ball increased the crater depth increased also the increasing of crater depth depends on the mass of the object as shown in fig. 7. (a, b) respectively. Fig. 8 (a, b) shows that the line of ejecta distance increased as the velocity of the object increased.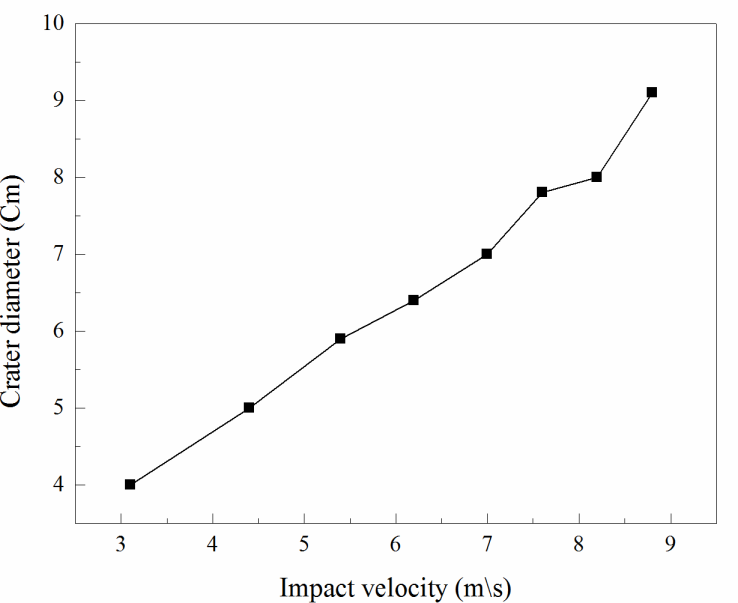 | Figure 6a. Shows the crater diameter vs. Impact velocity for the stone whose mass = 26 g |
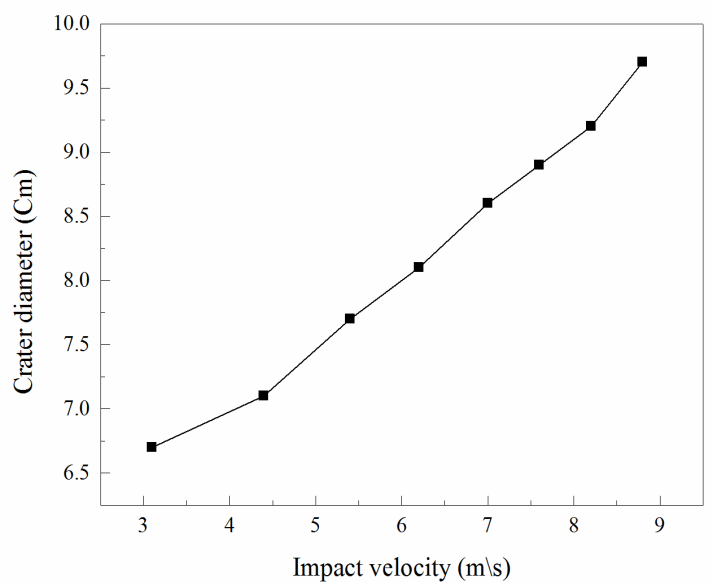 | Figure 6b. Shows the crater diameter vs. impact velocity for the stone whose mass = 75.8 g |
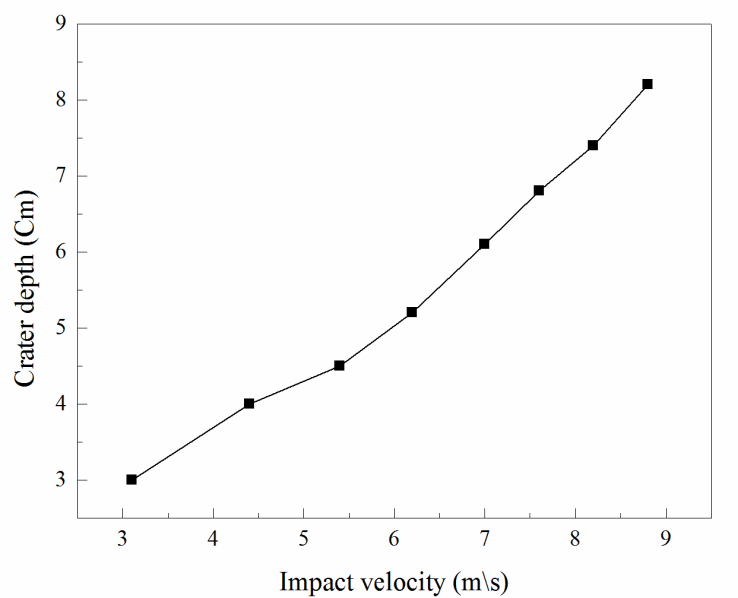 | Figure 7a. Shows the crater depth vs. impact velocity for the stone whose mass = 26 g |
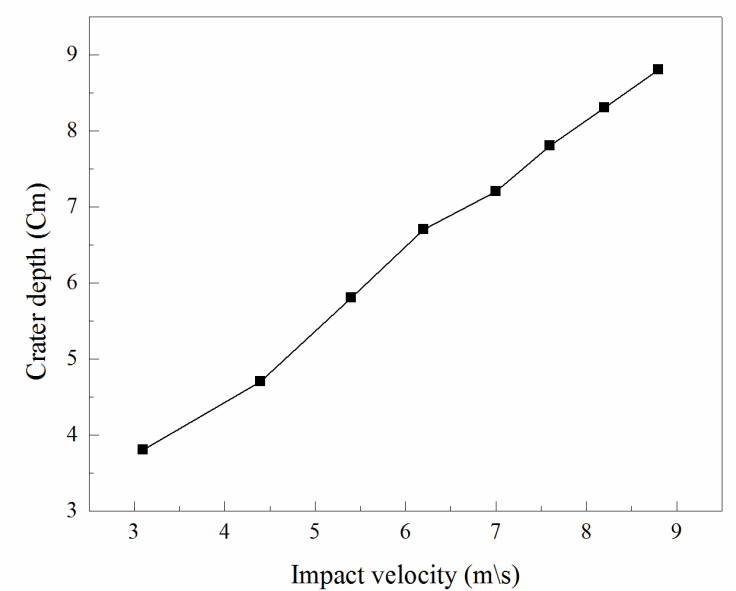 | Figure 7b. Shows the crater depth vs. impact velocity for the stone whose mass = 75.8 g |
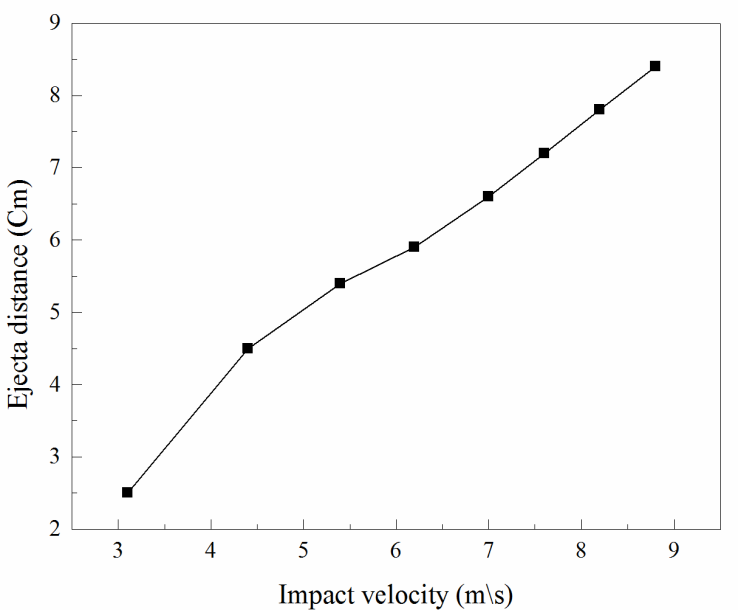 | Figure 8a. Shows the Ejecta distance vs. impact velocity for the stone whose mass = 26 g |
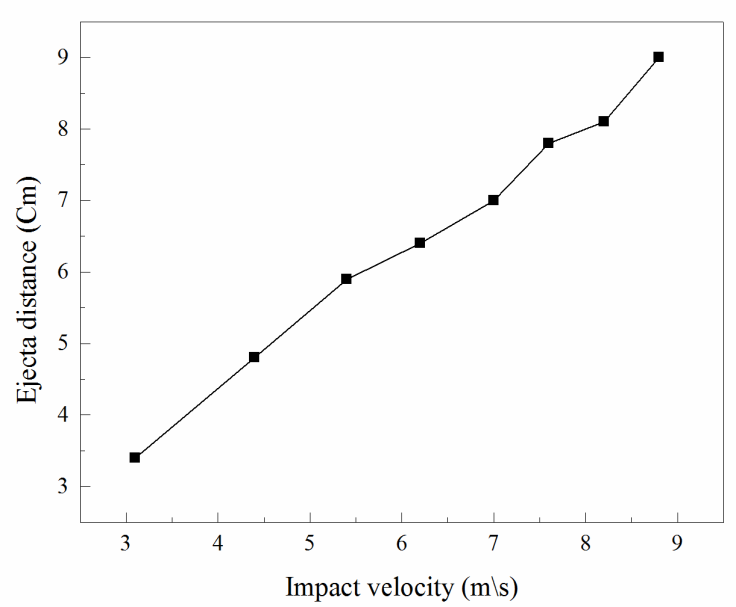 | Figure 8b. Shows the Ejecta distance vs. impact velocity for the stone whose mass = 75.8 g |
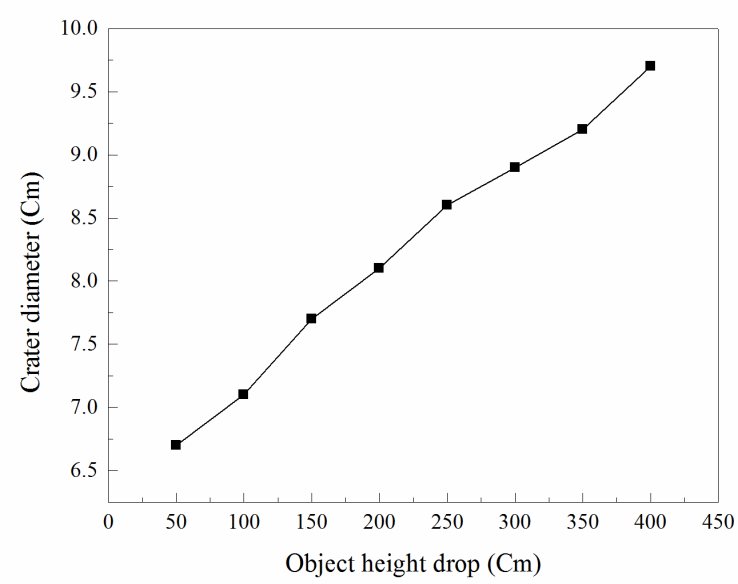
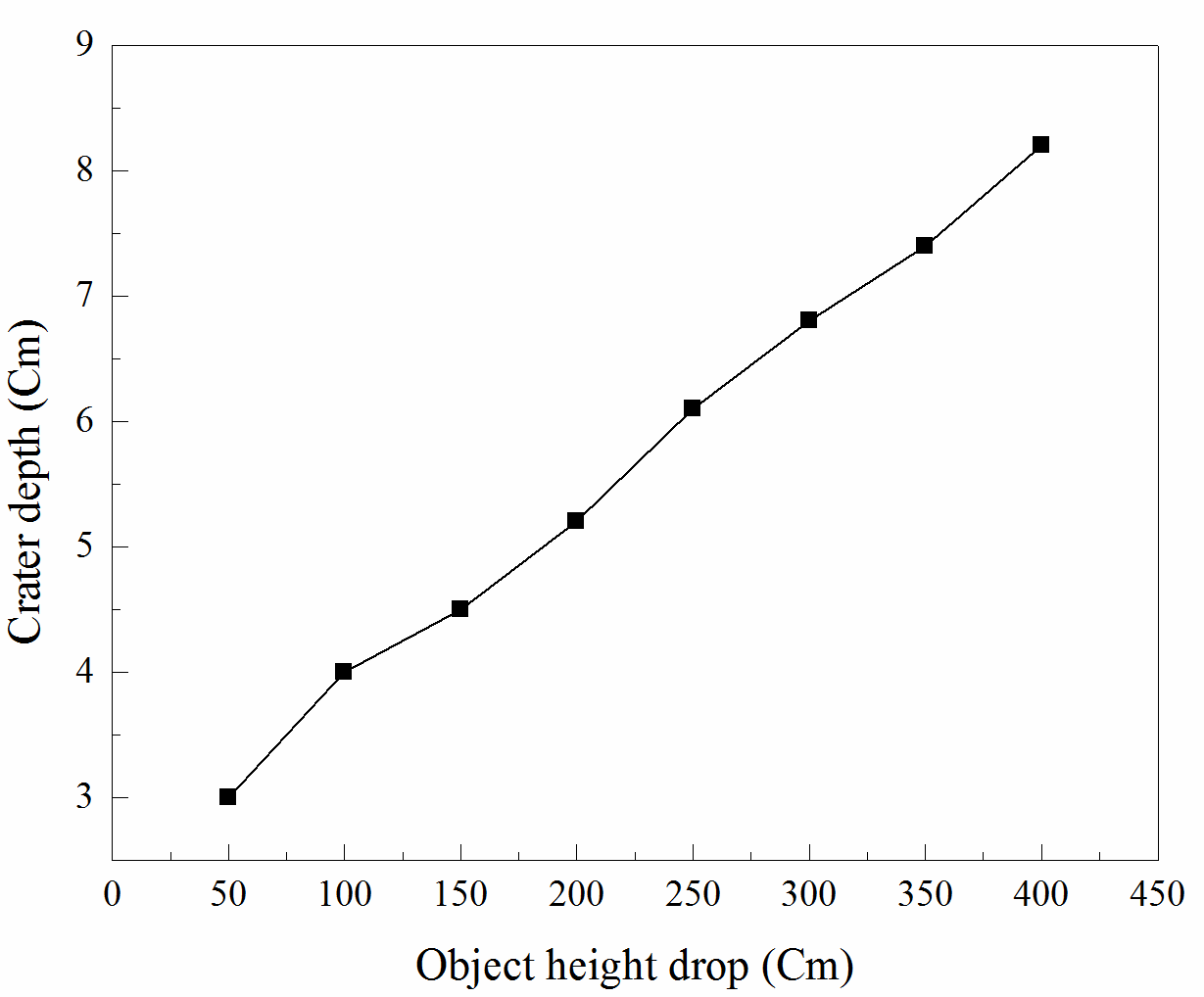
Thus as the height and the speed of the ball increased the crater diameter and the crater depth increased as shown in fig. 9, 10. In this study also we used a layer of small sprinkles on the flour is to act as a mineral diversity of the surface impacted when the ball dropped on the flour to form a crater. In general the mass, size, velocity and angle of the falling object determine the shape, size and complexity of the resulting crater. Small object with slow moving have low impact energy and cause small craters. Large object with fast moving release a lot of energy and form large, complex craters. Very large impacts can cause secondary craters as ejected material falls back to the ground forming new, smaller craters or a series of craters.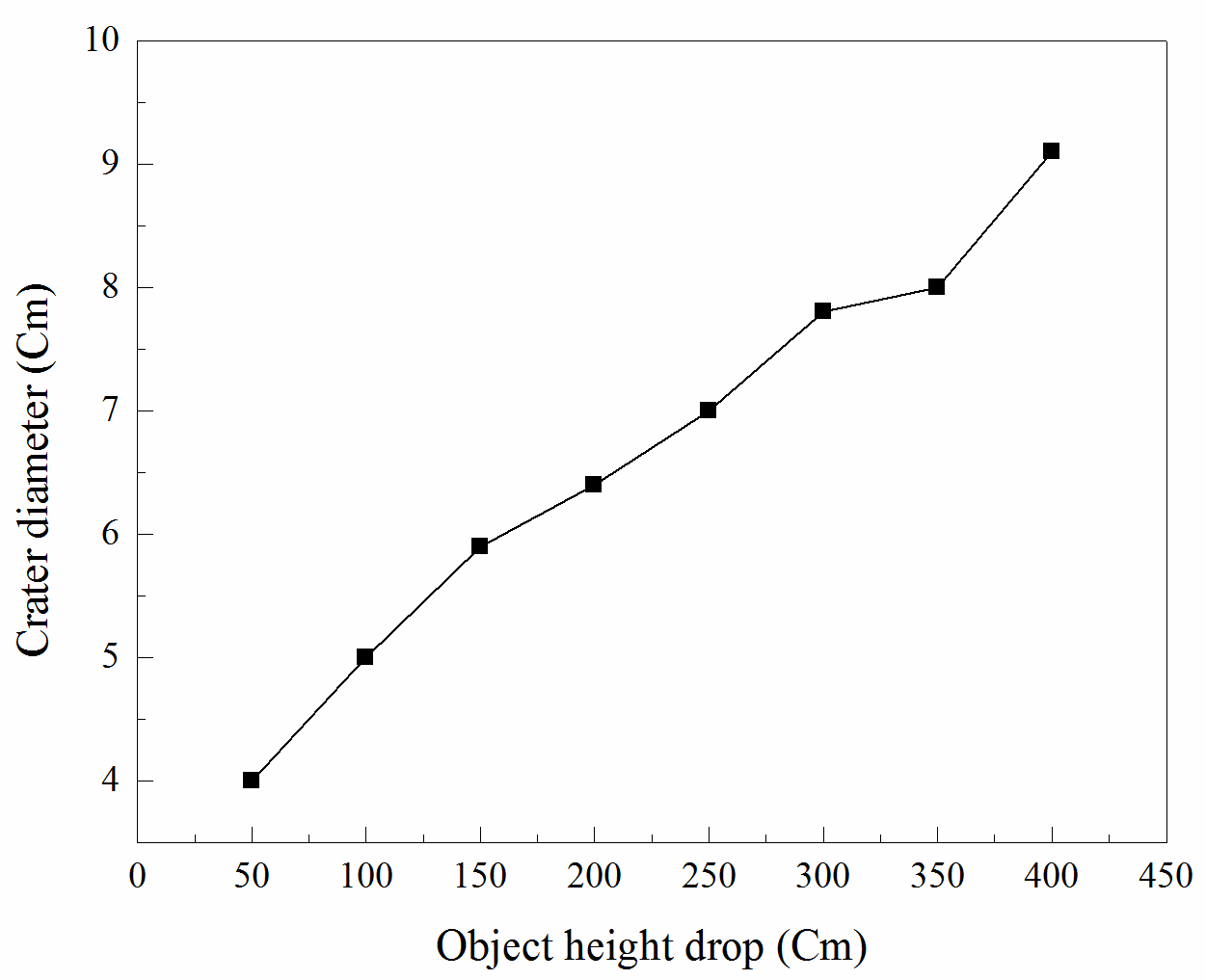 | Figure 9a. Shows the crater diameter vs. object height drop for the stone whose mass = 26 g |
 | Figure 9b. Shows the crater diameter vs. object height drop for the stone whose mass = 75.8 g |
 | Figure 10a. Shows the crater depth vs. object height drop for the stone whose mass = 26 g |
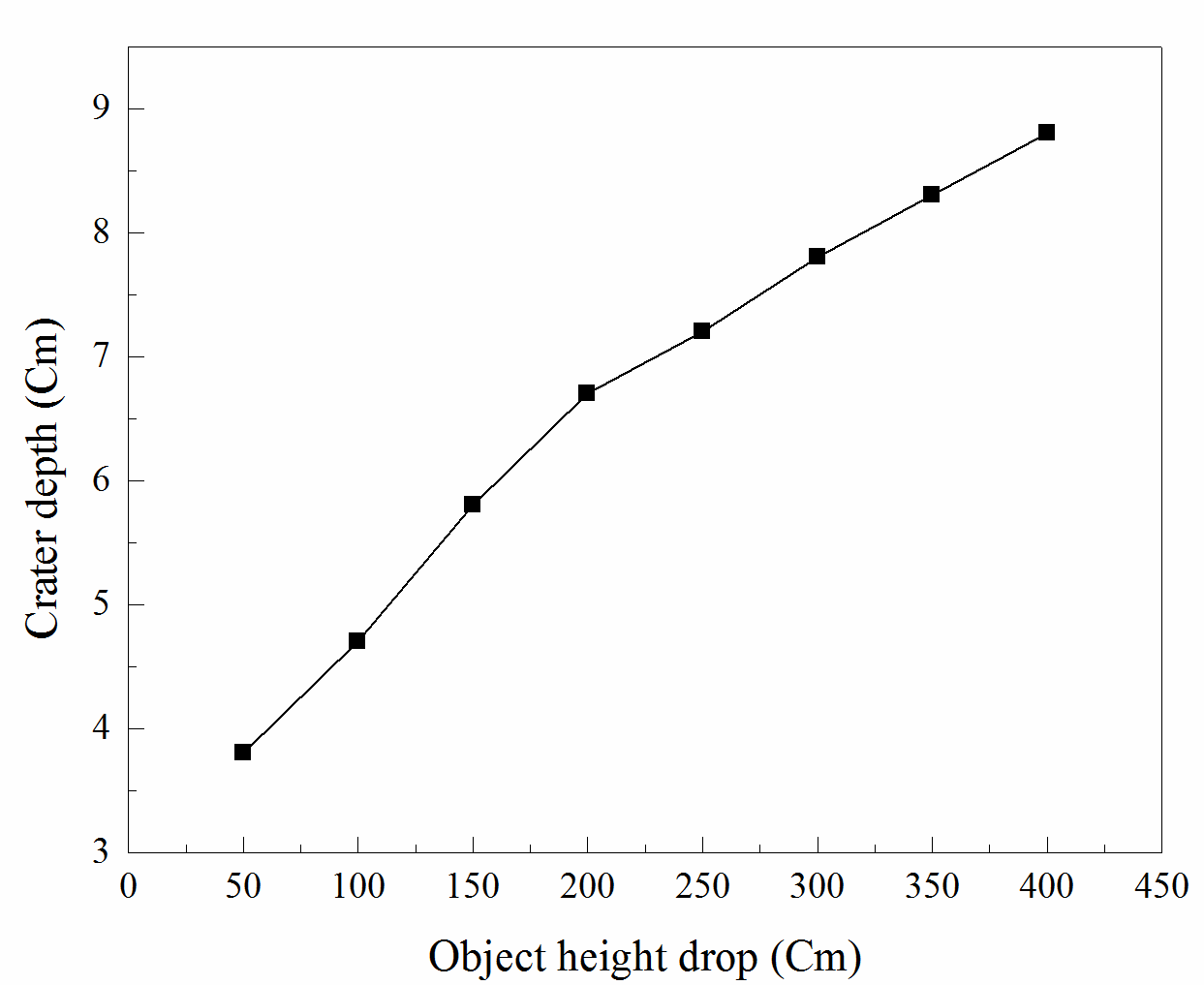 | Figure 10b. Shows the crater depth vs. object height drop for the stone whose mass = 75.8 g |
4. Conclusions
Thus far in the research project, we have been concluded that the crater diameter and the crater depth increased with increasing mass, velocity of the object in addition of increasing ejecta distance. The mass, size, velocity and angle of the falling object determine the shape, size and complexity of the resulting crater. Small object with slow moving have low impact energy and cause small craters. Large object with fast moving release a lot of energy and form large, complex craters. Very large impacts can cause secondary craters as ejected material falls back to the ground forming new, smaller craters or a series of craters. Therefore, we have come to the conclusion that the crater diameter is direct proportional to the mass and velocity of the object. Thus based on the kinetic energy equation:KE = ½ mv2By squaring velocity the object will have more effective on the crater diameter than the increasing mass of the object.
References
| [1] | Melosh HJ. Impact cratering: A geologic process. Research supported by NASA. New York, Oxford University Press (Oxford Monographs on Geology and Geophysics, No. 11), 1989, 253 p.. 1989; 1. |
| [2] | Barlow NG. A review of Martian impact crater ejecta structures and their implications for target properties. Geological Society of America Special Papers. 2005 Jan 1; 384: 433-42. |
| [3] | Neukum, G., and B. A. Ivanov. "Crater size distributions and impact probabilities on Earth from lunar, terrestrial-planet, and asteroid cratering data." Hazards due to Comets and Asteroids 359 (1994). |
| [4] | Sato, Hiroaki, and Hiromitsu Taniguchi. "Relationship between crater size and ejecta volume of recent magmatic and phreato‐magmatic eruptions: Implications for energy partitioning." Geophysical Research Letters 24, no. 3 (1997): 205-208. |
| [5] | Fudali, R. F., and W. G. Melson. "Ejecta velocities, magma chamber pressure and kinetic energy associated with the 1968 eruption of Arenal volcano." Bulletin Volcanologique 35, no. 2 (1971): 383-401. |
| [6] | Melosh HJ, Ivanov BA. Impact crater collapse. Annual Review of Earth and Planetary Sciences. 1999 May; 27(1): 385-415. |
| [7] | Shoemaker EM. 1963. Impact mechanics at Meteor Crater, Arizona. In The Moon, Meteorites and Comets, ed. BM Middlehurst, GP Kuiper, 4:301–36. Chicago, IL: Univ. Chicago Press. |
| [8] | Pike RJ. 1977. Size dependence in the shape of fresh impact craters on the Moon. See Roddy et al 1977, p. 489–509. |
| [9] | Johnson WE, Anderson CE. 1987. History and application of hydrocodes in hypervelocity impact. Int. J. Impact Eng. 5:423–39. |
| [10] | Melosh HJ. 1985. Impact cratering mechanics: Relationship between the shockwave and excavation flow. Icarus 62:339–43. |
| [11] | O’Keefe JD, Ahrens TJ. 1993. Planetary cratering mechanics. J. Geophys. Res. 98:17011–28. |
| [12] | Holsapple KA, Schmidt RM. 1982. On the scaling of crater dimensions–2. Impact processes. J. Geophys. Res. 87: 1849–70. |
| [13] | Quaide WL, Gault DE, Schmidt RA. 1965. Gravitative effects on lunar impact structures. Ann. NY Acad. Sci. 123:563–72. |
| [14] | Melosh HJ. 1979. Acoustic fluidization: A new geologic process? J.Geophys. Res. 84:7513–20. |
| [15] | Ivanov BA, Kostuchenko VN. 1997. Block oscillation model for impact crater collapse. In Lunar Planet. Sci. Conf. 27th, Abstr. 1655. |

















 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML