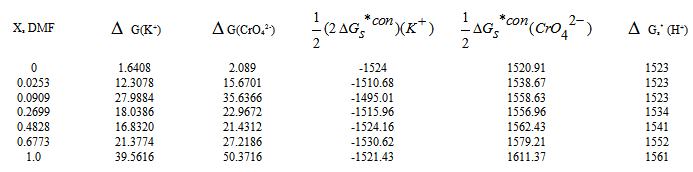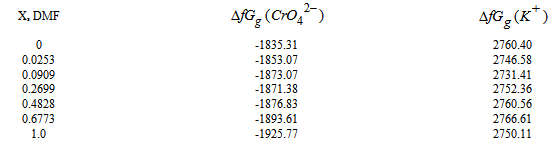-
Paper Information
- Paper Submission
-
Journal Information
- About This Journal
- Editorial Board
- Current Issue
- Archive
- Author Guidelines
- Contact Us
American Journal of Systems Science
2014; 3(1): 12-17
doi:10.5923/j.ajss.20140301.02
Thermodynamics of the Solvation of Potassium Chromate in Mixed DMF-H2O Solvents at 301.15 K
Esam A. Gomaa
Chemistry Department, Faculty of Science, Mansoura University, 35516-Mansoura, Egypt
Correspondence to: Esam A. Gomaa, Chemistry Department, Faculty of Science, Mansoura University, 35516-Mansoura, Egypt.
| Email: |  |
Copyright © 2012 Scientific & Academic Publishing. All Rights Reserved.
The Gibbs free energies for K2CrO4 were evaluated in mixed dimethylformamide (DMF)-H2O solvents at 301.15 K from the experimental solubility measurements. From the experimental solubility data also the ionic radii of potassium and chromate ions are evaluated . The total free energy of the salt is divided into its individual contribution in the mixtures used. Libration Gibbs free energy for moving K2CrO4 from standard gas state to standard solution state was calculated according to specific cycle for the solvation process using the solubility product. Also the lattice energy for solid K2CrO4 (cr) was also calculated and used for further evaluation. The conventional Gibbs free energies for the cation (K+) and the anion (CrO42-) were estimated theoretically and also the Gibbs free energy of CrO42- gas was evaluated and all values were discussed.
Keywords: Thermodynamics, Gibbs Free Energies, Potassium Chromate, Solvation, Solubility, Aqueous and Dimethylformamide Mixtures
Cite this paper: Esam A. Gomaa, Thermodynamics of the Solvation of Potassium Chromate in Mixed DMF-H2O Solvents at 301.15 K, American Journal of Systems Science, Vol. 3 No. 1, 2014, pp. 12-17. doi: 10.5923/j.ajss.20140301.02.
Article Outline
1. Introduction
- For neutral species experimental solvation Gibbs free energies have been tabulated large number of solutes in both aqueous[1-7] and non-aqueous[7, 8] solvents. Typically, these solvation free energies are determined experimentally [8] and their uncertainty is relatively low (~ 0.8 KJ/mol)[9].Determining accurate values for the Gibbs free energies of ionic solutes like K2CrO4 is important than that of neutral solutes. Understanding the partitioning of single ions between different liquid phases is important in many areas of biology. For example, the electrical signals sent by nerve cells are activated by changes in cell potential that are caused by the movement of various ions across the neuronal membrane[10]. The division of thermodynamic Gibbs free energies of solvation of electrolytes into ionic constituents is conventionally accomplished by using the single ion solvation Gibbs free energy of one reference ion, conventionally, the proton, to set the single ion scales[11, 12]. The aim of this work is to estimate the single ion Gibbs free, energies for K+ & CrO42- ions in mixed DMF H2O solvents at 301.15 K. Sums of solvation free energies of cations and anions are well defined through the use of thermochemical cycles involving calorimetric or electrochemical measurements [13-17]. A number of different extra thermodynamic approximations have been used[18-25] for partition the sums of cation and anion Gibbs free energies into single ion contribution. Solvation Gibbs free energies for single ions are necessary for theoretical calculations.
2. Relative and Conventional Solvation Free Energies of Ions
- The Gibbs solvation free energies of ions as relative free energies by settling the free energy of solvation of some reference ion equal zero[26]. Proton was chosen as reference ion. For ions, this result in a set of conventional free energies of solvation that the cations are shifted from their absolute values by the value for the absolute Gibbs solvation free energy of the proton. The conventional Gibbs free energies of solvation for anions are shifted by an equal amount in the opposite direction.
3. Conventional Gibbs Free Energies from Reduction Potentials
- When the convention for the absolute Gibbs free energy of the proton is followed, the solution-phase free energy change associated with the half cell for reduction of hydrogen gas is equal to zero. Reduction potentials following this convention for hydrogen electrode are referred as standard reduction potentials. From the half cell reaction for the reduction of metal cation to crystalline phase and the half reduction reaction of hydrogen gas, the redox reaction can be illustrated through the use of thermochemical cycle[12]. This last procedure can be used to estimate the gas free energy of formation for CrO42- ion, to explain the ionic behaviour.
4. Experimental
- Potassium chromate K2CrO4 Griffinkamp and N-N, dimethylformamide (DMF) from Merck Co, were used. Saturated solutions of K2CrO4 were prepared by dissolving different amounts of the salt in closed test tubes containing different DMF-H2O mixtures. These mixtures were then saturated with nitrogen gas as inert atmosphere. The tubes were placed in a shaking thermostat (Model Gel) for a period of four days till equilibrium reached. The solubility of K2CrO4 in each mixture was measured gravimetrically by evaporating 1 ml of the saturated solution in small cup using I. R. lamp. The measurements were done by three readings for each solution at 301.15 K.
5. Results and Discussion
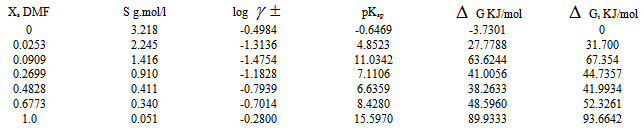
- The molar solubility (S) for K2CrO4 was measured gravimetrically in mixed DMF-H2O solvent at 301.15 K. The solubility values with an average number of the second number after comma are cited in Table (1). These (S) value in water agreed well with that in literature values[27]. The activity coefficients were calculated by the use of Debye-Hückel equation[28, 29].
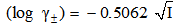 | (1) |
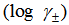 were tabulated also in Table (1). The solubility product was calculated by the use of equation 2[30].
were tabulated also in Table (1). The solubility product was calculated by the use of equation 2[30]. | (2) |
 | (3) |
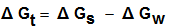 | (4) |
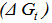 increase in negativity by increasing the mole fraction of DMF in the mixed DMF-H2O solvents. This is due to more solvation behaviour in the mixed solvents than that of water (see Fig. 1).
increase in negativity by increasing the mole fraction of DMF in the mixed DMF-H2O solvents. This is due to more solvation behaviour in the mixed solvents than that of water (see Fig. 1). 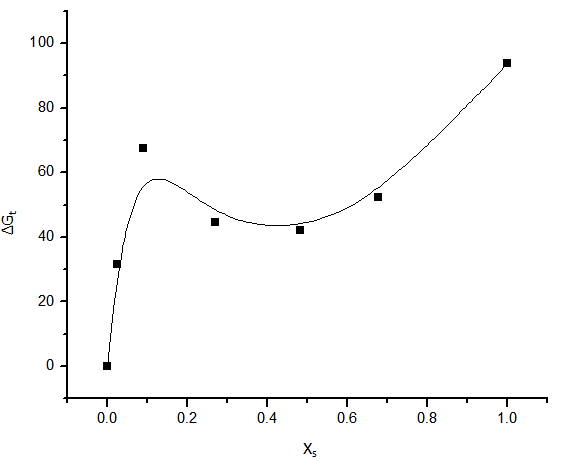 | Figure 1. Gibbs free energies of transfer for K2CrO4 versus the mole fraction of DMF at 301.15 K |
|
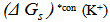 for potassium ion in solvents are shifted from their absolute values by the absolute free energy of the proton[34] according to equation (5).
for potassium ion in solvents are shifted from their absolute values by the absolute free energy of the proton[34] according to equation (5).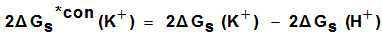 | (5) |
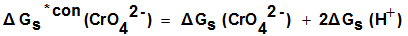 | (6) |
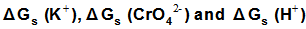 are the Gibbs free energies of solvation for potassium, chromate and proton in solvents. From the mean values of Gibbs free energies for the proton in water and other solvents given in literature[12, 35, 36], a straight line was drown between these mean proton values and the diameter for each solvent taken from[37]. From this line the proton solvation free energies in pure water and DMF were obtained and found to be 1523 and 1561 K J/mol, respectively. Multiplying these values by the mole fraction of each solvent and then sum the results. The mixed solvent proton free energies in DMF-H2O mixtures were obtained and their values are given in Table (2). Apply equations (5) and (6) we get the conventional Gibbs free energies for the cation K+ and the anion CrO4- , their values are given also in Table (2). Cation conventional free energy values are negative indicating exothermic character and anion values are positive indicating endothermic character. Both values conventional Gibbs free energies for potassium and chromate ions increase with increase of the mole fraction of DMF due to more solvation and the sum of them gives the values for the neutral salt.
are the Gibbs free energies of solvation for potassium, chromate and proton in solvents. From the mean values of Gibbs free energies for the proton in water and other solvents given in literature[12, 35, 36], a straight line was drown between these mean proton values and the diameter for each solvent taken from[37]. From this line the proton solvation free energies in pure water and DMF were obtained and found to be 1523 and 1561 K J/mol, respectively. Multiplying these values by the mole fraction of each solvent and then sum the results. The mixed solvent proton free energies in DMF-H2O mixtures were obtained and their values are given in Table (2). Apply equations (5) and (6) we get the conventional Gibbs free energies for the cation K+ and the anion CrO4- , their values are given also in Table (2). Cation conventional free energy values are negative indicating exothermic character and anion values are positive indicating endothermic character. Both values conventional Gibbs free energies for potassium and chromate ions increase with increase of the mole fraction of DMF due to more solvation and the sum of them gives the values for the neutral salt.
|
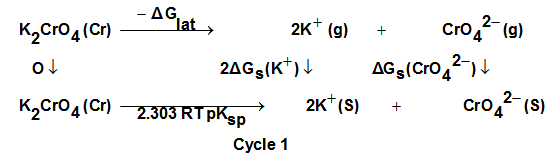 Where
Where 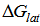 is the lattice free energy, (g) and (s) denote the gas and solution cases. The lattice energy was calculated following Bartlett’s relationship following equation (7)[38-41].
is the lattice free energy, (g) and (s) denote the gas and solution cases. The lattice energy was calculated following Bartlett’s relationship following equation (7)[38-41].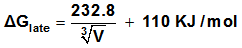 | (7) |
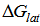 for K2CrO4.On the use of equation (8) cycle (1), the liberation free energy for K2CrO4was obtained (99.468 KJ/mol).
for K2CrO4.On the use of equation (8) cycle (1), the liberation free energy for K2CrO4was obtained (99.468 KJ/mol). 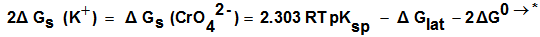 | (8) |
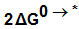 , the free energy change associated with moving K2CrO4 from standard gas phase at 1 atmosphere to solution phase. This free energy change has been referred as “compression” work of the gas or liberation free energy.Conventional free energies from reduction potentials: The absolute Gibbs free energy of the proton is followed solution phase free energy change associated with the following half cell.
, the free energy change associated with moving K2CrO4 from standard gas phase at 1 atmosphere to solution phase. This free energy change has been referred as “compression” work of the gas or liberation free energy.Conventional free energies from reduction potentials: The absolute Gibbs free energy of the proton is followed solution phase free energy change associated with the following half cell. 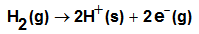 | (9) |
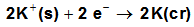 | (10) |
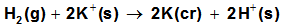 | (11) |
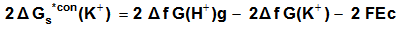 | (12) |
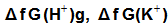 are the gas free energy of formation for H+ and K+ ions. F faraday’s constant, equal = 23.061 Kcal/mol and Ec is the standard reduction potential of K+.
are the gas free energy of formation for H+ and K+ ions. F faraday’s constant, equal = 23.061 Kcal/mol and Ec is the standard reduction potential of K+. 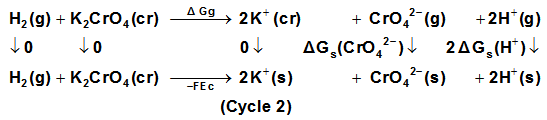 Also the conventional free energy of the chromate ion can be written following Truhlar[ 12 ] explanation as:
Also the conventional free energy of the chromate ion can be written following Truhlar[ 12 ] explanation as: 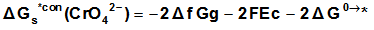 | (13) |
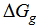 , gas free energies of formation for the anion CrO42- and K+ estimated in the mixed DMF H2O solvents and their values are given in Table (3) and Fig. (2). The
, gas free energies of formation for the anion CrO42- and K+ estimated in the mixed DMF H2O solvents and their values are given in Table (3) and Fig. (2). The 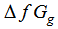 values increase by increase of the mole fraction of DMF favoring less solvation.
values increase by increase of the mole fraction of DMF favoring less solvation.
|
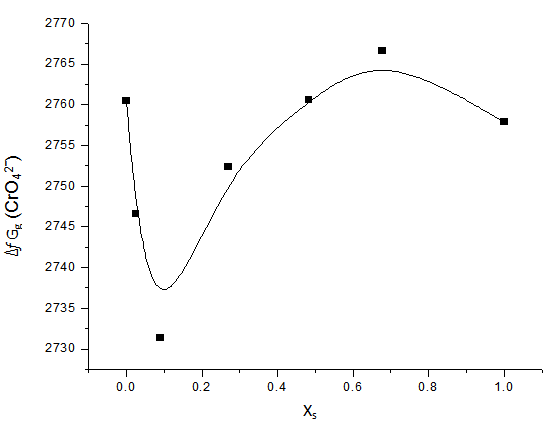 | Figure 2. Relation between against the mole fraction of DMF at 301.15 K |
6. Conclusions
- By the use of combination of experimental gas-phase free energies of formation and solution-phase reduction potentials, we determined conventional solvation free energies of K2CrO4 in mixed DMF-H2O solvents at 301.15 K from the experimental solubility measurements. Libration Gibbs free energy associated with moving K2CrO4 in standard gas state to standard stat in solution was evaluated according to thermochemical cycle for the solvation process using the solubility product. Also the lattice energy for solid K2CrO4 (cr) was also calculated and used for further evaluation. These conventional solvation free energies were then combined with experimental and calculated gas-phase clustering free energies to determine conventional solvation free energies of ion-solvent clusters containing up to solvent molecules. The values for the absolute solvation free energy of the proton obtained in this work should be useful as standards against which the absolute solvation free energies of other single ions can be derived.
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML