-
Paper Information
- Next Paper
- Paper Submission
-
Journal Information
- About This Journal
- Editorial Board
- Current Issue
- Archive
- Author Guidelines
- Contact Us
American Journal of Operational Research
p-ISSN: 2324-6537 e-ISSN: 2324-6545
2015; 5(1): 1-8
doi:10.5923/j.ajor.20150501.01
Inventory Flow in Supply Chain with Deteriorating Items for Customers in Queue: Computation of Profit Optimization in Fuzzy Environment
Mishra S. S., Yadav S. K., Rawat S.
Department of Mathematics & Statistics (Centre of Excellence on Advanced Computing), Dr. R. M. L. Avadh University, Faizabad, India
Correspondence to: Mishra S. S., Department of Mathematics & Statistics (Centre of Excellence on Advanced Computing), Dr. R. M. L. Avadh University, Faizabad, India.
| Email: |  |
Copyright © 2015 Scientific & Academic Publishing. All Rights Reserved.
The present paper makes a fresh attempt to discuss the inventory flow in supply chain with deteriorating items for customers in queue and its profit optimization in fuzzy environment by using computational approach. The system has been fuzzified by using Zadeh’s extension principle and triangular and defuzzified by centroid method. Consequently, by applying optimization technique, a system of non-linear equation has been developed and it has been solved by using fast converging Newton Raphson’s (N-R) method. A sensitivity analysis has been also carried out to gain deeper insight into the model under consideration.
Keywords: Customers in Queue, Inventory Flow and Supply Chain, System of Nonlinear Equation, Profit Optimization in Fuzzy Environment
Cite this paper: Mishra S. S., Yadav S. K., Rawat S., Inventory Flow in Supply Chain with Deteriorating Items for Customers in Queue: Computation of Profit Optimization in Fuzzy Environment, American Journal of Operational Research, Vol. 5 No. 1, 2015, pp. 1-8. doi: 10.5923/j.ajor.20150501.01.
Article Outline
1. Introduction
- All possible channels involved in acquiring raw materials, manufacturing of products, storing and distributing of inventory items to end-customers form the basis of supply chain management. Inventory items flowing in supply chain network are also elaborated by men, materials, machines, money and method to optimize the cost of inventory control system, vide for example Ackoff and Sasieni (1993), Chopra et al. (2001), Lam and Ip (2010), Ganeshan et al. (1995) and Sharma (2012).Each production system undergoes the process of deterioration of inventory items. A model for deteriorating items for optimal ordering policy with power from stock dependent demand over two-warehouse storage facility was developed by Singh et al. (2010). Mishra & Mishra (2008) discussed an economic order quantity (EOQ) model for deteriorating items under perfect competition with price determination. Mishra and Singh (2011) proposed a model for deteriorating items with power form stock-dependent demand by considering cubic deterioration in inventory. Further, Mishra and Mishra (2012) attempted an economic order quantity (EOQ) model for queued customers with normal life time of inventory flowing in supply chain. An economic order quantity (EOQ) model with quadratic deterioration items allowing shortages for computing the total optimal cost has been analyzed by Mishra and Singh (2012). A computational approach was developed by Mishra and Singh (2012) to provide the optimal cost of the inventory model for ramp-type demand and linear deterioration. Mishra and Singh (2013) analyzed and an inventory model with queued customers and power demand and quadratic deterioration under partial backlogging. An ordering policy for deteriorating inventory for two ware houses with power form stock dependent demand has been developed by Singh and Singh (2013). Some more researches can be reviewed in this area such as Mishra et al. (2012), Roy and Maiti (2009) and Mishra (2014).Zadeh (1965) introduced the concept of fuzzy set and its application. Belloman and Zadeh (1970) developed a model for decision-making to be made in the case of fuzzy approach. Fuzzy set was ushered in by Zadeh (1978) to propound the theory of possibility. Lengari (2005) has attempted to deal with fuzzy logic intelligence control system of information. Zadeh (2001) conceptualized the fuzzy set and developed its applications. Mahata and Goswami (2007) investigated an economic order quantity (EOQ) model for deteriorating items over trade credit financing in the fuzzy sense. Pathak and Mondal (2013) considered an economic order quantity (EOQ) model for random Weibull deterioration with ramp-type demand, partial backlogging and inflation under trade credit financing in the fuzzy sense. Roy and Maity (2008) proposed an inventory model with remanufacturing. Mishra and Mishra (2011) investigated an inventory model for deterioration item under cobweb phenomenon and permissible delay in payment for fuzzy environment. A technique of possibility and necessity approach was developed by Pathak and Sarkar (2012) for fuzzy production model of deteriorating inventory allowing shortage subject to time dependent learning and forgetting. Prasath and Seshaiah (2013) discussed an inventory model allowing shortage for fuzzy production distribution. Pattnaik (2013) investigated an economic order quantity (EOQ) model for deteriorating items which includes promotional effort cost, variable ordering cost and units lost due to deterioration. Schwarz et al. (2006) derived stationary distribution of joint queue length and inventory process in explicit product form for various M/M/1 systems with inventory under continuous review and different inventories management policies with lost sales. Cachon and Zhang (2007) discussed a queueing model in which two strategic servers based on their performance; the faster a server works, the more demand the server is allocated. The buyer’s objective is to minimize the average lead time received from servers. Mishra and Yadav (2008) dealt with profit optimization of a loss queuing system with the finite capacity and computed total expected cost, total expected revenue and total optimal profit of the system. Mishra and Mishra (2012) discussed phase wise supply chain model inventory with normal life time for customers who are in queue.In this paper, we attempt to discuss profit optimization of deteriorated inventory flow in supply chain for queued customers in fuzzy environment. A system of non-linear equation has been developed by using Zadeh’s extension principle and its solution has been computed by using fast converging Newton Raphson’s (N-R) method. A sensitivity analysis has been also presented to demonstrate the use of the model under consideration. The paper has been divided into sections such as introduction, notations, mathematical formulation, mathematical formulation of fuzzification and its analysis, computing algorithm, sensitivity analysis and conclusion.
2. Notations
- We use the following notations throughout the paper:• P: profit per item during time•
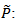 profit per item in fuzzification •
profit per item in fuzzification • 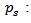 the selling price per unit item•
the selling price per unit item• 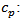 the purchasing cost per unit item•
the purchasing cost per unit item• 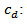 the deterioration cost per unit deteriorated item•
the deterioration cost per unit deteriorated item• 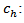 the holding cost per unit per unit time•
the holding cost per unit per unit time• 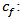 the fixed replenishment cost•
the fixed replenishment cost• 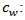 cost of one customer waiting in queue for an hour •
cost of one customer waiting in queue for an hour • 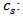 hourly cost per server in queue• N: number of servers•
hourly cost per server in queue• N: number of servers• 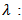 arrival rate of the customers•
arrival rate of the customers• 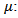 service rate served per unit time• q: the replenishment quantity per replenishment•θ: deteriorating rate during production•
service rate served per unit time• q: the replenishment quantity per replenishment•θ: deteriorating rate during production• 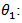 lower value of deterioration•
lower value of deterioration• 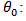 middle value of deterioration•
middle value of deterioration• 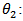 upper value of deterioration• α: size parameter• β: demand parameters• T: the length of replenishment cycle• I(t): the inventory level at time t• HC: total holding cost of the items • DI: total Deteriorating items• DC: deteriorating cost of the items• SI: total selling cost of the items• Z[A,P]: total average profit
upper value of deterioration• α: size parameter• β: demand parameters• T: the length of replenishment cycle• I(t): the inventory level at time t• HC: total holding cost of the items • DI: total Deteriorating items• DC: deteriorating cost of the items• SI: total selling cost of the items• Z[A,P]: total average profit 3. Mathematical Formulation
- If q is retailer ordered quantity in each of the replenishment. The inventory level decreases due to the combined effect the demand and the deterioration in the interval [0, T]. Then according to Singh and Singh (2013), we can have
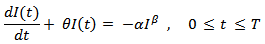 with boundary condition I (0)=q, and we have I (T) = 0, on solving the above equation, we get
with boundary condition I (0)=q, and we have I (T) = 0, on solving the above equation, we get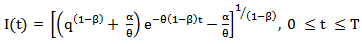 Using the condition
Using the condition 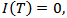 we have
we have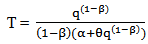 Holding cost of the items is
Holding cost of the items is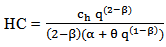 Total number of deteriorated items is
Total number of deteriorated items is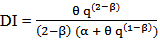 Deteriorating cost is
Deteriorating cost is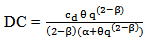 Therefore, total number of sold items is
Therefore, total number of sold items is 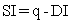
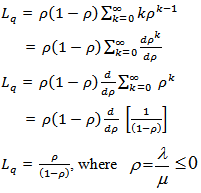 Average total profit of single-warehouse system is given as
Average total profit of single-warehouse system is given as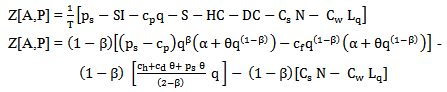 We have the following total average profit as below
We have the following total average profit as below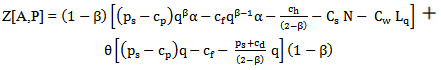 | (3.1) |
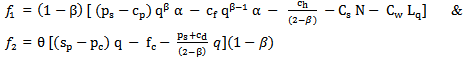 | (3.2) |
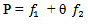 where, θ is rate of deterioration.
where, θ is rate of deterioration. 4. Mathematical Formulation of Fuzzification
- In case of fuzzification, deteriorating rate θ convert in to fuzzified deteriorating rate
 which gives the following equation
which gives the following equation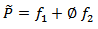 | (4.1) |
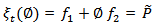 and let traingular membership funtion of fuzzified dereriotion rate
and let traingular membership funtion of fuzzified dereriotion rate 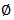 be given as:
be given as: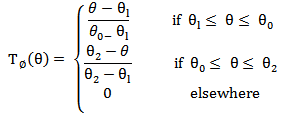 where θ1, θo & θ2 are the +ve variables and
where θ1, θo & θ2 are the +ve variables and 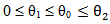
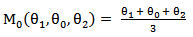 We further observe that
We further observe that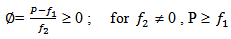 This shows that
This shows that 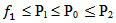 where Pl, P0, P2 are lower, middle and upper profit at time t.From above equation, we can express membership function for profit at time t as
where Pl, P0, P2 are lower, middle and upper profit at time t.From above equation, we can express membership function for profit at time t as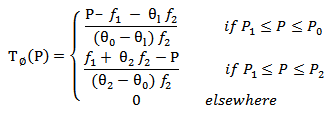 As we know that the extension principle of Zadeh is very important tool in the fuzzy set theory for providing procedure to fuzzify a crisp function which is given below.After applying Zadeh’s extension principle, we get
As we know that the extension principle of Zadeh is very important tool in the fuzzy set theory for providing procedure to fuzzify a crisp function which is given below.After applying Zadeh’s extension principle, we get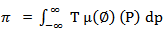 and
and 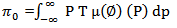 The centroid to
The centroid to 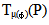 is given by
is given by 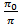 which gives the total profit in fuzzified system. We have
which gives the total profit in fuzzified system. We have 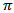 and
and 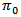 as
as 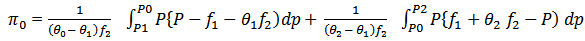
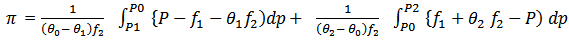 After evaluating integral of
After evaluating integral of 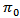 and
and 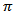 from the above equations, we get the centroid of
from the above equations, we get the centroid of 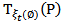 as
as 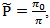 which finally turns out to be
which finally turns out to be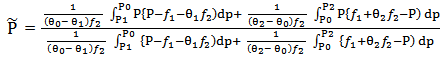
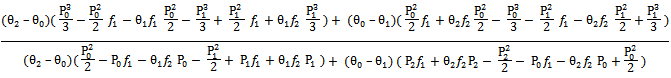 For obtaining optimal solution of
For obtaining optimal solution of 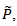 for total profit of the model, we take first order partial derivatives w. r. t. θ1 and θ2 finding the middle value θ0 as constant and equating them to zero with the condition of
for total profit of the model, we take first order partial derivatives w. r. t. θ1 and θ2 finding the middle value θ0 as constant and equating them to zero with the condition of 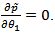 Then, we have
Then, we have 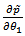 as
as 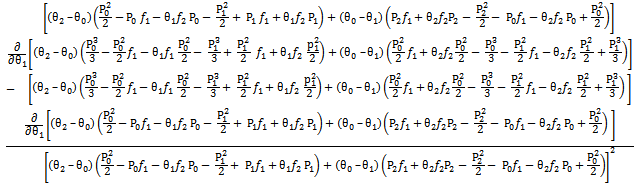 Similarly, we can differentiate w. r. t.
Similarly, we can differentiate w. r. t. 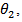 we have
we have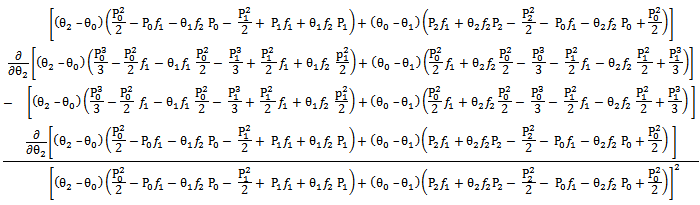 After solving the both partial derivative with respect to θ1 and θ2 for fixed values of θ0 and t, we get a system of non-linear equations of θ1 and θ2 respectively as follows:
After solving the both partial derivative with respect to θ1 and θ2 for fixed values of θ0 and t, we get a system of non-linear equations of θ1 and θ2 respectively as follows: 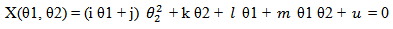 | (4.2) |
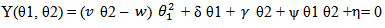 | (4.3) |
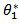 and
and 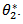
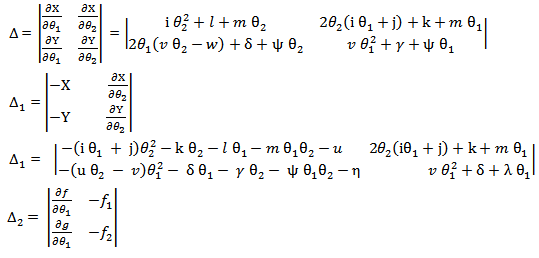
5. Computing Algorithm
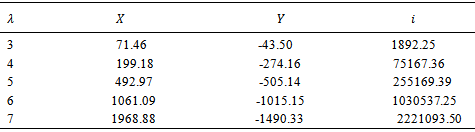
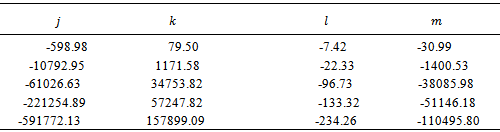
- We use the following algorithm with C++ language to compute the optimal results. Step 1: Begin.Step 2: Input data.Step 3: Compute the value of X, Y, i, j, k, l, m, ψ, η, μ, u, v, ϒ, δ.Step 3: Compute the lower profit.Step 4: Compute the middle profit.Step 5: Compute the upper profit.Step 6: Compute the coefficient of first non-linear equation by Jacobi method.Step 7: Compute the coefficient of second non-linear equation by Jacobi method.Step 8: Compute optimal value of theta one.Step 9: Compute optimal value of theta two. Step 10: Compute optimal value of profit in fuzzy case.Step 11: End
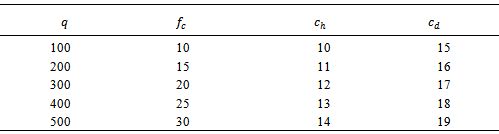
6. Sensitivity Analysis of the Model
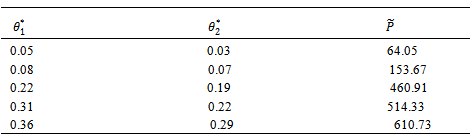
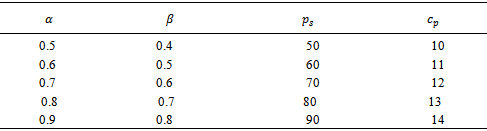
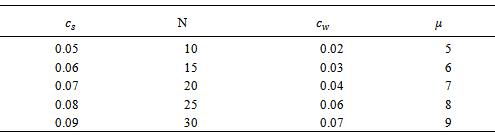
- A sensitivity analysis seeks to reveal the variational propensity of the model based on the various parameters of the performance measures involved in the study of the model. Optimal performance measure depends on various parameters involved in the model and change of one parameter depends on another, this inclusive approach of variation is altogether forms the basis of sensitivity analysis of the model.In the present problem, data-input and its impact on the findings of the model are reflected by sensitivity analysis. Various parameters are subject to computation and placed through tables. Observations related to the sensitivity analysis of the model under consideration are presented. Table (1) shows that the selling price and purchasing cost increase as shape and size parameters increase constantly. It evinces simple positive correlation between them in the fuzzy environment. It is evident from the table (2) that holding cost as well as deteriorating cost increases as size of item and cost function increase leading to simple positive correlation between them when environment is fuzzy. In tables (3), (4), (5) and (7), values of all parameters are computed in order to produce the optimal values of deterioration of items and their profits given in table (8). A close observation sighted on table (8) exhibits the optimal deterioration and corresponding optimal price for the model.
|
|
|
|
|
|
|
|
7. Conclusions
- The results reported in the paper are by product of interface of fuzzified inventory system and computational technique applied in the problem. Observations are well articulated in the sensitivity analysis which is endowed with an opportunity to discuss the performance measure as fuzzified profit of the system given in the table (8). This projects a very concrete base to analyze any enterprising system using supply chain and its strategic plans.Zadeh’s extension principle and system of non-linear system of equations have interminglingly formed the basis for the optimal solutions given in the table (8). Any robust supply chain management needs to frame appropriate building blocks of solvable model or system and solution technique as considered in the present problem. The results computed through tables (1)-(7) are obtained by applying Newton Raphson’s (N-R) technique with its suitable computing algorithm. For future research, present problem may be extended for multi-ware house and classified customers.
ACKNOWLEDGMENTS
- The work is supported by the grant of a research project of National Board for Higher Mathematics, Bombay, India (vide Ref. No. 2/48(6)/2012/NBHM/R&D II/13614, December 29, 2012).
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML
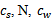 and
and 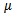 with respect to
with respect to 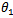 and
and 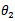
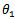 and
and 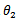
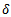 of the model
of the model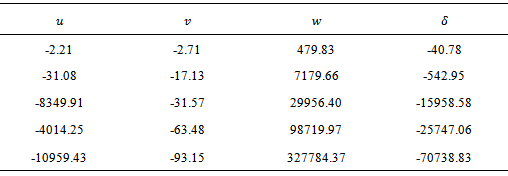
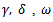 and
and 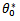 of the model
of the model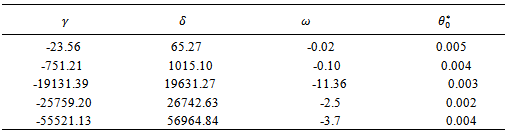
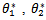 and
and 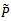 of the model
of the model