| [1] | Sabareesh GR, Matsui M, Tamura Y. Dependence of surface pressures on a cubic building in tornado like flow on building location and ground roughness. Journal of wind engineering and industrial aerodynamics. 2012 Apr 1;103:50-9. |
| [2] | Refan M, Hangan H, Wurman J. Reproducing tornadoes in laboratory using proper scaling. Journal of Wind Engineering and Industrial Aerodynamics. 2014 Dec 1;135:136-48. |
| [3] | Liu, Z. and Ishihara, T., 2016. Study of the effects of translation and roughness on tornado-like vortices by large-eddy simulations. Journal of Wind Engineering and Industrial Aerodynamics, 151, pp.1-24. |
| [4] | Razavi A, Ashrafizadeh A. Numerical Investigation on Damage Patterns of Low Swirl Translating Tornadoes. MME. 2014; 14 (6): 81-90 URL: http://journals.modares.ac.ir/article-15-6936-en.html. |
| [5] | Razavi A, Sarkar PP. Laboratory investigation of the effect of tornado translation on its near-ground flow field. Laboratory investigation. 2016 Jun 7. |
| [6] | Razavi A, Sarkar PP. Laboratory Study of Topographic Effects on the Near-surface Tornado Flow Field. Boundary-Layer Meteorology. 2018 Mar:1-24. |
| [7] | Haghighat, A.K., Roumi, S., Madani, N., Bahmanpour, D. and Olsen, M.G. 2018. An intelligent cooling system and control model for improved engine thermal management. Applied Thermal Engineering, 128, pp.253-263. |
| [8] | Razavi A, Sarkar PP. Tornado-induced wind loads on a low-rise building: Influence of swirl ratio, translation speed and building parameters. Engineering Structures. 2018 Jul 15;167:1-2. |
| [9] | Razavi A, Sarkar PP. Laboratory investigation of the effects of translation on the near-ground tornado flow field. Wind and Structures. 2018; 26(3): 179-190. |
| [10] | Eckert, E.R.G. (1960), Analyses of Heat and Mass Transfer, McGraw-Hill, New York, NY. |
| [11] | Ozisik, O.M., (1985), Heat Transfer a Basic Approach, McGraw-Hill, New York, NY. |
| [12] | Ramezani, M., Legg, M.J., Haghighat, A., Li, Z., Vigil, R.D. and Olsen, M.G., 2017. Experimental investigation of the effect of ethyl alcohol surfactant on oxygen mass transfer and bubble size distribution in an air-water multiphase Taylor-Couette vortex bioreactor. Chemical Engineering Journal, 319, pp.288-296. |
| [13] | Nagendra. H.R., Tirunarayan, M.A., and Ramachandran, A. (1969), “Free convection heat transfer from vertical cylinder and wires”, Chemical Engineering Science, Vol. 24, pp. 1491-5. |
| [14] | McEligot, D.M., Stoots, C.M., Chritenson, W.A., Mecham, D.C. and Lussie, W.G. (1993), “Turbulent natural convection from a vertical cylinder to an array of cooled tubes”, Journal of heat transfer, Transactions of the ASME Series C, Vol. 115. pp. 928-37. |
| [15] | Keyhani, M., Kaluchi, A. and Christensen, R.N. (1983), “Free convection in a vertical annulus with constant heat flux on the inner wall”, Journal of heat transfer, Transactions of the ASME Series C, Vol. 105. pp. 454-9. |
| [16] | Rogers, B.B. and Yao, L.S. (1993), “Natural convection in a heated annulus”, International Journal of Heat and Mass Transfer, Vol. 36, pp. 35-47. |
| [17] | Schneider, S. and Strub, J. (1992), “Laminer natural convecyion in a cylindrical enclosure with different and temperatures”, International Journal of Heat and Mass Transfer, Vol. 35, pp. 545-57. |
| [18] | Lee, H.R., Chen, T.S. and Armaly, B.F. (1988), “Natural convection along slender vertical cylinder with variable surface temperature”, Journal of heat transfer, Vol. 110, pp. 103-9. |
| [19] | Badruddin, I.A., Zainal, Narayana, P.A.A. and Seetharamu, K.N. (2006), “Heat transfer by radiation and natural convection through a vertical annulas embedded in porous medium”, International Communications in Heat and Mass Transfer, Vol. 33 No. 4, pp. 500-7. |
| [20] | A/K Abu-Hijleh, B., 2003. Optimized use of baffles for reduced natural convection heat transfer from a horizontal cylinder. International journal of thermal sciences, 42(11), pp.1061-1071. |
| [21] | Jafari, M.M., Atefi, G., Khalesi, J., Soleymani, A., 2012. A new conjugate heat transfer method to analyse a 3D steam cooled gas turbine blade with temperature-dependent material properties. Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science, 226(5), pp.1309-1320. |
| [22] | Jafari, M.M., Atefi, G.A., Khalesi, J., 2012. Advances in nonlinear stress analysis of a steam cooled gas turbine blade. Latin American applied research, 42(2), pp.167-175. |
| [23] | Khaleghi, H., Dehkordi, M.A.S., Tousi, A.M., 2016. Role of tip injection in desensitizing the compressor to the tip clearance size. Aerospace Science and Technology, 52, pp.10-17. |
| [24] | Hafezisefat P, Esfahany MN, Jafari M. An experimental and numerical study of heat transfer in jacketed vessels by SiO2 nanofluid. Heat and Mass Transfer. 2017 Jul 1; 53(7): 2395-405. |
| [25] | Moheimani, R., Damadam, M., Nayebi, A. and Dalir, H., 2018, July. Thick-Walled Functionally Graded Material Cylinder under Thermo-Mechanical Loading. In 2018 9th International Conference on Mechanical and Aerospace Engineering (ICMAE) (pp. 505-510). IEEE. |
| [26] | Jafari, M., Razavi, A. and Mirhosseini, M., 2018. Effect of Airfoil Profile on Aerodynamic Performance and Economic Assessment of H-rotor Vertical Axis Wind Turbines. Energy. |
| [27] | Li, K., Wright, J., Modaresahmadi, S., Som, D., Williams, W. and Bird, J.Z., 2017, October. Designing the first stage of a series connected multistage coaxial magnetic gearbox for a wind turbine demonstrator. In Energy Conversion Congress and Exposition (ECCE), 2017 IEEE (pp. 1247-1254). IEEE. |
| [28] | Jafari, M., Razavi, A. and Mirhosseini, M., 2018. Effect of Steady and Quasi-Unsteady Wind on Aerodynamic Performance of H-Rotor Vertical Axis Wind Turbines. Journal of Energy Engineering, 144(6), p.04018065. |
| [29] | Moheimani, R. and Ahmadian, M.T., 2012, November. On Free Vibration of Functionally Graded Euler-Bernoulli Beam Models Based on the Non-Local Theory. In ASME 2012 International Mechanical Engineering Congress and Exposition (pp. 169-173). American Society of Mechanical Engineers. |
| [30] | Modares Ahmadi, S., Ghazavi, M. and Sheikhzad, M., 2015. Dynamic analysis of a rotor supported on ball bearings with waviness and centralizing springs and squeeze lm dampers. International Journal of Engineering, 28, pp.1351-1358. |
| [31] | Jafari, M., Babajani, A., Hafezisefat, P., Mirhosseini, M., Rezania, A., Rosendahl, L., 2018. Numerical simulation of a novel ocean wave energy converter. Energy Procedia, 147, pp.474-481. |
| [32] | Babajani, A., Jafari, M., Hafezisefat, P., Mirhosseini, M., Rezania, A. and Rosendahl, L., 2018. Parametric study of a wave energy converter (Searaser) for Caspian Sea. Energy Procedia, 147, pp.334-342. |
| [33] | Khatibi, M., Zargarzadeh, H. and Barzegaran, M., 2016, September. Power system dynamic model reduction by means of an iterative SVD-Krylov model reduction method. In Innovative Smart Grid Technologies Conference (ISGT), 2016 IEEE Power & Energy Society (pp. 1-6). IEEE. |
| [34] | Khatibi, M. and Ahmed, S., 2018, February. Optimal resilient defense strategy against false data injection attacks on power system state estimation. In 2018 IEEE Power & Energy Society Innovative Smart Grid Technologies Conference (ISGT) (pp. 1-5). IEEE. |
| [35] | Imani, M.H., Yousefpour, K., Ghadi, M.J. and Andani, M.T., 2018, February. Simultaneous presence of wind farm and V2G in security constrained unit commitment problem considering uncertainty of wind generation. In Texas Power and Energy Conference (TPEC), 2018 IEEE (pp. 1-6). IEEE. |
| [36] | Andani, M.T., Pourgharibshahi, H., Ramezani, Z. and Zargarzadeh, H., 2018, February. Controller design for voltage-source converter using LQG/LTR. In Texas Power and Energy Conference (TPEC), 2018 IEEE (pp. 1-6). IEEE. |
| [37] | Poddar S, Ozcan K, Chakraborty P, Ahsani V, Sharma A, Sarkar S. Comparison of Machine Learning Algorithms to Determine Traffic Congestion from Camera Images. 2018. |
| [38] | Sharma A, Ahsani V, Rawat S. Evaluation of Opportunities and Challenges of Using INRIX Data for Real-Time Performance Monitoring and Historical Trend Assessment. |
| [39] | Chakraborty P, Adu-Gyamfi YO, Poddar S, Ahsani V, Sharma A, Sarkar S. Traffic Congestion Detection from Camera Images Using Deep Convolution Neural Networks. 2018. |
| [40] | Esmaili Torshabi, A. and Ghorbanzadeh, L., 2017. A Study on Stereoscopic X-ray Imaging Data Set on the Accuracy of Real-Time Tumor Tracking in External Beam Radiotherapy. Technology in cancer research & treatment, 16(2), pp.167-177. |
| [41] | Ghorbanzadeh, L. and Torshabi, A.E., 2017. An Investigation into the Performance of Adaptive Neuro-Fuzzy Inference System for Brain Tumor Delineation Using Expectation Maximization Cluster Method; a Feasibility Study. Frontiers in Biomedical Technologies, 3(1-2), pp.8-19. |
| [42] | Chouikh, R., Guizani, A., Maâlej, M. and Belghith, A. (1999), “Numerical study of the laminar natural convection flow around an array of two horizontal isothermal cylinders”, International Communications in Heat and Mass Transfer, Vol. 26 No.3, pp. 329-38. |
| [43] | Yousefi, T. and Ashjaee, M. (2007), “Experimental study of natural convection heat transfer from vertical array of isothermal horizontal elliptic cylinders”, Experimental Thermal and Fluid Science, Vol. 32 No. 1, pp. 240-8. |
| [44] | Davani A.A., Kashfi M., Mozafary V., “Experimental analysis of a multistage water desalination system utilizing an evacuated parabolic solar trough with a solar tracker”, arXiv preprint arXiv:1712.06576, (2017). |
| [45] | Davani A., Kashfi M., Mozafary V. “Multi-Effect Solar Driven Distillation Still System”, IR patent 70325, (2011). |
| [46] | Divsalar A, Entesari N., Dods M.N., Prosser R.W., Egolfopoulos F.N., Tsotsis T.T., "A UV Photodecomposition Reactor for Siloxane Removal from Biogas: Modeling Aspects" Chemical Engineering Science (2018). |
| [47] | Gharraei R., Vejdani A., Baheri S., Davani A. “Numerical investigation on the fluid flow and heat transfer of non-Newtonian multiple impinging jets” International Journal of Thermal Sciences, 104 (2016) 257-265. |
| [48] | Kamrani, M., Levitas, V.I., Feng, B., (2017). FEM simulation of large deformation of copper in the quasi constrain high-pressure torsion setup. Materials Science & Engineering A, (705), 219-230. |
| [49] | Kamrani, M., Feng, B., Levitas, V.I., (2018). Modeling of Strain-Induced Phase Transformations under High Pressure and Shear. The Minerals, Metals & Materials Series, https://doi.org/10.1007/978-3-319-76968-4_8. |
| [50] | Feng, B., Levitas, V.I. and Kamrani, M., 2018. Coupled strain-induced alpha to omega phase transformation and plastic flow in zirconium under high pressure torsion in a rotational diamond anvil cell. Materials Science and Engineering: A. |



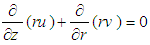
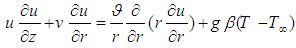

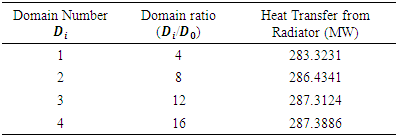
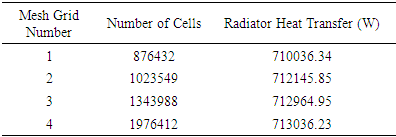
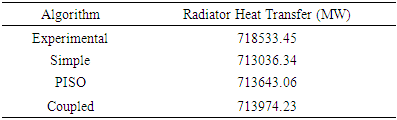
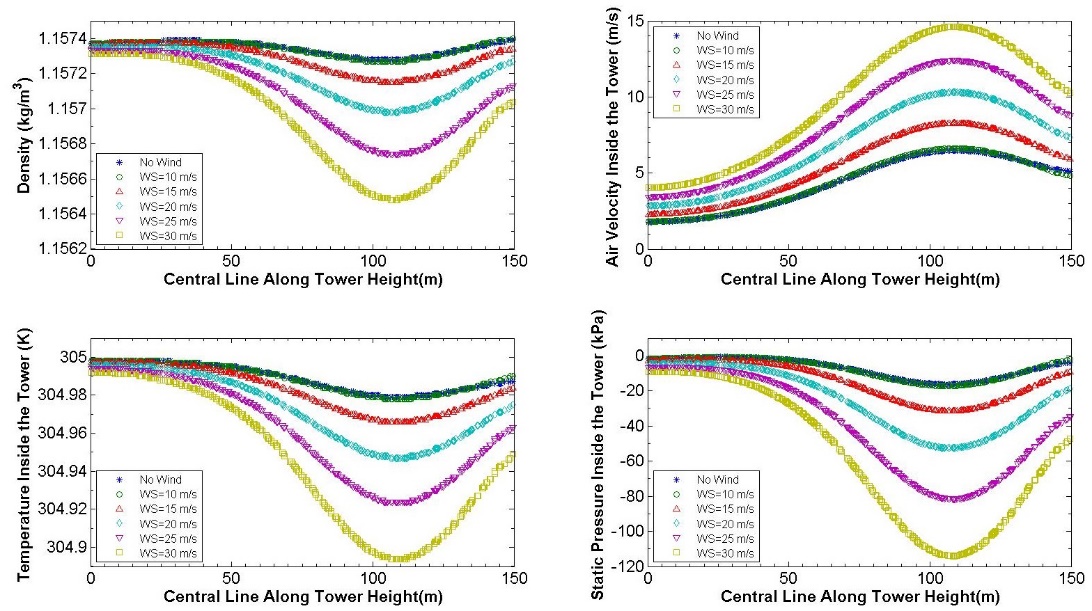
 is kinetic viscosity, u and v are velocity component in r and θ directions, respectively. In natural convection problems, the driving force is caused by density differences which is a function of temperature gradient. Hence, identifying temperature field is essential for solving the velocity field. Consequently, momentum and energy equations should be solved simultaneously. The main flow in this type of heat exchangers is natural convection heat transfer or in other words, buoyancy-driven flow. Principal reason behind natural convection heat transfer process is reduction in density due to increase in temperature; Thus, warmer fluid will be replaced by colder and consequently lighter fluid will move against gravity. For this reason, a function for density variation and change in temperature should be used. Ideal gas law and Boussinesq equation are used for this purpose. Normally when the temperature difference is less than 20 degrees, Boussinesq equation is used to model density variations with temperature change. Using the ideal gas law decreases total run time in simulation, but occasionally has higher error. In this study, both methods are used for comparison to experimental data and results are presented in Table 3.
is kinetic viscosity, u and v are velocity component in r and θ directions, respectively. In natural convection problems, the driving force is caused by density differences which is a function of temperature gradient. Hence, identifying temperature field is essential for solving the velocity field. Consequently, momentum and energy equations should be solved simultaneously. The main flow in this type of heat exchangers is natural convection heat transfer or in other words, buoyancy-driven flow. Principal reason behind natural convection heat transfer process is reduction in density due to increase in temperature; Thus, warmer fluid will be replaced by colder and consequently lighter fluid will move against gravity. For this reason, a function for density variation and change in temperature should be used. Ideal gas law and Boussinesq equation are used for this purpose. Normally when the temperature difference is less than 20 degrees, Boussinesq equation is used to model density variations with temperature change. Using the ideal gas law decreases total run time in simulation, but occasionally has higher error. In this study, both methods are used for comparison to experimental data and results are presented in Table 3.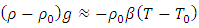
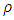 and
and  are density and reference density, g is gravitational acceleration,
are density and reference density, g is gravitational acceleration, 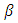 is thermal expansion coefficient,
is thermal expansion coefficient, 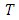 and
and 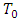 are temperature and reference temperature. Coefficient of thermal expansion in Boussinesq equation is 0.0003331 (1/T) at the room temperature. Ideal gas law is in the form of:
are temperature and reference temperature. Coefficient of thermal expansion in Boussinesq equation is 0.0003331 (1/T) at the room temperature. Ideal gas law is in the form of: 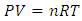
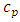 is 1006.43
is 1006.43 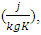 thermal conductivity is 0.0242
thermal conductivity is 0.0242 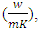 viscosity is 1.79e-5
viscosity is 1.79e-5 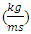 and coefficient of thermal expansion is 0.00333
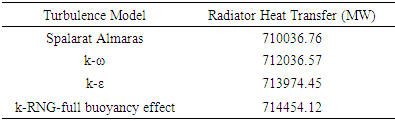
and coefficient of thermal expansion is 0.00333 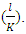 Modeling Turbulence: 4 different turbulence models are used in this study. Results are presented in Table 5.
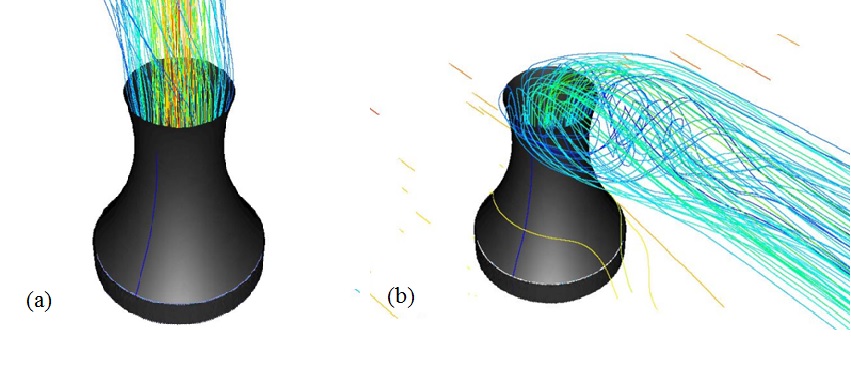
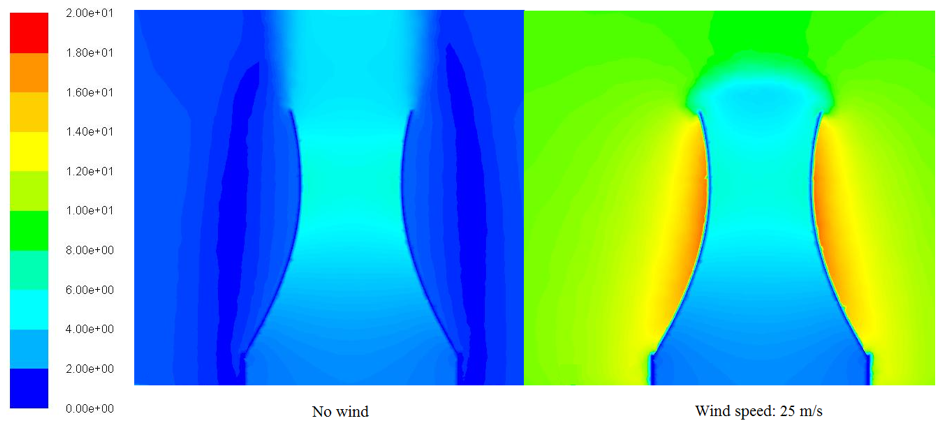
Modeling Turbulence: 4 different turbulence models are used in this study. Results are presented in Table 5. 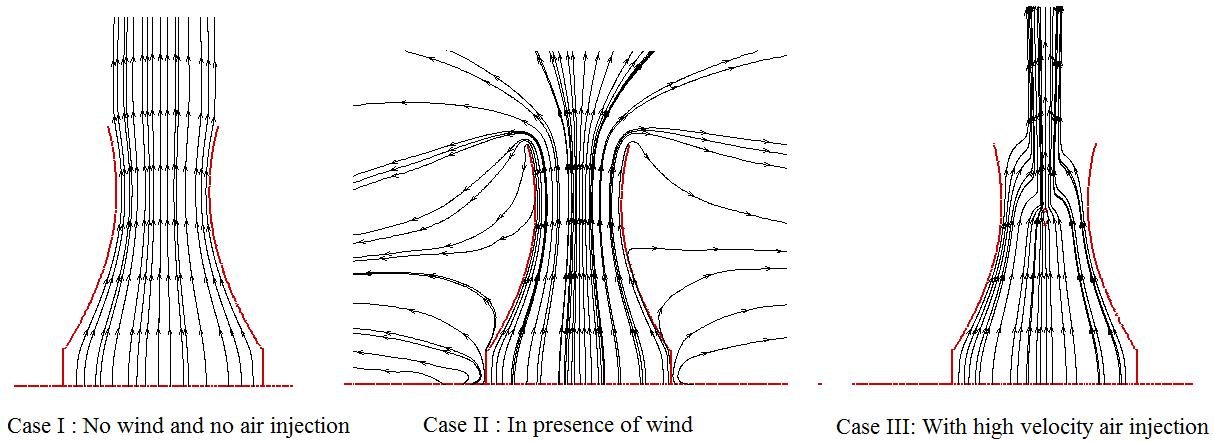
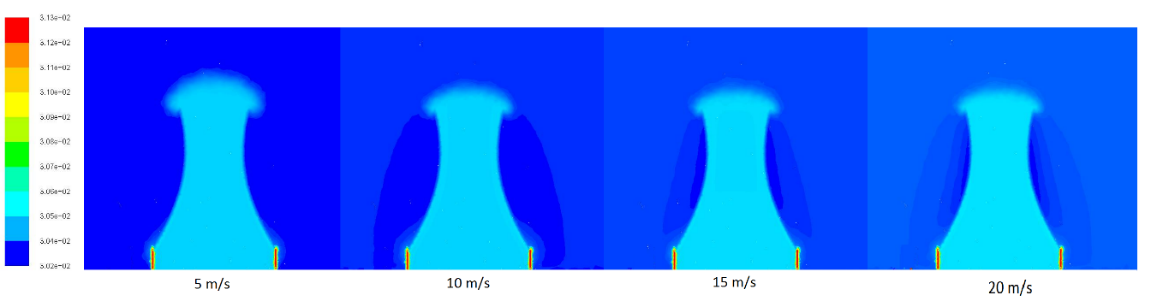
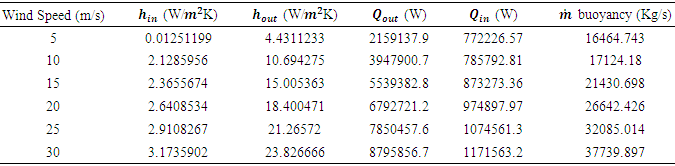
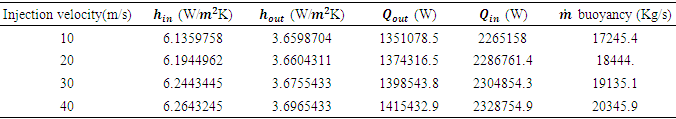
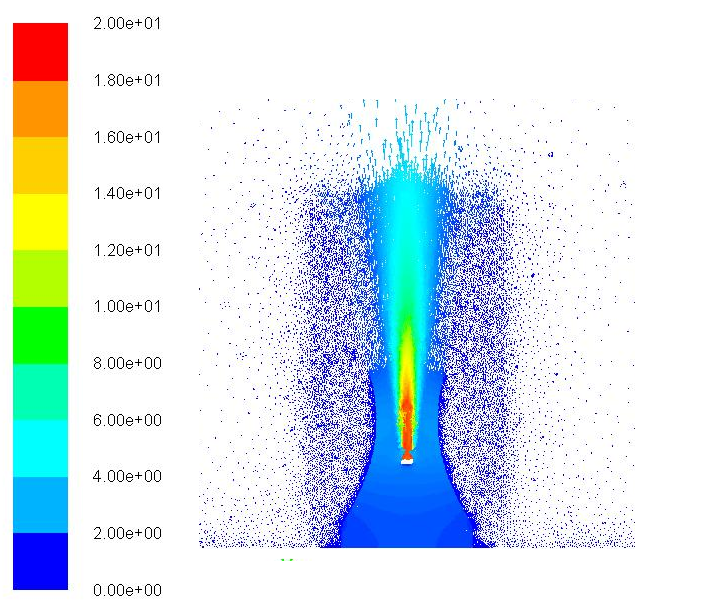
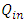 is the amount of heat being transferred from inner surface of the radiators to the air inside the tower. It should be noted that
is the amount of heat being transferred from inner surface of the radiators to the air inside the tower. It should be noted that 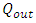 is the amount of heat transfer from radiator’s outer surface to the air outside the tower.
is the amount of heat transfer from radiator’s outer surface to the air outside the tower.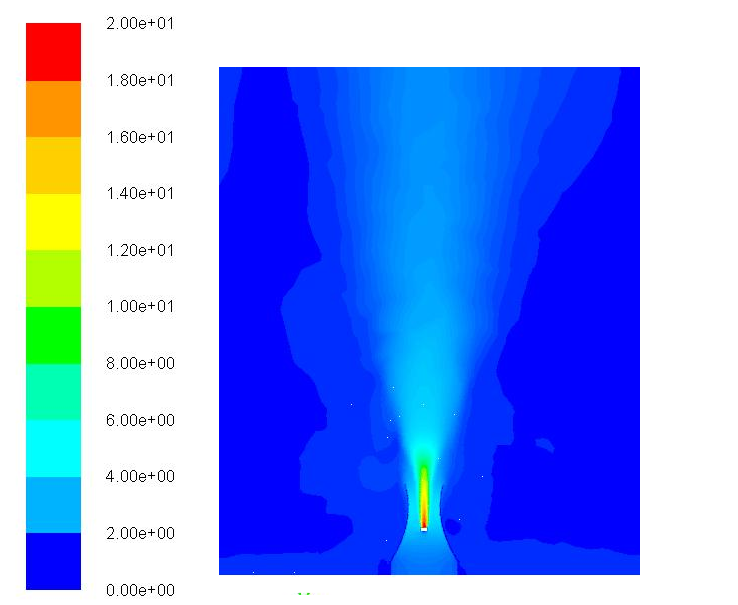

 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML