-
Paper Information
- Paper Submission
-
Journal Information
- About This Journal
- Editorial Board
- Current Issue
- Archive
- Author Guidelines
- Contact Us
American Journal of Condensed Matter Physics
p-ISSN: 2163-1115 e-ISSN: 2163-1123
2018; 8(1): 1-4
doi:10.5923/j.ajcmp.20180801.01

Mechanisms of Electroluminescence in Silicon Nanostructures
Gezahegn Assefa
Department of Physics, Wollo University, Dessie, Ethiopia
Correspondence to: Gezahegn Assefa, Department of Physics, Wollo University, Dessie, Ethiopia.
| Email: |  |
Copyright © 2018 The Author(s). Published by Scientific & Academic Publishing.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/

The mechanism of electroluminescence (EL) in silicon nanostructure (NC) is investigated. The influence of quantum confinement effect (QCE) on the radiative recombination rate and the radiative recombination time is calculated. We found that the radiative recombination rate depends on the diameter d of the spherical crystallites and the radiative recombination rate decreases with decreasing size of the Si-QDs. As a result, the EL emission intensity enhanced due to QCE.
Keywords: Electroluminescence, Quantum confinement effect, Radiative recombination, Quantum dots
Cite this paper: Gezahegn Assefa, Mechanisms of Electroluminescence in Silicon Nanostructures, American Journal of Condensed Matter Physics, Vol. 8 No. 1, 2018, pp. 1-4. doi: 10.5923/j.ajcmp.20180801.01.
Article Outline
1. Introduction
- Semiconductor quantum dots (QDs) are the leading materials regarding high-density electronic devices. QDs are nanocrystals with nanometric size (ranging in size from 2 to 10 nm in diameter) provide a nearly zero-dimensional system, where carriers are confined in all spatial directions [1]. They have attracted much attention due to their applications in light emitting diodes (LED), biological imaging etc [2, 3]. Silicon nanostructure (Si-ns) is one of the candidates for photonic material. The reduced size to nanoscale results in new quantum phenomena that yield some modification in material properties because of quantum confinement effects of electrons and holes in the material [4]. Electroluminescence (EL) is a phenomenon of light emitting in certain materials such as semiconductors due to an electric field or passage of an electric current through the semiconductor. It occurs due to radiative recombination of excited electrons with holes and as a result photon are released. EL is an optical property which depends on the nature of the material. In silicon quantum dots the EL depends on the size and the EL intensity is higher for smaller size [5]. The optical properties of silicon quantum dots specially the EL is affected by different factors such as the preparation methods and conditions (laser ablation, deposition temperature, annealing, and oxidation process etc) [6]. In addition to these factors, the QCE is the one which affects the optical phenomena in nano range systems that can changes the EL spectra [4].One of the important parameter that determines the optical and transport properties of the of QDs is the energy gap between the highest occupied and lowest unoccupied molecular orbitals (HOMO-LUMO) levels. In EL phenomena carriers-excitation is due to the applied electric field. When an electron in the HOMO gets enough energy, it can jump to the LUMO and the emission happens when the charge carriers (electrons and holes) recombine. The electron-hole recombination time (life time of exciton) is strongly dependent on the particle size [7]. Recently, there has been great interest in developing quantum dot (QD) light emitting devices (LED) since the work of Colvin et al [8] for the application of displays with quality images and low power consumption. The fabrication of QD-LED based display by Kim et al proves the feasibility of this type of display [9]. Due to their high photoluminescence quantum yields, QD-LED also used for solid state lighting applications. The fabrication of different colored QD-LED by Anikeeva et al demonstrated the possibility of EL in the visible wavelength range [10].In this paper, we present a theoretical model for the calculation the radiative recombination rate and life time for Si-QDs EL phenomena. The second section discusses the model of optical transition and formulation of the problem. The results of our calculation are presented and discussed in section three and section four gives the conclusion.
2. Model of the System
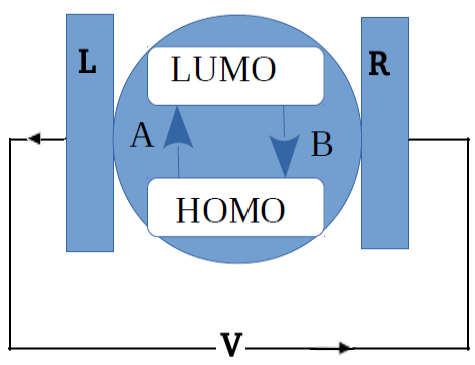
- In order to formulate and describe the EL phenomena from the QD structures, we consider the QDs as ensembles of nanometric size spherical particles, having a well-defined size distribution. We assume both excitation and emission process for electrons takes place inside the QDs. The QDs system will have a quasi-direct band structure owing to the quantum confinement effect (QCE) and the valance and conduction bands are represented as the HOMO and LUMO respectively (fig. 1).
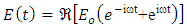 | (1) |
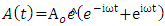 is the polarization unit vector. Then Hamiltonian reads as:
is the polarization unit vector. Then Hamiltonian reads as: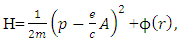 | (2) |
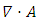 and
and 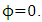 Since the operator p as an operator does not commute with A and for the purpose of calculating linear optical properties we neglect the term
Since the operator p as an operator does not commute with A and for the purpose of calculating linear optical properties we neglect the term 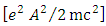 from the expansion of
from the expansion of 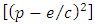 . Considering A as a classical vector, the Hamiltonian can be written as
. Considering A as a classical vector, the Hamiltonian can be written as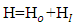 | (3) |
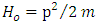 is the unperturbed Hamiltonian and the interaction Hamiltonian
is the unperturbed Hamiltonian and the interaction Hamiltonian 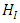 is given by
is given by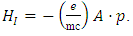 | (4) |
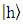 with energy
with energy 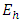 and wavevector
and wavevector 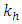 to the LUMO state
to the LUMO state 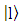 with corresponding energy
with corresponding energy 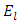 and wavevector
and wavevector 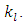 We also assume that the system at
We also assume that the system at 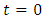 is in its ground state and the transition between HOMO-LUMO state of N identical atoms is described by the Bloch wave functions of the form
is in its ground state and the transition between HOMO-LUMO state of N identical atoms is described by the Bloch wave functions of the form 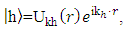 | (5) |
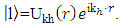 | (6) |
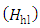 of the perturbation
of the perturbation 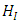 is given by
is given by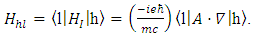 | (7) |
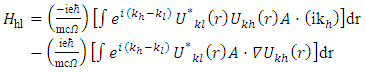 | (8) |
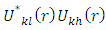 , vanishes because
, vanishes because 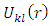 and
and 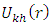 are orthogonal and for direct transition
are orthogonal and for direct transition 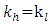 then equation (10) can be written as
then equation (10) can be written as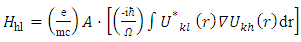 | (9) |
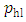
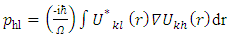 | (10) |
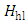 then becomes
then becomes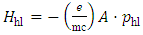 | (11) |
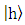 and final state
and final state 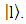 The electric field is the time dependent perturbation which induces this event. The radiative transition rate (R) for energy emission and absorption per unit time obtained by substituting equation (11) into the Fermi Golden Rule:
The electric field is the time dependent perturbation which induces this event. The radiative transition rate (R) for energy emission and absorption per unit time obtained by substituting equation (11) into the Fermi Golden Rule: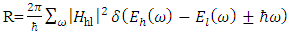 | (12) |
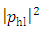 . Equation (12) can then be simplified to read
. Equation (12) can then be simplified to read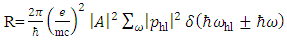 | (13) |
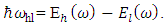 The oscillator strength
The oscillator strength 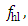 which is the measure of the radiative probability of a quantum mechanical transition between two atomic levels (HOMO and LUMO), is related to the momentum matrix element as
which is the measure of the radiative probability of a quantum mechanical transition between two atomic levels (HOMO and LUMO), is related to the momentum matrix element as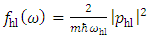 | (14) |
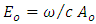 and substituting equation (14) in to equation (15), the radiative recombination rate becomes
and substituting equation (14) in to equation (15), the radiative recombination rate becomes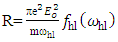 | (15) |
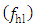 with the crystallite diameter d is usually assumed to be of the form [11]
with the crystallite diameter d is usually assumed to be of the form [11]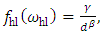 | (16) |
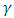 is arbitrary proportionality constant and the power exponent
is arbitrary proportionality constant and the power exponent 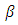 depends on the material property as well as range of the crystallite size being used, and the values of
depends on the material property as well as range of the crystallite size being used, and the values of 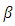 had been taken to be 5 by Sanders et al [12] and 6 by Khurgin et al [13].Assuming each atom in the quantum dots contains at least one excited carriers due to the applied external electric field, the number of excited carriers
had been taken to be 5 by Sanders et al [12] and 6 by Khurgin et al [13].Assuming each atom in the quantum dots contains at least one excited carriers due to the applied external electric field, the number of excited carriers 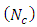 in the QDs is proportional to its volume. i.e
in the QDs is proportional to its volume. i.e 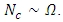 For spherical QDs of diameter d, the volume is
For spherical QDs of diameter d, the volume is 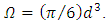 Combining these assumption and facts we arrive at
Combining these assumption and facts we arrive at 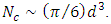 For every transition in the QD, the oscillator strength is proportional to
For every transition in the QD, the oscillator strength is proportional to 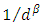 (equation 16), so the total oscillator strength over the volume is proportional to
(equation 16), so the total oscillator strength over the volume is proportional to 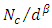 . Therefore, for equation (16) we have
. Therefore, for equation (16) we have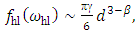 | (17) |
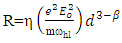 | (18) |
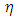 is a proportionality constant parameter. The radiative recombination time
is a proportionality constant parameter. The radiative recombination time 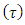 is the inverse of the radiative recombination rate
is the inverse of the radiative recombination rate 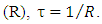
3. Results and Discussion
- In this section, we present the main results that we obtained based on model calculation in section 2. Various parameters are considered here. The dot size is taken in the range 1nm-10nm. The parameter
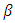 is taken in the range 5-6. It is known that the band gap Si-QD is size dependent. It is reported recently that the band gap of Si QD ensemble, follows a fit with equation,
is taken in the range 5-6. It is known that the band gap Si-QD is size dependent. It is reported recently that the band gap of Si QD ensemble, follows a fit with equation, 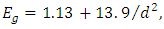 where d is the dots diameter [14]. Even if the band gap depends on size for our numerical calculation we use
where d is the dots diameter [14]. Even if the band gap depends on size for our numerical calculation we use 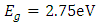 [15]. The applied electric field
[15]. The applied electric field 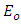 is taken to be
is taken to be 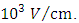 As can be seen from figure 2, the radiative recombination rate (R) of silicon quantum dots increases as the size of Si-QDs decreases and we also observe that R increases with values of
As can be seen from figure 2, the radiative recombination rate (R) of silicon quantum dots increases as the size of Si-QDs decreases and we also observe that R increases with values of 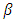 . To obtain an insight of the effect of size on the radiative recombination rates and hence on the EL spectral profile of the system, both excitation and emission processes for electron hole pairs occur in side the Si-QDs are considered. The EL emission in visible range is observed when optical transitions between HOMO-LUMO gaps are induced by the electric field.
. To obtain an insight of the effect of size on the radiative recombination rates and hence on the EL spectral profile of the system, both excitation and emission processes for electron hole pairs occur in side the Si-QDs are considered. The EL emission in visible range is observed when optical transitions between HOMO-LUMO gaps are induced by the electric field.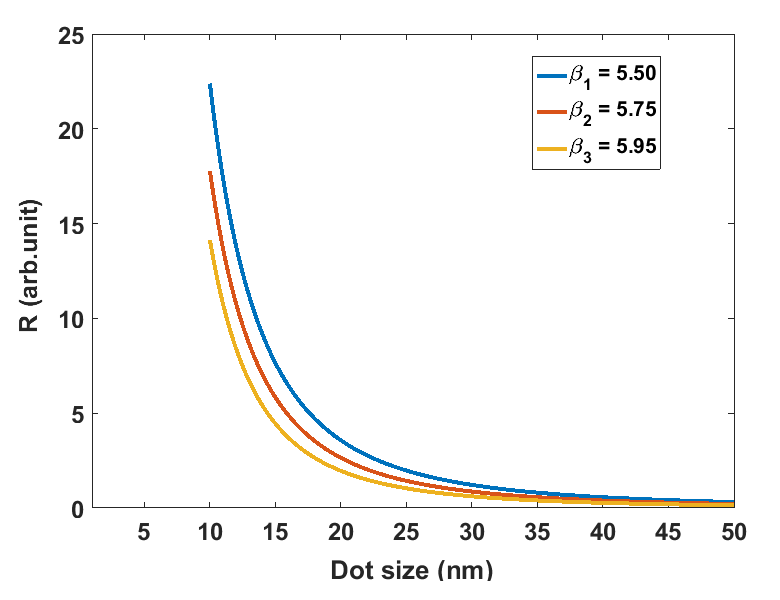 | Figure 2. Variation of radiative recombination rate with size of the quantum dot |
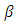 indicates the EL depends on the properties of the Si-QDs.
indicates the EL depends on the properties of the Si-QDs. 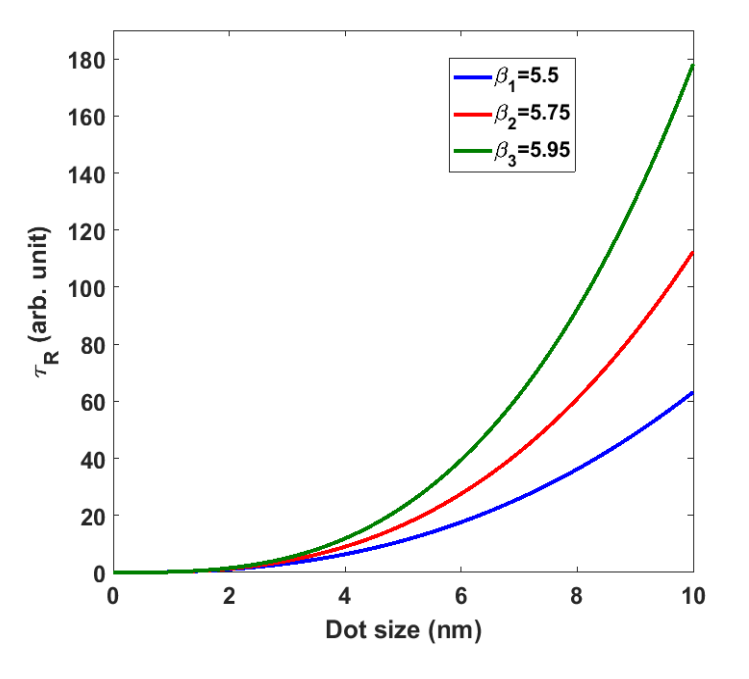 | Figure 3. Size dependent radiative life time |
4. Conclusions
- In conclusion, the effect of the QCE on the EL of Si-QDs were investigated theoretically. Enhanced radiative recombination rate is observed in small-sized Si-QDs. It is also seen that the recombination life time decreases as the Si-QDs size decreases owing to the QCE.
ACKNOWLEDGEMENTS
- The author would like to thank Wollo university college of Natural Science.
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML