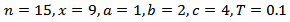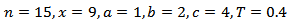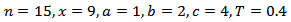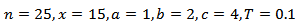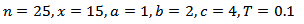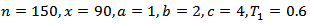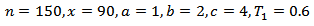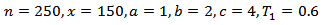| [1] | Adebola, F.B. and Adepetun, A.O. (2011): A new Tripartite Randomized Response Technique. Journal of the Nigerian Association of Mathematical Physics, Volume 19: pp 119-122. |
| [2] | Adebola, F.B. and Adepetun, A.O. (2012): On a Numerical Comparison of the Proposed Randomized Response Technique with Hussain and Shabbir. Journal of the Nigerian Association of Mathematical Physics, Volume 20: pp 379-384. |
| [3] | Adebola, F.B. and Adepetun, A.O. (2012): On a Qualitative Comparison of the Proposed Randomized Response Technique with Hussain and Shabbir (2007). International Journal of Mathematical Theory and Modeling, Volume 2:pp 61-67. |
| [4] | Adepetun, A.O. and Adebola, F.B. (2014): On the Relative Efficiency of the Proposed Reparametized Randomized Response Model. International Journal of Mathematical Theory and Modeling, Volume 4: pp 58-67. |
| [5] | Barabesi, L., Marcheselli, M. (2006): A practical implementation and Bayesian estimation in Franklin’s randomized response procedure. Communication in Statistics- Simulation and Computation, 35, 365-573. |
| [6] | Barabesi, L., Marcheselli, M. (2010): Bayesian estimation of proportion and sensitivity level in randomized response procedures. Metrika, 72, 75-88. |
| [7] | Christofides, T.C. (2003): A generalized randomized response technique. Metrika, 57, 195-200. |
| [8] | Folsom, R. E., Greenberg, B. G., Horvitz, D. G., Abernathy, J. R. (1973): The two alternate question randomized response model for human surveys. Journal of the American Statistical Association, 68, 525-530. |
| [9] | Greenberg, B., Abul-Ela, A., Simmons, W., Horvitz, D. (1969): The unrelated question randomized response: theoretical framework. Journal of the American Statistical Association, 64, 529-539. |
| [10] | Hussain, Z., Shabbir, J. (2009a): Bayesian estimation of population proportion of a sensitive characteristic using simple Beta prior. Pakistan Journal of Statistics, 25(1), 27-35. |
| [11] | Hussain, Z., Shabbir, J. (2009b): Bayesian Estimation of population proportion in Kim and Warde (2005) Mixed Randomized Response using Mixed Prior Distribution. Journal of probability and Statistical Sciences, 7(1), 71-80. |
| [12] | Hussain, Z., Shabbir, J. (2012): Bayesian Estimation of population proportion in Kim and Warde Mixed Randomized Response Technique. Electronic Journal of Applied Statistical Analysis, Vol. 5, Issue 2, 213 – 225. |
| [13] | Hussain, Z. Shabbir, J., Riaz, M. (2011): Bayesian Estimation Using Warner’s randomized Response Model Through Simple and Mixture Prior Distributions. Communications in Statistics- Simulation and Computation, 40(1), 159-176. |
| [14] | Kim, J. M., Tebbs, J. M., An, S. W. (2006): Extension of Mangat’s randomized response model. Journal of Statistical Planning and Inference, 36(4), 1554-1567. |
| [15] | Kim, J.M. and Warde, D.W. (2004): A stratified Warner’s Randomized Response Model. J. Statist. Plann. Inference, 120(1-2), 155-165. |
| [16] | Mangat, N.S. (1994): An improved randomized response strategy. J. Roy. Statist. Soc. Ser. B, 56(1), 93-95. |
| [17] | Migon, H., Tachibana, V. (1997): Bayesian approximations in randomized response models. Computational Statistics and Data Analysis, 24, 401-409. |
| [18] | O’Hagan, A. (1987): Bayes linear estimators for randomized response models. Journal of the American Statistical Association, 82, 580-585. |
| [19] | Oh, M. (1994): Bayesian analysis of randomized response models: a Gibbs sampling approach. Journal of the Korean Statistical Society, 23, 463-482. |
| [20] | Spurrier, J., Padgett, W. (1980): The application of Bayesian techniques in randomized response. Sociological Methodology, 11, 533-544. |
| [21] | Unnikrishnan, N., Kunte, S. (1999): Bayesian analysis for randomized response models. Sankhya, B, 61, 422-432. |
| [22] | Warner, S.L. (1965): Randomized Response: a survey technique for eliminating evasive answer bias. J. Amer. Statist. Assoc., 60, 63-69. |
| [23] | Winkler, R., Franklin, L. (1979): Warner’s randomized response model: A Bayesian approach. Journal of the American Statistical Association, 74, 207-214. |

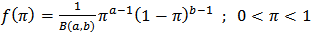
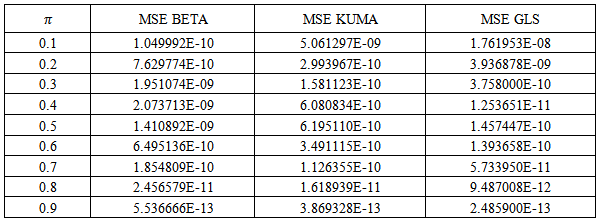
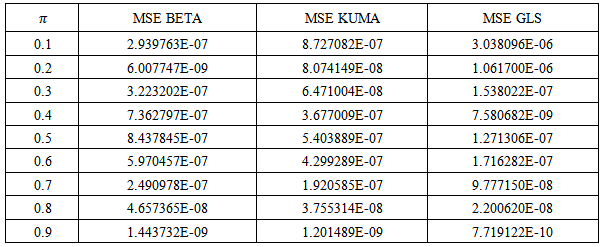
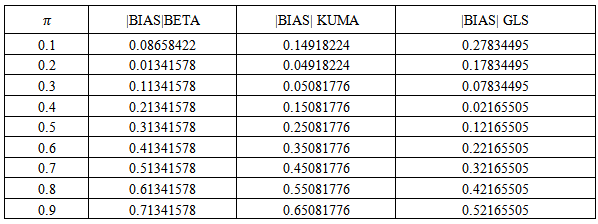
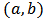 are the shape parameters of the distribution and
are the shape parameters of the distribution and 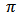 is the population proportion of respondents possessing the stigmatized attribute.By letting
is the population proportion of respondents possessing the stigmatized attribute.By letting 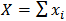 denotes the total number of the yes response in a sample of size n drawn from the population with simple random sampling with replacement (srswr). The conditional distribution of
denotes the total number of the yes response in a sample of size n drawn from the population with simple random sampling with replacement (srswr). The conditional distribution of 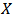 given
given 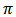 is
is 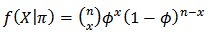
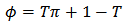 is the probability of “yes response” in a sample of size n and
is the probability of “yes response” in a sample of size n and 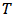 and
and 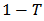 are the pre-assigned probabilities respectively.Then
are the pre-assigned probabilities respectively.Then 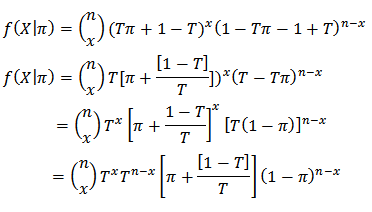 Let
Let 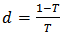
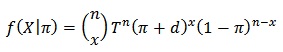 Using binomial series expansion
Using binomial series expansion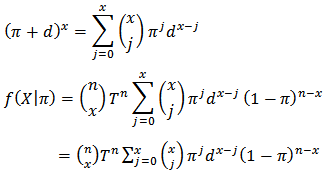
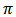 and X was derived as follows
and X was derived as follows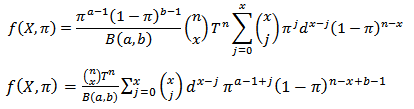
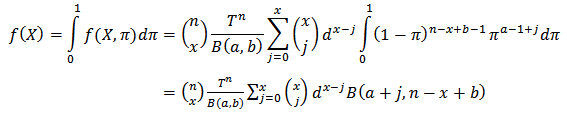
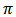 given X was
given X was 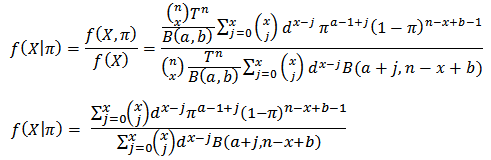
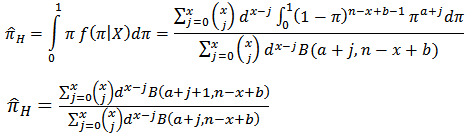
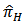 corresponding to the sample of size n was given by
corresponding to the sample of size n was given by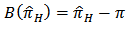
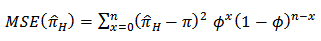
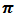 Using Kumaraswamy Prior
Using Kumaraswamy Prior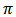 is given as
is given as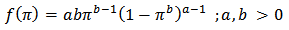
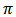 and X with Kumaraswamy Prior is as follows
and X with Kumaraswamy Prior is as follows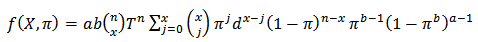
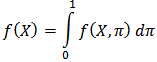 Recall that
Recall that 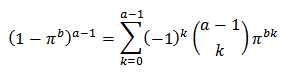 Then
Then 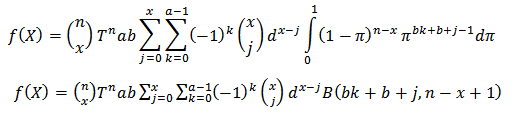
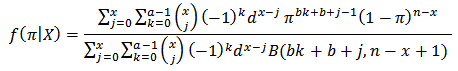 Thus the posterior mean is
Thus the posterior mean is 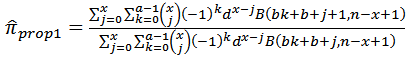
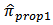 is computed as
is computed as 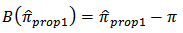
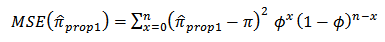
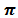 Using Generalised Beta Prior
Using Generalised Beta Prior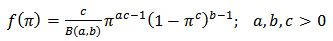
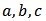 are the shape parameters of the prior distribution as given in formula (3.7)We recall from Binomial series expansion that
are the shape parameters of the prior distribution as given in formula (3.7)We recall from Binomial series expansion that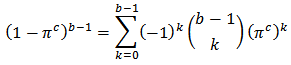 So that
So that 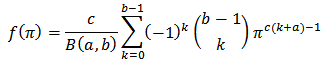 The joint density function of
The joint density function of 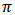 and X with generalized beta prior is
and X with generalized beta prior is 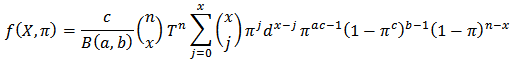 which simplify to
which simplify to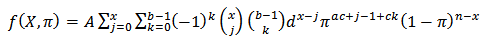
 The marginal probability density function is
The marginal probability density function is 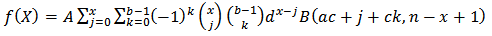
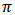 given X is
given X is 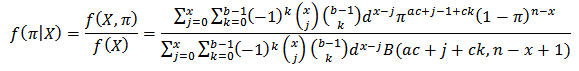 The posterior mean which is the Bayes estimator is found by using
The posterior mean which is the Bayes estimator is found by using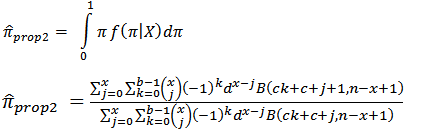
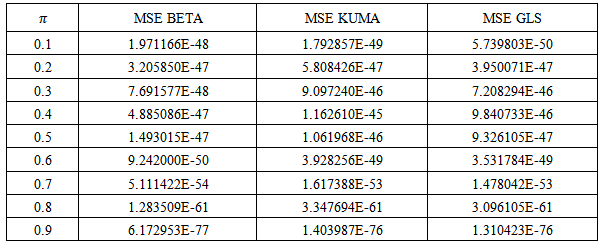
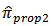 is
is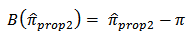
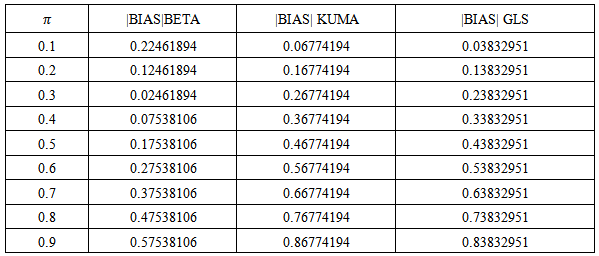
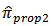 is
is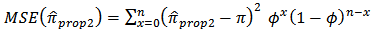
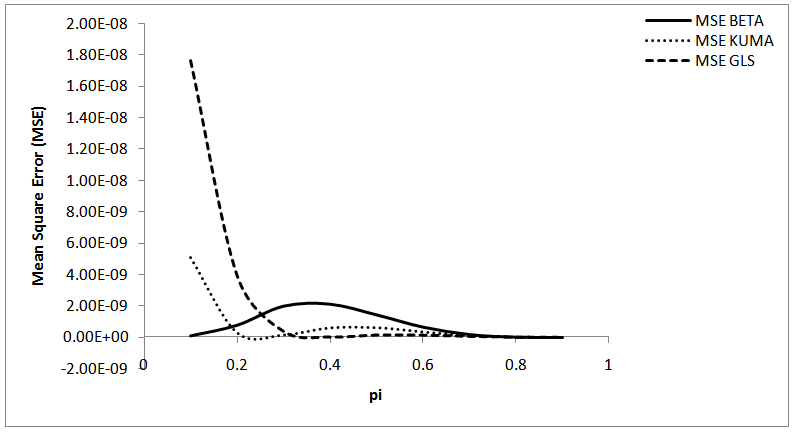
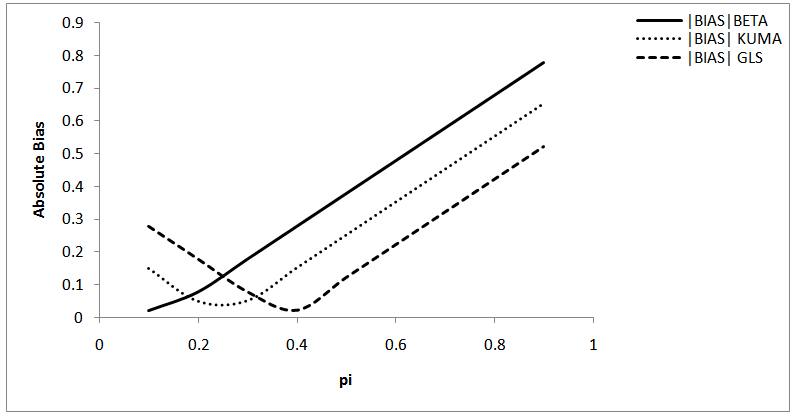
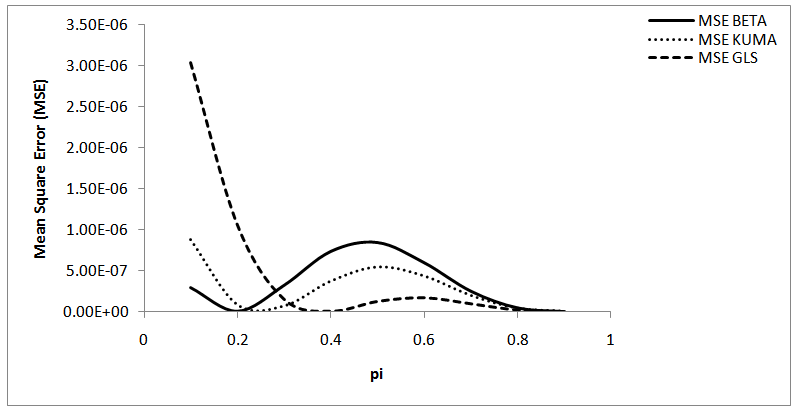
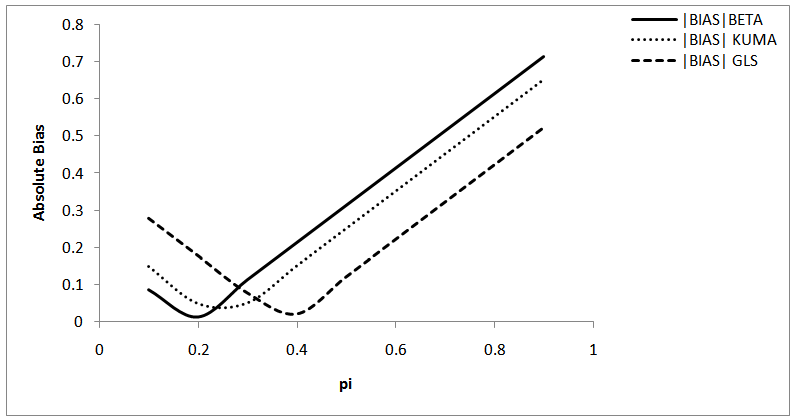
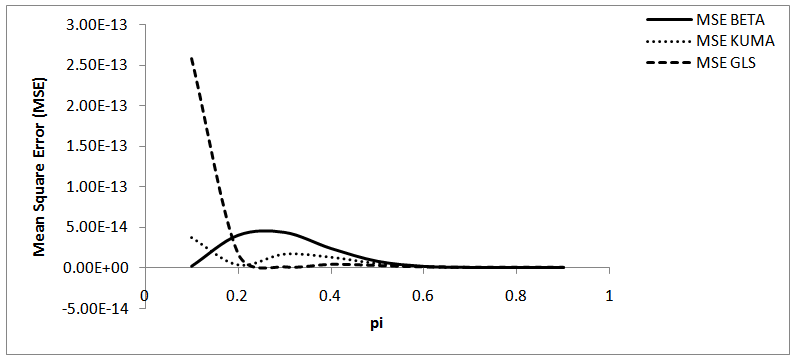
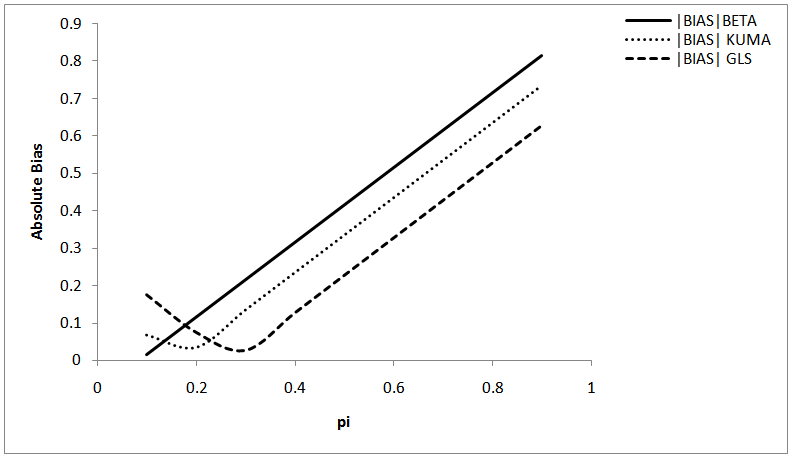
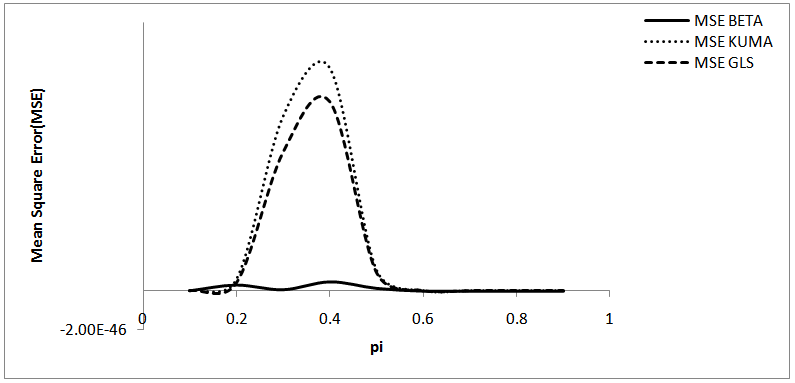
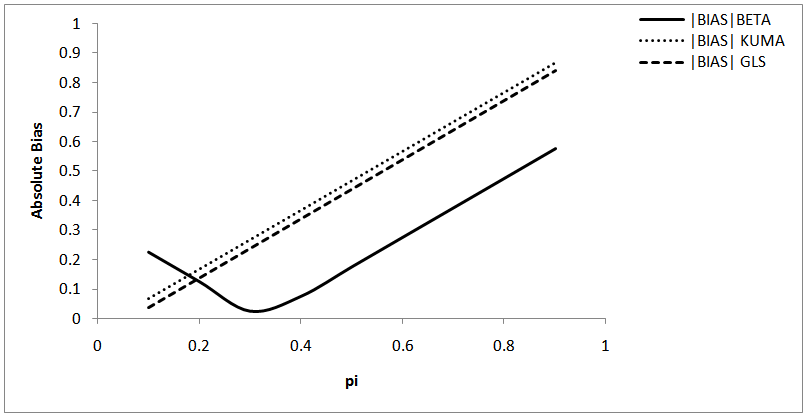
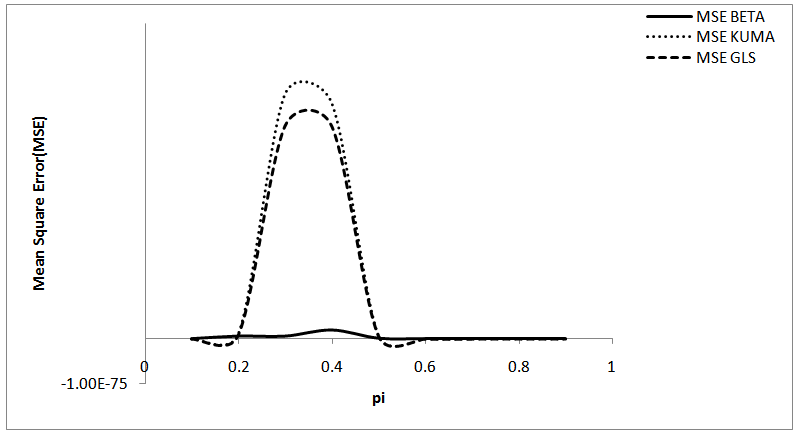
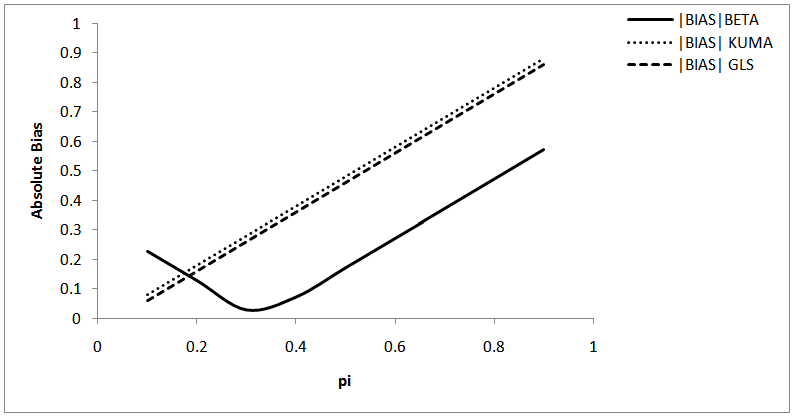
 Abstract
Abstract Reference
Reference Full-Text PDF
Full-Text PDF Full-text HTML
Full-text HTML